Research Article

Revealing the structure of information flows discriminates similar animal social behaviors

Arizona State University, School of Earth and Space Exploration, United States
Arizona State University, School of Life Sciences, United States
Okinawa Institute of Science & Technology Graduate University, Onna-son, Japan
Arizona State University, ASU–SFI Center for Biosocial Complex Systems, United States
Arizona State University, Beyond Center for Fundamental Concepts in Science, United States
Arizona State University, School of Computing, Informatics, and Decision Systems Engineering, United States
Arizona State University, School of Sustainability, United States

Jul 30, 2020

https://doi.org/10.7554/eLife.55395

Open access
Copyright information

Figures
Tables
Additional files

7 figures, 3 tables and 1 additional file

Figures

Figure 1

Download asset Open asset

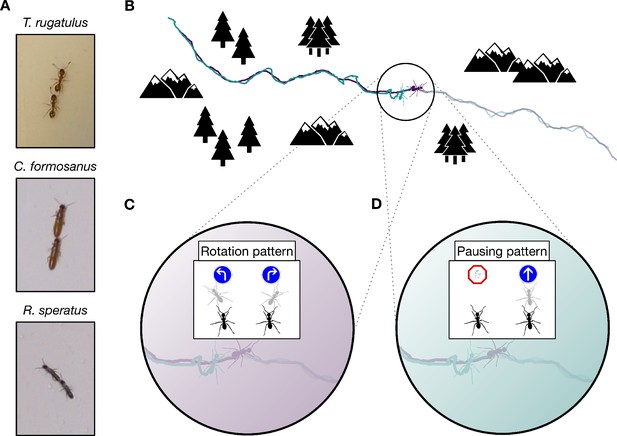
Tandem run recruitment by ants and termites.

(A) Tandem running pairs of the ant species *T. rugatulus* and the termite species *C. formosanus* and *R. speratus*. (B) A sampled tandem run trajectory within an idealized environment. The encoding schemas used to discretise spatial trajectories of each ant and each termite on the basis of (C) the rotation pattern and (D) the pausing pattern.

Figure 2

Download asset Open asset

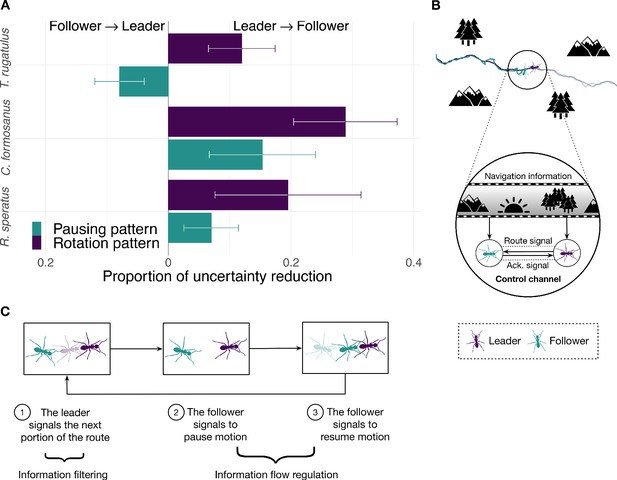
Information flow during tandem run recruitment.

(A) The predominant direction of predictive information given by the proportion of uncertainty reduction explained by the interaction between leader and follower (mean normalized transfer entropy and standard error). (B) A schematic illustration and (C) a mechanistic illustration of the regulation of information flow in ants' tandem runs. Source data of predictive information are available in Figure 2—source data 1.

Figure 2—source data 1 Conditional entropy and transfer entropy for selected parameter configurations.: https://cdn.elifesciences.org/articles/55395/elife-55395-fig2-data1-v1.csv
Download elife-55395-fig2-data1-v1.csv

Figure 3

Download asset Open asset

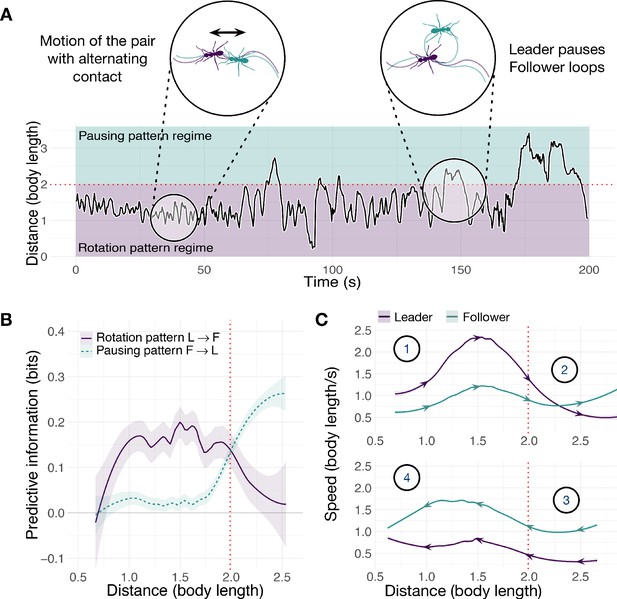
Spatiotemporal dynamics of the ant *T. rugatulus*.

(A) Distance between the centroids of runners as a function of time. (B) Average predictive information (measured with local transfer entropy) as a function of the distance between centroids of runners (smoothed conditional means with LOESS smoothing and 0.3 span). (C) Average speed of leader and follower as a function of the distance between their centroids for increasing and decreasing distance. Purple represents the leader, green represents the follower. Source data of predictive information are available in Figure 3—source data 1.

Figure 3—source data 1 Average local transfer entropy as a function of distance between runners.: https://cdn.elifesciences.org/articles/55395/elife-55395-fig3-data1-v1.csv
Download elife-55395-fig3-data1-v1.csv

Figure 4 with 1 supplement

Download asset Open asset

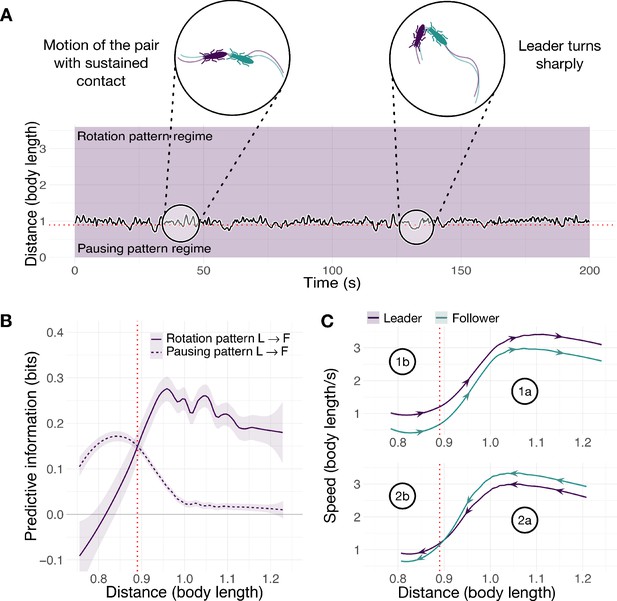
Spatiotemporal dynamics of the termite *C. formosanus*.

(A) Distance between the centroids of runners as a function of time. (B) Average predictive information (measured with local transfer entropy) as a function of the distance between centroids of runners (smoothed conditional means with LOESS smoothing and 0.3 span). (C) Average speed of leader and follower as a function of the distance between their centroids for increasing and decreasing distance. Purple represents the leader, green represents the follower. See also Figure 4—figure supplement 1. Source data of predictive information are available in Figure 4—source data 1 and Figure 4—source data 2.

Figure 4—source data 1 Average local transfer entropy as a function of distance between runners for C. formosanus.: https://cdn.elifesciences.org/articles/55395/elife-55395-fig4-data1-v1.csv
Download elife-55395-fig4-data1-v1.csv
Figure 4—source data 2 Average local transfer entropy as a function of distance between runners for R. speratus.: https://cdn.elifesciences.org/articles/55395/elife-55395-fig4-data2-v1.csv
Download elife-55395-fig4-data2-v1.csv

Figure 4—figure supplement 1

Download asset Open asset

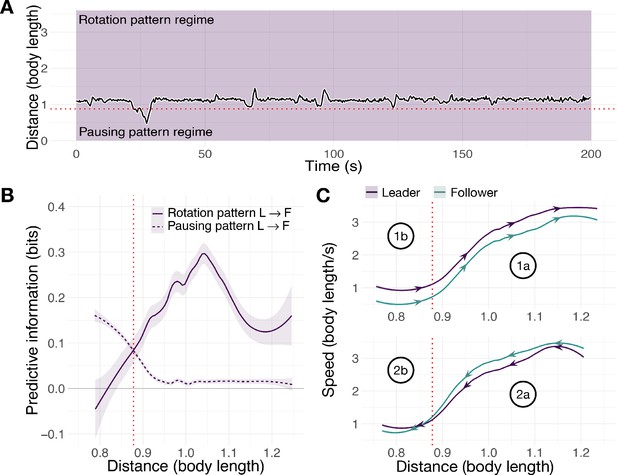
Spatiotemporal dynamics of the termite *R. speratus*.

(A) Distance between the centroids of runners as a function of time. (B) Average predictive information (measured with local transfer entropy) as a function of the distance between centroids of runners (smoothed conditional means with LOESS smoothing and 0.3 span). (C) Average speed of leader and follower as a function of the distance between their centroids for increasing and decreasing distance. Purple represents the leader, green represents the follower.

Figure 5

Download asset Open asset

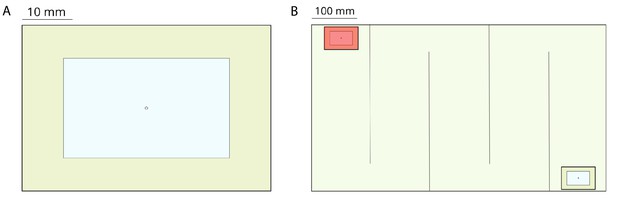
Experimental setup for experiments performed with the ant *T. rugatulus*.

(A) Nest architecture with the entrance in the center of the roof. (B) Experimental arena partitioned into a corridor with the old nest (bottom right) and the new nest (top left) positioned at the extremities.

Figure 6 with 2 supplements

Download asset Open asset

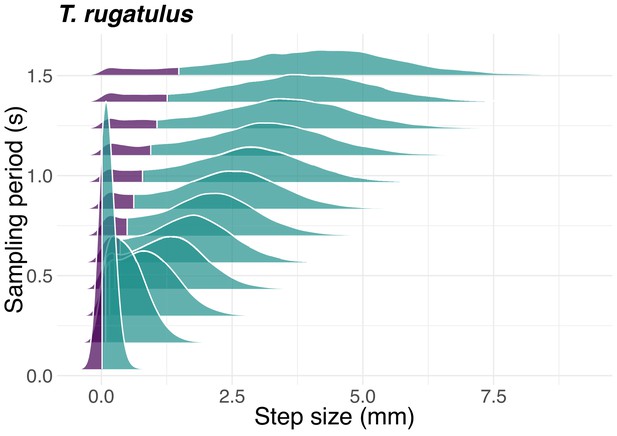
Step-size distribution as a function of sampling period.

Probability density function of the step size for the ant *T. rugatulus* as a function of the sampling period. Purple represents the 10% probability mass used to define the pause state; green represents the remaining 90% of the probability mass defining the motion state. See also Figure 6—figure supplement 1 for the termite *C. formosanus* and Figure 6—figure supplement 2 for *R. speratus*.

Figure 6—figure supplement 1

Download asset Open asset

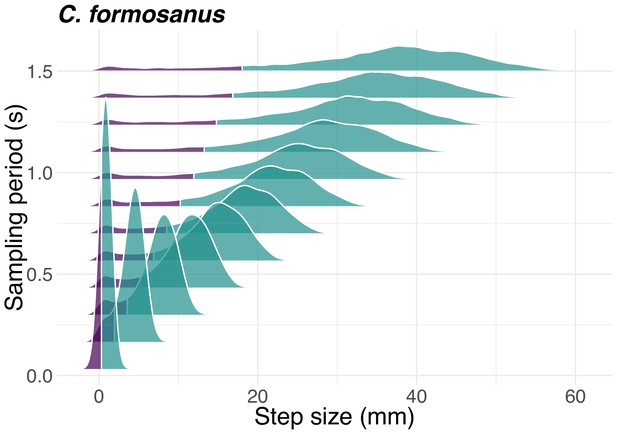
Step-size distribution as a function of sampling period.

Probability density function of the step size for the termite *C. formosanus* as a function of the sampling period. Purple represents the 10% probability mass used to define the pause state; Green represents the remaining 90% of the probability mass defining the motion state.

Figure 6—figure supplement 2

Download asset Open asset

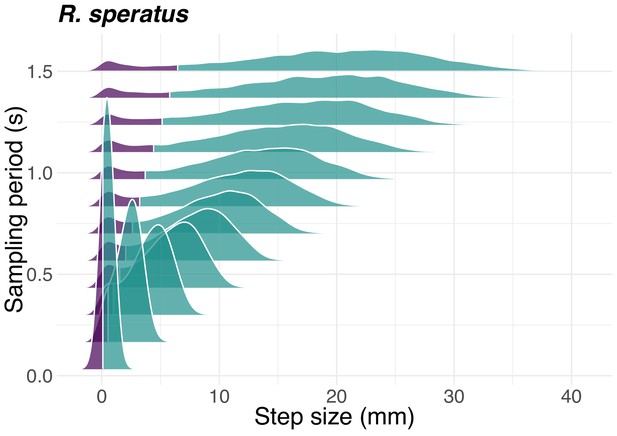
Step-size distribution as a function of sampling period.

Probability density function of the step size for the termite *R. speratus* as a function of the sampling period. Purple represents the 10% probability mass used to define the pause state; green represents the remaining 90% of the probability mass defining the motion state.

Figure 7 with 3 supplements

Download asset Open asset

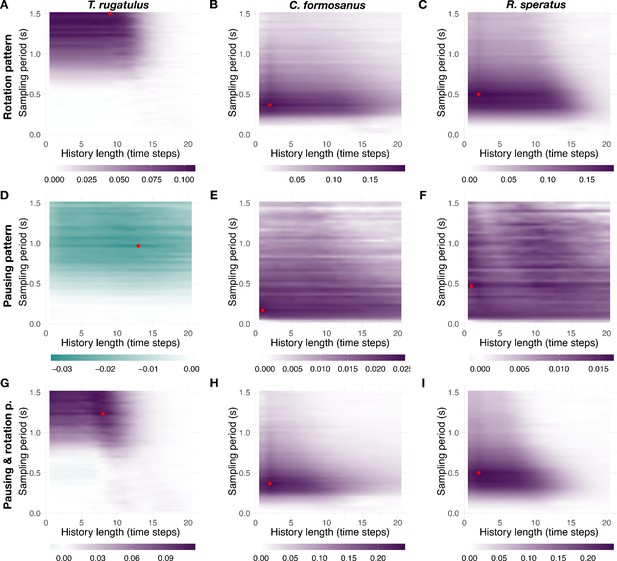
Landscape of net information transfer.

Net transfer entropy (bits) as a function of the sampling period and of the history length. (A), (B), and (C) show the results for the rotation pattern, (D), (E), and (F) show the results for the pausing pattern, and (G), (H), and (I) show the results for the compound pausing and rotation pattern. The first, second, and third columns show the results, respectively, for *T. rugatulus*, *C. formosanus*, and *R. speratus*. Colors indicate the intensity and predominant direction of information transfer (purple, from leader to follower; green, from follower to leader); the red diamond symbol indicates the configuration with maximum magnitude. See also Figure 7—figure supplements 1–3. Source data of net transfer entropy are available in Figure 7—source data 1.

Figure 7—source data 1 Transfer entropy as a function of the sampling period and of the history length.: https://cdn.elifesciences.org/articles/55395/elife-55395-fig7-data1-v1.csv
Download elife-55395-fig7-data1-v1.csv

Figure 7—figure supplement 1

Download asset Open asset

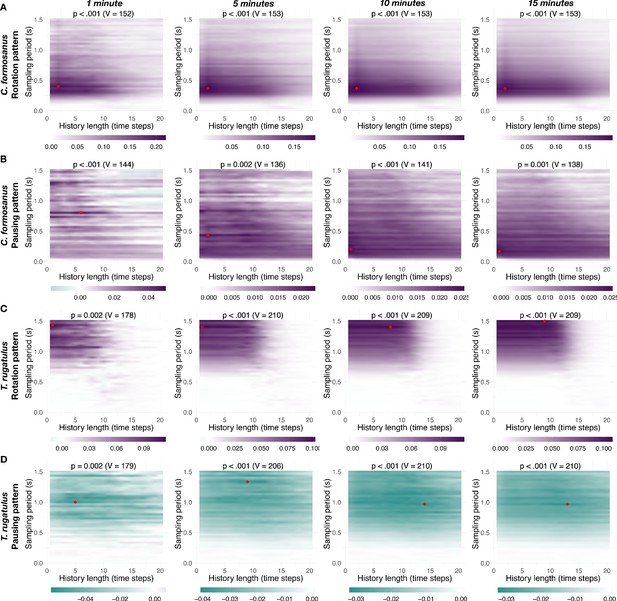
Impact of amount of data on the landscape information transfer.

Net transfer entropy (bits) as a function of the sampling period and of the history length for increasing length of time series (1, 5, 10, and 15 min). (A) and (B) show the results for the rotation pattern and for the pausing pattern for *C. formosanus*. (C) and (D) show the results for the rotation pattern and for the pausing pattern for *T. rugatulus*. Colors indicate the intensity and predominant direction of information transfer (purple, from leader to follower; green, from follower to leader); the red diamond symbol indicates the configuration with maximum absolute magnitude and each panel reports the result of a one-sided paired Wilcoxon signed-rank test with continuity correction ( $p$ -value and $V$ statistic) testing the significance of the observed effect for this parameter configuration.

Figure 7—figure supplement 2

Download asset Open asset

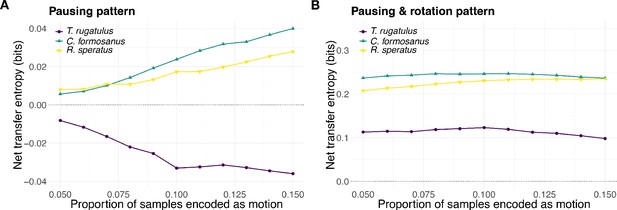
Perturbation analysis of pause-motion threshold.

Net transfer entropy as a function of the probability threshold used to encode step sizes into the motion state and pausing state. (A) Results for the pausing pattern. (B) Results for the compound pausing and rotation pattern. Sampling period and history length correspond to those in Table 1. Positive values represent information transfer from leader to follower, negative values represent information transfer from follower to leader.

Figure 7—figure supplement 3

Download asset Open asset

Predictive information over distance between runners.

Average local transfer entropy as a function of the distance between centroids of runners for the compound pausing and rotation pattern (smoothed conditional means with LOESS smoothing and 0.3 span). (A) *T. rugatulus*, (B) *C. formosanus*, and (C) *R. speratus*.

Tables

Table 1

Selected parameter configurations.

For each species and behavioral pattern, the table provides the number of tandem runs used in the study, the sampling period and corresponding number of time steps in each time series, and the history length.

Species	#Tandem runs	Behavioral pattern	Sampling period (s)	#Time steps	History length $k$
T. rugatulus	20	Rotation	1.5015	599	9
		Pausing	0.9676	930	13
		Pausing and Rotation	1.2346	728	8
C. formosanus	17	Rotation	0.3670	2452	2
		Pausing	0.1668	5395	1
		Pausing and Rotation	0.3670	2452	2
R. speratus	20	Rotation	0.5005	1798	1
		Pausing	0.4671	1926	1
		Pausing and Rotation	0.5005	1798	2

Table 2

Statistics about transfer entropy.

Mean value and standard error of transfer entropy for the experimental ( $T_{X \to Y}$ ) and surrogate ( $T_{X \to Y}^{s}$ ) datasets and of normalized transfer entropy ( ${\tilde{T}}_{X \to Y}$ ) from the predominant source of predictive information to its destination (i.e., always from leader to follower except for the pausing pattern of T. rugatulus when information is transferred from follower to leader).

Species	Behavioral pattern	$T_{L \to F}$	$T_{L \to F}^{s}$	$T_{F \to L}$	$T_{F \to L}^{s}$	${\tilde{T}}_{L \to F} ({\tilde{T}}_{F \to L})$
T. rugatulus	Rotation	$.1488 \pm .0504$	$.0366 \pm .0026$	$.0368 \pm .0125$	$.0371 \pm .0073$	$.1197 \pm .0543$
	Pausing	$.0058 \pm .0033$	$.0047 \pm .0015$	$.0423 \pm .021$	$.0081 \pm .0044$	$.0795 \pm .0401$
	Pausing and Rotation	$.2656 \pm .034$	$.1429 \pm .0092$	$.1836 \pm .0368$	$.1847 \pm .0269$	$.1246 \pm .035$
C. formosanus	Rotation	$.2063 \pm .0731$	$.0009 \pm .0019$	$.0091 \pm .0077$	$.0012 \pm .0017$	$.2884 \pm .0844$
	Pausing	$.0303 \pm .0209$	$.0001 \pm .0003$	$.0064 \pm .0056$	$.0001 \pm .0001$	$.1532 \pm .0865$
	Pausing and Rotation	$.2593 \pm .072$	$.0024 \pm .0028$	$.013 \pm .0135$	$.0026 \pm .0014$	$.2991 \pm .0583$
R. speratus	Rotation	$.1804 \pm .1097$	$.0001 \pm .0003$	$.0027 \pm .0137$	$.0001 \pm .0003$	$.1949 \pm .119$
	Pausing	$.0228 \pm .0149$	$.0001 \pm .0002$	$.0055 \pm .0087$	$.0001 \pm .0001$	$.0698 \pm .0445$
	Pausing and Rotation	$.2385 \pm .1446$	$.0011 \pm .0013$	$.0081 \pm .0121$	$.0011 \pm .0007$	$.2082 \pm .1359$

Table 3

Results of hypothesis testing of information transfer between leaders and followers.

Columns 3 and 4 report the results of one-sided two-sample Wilcoxon rank-sum tests with continuity correction ( $p$ -value and $W$ statistic) testing if the experimental dataset has significantly higher transfer entropy than the surrogate one. Columns 5 and 6 report the results of one-sided paired Wilcoxon signed-rank tests with continuity correction ( $p$ -value and $V$ statistic) testing, respectively, if the leader is significantly more informative than the follower and vice versa. Significant $p$ -values are reported in bold.

Species	Behavioral pattern	$H_{1} : T_{L \to F} > T_{L \to F}^{s}$	$H_{1} : T_{F \to L} > T_{F \to L}^{s}$	$H_{1} : T_{L \to F} > T_{F \to L}$	$H_{1} : T_{F \to L} > T_{L \to F}$
T. rugatulus	Rotation	$p < .001 (W = 380)$	$p = .51 (W = 200)$	$p < .001 (V = 209)$	$p = 1.0 (V = 1)$
	Pausing	$p = .21 (W = 230)$	$p < .001 (W = 388)$	$p = 1.0 (V = 0)$	$p < .001 (V = 210)$
	Pausing and Rotation	$p < .001 (W = 400)$	$p = .7 (W = 181)$	$p < .001 (V = 207)$	$p = 1.0 (V = 3)$
C. formosanus	Rotation	$p < .001 (W = 289)$	$p = .002 (W = 226)$	$p < .001 (V = 153)$	$p = 1.0 (V = 0)$
	Pausing	$p < .001 (W = 272)$	$p < .001 (W = 272)$	$p = .001 (V = 138)$	$p = 1.0 (V = 15)$
	Pausing and Rotation	$p < .001 (W = 289)$	$p = .001 (W = 229)$	$p < .001 (V = 153)$	$p = 1.0 (V = 0)$
R. speratus	Rotation	$p < .001 (W = 380)$	$p = .3 (W = 220)$	$p < .001 (V = 201)$	$p = 1.0 (V = 9)$
	Pausing	$p < .001 (W = 380)$	$p < .001 (W = 340)$	$p < .001 (V = 195)$	$p = 1.0 (V = 15)$
	Pausing and Rotation	$p < .001 (W = 380)$	$p = .018 (W = 278)$	$p < .001 (V = 193)$	$p = 1.0 (V = 17)$