Correction: Systems analysis of the CO2 concentrating mechanism in cyanobacteria

Main text
Article and author information
Metrics

Main text

Mangan NM, Brenner MP. 2014. Systems analysis of the CO₂ concentrating mechanism in cyanobacteria. eLife 3:e02043. doi: 10.7554/eLife.02043

Published 29 April 2014

We would like to issue the following corrections to our research article entitled, ‘Systems analysis of the CO₂ concentrating mechanism in cyanobacteria.’ The code used to generate the plots in the paper, mistakenly used 6.022 × 10²² instead of 6.022 × 10²³ for Avogadro's number. This correction changes the quantitative numbers in the original paper but none of the conclusions are affected. Here are the corrected tables, figures, and text that changed. We thank Avi Flamholz for catching this error.

The article has been corrected accordingly. The original version published on 29 April 2014 is provided as Supplementary file 1 (file held on figshare under doi: 10.6084/m9.figshare.1352030).

Table 2. Enzymatic rates

We recalculated the enzymatic rates for carbonic anhydrase and RuBisCO. The values for V_max and K_1/2 listed in the previous version of Table 2 used the incorrect 6.022 × 10²² value for Avogadro's number. All plots in the original paper were for those values. All new plots are for the values listed in the updated table, using Avogadro's number 6.022 × 10²³. The V_max values are all about an order of magnitude lower than previously calculated.

Enzyme reaction	active sites	k_cat $[\frac{1}{s}]$	V_max in ‘cell’ $[\frac{μ M}{s}]$	V_max in carboxysome $[\frac{μ M}{s}]$	K_1/2 $[μ M]$
carbonic anhydrase hydration	80	8 × 10⁴	8.8 × 10³	1.5 × 10⁷	3.2 × 10³
carbonic anhydrase dehydration	80	4.6 × 10⁴	1.5 × 10⁴	8.8 × 10⁶	9.3 × 10³
RuBisCO carboxylation	2160	26	178	1.8 × 10⁵	270

Figure 2. Phase space for ${HCO}_{3}^{-}$ transport and carboxysome permeability

The main effect of correcting Avagadro's number is that the peak defining optimal carboxysome permeability is lower and broader. The optimal carboxhysome permeability for a target carboxysomal CO₂ concentration is found by looking at the leftmost value on a line of constant concentration on the permeability vs ${HCO}_{3}^{-}$ transport plot. This point represents the carboxysome permeability where the least amount of ${HCO}_{3}^{-}$ transport is needed to achieve a given carboxysomal CO₂ concentration. For 99% carboxylation (cyan curve below) this value is now around k_c = 10⁻³ cm/s (previously k_c = 6 × 10⁻³ cm/s). Carbonic anhydrase also saturates at a lower ${HCO}_{3}^{-}$ transport value.

Figure 2

Download asset Open asset

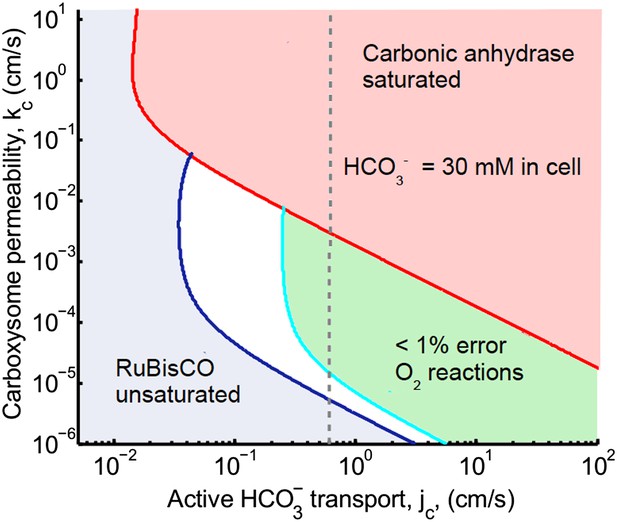
Plotted are the parameter values at which the CO₂ concentration reaches some critical value.

The leftmost line (dark blue) indicates for what values of j_c and k_c the CO₂ concentration in the carboxysome would half-saturate RuBisCO (K_m). The middle line (light blue) indicates the parameter values which would result in a CO₂ concentration where 99% of all RuBisCO reactions are carboxylation reactions and only 1% are oxygenation reactions when O₂ concentration is 260 μM. To the left of the line the error is greater and to the right it is smaller. The right most (red) line indicates the parameter values which result in carbonic anhydrase saturating. Here the rate of conversion from CO₂ to ${HCO}_{3}^{-}$ , α = 0, so there is no CO₂ scavenging or facilitated uptake of CO₂. The dotted line (grey) shows the k_c and j_c values, where the ${HCO}_{3}^{-}$ concentration in the cytosol is 30 mM. The ${HCO}_{3}^{-}$ concentration in the cytosol does not vary appreciably with k_c in this parameter regime, and reaches 30 mM at $j_{c} \approx 0.6 c m / s$ . All other parameters, such as reaction rates are held fixed and the values can be found in the Tables 1 and 2.

Figure 2—figure supplement 1. Effect of decreased diffusion in the carboxysome

With the correction, decreasing the diffusion constant in the carboxysome has much less of an effect on the phase space plot. For optimal or larger carboxysome permeability, there is very little effect (k_c ≥ 10⁻³ cm/s).

Figure 2—figure supplement 1

Download asset Open asset

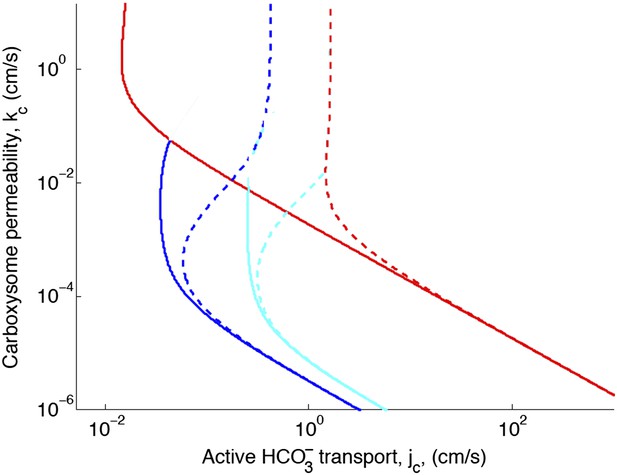
Phase space for ${HCO}_{3}^{-}$ transport and carboxysome permeability for varying diffusion in the carboxysome.

Solid lines show lines of constant CO₂ concentration in the carboxysome for $D_{c} = 1 \times 10^{- 5} \frac{{cm}^{2}}{s}$ , or the diffusion constant of small molecule in water. Dashed lines show the same lines of constant CO₂ concentration, bur for $D_{c} = 1 \times 10^{- 7} \frac{{cm}^{2}}{s}$ , or the diffusion constant of a small molecule in a 60% sucrose solution.

Figure 2—figure supplement 2. Effect of CO₂ scavenging or facilitated uptake

The correction reduces the effect of facilitated uptake. At the optimal permeability it has nearly no effect (difference between dashed and solid lines near k_c = 10⁻³ cm/s).

Figure 2—figure supplement 2

Download asset Open asset

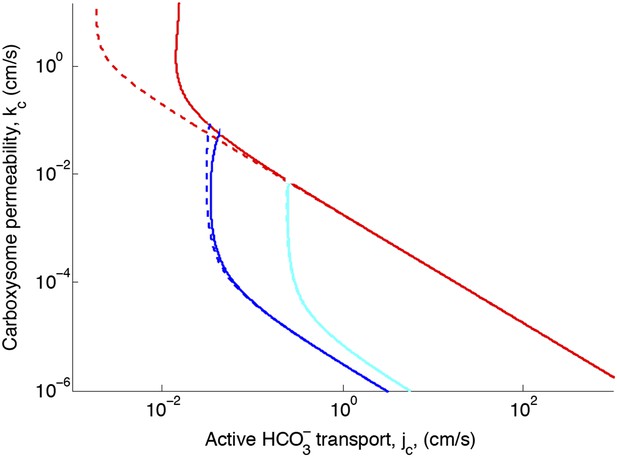
Effect of CO2 scavenging or facilitated uptake on phase space for ${HCO}_{3}^{-}$ transport, j_c, and carboxysome permeability, k_c.

Plotted are the parameter values at which CO₂ concentration reaches some critical value. The leftmost line (dark blue) indicates for what values of j_c and k_c the CO₂ concentration in the carboxysome would saturate RuBisCO. The middle line (light blue) indicates the parameter values which would result in a CO₂ concentration where 99% of all RuBisCO reactions are carboxylation reactions and only 1% are oxygenation reactions when O2 concentration is 260 μM. The rightmost (red) line indicates the parameter values which result in carbonic anhydrase saturating. Here α = 0 cm/s (solid lines) and α = 1 cm/s (dashed line), showing the effect of CO2 scavenging or facilitated uptake on the phase space. All other parameters, such as reaction rates are held fixed and the values can be found Table 1.

Figure 3. CO₂ concentration in the carboxysome as function of ${HCO}_{3}^{-}$ transport: comparison of numeric and analytic solutions

We plot Figure 3 assuming the new optimal carboxysome permeability of k_c = 10⁻³ cm/s (previously k_c = 6 × 10⁻³ cm/s). There is very little change from the previous Figure 3, with the updated carboxysome permeability. Had we plotted at the same k_c = 6 × 10⁻³ cm/s, the CO₂ would have been lower, because the system would not be at the optimal permeability.

Figure 3

Download asset Open asset

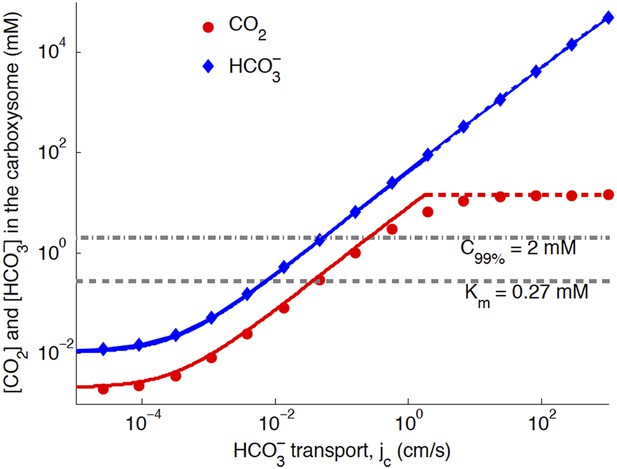
Numerical solution (diamonds and circles) and analytic solutions (carbonic anhydrase unsaturated, solid lines, and saturated, dashed lines) correspond well.

${HCO}_{3}^{-}$ transport is varied, and all other system parameters are held constant. The CO₂ concentration above which RuBisCO is saturated is K_m (grey dashed line). The CO₂ concentration where the oxygen reaction error rate will be 1% is C_99% (grey dash-dotted line). The transition between carbonic anhydrase being unsaturated and saturated happens where the two analytic solutions meet (where the dashed and solid red lines meet). Below a critical value of transport, $j_{c} \approx 10^{- 3} cm / s$ the level of transport is lower than the ${HCO}_{3}^{-}$ leaking through the cell membrane. For these simulations and solutions the carboxysome permeability was k_c = 10⁻³ cm/s (in previous version k_c = 6 × 10⁻³ cm/s was used).

Figure 3—figure supplement 1. No effect of localizing carbonic anhydrase to the shell of the carboxysome

The correction did not at all effect the conclusion that the organization of carbonic anhydrase within the carboxysome has no effect on the resulting concentrations. Consistent with updated Figure 2—figure supplement 1, the effect of decreasing the diffusion in carboxysome is much less prominent than in the original manuscript.

Figure 3—figure supplement 1

Download asset Open asset

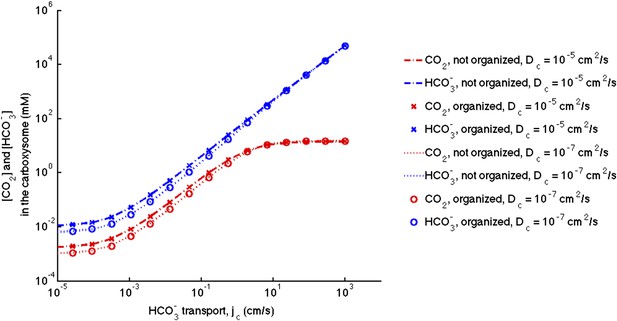
No effect of localizing carbonic anhydrase to the shell of the carboxysome.

We assume the same amount of carbonic anhydrase and RuBisCO activity for each simulation and compare the case with the enzymes evenly distributed throughout the carboxysome to the case where the carbonic anhydrase is localized to the inner carboxysome shell. The (-.-) lines are for no organization and (x) for organized with D_c = 10⁻⁵ cm²/s. The (…) lines are for no organization and (o) are for organized with D_c = 10⁻⁵ cm²/s.

Figure 4. CO₂ concentration in the carboxysome with varying carboxysome permeability

The correction does not effect the conclusion that an optimal carboxysome permeability exists, or what causes it, but it changes its absolute value. The correction has two main effects: (1) it decreases the optimal carboxysome permeability (seen as a shift in the peak) by a factor of 6, and (2) for carboxysome permeabilities higher than optimal (k_c > 0.1 cm/s) the CO₂ concentration is an order of magnitude lower. Therefore the optimal carboxysome permeability peak is at a lower k_c value and more prominent.

Figure 4

Download asset Open asset

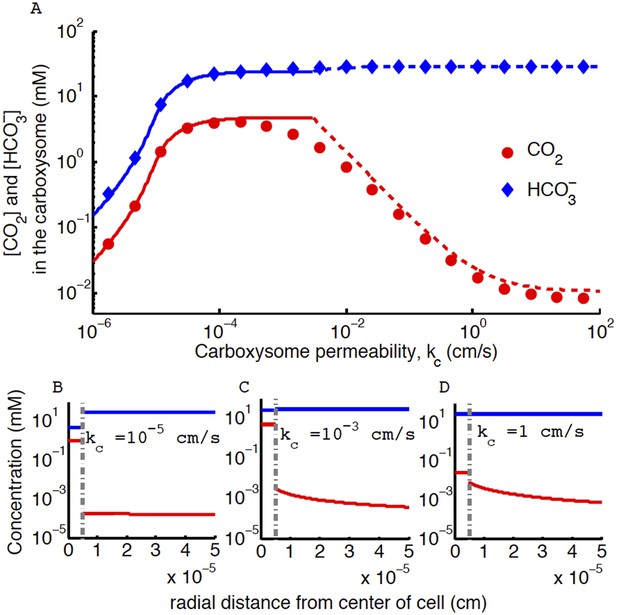
Concentration of CO₂ in the carboxysome with varying carboxysome permeability.

(A) Numerical solution (diamonds and circles) and analytic solutions (carbonic anhydrase unsaturated, solid lines, and saturated, dashed lines) correspond well. On all plots CO₂ (red circle) $< {HCO}_{3}^{-}$ (blue diamond). Concentration in the cell along the radius, r, with carboxysome permeability k_c = 10⁻⁵ cm/s (B), k_c = 10⁻³ cm/s (C), k_c = 1 cm/s (D). Grey dotted lines in (B), (C), (D) indicate location of the carboxysome shell boundary. The transition from low CO₂ at high permeability (D) to maximum CO2 concentration at optimal permeability (C) occurs at $k_{c} = \frac{D}{R_{C}} = 2 c m / s$ . At low carboxysome permeability (B) ${HCO}_{3}^{-}$ diffusion into the carboxysome is slower than consumption. For all subplots the rate of CO₂ to ${HCO}_{3}^{-}$ conversion at the cell membrane, α = 0 *cm/s* and ${HCO}_{3}^{-}$ uptake, j_c = 0.6 *cm/s*. Qualitative results remain the same with varying j_c, increasing α will increase the gradient of CO₂ across the cell as CO₂ is converted to ${HCO}_{3}^{-}$ at the cell membrane.

Table 3. Fate of carbon brought into the cell for j_c = 0.6 cm/s and k_c = 1 × 10⁻³ cm/s

We updated the flux to the value at which cytosolic ${HCO}_{3}^{-}$ is 30 mM and carboxysome permeability is optimal. Previously they were j_c = 0.7 cm/s and k_c = 6 × 10⁻³ cm/s.

Table 3

Fate of carbon brought into the cell for j_c = 0.6 cm/s and k_c = 1 × 10⁻³ cm/s

	Formula	$[\frac{p i c o m o l e s}{(c e l l s)}]$	% Of flux
${HCO}_{3}^{-}$ transport	j_cH_out	3.26 × 10⁻⁴
${HCO}_{3}^{-}$ leakage	$k_{m}^{H} (H_{o u t} - H_{c y t o s o l} (R_{b}))$	3.2 × 10⁻⁴	98.6%
CO₂ leakage	$k_{m}^{C} (C_{o u t} - C_{c y t o s o l} (R_{b}))$	4.5 × 10⁻⁶	1.4%
carboxylation	$\frac{V_{m a x} C}{C + K_{m} (1 + \frac{O}{K_{O}})}$	8.2 × 10⁻⁸	0.03%
oxygenation	$\frac{V_{m a x}^{O} C}{C + K_{m}^{O} (1 + \frac{C}{K_{C}})}$	6.7 × 10⁻¹⁰	2 × 10⁻⁴ %

Table 4. Fate of carbon brought into the cell for j_c = 0.06 cm/s and k_c = 1 × 10⁻³ cm/s

Table 4

Fate of carbon brought into the cell for j_c = 0.06 cm/s and k_c = 1 × 10⁻³ cm/s

	Formula	$[\frac{p i c o m o l e s}{(c e l l s)}]$	% Of flux
${HCO}_{3}^{-}$ transport	j_cH_out	2.8 × 10⁻⁵
${HCO}_{3}^{-}$ leakage	$k_{m}^{H} (H_{o u t} - H_{c y t o s o l} (R_{b}))$	2.7 × 10⁻⁵	96.6%
CO₂ leakage	$k_{m}^{C} (C_{o u t} - C_{c y t o s o l} (R_{b}))$	8.8 × 10⁻⁷	3.2%
carboxylation	$\frac{V_{m a x} C}{C + K_{m} (1 + \frac{O}{K_{O}})}$	5.4 × 10⁻⁸	0.2%
oxygenation	$\frac{V_{m a x}^{O} C}{C + K_{m}^{O} (1 + \frac{C}{K_{C}})}$	2.3 × 10⁻⁹	8 × 10⁻³%

Discussion of fluxes and resulting 2-phosphoglycolate, and 3-phosphoglycerate production

The discussion of the number of transporters needed for this magnitude of ${HCO}_{3}^{-}$ influx increases by an order of magnitude from $10^{3} \frac{transporters}{μ m^{2}}$ to $10^{4} \frac{transporters}{μ m^{2}}$ . While the change in uptake is less than a factor of two different, the conversion from picomoles/s to molecules/s for a cell used the incorrect value of Avogadro's number. So this is now about an order of magnitude larger than the number of ATP synthase complexes on the thylakoid membrane of spinach.

The numbers for net flux of ${HCO}_{3} -$ change slightly. For j_c = 0.6 cm/s the net flux is $6 \times 10^{- 6} \frac{pmol}{cell s}$ compared to the previous value of $10^{- 5} \frac{pmol}{cell s}$ and for j_c = 0.06 cm/s the net flux is $9 \times 10^{- 7} \frac{pmol}{cell s}$ compared with the previous value of $1 \times 10^{- 6} \frac{pmol}{cell s}$ . So the conclusion that measured net fluxes of $10^{- 6} \frac{pmol}{cell s}$ compares best with the lower ${HCO}_{3}^{-}$ flux still holds.

Since Avagadro's number effects the rate of carboxylation and oxygenation, the recalculated values for 2-phosphoglycolate and 3-phosphoglycerate production are lower. This exacerbates the large amount of energy that appears to go into CO₂ concentration compared to the actual fixation. Now around 0.03% of concentrated inorganic carbon is fixed into 3-phosphoglycerate. Over 99.9% is lost to leakage either in the form of CO₂ or ${HCO}_{3}^{-}$ (previously these values were calculated to be 99%). The higher ${HCO}_{3}^{-}$ flux assumption (Table 3) predicts that only 217 2-phosphoglycolate are produced a second, and the lower ${HCO}_{3}^{-}$ flux assumption (Table 4) predicts that 1.4 × 10³ 2-phosphoglycolate are produced a second. So the higher flux allows the production of many fewer 2-phosphoglycolate than previously estimated. Recalculating the time to fix the necessary carbon to replicate a cell results in 7–21 hr for the higher flux rate (Table 3) and 11–35 hr for the lower flux rate (Table 4). Both are consistant with the division times of cyanobacteria, but the higher flux rate is a better match.

In conclusion, the correction further highlights the puzzle of how much energy cyanobacteria must use ${HCO}_{3}^{-}$ to achieve a level of CO₂ which creates adequate fixation rates. Either cyanobacteria use a much larger amount of energy to achieve this internal concentration than to fix each CO₂, the permeability of the cell must be much lower for ${HCO}_{3}^{-}$ than assumed here, or there is another mechanism not covered in this model.

Figure 5. Flux of ${HCO}_{3}^{-}$ from varying sources as the proportion of CO₂ to ${HCO}_{3}^{-}$ outside the cell changes

The correction decreases the effect of CO₂ scavenging at high permeabilities (k_c = 1 cm/s and 10⁻² cm/s). It now appears to be negligible compared to ${HOC}_{3}^{-}$ uptake and CO₂ uptake for all permeabilities. Only when CO₂ concentration is a very small percentage of the external carbon, does the scavenging contribute more than the CO₂ uptake, and both are far less than ${HCO}_{3}^{-}$ uptake.

Figure 5

Download asset Open asset

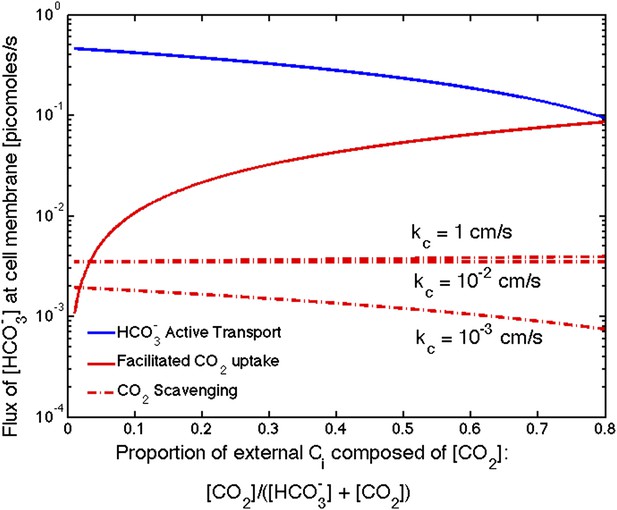
Size of the ${HCO}_{3}^{-}$ flux in one cell from varying sources, as the proportion of CO₂ to ${HCO}_{3}^{-}$ outside the cell changes changes.

We show results for three carboxysome permeabilities, k_c, and only the scavenging is effected. Total external inorganic carbon is 15 μM, j_c = 1 cm/s and $\frac{α}{K_{α}} = 1 cm / s$ . Scavenging is negligibly small for all values of k_c shown. Unless there is very little ${HCO}_{3}^{-}$ in the environment, ${HCO}_{3}^{-}$ transport seems to be more efficient than CO₂ facilitated uptake.

Figure 6. CO₂ concentration with various organizations of enzymes in the cell

With the corrected Avagadro's number the CO₂ concentration is significantly lower in all but the optimal permeability case, making the error rate drastically higher. The effect of optimal permeability on CO₂ concentration is, therefore, much larger.

Figure 6

Download asset Open asset

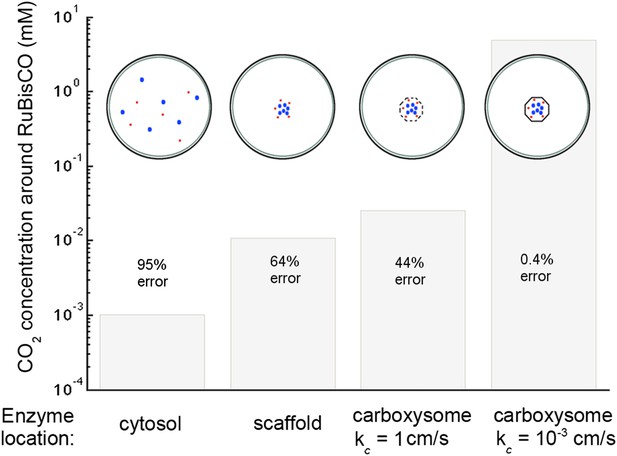
Concentration of CO₂ achieved through various cellular organizations of enzymes, where we have selected the ${HCO}_{3}^{-}$ transport level such that the ${HCO}_{3}^{-}$ concentration in the cytosol is 30 mM.

O2 concentration is 260 μM. The oxygenation error rates, as a percent of total RuBisCO reactions are indicated on the concentration bars. The cellular organizations investigated are RuBisCO and carbonic anhydrase distributed throughout the entire cytosol, co-localizing RuBisCO and carbonic anhydrase on a scaffold at the center of the cell without a carboxysome shell, RuBisCO and carbonic anhydrase encapsulated in a carboxysome with high permeability at the center of the cell, and RuBisCO and carbonic anhydrase encapsulated in a carboxysome with optimal permeability at the center of the cell.

Corrections to text

Throughout the text the default ${HCO}_{3}^{-}$ flux has been changed from $j_{c} = 0.7 \frac{cm}{s}$ to $j_{c} = 0.6 \frac{cm}{s}$ and optimal carboxysome permeability from $k_{c} = 6 \times 10^{- 3} \frac{cm}{s}$ to $k_{c} = 10^{- 3} \frac{cm}{s}$ .

In the discussion of fluxes and concentrations we previously had written:

‘Our simulated cell has a flux of 2 × 10⁷ molecules/s. Assuming the rate of transport per transporter of $10^{3} \frac{molecules}{s}$ and our cell’s surface area this requires about $10^{3} \frac{transporters}{μ m^{2}}$ . This is actually not that far off from the number of ATP synthase complexes on the thylakoid membrane in spinach, $700 \frac{complexes}{μ m^{2}}$ (Miller and Staehelin, 1979), although it is still quite high.’

This is now: ‘Our simulated cell has a flux of 2 × 10⁸molecules/s. Assuming the rate of transport per transporter of $10^{3} \frac{molecules}{s}$ and our cell’s surface area this requires about $10^{4} \frac{transporters}{μ m^{2}}$ . This is about an order of magnitude higher than the number of ATP synthase complexes on the thylakoid membrane in spinach, $700 \frac{complexes}{μ m^{2}}$ (Miller and Staehelin, 1979).’

In the discussion of fluxes and concentrations we previously had written:

‘At the higher flux rate (Table 3) this means that a cell could replicate every 1–2 hr, so faster than cyanobacteria replicate. The lower flux rate (Table 4) would produce fix enough CO₂ for the cell to replicate every 8 to 21 hr, which is similar to the division times of cyanobacteria.’

This is now: ‘At the higher flux rate (Table 3) this means that a cell could replicate every 7–21 hr and the lower flux rate (Table 4) allows replication every 11 to 35 hr. Both are consistent with the division times of cyanobacteria.’

In the caption of Figure 5 we had written:

‘When the carboxysome permeability is larger than optimal, k_c = 1 cm/s, scavenging can contribute more than facilitated uptake at low external CO₂ concentrations. However, when the carboxysome permeability at or below our geometric bound, k_c <= 0.02 cm/s, scavenging is negligibly small.’

This is now: ‘Scavenging is negligibly small for all values of k_c shown.’

In the text of the Benefit of CO₂ to ${HCO}_{3}^{-}$ conversion: facilitated uptake or scavenging of CO₂ we had written: ‘Scavenging only contributes significantly to total incoming ${HCO}_{3}^{-}$ when the carboxysome permeability is higher than optimal, Figure 5, and does not contribute significantly below our calculated upper bound of $k_{c} < = 0.02 c m / s$ . In these ranges for carboxysome permeability, there is very little CO2 leaking out of the carboxysome into the cytosol, so there is very little CO2 to scavenge, Figure 5.’

This is now: ‘Scavenging is negligibly small for all values of k_c shown. There is very little CO₂ in the cytosol, so there is very little CO₂ to scavenge, Figure 5.’

There were also a few typos we have now corrected:
1. In the Reaction diffusion model section we had: ‘RuBisCO also requires ribulose-1,5-bisphosphate, the substrate which CO₂ reacts with to produce 3-phosphoglycolate.’ This has been corrected to ‘… CO₂ reacts with to produce 3-phosphoglycerate.’
2. in Table 1: ‘ $k_{m}^{H}$ permeability of cell membrane to CO₂’ has been corrected to: ‘ $k_{m}^{H}$ permeability of cell membrane to ${HCO}_{3}^{-}$ ’.
3. Table 2 header said k_cut which has been corrected to k_cat.
4. In the Varying ${HCO}_{3}^{-}$ transport saturates enzyme section we had:
  - The chemical equilibrium is $K_{e q} = H / C = (K_{b a} V_{c a}) (K_{c a} V_{b a}) \approx 5$ , for pH around 7 (DeVoe and Kistiakowsky, 1961), so that ${HCO}_{3}^{-}$ > CO₂ in the carboxysome.
  - The equation is now: $K_{e q} = H / C = (K b a V c a) / (K c a V b a) \approx 5$ .
5. In Figure 3—figure supplement 1 the units for D_c on both the plot and caption are cm/s, they should be cm²/s.

Article and author information

Author details

Niall M Mangan

Competing interests
The authors declare that no competing interests exist.
Michael P Brenner

Competing interests
The authors declare that no competing interests exist.

Version history

Version of Record published: March 26, 2015

Copyright

This article is distributed under the terms of the Creative Commons Attribution License, which permits unrestricted use and redistribution provided that the original author and source are credited.

Metrics

607

views
1

downloads
1

citations

Views, downloads and citations are aggregated across all versions of this paper published by eLife.

Download links

A two-part list of links to download the article, or parts of the article, in various formats.

Open citations (links to open the citations from this article in various online reference manager services)

Mendeley

Cite this article (links to download the citations from this article in formats compatible with various reference manager tools)

Niall M Mangan
Michael P Brenner

(2015)

Correction: Systems analysis of the CO₂ concentrating mechanism in cyanobacteria

eLife 4:e06400.

https://doi.org/10.7554/eLife.06400

Main text

Table 2. Enzymatic rates

Figure 2. Phase space for ${HCO}_{3}^{-}$ transport and carboxysome permeability

Plotted are the parameter values at which the CO₂ concentration reaches some critical value.

Figure 2—figure supplement 1. Effect of decreased diffusion in the carboxysome

Phase space for ${HCO}_{3}^{-}$ transport and carboxysome permeability for varying diffusion in the carboxysome.

Figure 2—figure supplement 2. Effect of CO₂ scavenging or facilitated uptake

Effect of CO2 scavenging or facilitated uptake on phase space for ${HCO}_{3}^{-}$ transport, j_c, and carboxysome permeability, k_c.

Figure 3. CO₂ concentration in the carboxysome as function of ${HCO}_{3}^{-}$ transport: comparison of numeric and analytic solutions

Numerical solution (diamonds and circles) and analytic solutions (carbonic anhydrase unsaturated, solid lines, and saturated, dashed lines) correspond well.

Figure 3—figure supplement 1. No effect of localizing carbonic anhydrase to the shell of the carboxysome

No effect of localizing carbonic anhydrase to the shell of the carboxysome.

Figure 4. CO₂ concentration in the carboxysome with varying carboxysome permeability

Concentration of CO₂ in the carboxysome with varying carboxysome permeability.

Table 3. Fate of carbon brought into the cell for j_c = 0.6 cm/s and k_c = 1 × 10⁻³ cm/s

Table 4. Fate of carbon brought into the cell for j_c = 0.06 cm/s and k_c = 1 × 10⁻³ cm/s

Discussion of fluxes and resulting 2-phosphoglycolate, and 3-phosphoglycerate production

Figure 5. Flux of ${HCO}_{3}^{-}$ from varying sources as the proportion of CO₂ to ${HCO}_{3}^{-}$ outside the cell changes

Size of the ${HCO}_{3}^{-}$ flux in one cell from varying sources, as the proportion of CO₂ to ${HCO}_{3}^{-}$ outside the cell changes changes.

Figure 6. CO₂ concentration with various organizations of enzymes in the cell

Concentration of CO₂ achieved through various cellular organizations of enzymes, where we have selected the ${HCO}_{3}^{-}$ transport level such that the ${HCO}_{3}^{-}$ concentration in the cytosol is 30 mM.

Corrections to text

Article and author information

Author details

Niall M Mangan

Competing interests

Michael P Brenner

Competing interests

Version history

Copyright

Metrics

Download links

Open citations (links to open the citations from this article in various online reference manager services)

Cite this article (links to download the citations from this article in formats compatible with various reference manager tools)

Categories and tags

Be the first to read new articles from eLife

Share this article

Cite this article

Plotted are the parameter values at which the CO2 concentration reaches some critical value.

Phase space for HCO3− transport and carboxysome permeability for varying diffusion in the carboxysome.

Effect of CO2 scavenging or facilitated uptake on phase space for HCO3− transport, jc, and carboxysome permeability, kc.

Numerical solution (diamonds and circles) and analytic solutions (carbonic anhydrase unsaturated, solid lines, and saturated, dashed lines) correspond well.

No effect of localizing carbonic anhydrase to the shell of the carboxysome.

Concentration of CO2 in the carboxysome with varying carboxysome permeability.

Size of the HCO3− flux in one cell from varying sources, as the proportion of CO2 to HCO3− outside the cell changes changes.

Concentration of CO2 achieved through various cellular organizations of enzymes, where we have selected the HCO3− transport level such that the HCO3− concentration in the cytosol is 30 mM.

Author details

Niall M Mangan

Competing interests

Michael P Brenner

Competing interests

Open citations (links to open the citations from this article in various online reference manager services)

Cite this article (links to download the citations from this article in formats compatible with various reference manager tools)

Categories and tags

Plotted are the parameter values at which the CO₂ concentration reaches some critical value.

Phase space for ${HCO}_{3}^{-}$ transport and carboxysome permeability for varying diffusion in the carboxysome.

Effect of CO2 scavenging or facilitated uptake on phase space for ${HCO}_{3}^{-}$ transport, j_c, and carboxysome permeability, k_c.

Concentration of CO₂ in the carboxysome with varying carboxysome permeability.

Size of the ${HCO}_{3}^{-}$ flux in one cell from varying sources, as the proportion of CO₂ to ${HCO}_{3}^{-}$ outside the cell changes changes.

Concentration of CO₂ achieved through various cellular organizations of enzymes, where we have selected the ${HCO}_{3}^{-}$ transport level such that the ${HCO}_{3}^{-}$ concentration in the cytosol is 30 mM.