Figures and data in Spatial self-organization favors heterotypic cooperation over cheating | eLife

Figures
Additional files

9 figures and 1 additional file

Figures

Figure 1 with 2 supplements

Download asset Open asset

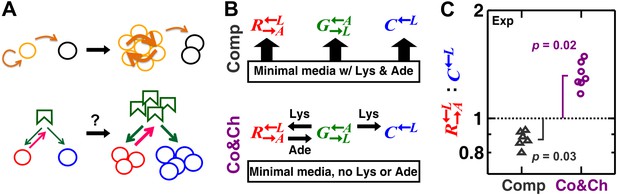
A spatial environment favors heterotypic cooperation over cheating.

(A) Top: clustering with self-type can favor homotypic cooperators (yellow) over cheaters (black). Bottom: clustering with self-type should not favor heterotypic cooperation since cooperator clusters (red) and competitively superior cheater clusters (blue) should have equivalent access to the heterotypic cooperative partner (green). (B) We engineered three yeast strains: a red-fluorescent $R_{\to A}^{\leftarrow L}$ strain requiring lysine and releasing adenine; a green-fluorescent $G_{\to L}^{\leftarrow A}$ strain requiring adenine and releasing lysine; and a cyan-fluorescent $C^{\leftarrow L}$ strain requiring lysine and not releasing adenine. The three strains purely competed (‘Comp’) or additionally cooperated and cheated (‘Co&Ch’), depending on whether the medium contained or lacked adenine (‘Ade’) and lysine (‘Lys’), respectively. (C) In competitive communities, $R_{\to A}^{\leftarrow L}$ : $C^{\leftarrow L}$ dropped below the initial value of 1 (dotted line) during community growth due to the fitness advantage of $C^{\leftarrow L}$ over $R_{\to A}^{\leftarrow L}$ . In contrast, $R_{\to A}^{\leftarrow L}$ : $C^{\leftarrow L}$ rose above 1 when the strains engaged in cooperation and cheating. Population ratios in experiments were measured using flow cytometry. All communities started from an initial density of 3000 total cells/mm² with the three strains at a 1:1:1 ratio on top of an agarose column (Figure 1—figure supplement 1A) and were analyzed after approximately six to eight generations (Figure 1—figure supplement 2). p Values are from the Wilcoxon signed rank test, comparing the median with 1.

https://doi.org/10.7554/eLife.00960.003

Figure 1—figure supplement 1

Download asset Open asset

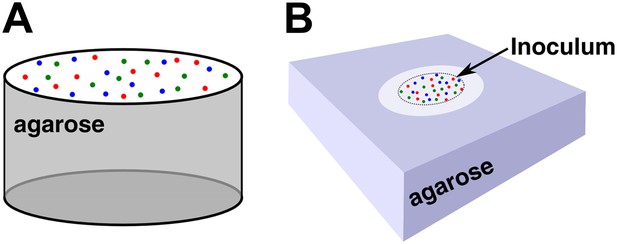
Community setup.

(A) Unless otherwise stated (see B), experiments and simulations were initiated by randomly distributing the three cell populations on top of a filter placed above an agarose column of equal size. The agarose column was 6 mm in diameter and 11 mm in height. (B) Alternatively, a 2 mm-diameter spot of cell mixture was deposited at the center of a membrane filter on top of an agarose pad (24 mm × 24 mm × 4 mm). In this configuration, the initial inoculum spot had ∼8 × 10⁴ total cells/mm². Unless otherwise stated, all communities were initiated from equal proportions of $R_{\to A}^{\leftarrow L}$ , $G_{\to L}^{\leftarrow A}$ , and $C^{\leftarrow L}$ populations.

https://doi.org/10.7554/eLife.00960.004

Figure 1—figure supplement 2

Download asset Open asset

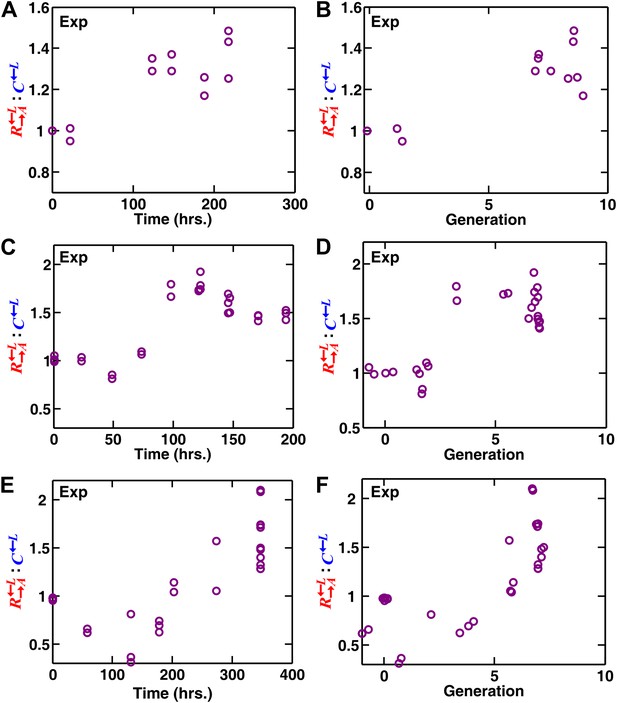
In experimental spatial communities, cooperators $R_{\to A}^{\leftarrow L}$ are favored over cheaters $C^{\leftarrow L}$ .

(A–D) In the communities of heterotypic cooperation and cheating (Figure 1—figure supplement 1A), the $R_{\to A}^{\leftarrow L}$ : $C^{\leftarrow L}$ ratio increased over time before leveling off. The initial surface cell density was 3000 cells/mm² in (A and B) and 10⁴ cells/mm² in (C and D). The ratios did not change considerably in late stages of community growth (approximately six to eight generations in B and D) and were therefore used for reporting results (e.g., in Figure 1C). Since sampling was destructive, all data points were from independent communities grown to various times and generations. The consistency in the data trend highlights small variations among independent spatial communities. The leveling off of the $R_{\to A}^{\leftarrow L}$ : $C^{\leftarrow L}$ ratio in later generations likely reflects the fact that by that time, not much open space is available for self-organization (Figure 7). As a reference, 10⁴/mm² is two generations away from confluence. (E and F) Unlike in (A–D) where the growth area was limited to the original inoculation domain (Figure 1—figure supplement 1A), the $R_{\to A}^{\leftarrow L}$ : $C^{\leftarrow L}$ ratio continued to increase during later generations when the growth medium extended beyond the original inoculation domain (Figure 1—figure supplement 1B). The initial decrease in $R_{\to A}^{\leftarrow L}$ : $C^{\leftarrow L}$ suggests that $C^{\leftarrow L}$ was more starvation-tolerant and therefore fitter than $R_{\to A}^{\leftarrow L}$ in this lysine-limited environment before sufficient cell growth and spatial organization occurred. Exp: experiment.

https://doi.org/10.7554/eLife.00960.005

Figure 2 with 2 supplements

Download asset Open asset

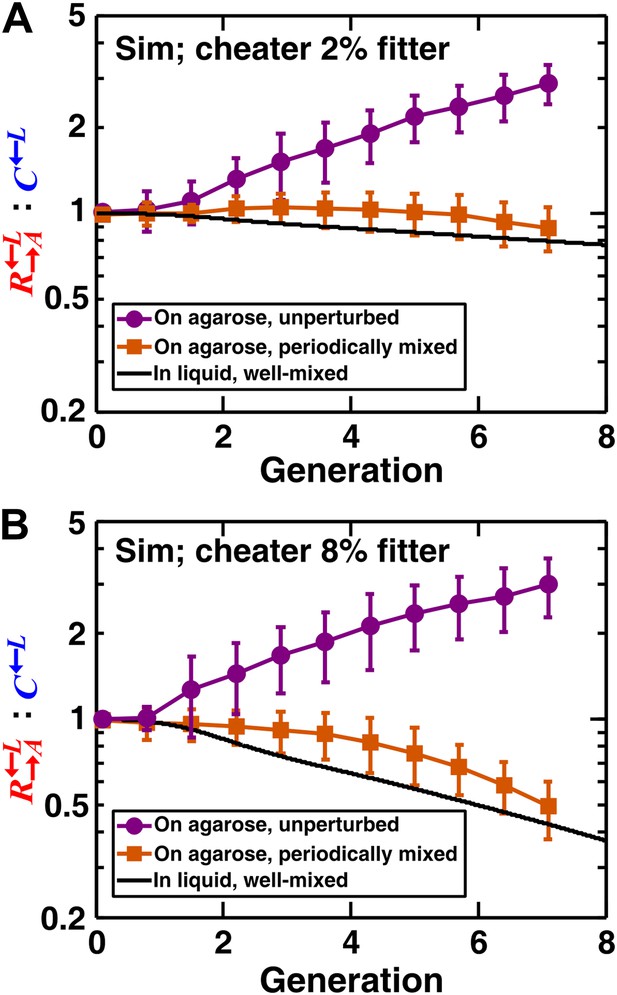
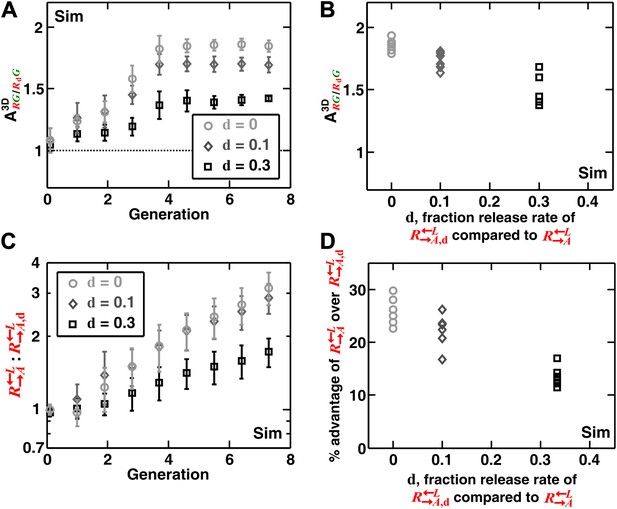
In simulated communities, a spatial environment is required to promote heterotypic cooperation.

The $R_{\to A}^{\leftarrow L}$ : $C^{\leftarrow L}$ ratios of simulated (‘Sim’) spatial cooperating and cheating communities were grown either unperturbed (purple circles) or periodically mixed (orange squares). To simulate periodic mixing, the arrangement of cells was completely randomized every 12 hr. In each mixing event, the concentration of adenine and lysine throughout the community was assigned to be the average value over the entire community. Error bars show the standard deviation of ratios in six independent communities. The solid black line shows the ratio in a simulated well-mixed liquid coculture using the same parameters as simulated communities on agarose (Figure 2—source data 1). The fitness advantage of cheater over cooperator was either 2% (top panel) or 8% (bottom panel).

https://doi.org/10.7554/eLife.00960.006

Figure 2—source data 1 Parameter values used in the diffusion model simulations.: https://doi.org/10.7554/eLife.00960.007
Download elife-00960-fig2-data1-v1.xls

Figure 2—figure supplement 1

Download asset Open asset

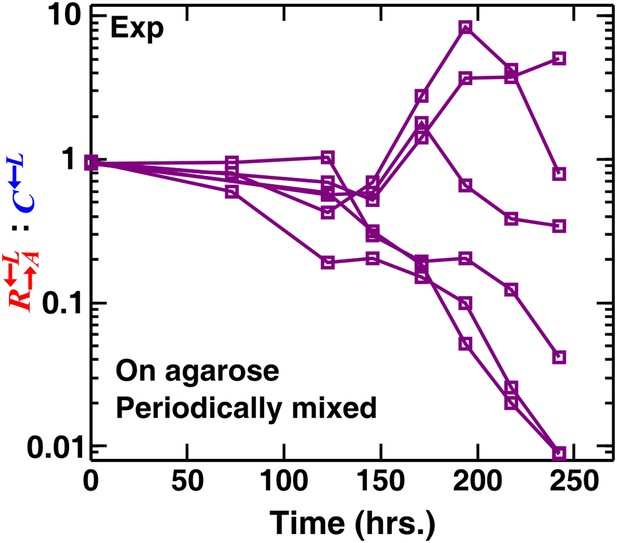
Stochastic cheater outcomes in periodically mixed communities of ancestral cooperators, cheaters, and partners.

The $R_{\to A}^{\leftarrow L}$ : $C^{\leftarrow L}$ ratios diverged in six replicate experimental communities periodically mixed during growth. Cell migration during mixing allowed the mutant type most adapted to the low-lysine environment to sweep through the entire community. The most adaptive mutation could occur in either $R_{\to A}^{\leftarrow L}$ or $C^{\leftarrow L}$ , which led to stochastic outcomes similar to those observed in well-mixed liquid cocultures (Waite and Shou, 2012). We started these communities from a spot at a density of ∼8 × 10⁴ total cells/mm² (Figure 1—figure supplement 1B) and physically mixed them daily (approximately every two generations) using a glass rod. The cells attached to the glass rod (estimated to be ∼10% of the community population) were suspended in water and analyzed using flow cytometry. Exp: experiment.

https://doi.org/10.7554/eLife.00960.008

Figure 2—figure supplement 2

Download asset Open asset

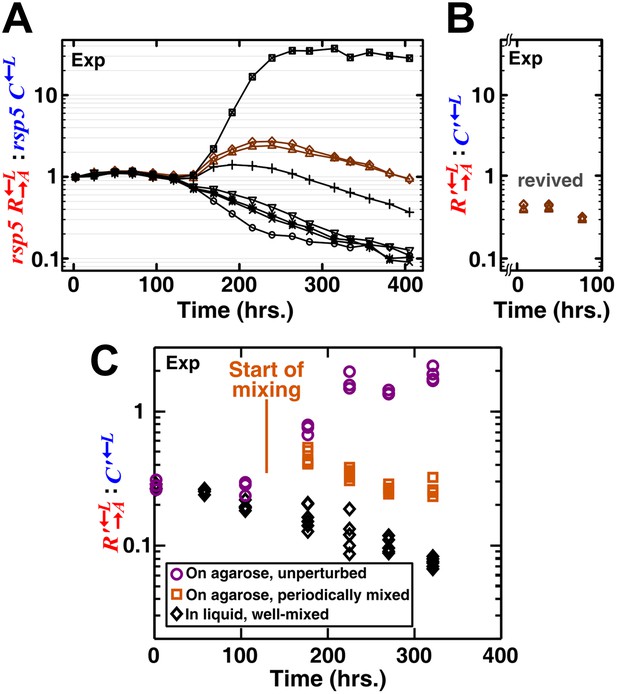
A spatial environment is required to favor heterotypic cooperation over cheating.

(A) To preadapt cooperators and cheaters in the lysine-limited coculture environment so that no new mutations of large fitness benefits could quickly arise, we started eight well-mixed replicates (marked by different symbols) consisting of rsp5 $R_{\to A}^{\leftarrow L}$ , rsp5 $C^{\leftarrow L}$ , and the ancestral $G_{\to L}^{\leftarrow A}$ (‘Materials and methods’). The rsp5 mutation was previously found to be highly adaptive for the lysine-requiring cells in a lysine-limited environment (Waite and Shou, 2012). The initial stochastic phase was indicative of additional rounds of adaptive races (Waite and Shou, 2012) between rsp5 $R_{\to A}^{\leftarrow L}$ and rsp5 $C^{\leftarrow L}$ . After 250 hr, the $R_{\to A}^{\leftarrow L}$ : $C^{\leftarrow L}$ ratios showed steady trends, suggesting no additional rapid adaptive races. Two of these cultures (brown) that had $R_{\to A}^{\leftarrow L}$ : $C^{\leftarrow L}$ ratios close to 1:1 were revived from frozen stocks. (B) In the two revived cocultures where the evolved populations were denoted with a “ ʹ ”, ${R^{'}}_{\to A}^{\leftarrow L}$ : ${C^{'}}^{\leftarrow L}$ continued to decline steadily. The broken axis indicates the period of time elapsed during which a small revived inoculum (30 μl frozen stock into 200 μl minimal medium and then expanded to 2 ml minimal medium) grew to detectable densities. (C) When preadapted communities were grown unperturbed on agarose pads, cooperators were favored as communities grew (purple circles). In contrast, cheaters were favored when the spatial aspect of the environment was either disrupted (daily mixing after day 5, orange squares) or absent (well-mixed liquid cocultures, black diamonds). We started all spatial communities at ∼8 × 10⁴ total cells/mm² in a ∼2 mm-diameter spot (Figure 1—figure supplement 1B) and liquid communities at 5 × 10⁵ total cells/ml in 3 ml SD. Exp: experiment.

https://doi.org/10.7554/eLife.00960.009

Figure 3 with 2 supplements

Download asset Open asset

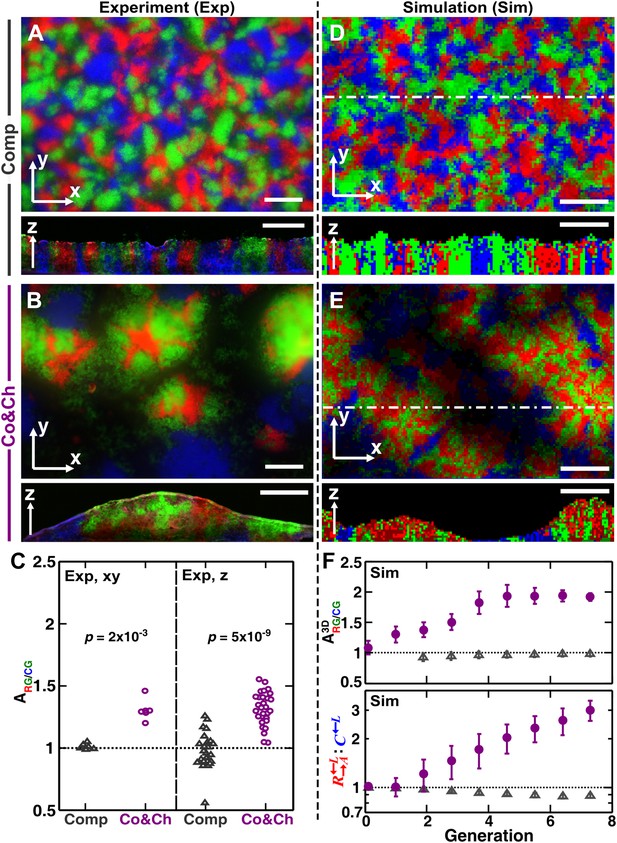
Growing cells self-organize to exclude cheaters from heterotypic cooperators.

(A and B) Experimentally, as the initially randomly distributed cells grew, different patterns emerged depending on whether the medium contained or lacked adenine and lysine supplements and consequently whether the dominant cell-cell interaction was respectively competition (‘Comp’) or cooperation and cheating (‘Co&Ch’). Top-views: ‘xy’; vertical sections: ‘z’. (C) Compared to $C^{\leftarrow L}$ , $R_{\to A}^{\leftarrow L}$ had a higher level of association with $G_{\to L}^{\leftarrow A}$ during cooperation and cheating ( $A_{R G / C G}$ > 1) but not during competition. In (C), the communities were analyzed after approximately six to eight generations. (D, E and F) We observed similar results in the simulated communities. In simulated top-views, higher color intensity indicates a greater number of cells of the corresponding fluorescent color stacked at that position. In simulated vertical cross-sections, low and high color intensity represent dead and live cells, respectively. Scale bar: 100 μm. In C and F, grey: competition; magenta: cooperation and cheating. p Values are from the Mann–Whitney U-test. All communities started from an initial density of 3000 total cells/mm² (Figure 1—figure supplement 1A).

https://doi.org/10.7554/eLife.00960.010

Figure 3—figure supplement 1

Download asset Open asset

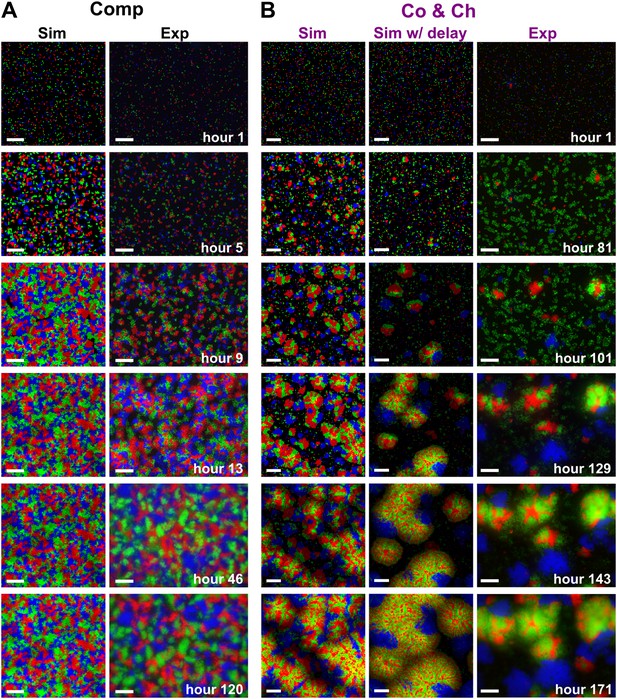
Cooperation-cheating and pure competition led to distinct community patterns in top-views.

(A) On media supplemented with adenine and lysine, $R_{\to A}^{\leftarrow L}$ , $G_{\to L}^{\leftarrow A}$ , and $C^{\leftarrow L}$ competed for shared resources. Individual cells initially grew into microcolonies (hour 5) and expanded until they reached other microcolonies (hours 9–13). Subsequent growth was mainly in the vertical direction due to physical constraints (hour 46), and top-view patterns remained static (hours 46–120). (B) On media not supplemented with adenine or lysine, $R_{\to A}^{\leftarrow L}$ and $G_{\to L}^{\leftarrow A}$ were the heterotypic cooperative pair and $C^{\leftarrow L}$ was the cheater. After small microcolonies formed (hour 81), the positive feedback between $R_{\to A}^{\leftarrow L}$ and $G_{\to L}^{\leftarrow A}$ led to the formation of pods consisting of mixed heterotypic cooperators (red and green) surrounded by isolated patches of cheaters (blue) (hours 101–171). All parameters in simulations are similar to those in Figure 3. ‘Sim w/delay’ incorporated a 60 hr delay in the death of $G_{\to L}^{\leftarrow A}$ and in the consequent release of lysine according to experimental observations (Momeni et al., 2013). This simulation generated results qualitatively similar to simulations without incorporating the delay (‘Sim’). For generality, we opted to use simulations without such a delay throughout the paper. Scale bar: 100 μm. Co&Ch: cooperating and cheating; Comp: competing; Exp: experiment; Sim: simulation.

https://doi.org/10.7554/eLife.00960.011

Figure 3—figure supplement 2

Download asset Open asset

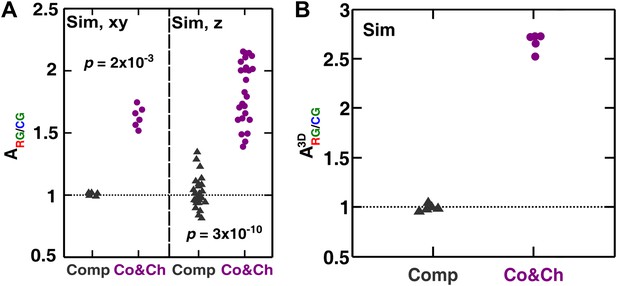
The partner association indexes in simulated communities showed more association between $R_{\to A}^{\leftarrow L}$ and $G_{\to L}^{\leftarrow A}$ than between $C^{\leftarrow L}$ and $G_{\to L}^{\leftarrow A}$ during cooperation and cheating but not during competition.

(A) Quantifying the partner association index in top-views (‘xy’) and vertical cross-sections (‘z’) of simulated communities shows trends similar to the experimental results in Figure 3C. (B) When quantified using a three-dimensional neighborhood, the partner association indexes showed similar results as in (A). The partner association indexes are less variable when averaged across each three-dimensional community using a three-dimensional neighborhood instead of across each two-dimensional slice of a community using a two-dimensional neighborhood (compare B with A). Co&Ch: cooperating and cheating; Comp: competing; Sim: simulation.

https://doi.org/10.7554/eLife.00960.012

Figure 4 with 3 supplements

Download asset Open asset

Self-organization leads the most giving cooperator to associate most with the heterotypic cooperative partner, allowing discrimination of cooperators of varying quality.

In diffusion model simulations, $R_{\to A, d}^{\leftarrow L}$ produced adenine at a rate d-fold (0 ≤ d < 1) of the release rate of $R_{\to A}^{\leftarrow L}$ . $R_{\to A, d}^{\leftarrow L}$ had a 5% fitness advantage over $R_{\to A}^{\leftarrow L}$ at all lysine concentrations. $R_{\to A, d}^{\leftarrow L}$ that released less (smaller values of d) were isolated more in spatial patterns (A, steady-state values summarized in B) and disfavored more as the community grew (C and D). In (D), the fitness advantage of $R_{\to A}^{\leftarrow L}$ over $R_{\to A, d}^{\leftarrow L}$ was calculated from the rate of changes in the ratio $R_{\to A}^{\leftarrow L}$ : $R_{\to A, d}^{\leftarrow L}$ between generations 2 and 6 in (C). In (B) and (D), data from six replicates were plotted. The communities were initiated at 3000 total cells/mm². Sim: simulation.

https://doi.org/10.7554/eLife.00960.013

Figure 4—figure supplement 1

Download asset Open asset

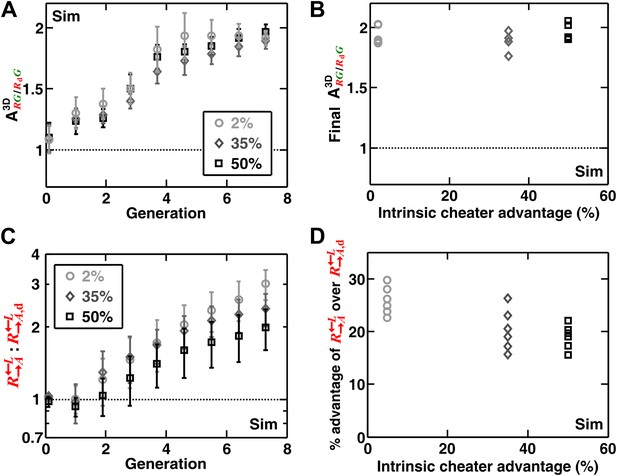
Spatial self-organization allowed $R_{\to A}^{\leftarrow L}$ to increase in frequency even when $R_{\to A, d = 0}^{\leftarrow L}$ was much fitter.

In diffusion model simulations, the percent growth rate advantage of $R_{\to A, d}^{\leftarrow L}$ over $R_{\to A}^{\leftarrow L}$ , marked in the insets, was assumed to be fixed at all concentrations of lysine. Here, d = 0. The partner association index ( $A_{R G / R_{d} G}^{3 D}$ ) did not show strong dependence on the intrinsic advantage of $R_{\to A, d}^{\leftarrow L}$ over $R_{\to A}^{\leftarrow L}$ (A and B). The overall advantage of $R_{\to A}^{\leftarrow L}$ over $R_{\to A, d}^{\leftarrow L}$ in the self-organized communities was reduced when the intrinsic growth rate advantage of $R_{\to A, d}^{\leftarrow L}$ over $R_{\to A}^{\leftarrow L}$ increased (C). However, $R_{\to A, d}^{\leftarrow L}$ were still disfavored compared to $R_{\to A}^{\leftarrow L}$ , and a larger intrinsic advantage of $R_{\to A, d}^{\leftarrow L}$ over $R_{\to A}^{\leftarrow L}$ did not translate proportionally into better overall survival of $R_{\to A, d}^{\leftarrow L}$ in the community (D). The partner association indexes in (B) corresponded to communities after seven generations of growth. The $R_{\to A}^{\leftarrow L}$ advantage over $R_{\to A, d}^{\leftarrow L}$ in (D) was calculated from changes in the ratio $R_{\to A}^{\leftarrow L}$ : $R_{\to A, d}^{\leftarrow L}$ between generations 2 and 6 in (C). The communities were initiated at 3000 total cells/mm². Sim: simulation.

https://doi.org/10.7554/eLife.00960.014

Figure 4—figure supplement 2

Download asset Open asset

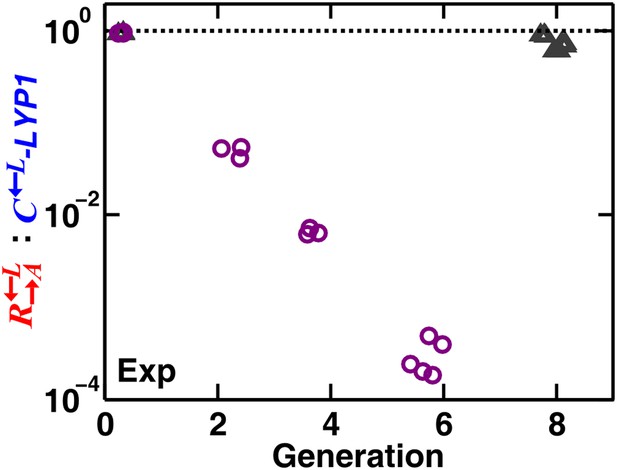
Cheaters with a much higher affinity for cooperative benefits than cooperators can destroy heterotypic cooperation even in a spatial environment.

The evolved cheater $C^{\leftarrow L}$ –LYP1 (with a mutation in LYP1 (see CT10 in Table 1 of Waite and Shou., 2012) is much more efficient in taking up lysine at low lysine concentrations than the ancestral cooperator $R_{\to A}^{\leftarrow L}$ (Waite and Shou, 2012). Thus, the evolved cheater consumed lysine and grew while keeping the lysine concentration at very low levels where the ancestral $R_{\to A}^{\leftarrow L}$ could hardly grow. In a spatial environment with no supplements, starting from equal proportions of $R_{\to A}^{\leftarrow L}$ : $G_{\to L}^{\leftarrow A}$ : $C^{\leftarrow L}$ –LYP1, cheaters were favored (purple circles). When supplied with abundant adenine and lysine (gray triangles), the high affinity of $C^{\leftarrow L}$ –LYP1 for low concentrations of lysine was no longer advantageous; the $R_{\to A}^{\leftarrow L}$ : $C^{\leftarrow L}$ –LYP1 ratio slowly changed in favor of cheaters in this case, similar to the ‘Comp’ case in Figure 1C. Exp: experiment.

https://doi.org/10.7554/eLife.00960.015

Figure 4—figure supplement 3

Download asset Open asset

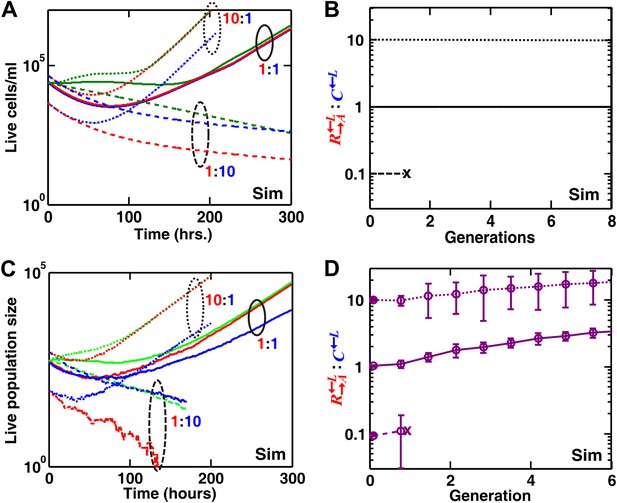
In obligatory byproduct mutualism (benefit production incurs no cost and non-producers have no fitness advantage over producers), high levels of non-producers can still destroy byproduct mutualism in a spatial environment.

In simulations, we examined the growth of communities of $R_{\to A}^{\leftarrow L}$ , $G_{\to L}^{\leftarrow A}$ , and $C^{\leftarrow L}$ at different initial ratios in well-mixed (A and B) and spatial (C and D) environments lacking supplements. The initial population size of $G_{\to L}^{\leftarrow A}$ was always one third of the initial total population size, which was kept constant. $R_{\to A}^{\leftarrow L}$ : $C^{\leftarrow L}$ varied among 1:10, 1:1, and 10:1 (dashed, solid, and dotted lines, respectively). Even though non-producer $C^{\leftarrow L}$ was assumed to have no fitness advantage over producer $R_{\to A}^{\leftarrow L}$ , high levels of non-producers still destroyed mutualism ( $R_{\to A}^{\leftarrow L}$ : $C^{\leftarrow L}$ = 1:10) in liquid (B) and spatial (D) environments (cross indicates the extinction of the community). Error bars in (D) are standard deviations calculated using six independent replicates. Note that non-producers are disfavored in spatial, but not well-mixed, environment. Sim: simulation.

https://doi.org/10.7554/eLife.00960.016

Figure 5

Download asset Open asset

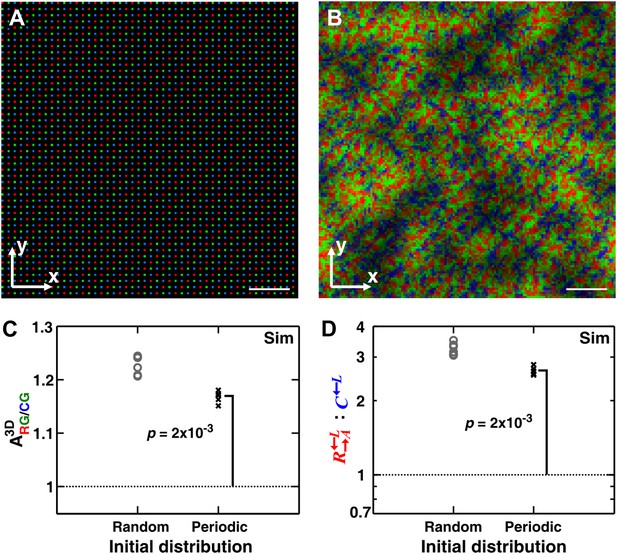
Heterogeneity in the initial spatial distribution of cells facilitates but is not required for cheater isolation.

Starting from a symmetric and periodic distribution in which all cooperators $R_{\to A}^{\leftarrow L}$ and cheaters $C^{\leftarrow L}$ had an equal access to the partner $G_{\to L}^{\leftarrow A}$ (A), heterotypic cooperators self-organized (B and C) and were favored (D). (B–D) corresponded to generation 6. Break of symmetry from the initial symmetric spatial distribution can be due to stochastic effects such as differences in the initial amounts of metabolites cells possessed, death of cells, or the random direction of cell division. Compared to a random initial distribution, communities with a periodic initial distribution showed smaller mean $A_{R G / C G}$ ; nonetheless, $A_{R G / C G}$ significantly exceeded 1 (Wilcoxon signed rank test). In these simulations, the growth rate advantage of $C^{\leftarrow L}$ over $R_{\to A}^{\leftarrow L}$ was assumed to be 10% at all concentrations of lysine. The communities were initiated at 4400 total cells/mm². Scale bar: 100 μm. In simulated top-views, higher color intensity indicates a greater number of cells of the corresponding color stacked at that position. Sim: simulation.

https://doi.org/10.7554/eLife.00960.017

Figure 6 with 1 supplement

Download asset Open asset

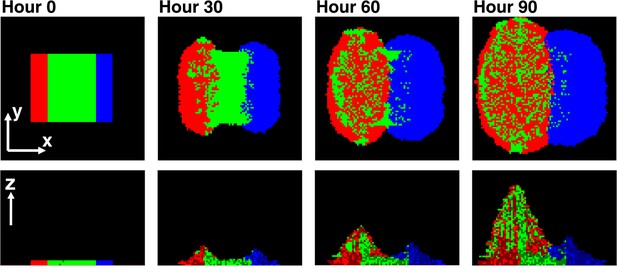
Asymmetric fitness effects of cooperators and cheaters on partners drive self-organization.

Time progression of self-organization in a simulated community as observed in top-views (top) and vertical cross-sections (bottom). Heterotypic cooperative partners (green) supply large benefits to both cooperators (red) and cheaters (blue). Since the benefit is spatially localized, only cooperators and cheaters that are close to partners will grow. Given that cells dividing toward partners will on average have more access to benefits than those dividing away from partners, both cooperators and cheaters pile over partners. Cooperators reciprocate by supplying a large, but different, localized benefit to the partner, while cheaters do not. Thus, partners grow and pile over cooperators but not cheaters. Consequently, further growth of cooperators is facilitated, while cheaters become isolated and disfavored. In this simulation, cheaters have an 8% fitness advantage over cooperators. To mimic the top-view from microscopy, the top-view in this simulation represents the top-most layer of cells instead of the total intensity integrated over z at each pixel.

https://doi.org/10.7554/eLife.00960.018

Figure 6—figure supplement 1

Download asset Open asset

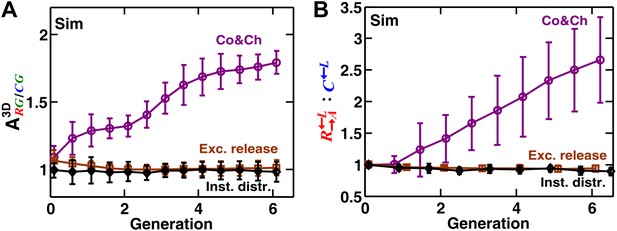
Localization of cooperative benefits is required for self-organization.

The partner association indexes and the $R_{\to A}^{\leftarrow L}$ : $C^{\leftarrow L}$ ratios of simulated cooperating and cheating spatial communities were compared at different levels of localization of benefits. ‘Co&Ch’ communities had parameters similar to those in Figure 3—figure supplement 2B (purple circles) and therefore had normal levels of spatial localization of benefits. In ‘Exc. release’ communities (brown squares), cooperators and partners released excessive amounts of adenine and lysine, respectively (200-fold higher compared to the original communities). Since the neighboring cells could not consume the released metabolites fast enough, the benefits no longer remained localized to the vicinity of the releasing cell. In ‘Inst. distr.’ cases (black diamonds), the diffusion coefficient in the community was assumed to be very large, such that any released metabolite was instantly distributed among all cells. When benefits were delocalized either because of excessive metabolite release or rapid distribution of metabolite throughout the community, self-organization was diminished (A) and cooperators were disfavored compared to cheaters (B). In all cases, cheaters had an 8% intrinsic fitness advantage over cooperators, and communities were not disturbed (i.e., cells were not repositioned). Error bars show the standard deviation of ratios in six independent communities. Sim: simulation.

https://doi.org/10.7554/eLife.00960.019

Figure 7 with 1 supplement

Download asset Open asset

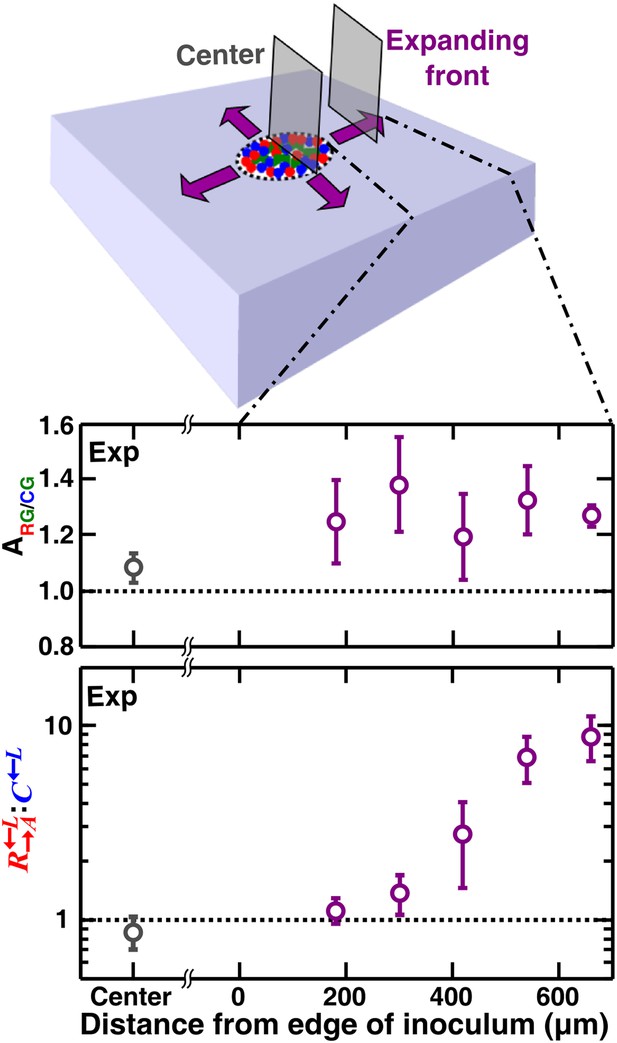
Cell growth into open space is required for self-organization and cheater isolation.

In an unperturbed spatial environment, a community starting from a high-density (∼10⁵ total cells/mm²) confluent inoculum expanded to new territories (purple). Compared to ‘Center’, self-organization was significantly more in ‘Expanding front’. Consequently, the initially 1:1 $R_{\to A}^{\leftarrow L}$ : $C^{\leftarrow L}$ ratio changed in favor of cooperators as the community expanded outward but not in ‘Center’. Sections were collected from three independent communities. Using the Mann–Whitney U-test, the association indexes of the ‘Center’ and of the ‘Expanding front’ were significantly different (p = 4 × 10⁻⁴). Exp: experiment.

https://doi.org/10.7554/eLife.00960.020

Figure 7—figure supplement 1

Download asset Open asset

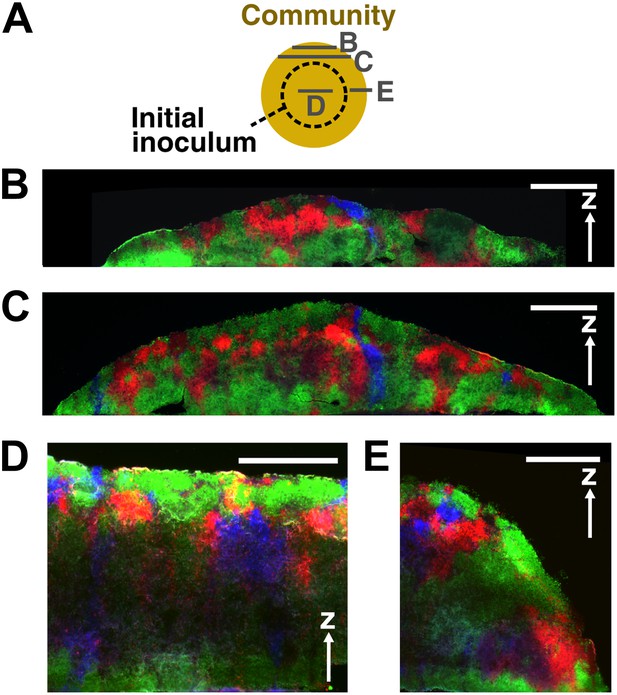
Vertical cross-sections of communities started from a high-density inoculum.

A schematic diagram (A) shows positions (gray solid lines) of cross-sections (B–E) taken from a community initiated from a high-density inoculum (dashed circle). Vertical cross-sections in (B) and (C) were 100 μm and 180 μm from the edge of the community, respectively. (D) and (E) show respectively the center portion and the expanding front portion of a vertical cross-section through the initial inoculum region. Cheater patches became smaller or disappeared as the community front expanded out. Scale bar: 200 μm.

https://doi.org/10.7554/eLife.00960.021

Author response image 1

Download asset Open asset

Adapted from Figures 1 and 6 in (Shou et al. 2007): When partner $G_{\to L}^{\leftarrow A}$ was paired with the cooperator $R_{\to A}^{\leftarrow L}$ , the coculture grew from low to high densities. No persistent growth was observed when partner $G_{\to L}^{\leftarrow A}$ was paired with the cheater $C^{\leftarrow L}$ .

Author response image 2

Download asset Open asset

Well-mixed evolved cocultures of heterotypic cooperators and cheaters showed a steady change in the $R_{\to A}^{\leftarrow L}$ : $C^{\leftarrow L}$ ratio. (A) To pre-adapt cooperators and cheaters in the lysine-limited coculture environment so that no new mutations of large fitness benefits could quickly arise, we started eight well-mixed replicates (marked by different symbols) consisting of rsp5 $R_{\to A}^{\leftarrow L}$ , rsp5 $C^{\leftarrow L}$ , and the ancestral $G_{\to L}^{\leftarrow A}$ (Materials and methods). The rsp5 mutation was previously found to be highly adaptive for the lysine-requiring cells in a lysine-limited environment (Waite & Shou 2012). The initial stochastic phase at 150 hours is indicative of additional rounds of adaptive races²¹ between rsp5 $R_{\to A}^{\leftarrow L}$ and rsp5 $C^{\leftarrow L}$ . After 250 hours, the $R_{\to A}^{\leftarrow L}$ : $C^{\leftarrow L}$ ratios showed steady trends, suggesting no additional rapid adaptive races. Two of these cultures (purple) were revived from frozen stocks. (B) In the two revived cocultures where the evolved populations were denoted with a “ ʹ ”, ${R^{'}}_{\to A}^{\leftarrow L}$ : ${C^{'}}^{\leftarrow L}$ continued to decline steadily before being used for experiments in Figure 2–figure supplement 2. Broken axis indicates the period of time elapsed during which a small revived inoculum (30 μl into 200 μl minimal medium and then expanded to 2 ml minimal medium) grew to detectable densities.

Additional files

Source code 1 A MATLAB code for simulating the progression of patterns in a community of $R_{\to A}^{\leftarrow L}$ , $G_{\to L}^{\leftarrow A}$ , and $C^{\leftarrow L}$ . The code is based on the diffusion model (‘Materials and methods’) and includes initially supplied glucose in the medium as a shared resource consumed by all populations (parameters in Figure 2—source data 1).: https://doi.org/10.7554/eLife.00960.022
Download elife-00960-code1-v1.m

Download links

A two-part list of links to download the article, or parts of the article, in various formats.

Downloads (link to download the article as PDF)

Open citations (links to open the citations from this article in various online reference manager services)

Mendeley

Cite this article (links to download the citations from this article in formats compatible with various reference manager tools)

Babak Momeni
Adam James Waite
Wenying Shou

(2013)

Spatial self-organization favors heterotypic cooperation over cheating

eLife 2:e00960.

https://doi.org/10.7554/eLife.00960

Sign up for email alerts

Privacy notice