Breaking down hierarchies of decision-making in primates
- CBC, DTIC, Universitat Pompeu Fabra, Spain
- Universitat Pompeu Fabra, Spain
Figures
Figure 1
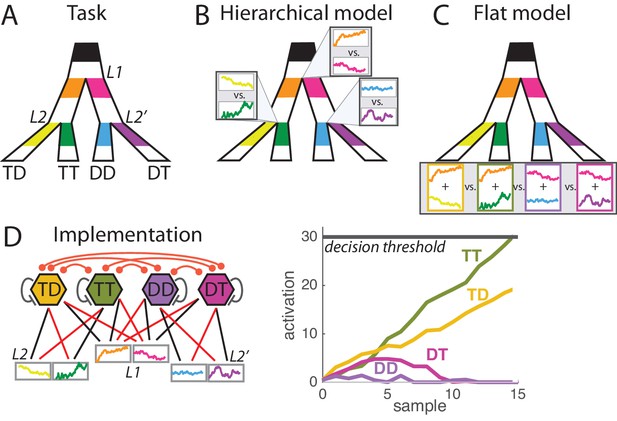
Hierarchical vs. flat models of perceptual decision during a hierarchically-structured visual task.
(A) Structure of the task. At each trial monkeys must detect the correct option out of four possible responses based on the visual information provided at the primary branching point L1 and at two secondary branching points L2 and L2’. Visual information consists of segments of flickering luminosity at the start of each branch (color segments in our depiction; visual samples changing every 50 ms for a total period of 1000 ms). Animals must make a saccade towards the final point that passes through branches of maximal luminosity; that is, must decide based on information scattered across the visual field. Each of the four responses is categorized as TT, TD, DT or DD depending on whether it corresponds to a target T (correct branch) or distractor D (incorrect branch) at first and second branching points. Detailed description of the task can be found in [Lorteije et al., 2015]. (B) Hierarchical decision model of perception. In the hierarchical model, parallel decision processes run at each branching point (L1, L2 and L2’) and are integrated into a motor response at a later stage. It can be implemented as a race model composed of three races, each with two possible sub-choices. (C) Flat decision model of perception. In the flat model, the decision space is composed of the four possible final responses, so for each response the animal must sum the information provided at the corresponding primary and secondary branches (here depicted by the sum of the two luminosity signals). (D) Implementation of the flat decision model. (Left panel) Four units coding for the four possible responses integrate information from both L1 and L2 branches leading to that response, as represented by the pattern of connections from sensory units (coding for instantaneous sensory value). Connectivity include self-excitation as well as homogeneous cross-inhibition between all units. Black and red arrows indicate respectively excitatory and inhibitory connections. (Right panel) Simulation for one trial, depicting the activity of each unit across time during perceptual integration. Activity is bounded to positive values (rectification). When activity of one unit reaches the decision threshold, the related response is selected.
Figure 2 with 2 supplements
Behavioral properties of the flat race model reproduce original monkey data.
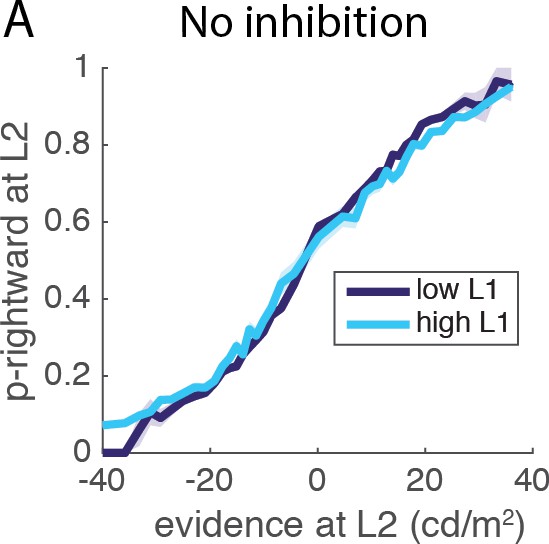
The figures presented here correspond to a parameter set adjusted to reproduce reaction times (see Introduction). (A) Response type for the flat model. We simulated a simple race model with four possible options that compete for selection, based on a standard implementation of race models [Drugowitsch et al., 2014]. The histogram shows the distribution of each of the four response types (TT, TD, DT, DD) for each level of trial difficulty from simulations of the race implementation of the flat model. (B) Influence of luminance fluctuations on the L1 decisions. Weights for each of the target (orange) and distractor (blue) samples across a trial for L1 decisions, as measured with logistic regression. The model reproduces the primacy effect observed in monkey behavioural data, whereby earlier samples have larger influence on L1 choices than later samples. Shades indicating 95% confidence intervals (barely visible) are nearly collapsed to the main line. Weights have been normalized. (C) Influence of luminance fluctuations on the L2 decisions. Legend same as B. (D) Psychometric curve. The curve represents the probability that the right segment was selected at the first (L1, red curve) and second (L2, light blue curve) level depending on the strength of the evidence in favor or right vs. left path at the corresponding branching point. Steeper curve indicates better performance at the primary than secondary branches. (E) Influence of L1 difficulty onto L2 performance. Psychometric curve for L2 decision was computed separately for easy and difficult L1 trials. Unlike the flat model implemented in the original study, we find no difference in L2 performance (permutation test; p>0.4), in accordance with observed animal behavior. The null effect emerges because the minimum accumulation time before reaching a decision impedes early response even in the presence of strong evidence in L1 (see Supp. Material). When inhibition was removed, a significance interaction was recovered (Figure 2—figure supplement 1A). (F) Mean reaction times. Reaction times for the 3 types of difficulty level (E: easy. I: intermediate, D:difficult) reproduce those reported in the companion paper by Zylberberg and colleagues (Zylberberg et al., 2017). (G) Influence of L2 and L2’ difficulty onto L1 choice. Psychometric curve is plotted separately for trials where decision is easier at the left than right secondary branch (green curve), and where decision is easier at right than left secondary branch (red curve). Inset represents the distribution of difference in evidence between the two branches. The effect emerges because strong evidence at one secondary branch will bias the race towards selecting the corresponding final option, thus appearing as a bias in L1 decision towards selecting the primary branch leading to this secondary branch. Information provided at secondary branches biases choice at L1 towards selecting the branch that leads to easier secondary branch, as observed in animal behavior. Lower panel represents the difference between the two psychometric curves. (Right Panel): Influence of difficulty of L2 on the L1 decision across time, as estimated from the logistic regression. Grey shades indicate 95% confidence intervals. L1 choice is affected by difference in perceptual difficulty between L2 and L2’ branches in early visual samples.
Figure 2—figure supplement 1
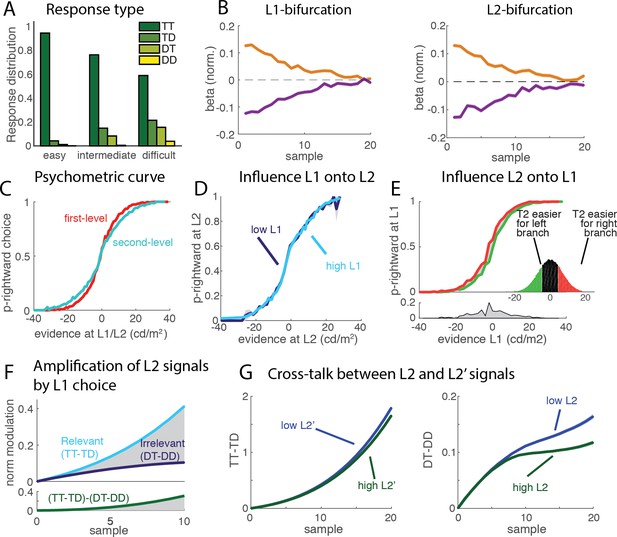
Reciprocal influence of L1 and L2 evidence and decisions.
(A) Influence of L1 difficulty on L2 performance for model without inhibition. The analysis of interaction between L1 difficulty and L2 performance was repeated in a control network without inhibition (). Threshold is set to six to yield similar level of performance and primacy effect; all other parameters remain unchanged. When removing inhibition performance for difficult L1 trials was degraded comparer to easy L1 trials (permutation test, p<10−4), exactly as observed in the original simulations of Lorteije and colleagues.
Figure 2—figure supplement 2
Behavioral and neural effects for a parameter set yielding longer reaction times than in monkey data.
In this original simulation set, commented in Zylberberg et al., decision threshold is large and there is no minimal viewing time before reaching a decision (see Methods). All qualitative behavioural (panels A-F) as well as neural (panels G-H) effects are qualitatively similar to those reported in main text (Figures 2–3) and in agreement with experimental findings of [Lorteije et al., 2015].
Figure 3
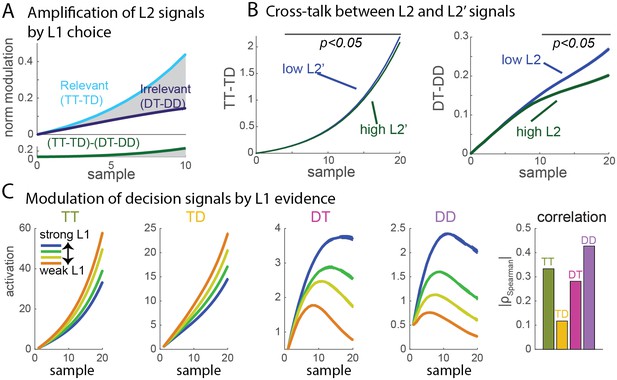
Signal properties of the flat race model reproduce original monkey neural data.
(A) Amplification of L2 signals by L1 choice. Decision signals for L2 (resp. L2’), depicted here in cyan (resp. dark blue) curves, correspond to the difference between the activation of the units coding for the target and distractor in that branch, i.e. TT-TD (resp. DT-DD). Lower panel represents the difference between the two selection signals, i.e. (TT-TD)-(DT-DD). While the two decision signals first increase in parallel, the selection signal rapidly turns much larger for the correct than for the incorrect branches, due to the inhibition between activation of options across different branches. Dynamics closely resembles that observed in neural multi-unit activity in V4. S.e.m. are too small to be visualized. (B) Cross-talk between L2 and L2’ selection signals. Selection signal for L2 was reduced when the first visual samples in L2’ provide clear information in favour of one option (left panel, blue curve for lowerL2' evidence, green curve for higher L2' evidence). The effect readily emerges in the flat model because of inhibition between options across the different branches. The converse effect of L2 difficulty impacting L2’ selection signal was also observed (right panel), and both effects reproduce observation from monkey visual cortex. S.e.m. are too small to be visualized. The horizontal bar indicates samples with significant activity difference (t-test, p<0.05). (C) Selection signals integrate L1 level of evidence. Unit activity is modulated by evidence at both L1 and L2 levels. Mean activity in each four units (classified as TT, TD, DT, DD) for 4 quartiles of strength of L1 evidence. TT and TD units show positive modulations with L1 evidence, while DT and DD units display negative modulations, consistent with the fact that larger L1 evidence biases competition towards the two options in the correct L1 branch. Right panel displays absolute value of Spearman’s rho correlation between strength of evidence and mean activity for all four branches.
Tables
Table 1
Each of reported behavioral and neural effects and the associated features from the flat decision model required to display such effects.
| Effect | Required model features |
|---|---|
| Independence of L2 from L1 difficulty | Signal-independent noise, low dependence of RTs on L1 difficulty |
| Bias of L1 choice by L2 difficulty | none |
| Amplification of L2 signals by L1 choice | Activation rectification |
| Cross-talk between L2 and L2' signals | Activation rectification |
Download links
A two-part list of links to download the article, or parts of the article, in various formats.
Downloads (link to download the article as PDF)
Open citations (links to open the citations from this article in various online reference manager services)
Cite this article (links to download the citations from this article in formats compatible with various reference manager tools)
Breaking down hierarchies of decision-making in primates
eLife 6:e16650.
https://doi.org/10.7554/eLife.16650
