Figures and data in Inferring genetic interactions from comparative fitness data

Figures
Tables
Additional files

10 figures, 3 tables and 1 additional file

Figures

Figure 1

Download asset Open asset

All $24$ rank orders of the biallelic two-locus system, where the $16$ colored rank orders imply epistasis.

Red (top row) indicates positive epistasis and blue (middle row) negative epistasis.

https://doi.org/10.7554/eLife.28629.002

Figure 2

Download asset Open asset

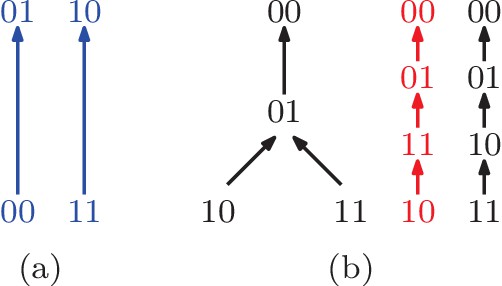
(a) A partial fitness order of genotypes.

The rank orders that extend this partial order are orders (9 , 10 , 12 , 13 , 14), and (16) in Figure 1. All of them imply negative epistasis ( $ε_{2} < 0$ ). (b) A partial order of genotypes with all its total extensions shown on the right. The first extension shown in red implies positive epistasis ( $ε_{2} > 0$ ), while the second one in black does not.

https://doi.org/10.7554/eLife.28629.003

Figure 3

Download asset Open asset

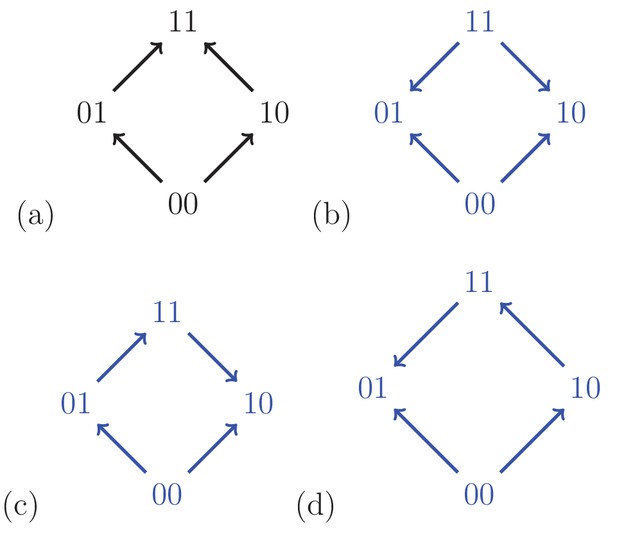
For a biallelic two-locus system where the genotype $00$ has the lowest fitness, there are four fitness graphs.

The graph (a) is compatible with additive fitness, whereas the remaining graphs imply negative epistasis.

https://doi.org/10.7554/eLife.28629.004

Figure 4

Download asset Open asset

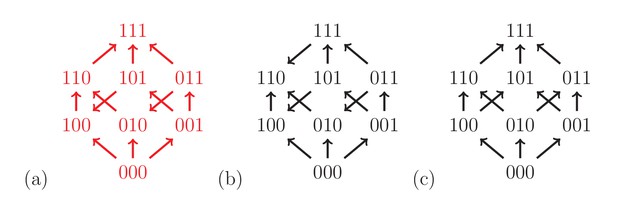
The fitness graph (a) implies three way epistasis, the graph (b) implies epistasis, but not higher order epistasis, and (c) does not imply epistasis, since all arrows point up.
https://doi.org/10.7554/eLife.28629.005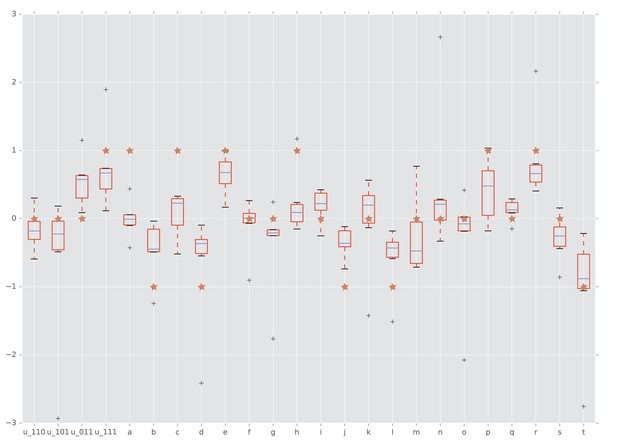

Figure 5

Download asset Open asset

Interactions detected from fitness summary statistics and from rank orders.

The horizontal axis is labeled by the four interaction coordinates $u_{110}$ , $\dots$ , $u_{111}$ and twenty circuits $a$ , $\dots$ , $t$ . The boxplots show the distributions of the various interactions induced by the empirical fitness distribution. The red star indicates whether the interaction has been detected by our rank order method. Specifically, a star with vertical coordinate $- 1$ , 0, and one means negative, no, and positive interaction, respectively.

https://doi.org/10.7554/eLife.28629.006

Figure 6

Download asset Open asset

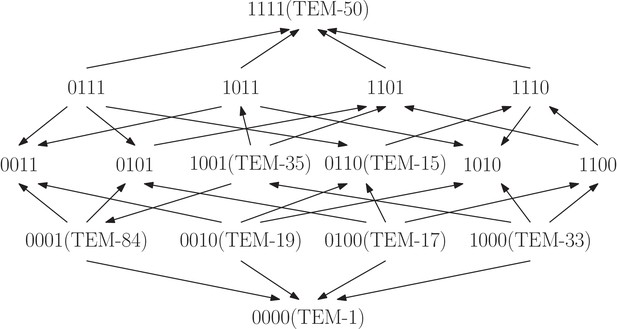
The TEM-family of $β$ -lactamase contributes to antibiotic resistance problems in hospitals.

The fitness graph shows a four-locus system consisting of the wild type, TEM-1, the quadruple mutant, TEM-50, and all intermediate mutants, including six clinically found mutants in the TEM family. The mutation M69L corresponds to $1000$ , E104K to $0100$ , G238S to $0010$ , and N276D to $0001$ . Growth rates were measured for the 16 genotypes under exposure to the antibiotic FEP Cefepime, and the fitness graph was determined accordingly (Mira et al., 2015). The graph reveals higher order epistasis.

https://doi.org/10.7554/eLife.28629.007

Figure 7

Download asset Open asset

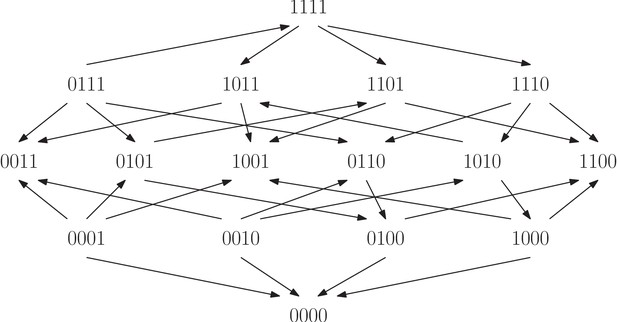
The fitness graph shows a four-locus system for the filamentous fungus *Aspergillus Niger*.

The system consists of all combinations of the four individually deleteriouis mutations *fwnA1*, *leuA1*, *oliC2* and *crnB12*. The landscape has in total four peaks, labeled $0000, 1100, 0011$ and $1001$ .

https://doi.org/10.7554/eLife.28629.008

Figure 8

Download asset Open asset

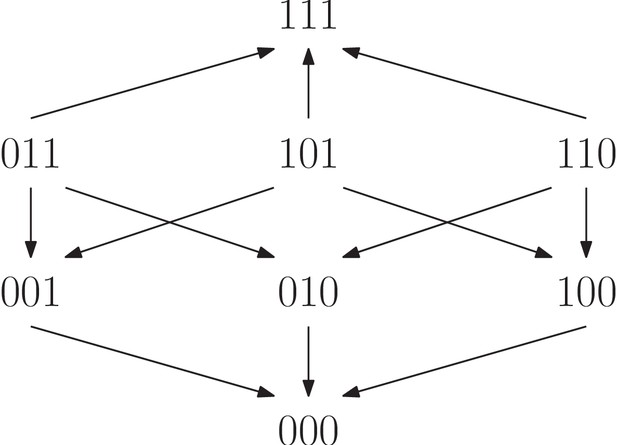
The fitness graph is compatible with the two rank orders (5) and (6).
https://doi.org/10.7554/eLife.28629.009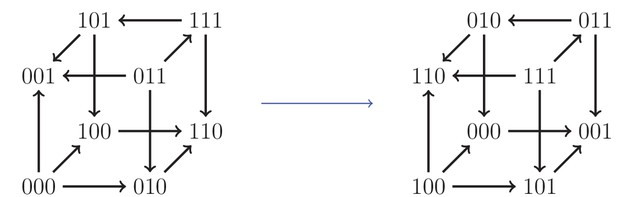

Figure 9

Download asset Open asset

An example of an isomorphism.

Here, the allele labels ‘0’ and ‘1’ in the first locus have been interchanged, as well as the second and third loci.

https://doi.org/10.7554/eLife.28629.010

Figure 10

Download asset Open asset

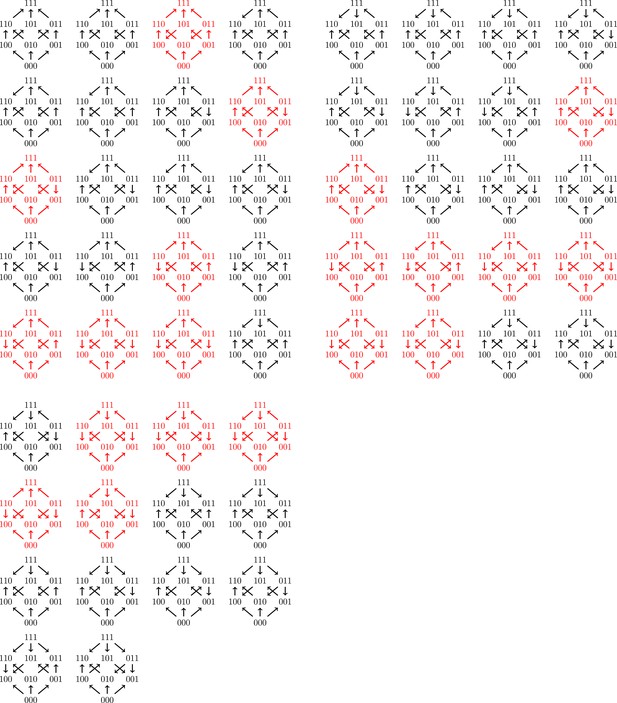
All 54 fitness graph types.

Those depicted in red imply three-way epistasis.

https://doi.org/10.7554/eLife.28629.012

Tables

Table 1

Numbers and fractions of rank orders that imply $n$ -way epistasis.

https://doi.org/10.7554/eLife.28629.011

Loci	Rank orders	Imply epistasis	Fraction
2	24	16	$2 / 3$
3	40,320	16,128	$2 / 5$
4	20,922,789,890,000	4,649,508,864,000	$2 / 9$

Table 2

Comparison of the rank order method with t-test.

The first column lists the four interaction coordinates and twenty circuits. The second column shows p-values returned by Student’s t-test based on fitness measurements. The third column shows which interactions are significant based on 0.03 threshold and their signs. For comparison, the last column displays the results obtained from rank order methods.

https://doi.org/10.7554/eLife.28629.013

Interaction	p-value	Result	From rank order
$u_{011}$	1.13e-31	$+$	$0$
$u_{101}$	2.67e-12	$-$	$0$
$u_{110}$	1.20e-24	$-$	$0$
$u_{111}$	1.50e-29	$+$	$+$
$a$	7.10e-16	$-$	$+$
$b$	5.23e-32	$-$	$-$
$c$	7.62e-04	$+$	$+$
$d$	8.36e-68	$-$	$-$
$e$	1.39e-38	$+$	$+$
$f$	2.59e-01	$0$	$0$
$g$	3.10e-59	$-$	$0$
$h$	2.22e-02	$-$	$+$
$i$	7.97e-05	$+$	$0$
$j$	2.20e-32	$-$	$-$
$k$	1.96e-05	$+$	$0$
$l$	7.50e-51	$-$	$-$
$m$	4.88e-07	$-$	$0$
$n$	9.87e-37	$+$	$0$
$o$	8.83e-03	$+$	$0$
$p$	7.18e-19	$+$	$+$
$q$	1.94e-01	$0$	$0$
$r$	5.02e-50	$+$	$+$
$s$	7.10e-27	$-$	$0$
$t$	8.49e-61	$-$	$-$

Table 3

Data from (Franke et al., 2011) on a 5-locus system determined by the mutations fwnA1, argH12, pyrA5, leuA1, and pheA1.

We consider 5-way epistasis for the system. The first column lists the ranking of the genotypes, where ”?” means missing measurement. The eighth column indicates whether the genotype is odd or even. The ninth and tenth columns show the cumulative number of $o$ ’s and $e$ ’s, respectively. The last column indicates whether the number of $o$ ’s exceeds the number of $e$ ’s ( $-$ ) or vice versa ( $+$ ). We see that if genotype $11101$ has higher fitness than genotype $10011$ , genotypes $11000, 10010$ are ranked arbitrarily, the missing genotype $10111$ has rank $1 - 15$ , and $11010$ rank $20 - 32$ , then the last column would change to all +’s, so the rank order would imply $u_{11111} > 0$ .

https://doi.org/10.7554/eLife.28629.014

Rank	fwn	arg	pyr	leu	phe	#mutations	$o$ / $e$	# $o$ cumul.	# $e$ cumul.	neg. vs pos.
1	0	0	0	0	0	0	$e$	0	1	+
2	1	0	0	0	1	2	$e$	0	2	+
3	0	1	0	1	1	3	$o$	1	2	+
4	0	1	0	0	1	2	$e$	1	3	+
5	1	1	0	1	1	4	$e$	1	4	+
6 (or 7)	1	1	0	0	0	2	$e$	1	5	+
7 (or 6)	1	0	0	1	0	2	$e$	1	6	+
8	0	0	0	0	1	1	$o$	2	6	+
9	1	1	1	0	0	3	$o$	3	6	+
10	0	1	0	0	0	1	$o$	4	6	+
11	0	1	1	0	1	3	$o$	5	6	+
12	1	0	1	0	0	2	$e$	5	7	+
13	0	0	0	1	0	1	$o$	6	7	+
14	1	0	0	0	0	1	$o$	7	7	+
15	1	1	0	0	1	3	$o$	8	7	$-$
16	0	1	1	0	0	2	$e$	8	8	+
17	0	0	1	1	1	3	$o$	9	8	$-$
18 (or 19)	1	1	1	0	1	4	$e$	9	9	$+$
19 (or 18)	1	0	0	1	1	3	$o$	10	9	$-$
20	0	0	0	1	1	2	$e$	10	10	+
21	0	1	0	1	0	2	$e$	10	11	+
22	0	0	1	0	1	2	$e$	10	12	+
23	0	0	1	0	0	1	$o$	11	12	+
24	0	1	1	1	1	4	$e$	11	13	+
25	0	1	1	1	0	3	$o$	12	13	+
26	0	0	1	1	0	2	$e$	12	14	+
27	1	0	1	0	1	3	$o$	13	14	+
28	1	1	1	1	0	4	$e$	13	15	+
29	1	1	1	1	1	5	$o$	14	15	+
30	1	0	1	1	0	3	$o$	15	15	+
?	1	1	0	1	0	3	$o$	?	?	?
?	1	0	1	1	1	4	$e$	?	?	?

Additional files

Transparent reporting form: https://doi.org/10.7554/eLife.28629.015
Download elife-28629-transrepform-v1.docx

Download links

A two-part list of links to download the article, or parts of the article, in various formats.

Downloads (link to download the article as PDF)

Article PDF

Open citations (links to open the citations from this article in various online reference manager services)

Mendeley

Cite this article (links to download the citations from this article in formats compatible with various reference manager tools)

Kristina Crona
Alex Gavryushkin
Devin Greene
Niko Beerenwinkel

(2017)

Inferring genetic interactions from comparative fitness data

eLife 6:e28629.

https://doi.org/10.7554/eLife.28629

Figures

All $24$ rank orders of the biallelic two-locus system, where the $16$ colored rank orders imply epistasis.

(a) A partial fitness order of genotypes.

For a biallelic two-locus system where the genotype $00$ has the lowest fitness, there are four fitness graphs.

The fitness graph (a) implies three way epistasis, the graph (b) implies epistasis, but not higher order epistasis, and (c) does not imply epistasis, since all arrows point up.

Interactions detected from fitness summary statistics and from rank orders.

The TEM-family of $β$ -lactamase contributes to antibiotic resistance problems in hospitals.

The fitness graph shows a four-locus system for the filamentous fungus Aspergillus Niger.

The fitness graph is compatible with the two rank orders (5) and (6).

An example of an isomorphism.

All 54 fitness graph types.

Tables

Numbers and fractions of rank orders that imply $n$ -way epistasis.

Comparison of the rank order method with t-test.

Data from (Franke et al., 2011) on a 5-locus system determined by the mutations fwnA1, argH12, pyrA5, leuA1, and pheA1.

Additional files

Transparent reporting form

Download links

Downloads (link to download the article as PDF)

Open citations (links to open the citations from this article in various online reference manager services)

Cite this article (links to download the citations from this article in formats compatible with various reference manager tools)

Be the first to read new articles from eLife

Share this article

Cite this article

All 24 rank orders of the biallelic two-locus system, where the 16 colored rank orders imply epistasis.

(a) A partial fitness order of genotypes.

For a biallelic two-locus system where the genotype 00 has the lowest fitness, there are four fitness graphs.

The fitness graph (a) implies three way epistasis, the graph (b) implies epistasis, but not higher order epistasis, and (c) does not imply epistasis, since all arrows point up.

Interactions detected from fitness summary statistics and from rank orders.

The TEM-family of β-lactamase contributes to antibiotic resistance problems in hospitals.

The fitness graph shows a four-locus system for the filamentous fungus Aspergillus Niger.

The fitness graph is compatible with the two rank orders (5) and (6).

An example of an isomorphism.

All 54 fitness graph types.

Numbers and fractions of rank orders that imply n-way epistasis.

Comparison of the rank order method with t-test.

Data from (Franke et al., 2011) on a 5-locus system determined by the mutations fwnA1, argH12, pyrA5, leuA1, and pheA1.

Transparent reporting form

Downloads (link to download the article as PDF)

Open citations (links to open the citations from this article in various online reference manager services)

Cite this article (links to download the citations from this article in formats compatible with various reference manager tools)

All $24$ rank orders of the biallelic two-locus system, where the $16$ colored rank orders imply epistasis.

For a biallelic two-locus system where the genotype $00$ has the lowest fitness, there are four fitness graphs.

The TEM-family of $β$ -lactamase contributes to antibiotic resistance problems in hospitals.

Numbers and fractions of rank orders that imply $n$ -way epistasis.