Swimming eukaryotic microorganisms exhibit a universal speed distribution

Version of Record

Accepted for publication after peer review and revision.

Download
Cite
Share
CommentOpen annotations (there are currently 0 annotations on this page).

Version of Record published: July 16, 2019 (This version)
Accepted: June 29, 2019
Received: January 5, 2019

1. Of interest
Vasculature: Exploring the dynamics of vascular adaptation

Thomas S Shimizu, E Toby Kiers, Howard A Stone

Insight May 25, 2023
Further reading

Article
Figures and data
Abstract
Introduction
Results and discussion
Materials and methods
Appendix 1
Appendix 2
Data availability
References
Decision letter
Author response
Article and author information
Metrics

Abstract

One approach to quantifying biological diversity consists of characterizing the statistical distribution of specific properties of a taxonomic group or habitat. Microorganisms living in fluid environments, and for whom motility is key, exploit propulsion resulting from a rich variety of shapes, forms, and swimming strategies. Here, we explore the variability of swimming speed for unicellular eukaryotes based on published data. The data naturally partitions into that from flagellates (with a small number of flagella) and from ciliates (with tens or more). Despite the morphological and size differences between these groups, each of the two probability distributions of swimming speed are accurately represented by log-normal distributions, with good agreement holding even to fourth moments. Scaling of the distributions by a characteristic speed for each data set leads to a collapse onto an apparently universal distribution. These results suggest a universal way for ecological niches to be populated by abundant microorganisms.

https://doi.org/10.7554/eLife.44907.001

Introduction

Unicellular eukaryotes comprise a vast, diverse group of organisms that covers virtually all environments and habitats, displaying a menagerie of shapes and forms. Hundreds of species of the ciliate genus Paramecium (Wichterman, 1986) or flagellated Euglena (Buetow, 2011) are found in marine, brackish, and freshwater reservoirs; the green algae Chlamydomonas is distributed in soil and fresh water world-wide (Harris et al., 2009); parasites from the genus Giardia colonize intestines of several vertebrates (Adam, 2001). One of the shared features of these organisms is their motility, crucial for nutrient acquisition and avoidance of danger (Bray, 2001). In the process of evolution, single-celled organisms have developed in a variety of directions, and thus their rich morphology results in a large spectrum of swimming modes (Cappuccinelli, 1980).

Many swimming eukaryotes actuate tail-like appendages called flagella or cilia in order to generate the required thrust (Sleigh, 1975). This is achieved by actively generating deformations along the flagellum, giving rise to a complex waveform. The flagellar axoneme itself is a bundle of nine pairs of microtubule doublets surrounding two central microtubules, termed the '9 + 2' structure (Nicastro et al., 2005), and cross-linking dynein motors, powered by ATP hydrolysis, perform mechanical work by promoting the relative sliding of filaments, resulting in bending deformations.

Although eukaryotic flagella exhibit a diversity of forms and functions (Moran et al., 2014), two large families, ‘flagellates’ and ‘ciliates’, can be distinguished by the shape and beating pattern of their flagella. Flagellates typically have a small number of long flagella distributed along the bodies, and they actuate them to generate thrust. The set of observed movement sequences includes planar undulatory waves and traveling helical waves, either from the base to the tip, or in the opposite direction (Jahn and Votta, 1972; Brennen and Winet, 1977). Flagella attached to the same body might follow different beating patterns, leading to a complex locomotion strategy that often relies also on the resistance the cell body poses to the fluid. In contrast, propulsion of ciliates derives from the motion of a layer of densely-packed and collectively-moving cilia, which are short hair-like flagella covering their bodies. The seminal review paper of Brennen and Winet (1977) lists a few examples from both groups, highlighting their shape, beat form, geometric characteristics and swimming properties. Cilia may also be used for transport of the surrounding fluid, and their cooperativity can lead to directed flow generation. In higher organisms this can be crucial for internal transport processes, as in cytoplasmic streaming within plant cells (Allen and Allen, 1978), or the transport of ova from the ovary to the uterus in female mammals (Lyons et al., 2006).

Here, we turn our attention to these two morphologically different groups of swimmers to explore the variability of their propulsion dynamics within broad taxonomic groups. To this end, we have collected swimming speed data from literature for flagellated eukaryotes and ciliates and analyze them separately (we do not include spermatozoa since they lack (ironically) the capability to reproduce and are thus not living organisms; their swimming characteristics have been studied by Tam and Hosoi, 2011). A careful examination of the statistical properties of the speed distributions for flagellates and ciliates shows that they are not only both captured by log-normal distributions but that, upon rescaling the data by a characteristic swimming speed for each data set, the speed distributions in both types of organisms are essentially identical.

Results and discussion

We have collected swimming data on 189 unicellular eukaryotic microorganisms ( $N_{fl} = 112$ flagellates and $N_{cil} = 77$ ciliates) (see Appendix 1 and Source data 1). Figure 1 shows a tree encompassing the phyla of organisms studied and sketches of a representative organism from each phylum. A large morphological variation is clearly visible. In addition, we delineate the branches involving aquatic organisms and parasitic species living within hosts. Both groups include ciliates and flagellates.

Figure 1

Download asset Open asset

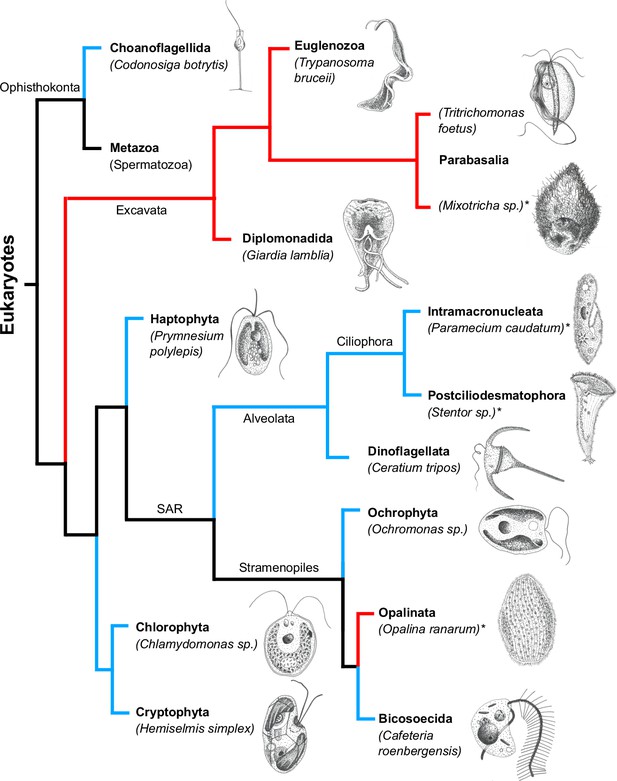
The tree of life (cladogram) for unicellular eukaryotes encompassing the phyla of organisms analyzed in the present study.

Aquatic organisms (living in marine, brackish, or freshwater environments) have their branches drawn in blue while parasitic organisms have their branches drawn in red. Ciliates are indicated by an asterisk after their names. For each phylum marked in bold font, a representative organism has been sketched next to its name. Phylogenetic data from Hinchliff et al. (2015).

https://doi.org/10.7554/eLife.44907.002

Due to the morphological and size differences between ciliates and flagellates, we investigate separately the statistical properties of each. Figure 2 shows the two swimming speed histograms superimposed, based on the raw distributions shown in Figure 2—figure supplement 1, where bin widths have been adjusted to their respective samples using the Freedman-Diaconis rule (see Materials and methods). Ciliates span a much larger range of speeds, up to 7 mm/s, whereas generally smaller flagellates remain in the sub-mm/s range. The inset shows that the number of flagella in both groups leads to a clear division. To compare the two groups further, we have also collected information on the characteristic sizes of swimmers from the available literature, which we list in Appendix 1. The average cell size differs fourfold between the populations (31 µm for flagellates and 132 µm for ciliates) and the distributions, plotted in Figure 2—figure supplement 2, are biased towards the low-size end but they are quantitatively different. In order to explore the physical conditions, we used the data on sizes and speeds to compute the Reynolds number $Re = U L / ν$ for each organism, where $ν = η / ρ$ is the kinematic viscosity of water, with $η$ the viscosity and $ρ$ the density. Since almost no data was available for the viscosity of the fluid in swimming speed measurements, we assumed the standard value $ν = 10^{- 6} m^{2} / s$ for water for all organisms. The distribution of Reynolds numbers (Figure 2—figure supplement 3), shows that ciliates and flagellates operate in different ranges of $Re$ , although for both groups $Re < 1$ , imposing on them the same limitations of inertia-less Stokes flow (Purcell, 1977; Lauga and Powers, 2009).

Figure 2 with 3 supplements see all

Download asset Open asset

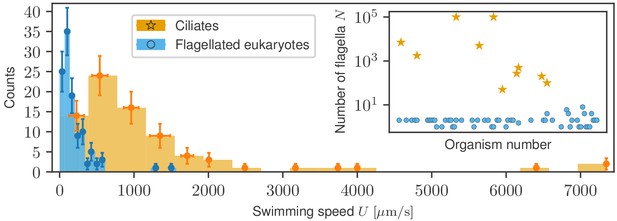
Histograms of swimming speed for ciliates and flagellates demonstrate a similar character but different scales of velocities.

Data points represent the mean and standard deviation of the data in each bin; horizontal error bars represent variability within each bin, vertical error bars show the standard deviation of the count. Inset: number of flagella displayed, where available, for each organism exhibits a clear morphological division between ciliates and flagellates.

https://doi.org/10.7554/eLife.44907.003

Furthermore, studies of green algae (Short et al., 2006; Goldstein, 2015) show that an important distinction between the smaller, flagellated species and the largest multicellular ones involves the relative importance of advection and diffusion, as captured by the Péclet number $P e = U L / D$ , where $L$ is a typical organism size and $D$ is the diffusion constant of a relevant molecular species. Using the average size $L$ of the cell body in each group of the present study ( $L_{fl} = 31 μ m$ , $L_{cil} = 132 μ m$ ) and the median swimming speeds ( $U_{fl} = 127 m / s$ , $U_{c i l} = 784 m / s$ ), and taking $D = 10^{3} (μ m)^{2} / s$ , we find $P e_{fl} \sim 3.9$ and $P e_{cil} \sim 103$ , which further justifies analyzing the groups separately; they live in different physical regimes.

Examination of the mean, variance, kurtosis, and higher moments of the data sets suggest that the probabilities $P (U)$ of the swimming speed are well-described by log-normal distributions,

P (U) = \frac{1}{U σ \sqrt{2 π}} \exp (- \frac{{(\ln U - μ)}^{2}}{2 σ^{2}}),

normalized as $\int_{0}^{\infty} 𝑑 U P (U) = 1$ , where $μ$ and $σ$ are the mean and the standard deviation of $\ln U$ . The median $M$ of the distribution is $e^{μ}$ , with units of speed. Log-normal distributions are widely observed across nature in areas such as ecology, physiology, geology and climate science, serving as an empirical model for complex processes shaping a system with many potentially interacting elements (Limpert et al., 2001), particularly when the underlying processes involve proportionate fluctuations or multiplicative noise (Koch, 1966).

The results of fitting (see Materials and methods) are plotted in Figure 3, where the best fits are presented as solid curves, with the shaded areas representing 95% confidence intervals. For flagellates, we find the $M_{fl} = 127 m / s$ and $σ_{fl} = 0.978$ while for ciliates, we obtain $M_{cil} = 784 m / s$ and $σ_{cil} = 0.936$ . Log-normal distributions are known to emerge from an (imperfect) analogy to the Gaussian central limit theorem (see Materials and methods). Since the data are accurately described by this distribution, we conclude that the published literature includes a sufficiently large amount of unbiased data to be able to see the whole distribution.

Figure 3 with 1 supplement see all

Download asset Open asset

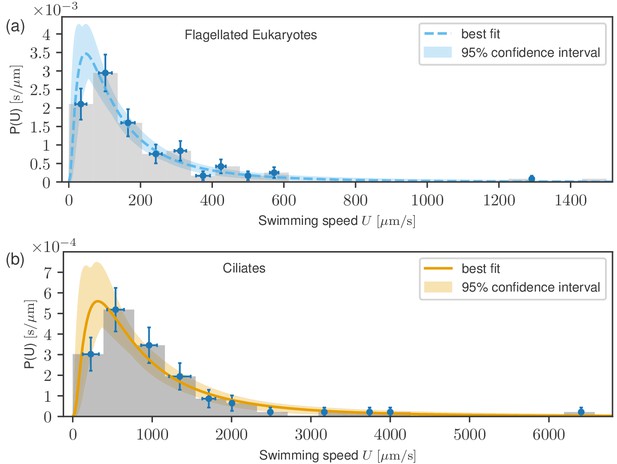
Probability distribution functions of swimming speeds for flagellates (a) and ciliates (b) with the fitted log-normal distributions.

Data points represent uncertainties as in Figure 2. Despite the markedly different scales of the distributions, they have similar shapes.

https://doi.org/10.7554/eLife.44907.007

We next compare the statistical variability within groups by examining rescaled distributions (Goldstein, 2018). As each has a characteristic speed $M$ , we align the peaks by plotting the distributions versus the variable $U / M$ for each group. Since $P$ has units of 1/speed, we are thus led to the form $P (U, M) = M^{- 1} F (U / M)$ for some function $F$ . For the log-normal distribution, with $M$ the median, we find

F (ξ) = \frac{1}{ξ σ \sqrt{2 π}} \exp (- \frac{\ln^{2} ξ}{2 σ^{2}}),

which now depends on the single parameter $σ$ and has a median of unity by construction. To study the similarity of the two distributions we plot the functions $F = M P (U / M)$ for each. As seen in Figure 4, the rescaled distributions are essentially indistinguishable, and this can be traced back to the near identical values of the variances $σ$ , which are within 5% of each other. The fitting uncertainties shown shaded in Figure 4 suggest a very similar range of variability of the fitted distributions. Furthermore, both the integrated absolute difference between the distributions (0.028) and the Kullback-Leibler divergence (0.0016) are very small (see Materials and methods), demonstrating the close similarity of the two distributions. This similarity is robust to the choice of characteristic speed, as shown in Figure 4—figure supplement 1, where the arithmetic mean $U^{*}$ is used in place of the median.

Figure 4 with 1 supplement see all

Download asset Open asset

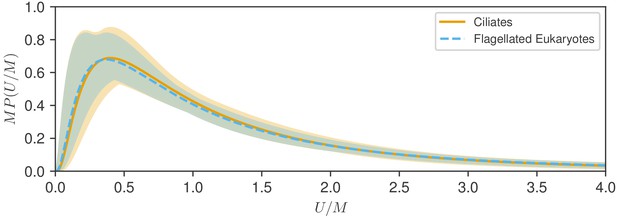
Test of rescaling hypothesis.

Shown are the two fitted log-normal curves for flagellates and ciliates, each multiplied by the distribution median $M$ , plotted versus speed normalized by $M$ . The distributions for show remarkable similarity and uncertainty of estimation.

https://doi.org/10.7554/eLife.44907.009

In living cells, the sources for intrinsic variability within organisms are well characterized on the molecular and cellular level (Kirkwood et al., 2005) but less is known about variability within taxonomic groups. By dividing unicellular eukaryotes into two major groups on the basis of their difference in morphology, size and swimming strategy, we were able to capture in this paper the log-normal variability within each subset. Using a statistical analysis of the distributions as functions of the median swimming speed for each population we further found an almost identical distribution of swimming speeds for both types of organisms. Our results suggest that the observed log-normal randomness captures a universal way for ecological niches to be populated by abundant microorganisms with similar propulsion characteristics. We note, however, that the distributions of swimming speeds among species do not necessarily reflect the distributions of swimming speeds among individuals, for which we have no available data.

Materials and methods

Data collection

Request a detailed protocol

Data for ciliates were sourced from 26 research articles, while that for flagellates were extracted from 48 papers (see Appendix 1). Notably, swimming speeds reported in the various studies have been measured under different physiological and environmental conditions, including temperature, viscosity, salinity, oxygenation, pH and light. Therefore we consider the data not as representative of a uniform environment, but instead as arising from a random sampling of a wide range of environmental conditions. In cases where no explicit figure was given for $U$ in a paper, estimates were made using other available data where possible. Size of swimmers has also been included as a characteristic length for each organism. This, however, does not reflect the spread and diversity of sizes within populations of individual but is rather an indication of a typical size, as in the considered studies these data were not available. Information on anisotropy (different width/length) is also not included.

No explicit criteria were imposed for the inclusion in the analyses, apart from the biological classification (i.e. whether the organisms were unicellular eukaryotic ciliates/flagellates). We have used all the data found in literature for these organisms over the course of an extensive search. Since no selection was made, we believe that the observed statistical properties are representative for these groups.

Data processing and fitting the log-normal distribution

Request a detailed protocol

Bin widths in histograms in Figure 2 and Figure 3 have been chosen separately for ciliates and flagellated eukaryotes according to the Freedman-Diaconis rule (Freedman and Diaconis, 1981) taking into account the respective sample sizes and the spread of distributions. The bin width $b$ is then given by the number of observations $N$ and the interquartile range of the data $IQR$ as

b = 2 \frac{IQR}{N^{1 / 3}} .

Within each bin in Figure 3, we calculate the mean and the standard deviation for the binned data, which constitute the horizontal error bars. The vertical error bars reflect the uncertainty in the number of counts $N_{j}$ in bin $j$ . This is estimated to be Poissonian, and thus the absolute error amounts to $\sqrt{N_{j}}$ . Notably, the relative error decays with the number of counts as $1 / \sqrt{N_{j}}$ .

In fitting the data, we employ the log-normal distribution Equation (1). In general, from from data comprising $N$ measurements, labelled $x_{i}$ ( $i = 1, \dots, N$ ), the $n$ -th arithmetic moment $ℳ_{n}$ is the expectation $𝔼 (X^{n})$ , or

ℳ_{n} = \frac{1}{N} \sum_{i = 1}^{N} x_{i}^{n}

Medians of the data were found by sorting the list of values and picking the middlemost value. For a log-normal distribution, the arithmetic moments are given solely by $μ$ and $σ$ of the associated normal distribution as

ℳ_{n} = M^{n} Σ^{n^{2}},

where we have defined $M = \exp (μ)$ and $Σ = \exp (σ^{2} / 2)$ , and note that $M$ is the median of the distribution. Thus, the mean is $M Σ$ and the variance is $M^{2} Σ^{2} (Σ^{2} - 1)$ . From the first and second moments, we estimate

μ = \ln (\frac{ℳ_{1}^{2}}{\sqrt{ℳ_{2}}}) and σ^{2} = \ln (\frac{ℳ_{2}}{ℳ_{1}^{2}}) .

Having estimated $μ$ and $σ$ , we can compute the higher order moments from Equation (5) and compare to those calculated directly from the data, as shown in Figure 3—figure supplement 1.

To fit the data, we have used both the MATLAB fitting routines and the Python scipy.stats module. From these fits we estimated the shape and scale parameters and the 95% confidence intervals in Figure 3 and Figure 4. We emphasize that the fitting procedures use the raw data via the maximum likelihood estimation method, and not the processed histograms, hence the estimated parameters are insensitive to the binning procedure.

For rescaled distributions, the average velocity for each group of organisms was calculated as $U^{*} = \frac{1}{N_{i}} \sum_{i = 1}^{N_{i}} U_{i}$ , with $i \in {cil, fl}$ . Then, data in each subset have been rescaled by the area under the fitted curve to ensure that the resulting probability density functions $p_{i}$ are normalized as

\int_{0}^{\infty} p_{i} (x) d x = 1 .

In characterizations of biological or ecological diversity, it is often assumed that the examined variables are Gaussian, and thus the distribution of many uncorrelated variables attains the normal distribution by virtue of the Central Limit Theorem (CLT). In the case when random variables in question are positive and have a log-normal distribution, no analogous explicit analytic result is available. Despite that, there is general agreement that a sum of independent log-normal random variables can be well approximated by another log-normal random variable. It has been proven by Szyszkowicz and Yanikome (2009) that the sum of identically distributed equally and positively correlated joint log-normal distributions converges to a log-normal distribution of known characteristics but for uncorrelated variables only estimations are available (Beaulieu et al., 1995). We use these results to conclude that our distributions contain enough data to be unbiased and seen in full.

Comparisons of distributions

Request a detailed protocol

In order to quantify the differences between the fitted distributions, we define the integrated absolute difference $Δ$ between two probability distributions $p (x)$ and $q (x)$ ( $x > 0$ ) as

Δ = \int_{0}^{\infty} | p (x) - q (x) | d x .

As the probability distributions are normalized, this is a measure of their relative ’distance’. As a second measure, we use the Kullback-Leibler divergence (Kullback and Leibler, 1951),

D (p, q) = \int_{0}^{\infty} p (x) \ln (\frac{p (x)}{q (x)}) d x .

Note that $D (p, q) \neq D (q, p)$ and therefore $D$ is not a distance metric in the space of probability distributions.

Appendix 1

The Appendix contains the data which form the basis of our study. The tables contain data on the sizes and swimming speed of ciliates organisms and flagellated eukaryotes from the existing literature. Data for ciliates were sourced from 26 research articles, while data for the flagellates were extracted from 48 papers. In the cases where two or more sources reported contrasting figures for the swimming speed, the average value is reported in our tables. The data itself is available in Source data 1.

Data for swimming flagellates

Abbreviations: dflg. – dinoflagellata; dph – dinophyceae; chlph. – chlorophyta; ochph. (het.) –ochrophyta (heterokont); srcm. – sarcomastigophora, pyr. – pyramimonadophyceae; prym. – prymnesiophyceae; dict. – dictyochophyceae; crypt. – cryptophyceae; chrys. – chrysophyceae

Species	Phylum	Class	$L$ [ $μ m$ ]	$U$ [ $μ m / s$ ]	References
Alexandrium minutum	dflg.	dph.	21.7	222.5	(Lewis et al., 2006)
Alexandrium ostenfeldii	dflg.	dph.	41.1	110.5	(Lewis et al., 2006)
Alexandrium tamarense	dflg.	dph.	26.7	200	(Lewis et al., 2006)
Amphidinium britannicum	dflg.	dph.	51.2	68.7	(Bauerfeind et al., 1986)
Amphidinium carterae	dflg.	dph.	16	81.55	(Gittleson et al., 1974; Bauerfeind et al., 1986)
Amphidinium klebsi	dflg.	dph.	35	73.9	(Gittleson et al., 1974)
Apedinella spinifera	ochph. (het.)	dict.	8.25	132.5	(Throndsen, 1973)
Bodo designis	euglenozoa	kinetoplastea	5.5	39	(Visser and Kiørboe, 2006)
Brachiomonas submarina	chlph.	chlorophyceae	27.5	96	(Bauerfeind et al., 1986)
Cachonina (Heterocapsa) niei	dflg.	dph.	21.4	302.8	(Levandowsky and Kaneta, 1987; Kamykowski and Zentara, 1977)
Cafeteria roenbergensis	bygira (heterokont)	bicosoecida	2	94.9	(Fenchel and Blackburn, 1999)
Ceratium cornutum	dflg.	dph.	122.3	177.75	(Levandowsky and Kaneta, 1987; Metzner, 1929)
Ceratium furca	dflg.	dph.	122.5	194	(Peters, 1929)
Ceratium fusus	dflg.	dph.	307.5	156.25	(Peters, 1929)
Ceratium hirundinella	dflg.	dph.	397.5	236.1	(Levandowsky and Kaneta, 1987)
Ceratium horridum	dflg.	dph.	225	20.8	(Peters, 1929)
Ceratium lineatus	dflg.	dph.	82.1	36	(Fenchel, 2001)
Ceratium longipes	dflg.	dph.	210	166	(Peters, 1929)
Ceratium macroceros	dflg.	dph.	50	15.4	(Peters, 1929)
Ceratium tripos	dflg.	dph.	152.3	121.7	(Peters, 1929; Bauerfeind et al., 1986)
Chilomonas paramecium	cryptophyta	crypt.	30	111.25	(Lee, 1954; Jahn and Bovee, 1967; Gittleson et al., 1974)
Chlamydomonas reinhardtii	chlph.	chlorophyceae	10	130	(Gittleson et al., 1974; Roberts, 1981; Guasto et al., 2010)
Chlamydomonas moewusii	chlph.	chlorophyceae	12.5	128	(Gittleson et al., 1974)
Chlamydomonas sp.	chlph.	chlorophyceae	13	63.2	(Lowndes, 1944; Lowndes, 1941; Bauerfeind et al., 1986)
Crithidia deanei	euglenozoa	kinetoplastea	7.4	45.6	(Gadelha et al., 2007)
Crithidia fasciculata	euglenozoa	kinetoplastea	11.1	54.3	(Gadelha et al., 2007)
Crithidia (Strigomonas) oncopelti	euglenozoa	kinetoplastea	8 .1	18.5	(Roberts, 1981; Gittleson et al., 1974)
Crypthecodinium cohnii	dflg.	dph.	n/a	122.8	(Fenchel, 2001)
Dinophysis acuta	dflg.	dph.	65	500	(Peters, 1929)
Dinophysis ovum	dflg.	dph.	45	160	(Buskey et al., 1993)
Dunaliella sp.	chlph.	chlorophyceae	10.8	173.5	(Gittleson et al., 1974; Bauerfeind et al., 1986)
Euglena gracilis	euglenozoa	euglenida (eugl.)	47.5	111.25	(Lee, 1954; Jahn and Bovee, 1967; Gittleson et al., 1974)
Euglena viridis	euglenozoa	euglenida (eugl.)	58	80	(Holwill, 1975; Roberts, 1981; Lowndes, 1941)
Eutreptiella gymnastica	euglenozoa	euglenida (aphagea)	23.5	237.5	(Throndsen, 1973)
Eutreptiella sp. R	euglenozoa	euglenida	50	135	(Throndsen, 1973)
Exuviaella baltica (Prorocentrum balticum)	dflg.	dph.	15.5	138.9	(Wheeler, 1966)
Giardia lamblia	srcm.	zoomastigophora	11.25	26	(Lenaghan et al., 2011; Campanati et al., 2002; Chen et al., 2012)
Gonyaulax polyedra	dflg.	dph.	39.2	254.05	(Hand et al., 1965; Gittleson et al., 1974; Kamykowski et al., 1992)
Gonyaulax polygramma	dflg.	dph.	46.2	500	(Levandowsky and Kaneta, 1987)
Gymnodinium aureolum	dflg.	dph.	n/a	394	(Meunier et al., 2013)
Gymnodinium sanguineum (splendens)	dflg.	dph.	47.6	220.5	(Kamykowski et al., 1992; Levandowsky and Kaneta, 1987)
Gymnodinium simplex	dflg.	dph.	10.6	559	(Jakobsen et al., 2006)
Gyrodinium aureolum	dflg.	dph.	30.5	139	(Bauerfeind et al., 1986; Throndsen, 1973)
Gyrodinium dorsum (bi-flagellated)	dflg.	dph.	37.5	324	(Hand et al., 1965; Gittleson et al., 1974; Kamykowski et al., 1992; Levandowsky and Kaneta, 1987; Brennen and Winet, 1977)
Gyrodinium dorsum (uni-flagellated)	dflg.	dph.	34.5	148.35	(Hand and Schmidt, 1975)
Hemidinium nasutum	dflg.	dph.	27.2	105.6	(Levandowsky and Kaneta, 1987; Metzner, 1929)
Hemiselmis simplex	cryptophyta	crypt.	5.25	325	(Throndsen, 1973)
Heterocapsa pygmea	dflg.	dph.	13.5	102.35	(Bauerfeind et al., 1986)
Heterocapsa rotundata	dflg.	dph.	12.5	323	(Jakobsen et al., 2006)
Heterocapsa triquetra	dflg.	dph.	17	97	(Visser and Kiørboe, 2006)
Heteromastix pyriformis	chlph.	nephrophyseae	6	87.5	(Throndsen, 1973)
Hymenomonas carterae	haptophyta	prym.	12.5	87	(Bauerfeind et al., 1986)
Katodinium rotundatum (Heterocapsa rotundata)	dflg.	dph.	10.8	425	(Levandowsky and Kaneta, 1987; Throndsen, 1973)
Leishmania major	euglenozoa	kinetoplastea	12.5	36.4	(Gadelha et al., 2007)
Menoidium cultellus	euglenozoa	euglenida (eugl.)	45	136.75	(Holwill, 1975; Votta et al., 1971)
Menoidium incurvum	euglenozoa	euglenida (eugl.)	25	50	(Lowndes, 1941; Gittleson et al., 1974)
Micromonas pusilla	chlph.	mamiellophyceae	2	58.5	(Bauerfeind et al., 1986; Throndsen, 1973)
Monas stigmata	ochph. (het.)	chrys.	6	269	(Gittleson et al., 1974)
Monostroma angicava	chlph.	ulvophyceae	6.7	170.55	(Togashi et al., 1997)
Nephroselmis pyriformis	chlph.	nephrophyseae	4.8	163.5	(Bauerfeind et al., 1986)
Oblea rotunda	dflg.	dph.	20	420	(Buskey et al., 1993)
Ochromonas danica	ochph. (het.)	chrys.	8.7	77	(Holwill and Peters, 1974)
Ochromonas malhamensis	ochph. (het.)	chrys.	3	57.5	(Holwill, 1974)
Ochromonas minima	ochph. (het.)	chrys.	5	75	(Throndsen, 1973)
Olisthodiscus luteus	ochph. (het.)	raphidophyceae	22.5	90	(Bauerfeind et al., 1986; Throndsen, 1973)
Oxyrrhis marina	dflg.	oxyrrhea	39.5	300	(Boakes et al., 2011; Fenchel, 2001)
Paragymnodinium shiwhaense	dflg.	dph.	10.9	571	(Meunier et al., 2013)
Paraphysomonas vestita	ochph. (het.)	chrys.	14.7	116.85	(Christensen-Dalsgaard and Fenchel, 2004)
Pavlova lutheri	haptophyta	pavlovophyceae	6.5	126	(Bauerfeind et al., 1986)
Peranema trichophorum	euglenozoa	euglenida (heteronematales)	45	20	(Lowndes, 1941; Gittleson et al., 1974; Brennen and Winet, 1977)
Peridinium bipes	dflg.	dph.	42.9	291	(Fenchel, 2001)
Peridinium cf. quinquecorne	dflg.	dph.	19	1500	(Bauerfeind et al., 1986; Levandowsky and Kaneta, 1987; Horstmann, 1980)
Peridinium cinctum	dflg.	dph.	47.5	120	(Bauerfeind et al., 1986; Levandowsky and Kaneta, 1987; Metzner, 1929)
Peridinium (Protoperidinium) claudicans	dflg.	dph.	77.5	229	(Peters, 1929)
Peridinium (Protoperidinium) crassipes	dflg.	dph.	102	100	(Peters, 1929)
Peridinium foliaceum	dflg.	dph.	30.6	185.2	(Kamykowski et al., 1992)
Peridinium (Bysmatrum) gregarium	dflg.	dph.	32.5	1291.7	(Levandowsky and Kaneta, 1987)
Peridinium (Protoperidinium) ovatum	dflg.	dph.	61	187.5	(Peters, 1929)
Peridinium (Peridiniopsis) penardii	dflg.	dph.	28.8	417	(Sibley et al., 1974)
Peridinium (Protoperidinium) pentagonum	dflg.	dph.	92.5	266.5	(Peters, 1929)
Peridinium (Protoperidinium) subinerme	dflg.	dph.	50	285	(Peters, 1929)
Peridinium trochoideum	dflg.	dph.	25	53	(Levandowsky and Kaneta, 1987)
Peridinium umbonatum	dflg.	dph.	30	250	(Levandowsky and Kaneta, 1987; Metzner, 1929)
Phaeocystis pouchetii	haptophyta	prym.	6.3	88	(Bauerfeind et al., 1986)
Polytoma uvella	chlph.	chlorophyceae	22.5	100.9	(Lowndes, 1944; Gittleson et al., 1974; Lowndes, 1941)
Polytomella agilis	chlph.	chlorophyceae	12.4	150	(Gittleson and Jahn, 1968; Gittleson and Noble, 1973; Gittleson et al., 1974; Roberts, 1981)
Prorocentrum mariae-lebouriae	dflg.	dph.	14.8	141.05	(Kamykowski et al., 1992; Bauerfeind et al., 1986; Miyasaka et al., 1998)
Prorocentrum micans	dflg.	dph.	45	329.1	(Bauerfeind et al., 1986; Levandowsky and Kaneta, 1987)
Prorocentrum minimum	dflg.	dph.	15.1	107.7	(Bauerfeind et al., 1986; Miyasaka et al., 1998)
Prorocentrum redfieldii Bursa (P.triestinum)	dflg.	dph.	33.2	333.3	(Sournia, 1982)
Protoperidinium depressum	dflg.	dph.	132	450	(Buskey et al., 1993)
Protoperidinium granii (Ostf.) Balech	dflg.	dph.	57.5	86.1	(Sournia, 1982)
Protoperidinium pacificum	dflg.	dph.	54	410	(Buskey et al., 1993)
Prymnesium polylepis	haptophyta	prym.	9.1	45	(Dölger et al., 2017)
Prymnesium parvum	haptophyta	prym.	7.2	30	(Dölger et al., 2017)
Pseudopedinella pyriformis	ochph. (het.)	dict.	6.5	100	(Throndsen, 1973)
Pseudoscourfieldia marina	chlph.	pyr.	4.1	42	(Bauerfeind et al., 1986)
Pteridomonas danica	ochph. (het.)	dict.	5.5	179.45	(Christensen-Dalsgaard and Fenchel, 2004)
Pyramimonas amylifera	chlph.	pyr.	24.5	22.5	(Bauerfeind et al., 1986)
Pyramimonas cf. disomata	chlph.	pyr.	9	355	(Throndsen, 1973)
Rhabdomonas spiralis	euglenozoa	euglenida (aphagea)	27	120	(Holwill, 1975)
Rhodomonas salina	cryptophyta	crypt.	14.5	588.5	(Jakobsen et al., 2006; Meunier et al., 2013)
Scrippsiella trochoidea	dflg.	dph.	25.3	87.6	(Kamykowski et al., 1992; Bauerfeind et al., 1986; Sournia, 1982)
Spumella sp.	ochph. (het.)	chrys.	10	25	(Visser and Kiørboe, 2006)
Teleaulax sp.	cryptophyta	crypt.	13.5	98	(Meunier et al., 2013)
Trypanosoma brucei	euglenozoa	kinetoplastea	18.8	20.5	(Rodríguez et al., 2009)
Trypanosoma cruzi	euglenozoa	kinetoplastea	20	172	(Jahn and Fonseca, 1963; Brennen and Winet, 1977)
Trypanosoma vivax	euglenozoa	kinetoplastea	23.5	29.5	(Bargul et al., 2016)
Trypanosoma evansi	euglenozoa	kinetoplastea	21.5	16.1	(Bargul et al., 2016)
Trypanosoma congolense	euglenozoa	kinetoplastea	18	9.7	(Bargul et al., 2016)
Tetraflagellochloris mauritanica	chlph.	chlorophyceae	4	300	(Barsanti et al., 2016)

https://doi.org/10.7554/eLife.44907.013

Appendix 2

Data for swimming ciliates

Abbreviations: imnc. = intramacronucleata; pcdph. = postciliodesmatophora; olig. – oligohymenophorea; spir. – spirotrichea; hettr. – heterotrichea; lit. – litostomatea; eugl. – euglenophyceae

Species	Phylum	Class	$L$ [ $μ m$ ]	$U$ [ $μ m / s$ ]	References
Amphileptus gigas	imnc.	lit.	808	608	(Bullington, 1925)
Amphorides quadrilineata	imnc.	spir.	138	490	(Buskey et al., 1993)
Balanion comatum	imnc.	prostomatea	16	220	(Visser and Kiørboe, 2006)
Blepharisma	pcdph.	hettr.	350	600	(Sleigh and Blake, 1977; Roberts, 1981)
Coleps hirtus	imnc.	prostomatea	94.5	686	(Bullington, 1925)
Coleps sp.	imnc.	prostomatea	78	523	(Bullington, 1925)
Colpidium striatum	imnc.	olig.	77	570	(Beveridge et al., 2010)
Condylostoma patens	pcdph.	hettr.	371	1061	(Bullington, 1925; Machemer, 1974)
Didinium nasutum	imnc.	lit.	140	1732	(Bullington, 1925; Machemer, 1974; Roberts, 1981; Sleigh and Blake, 1977)
Euplotes charon	imnc.	spir.	66	1053	(Bullington, 1925)
Euplotes patella	imnc.	spir.	202	1250	(Bullington, 1925)
Euplotes vannus	imnc.	spir.	82	446	(Wang et al., 2008; Ricci et al., 1997)
Eutintinnus cf. pinguis	imnc.	spir.	147	410	(Buskey et al., 1993)
Fabrea salina	pcdph.	hettr.	184.1	216	(Marangoni et al., 1995)
Favella panamensis	imnc.	spir.	238	600	(Buskey et al., 1993)
Favella sp.	imnc.	spir.	150	1080	(Buskey et al., 1993)
Frontonia sp.	imnc.	olig.	378.5	1632	(Bullington, 1925)
Halteria grandinella	imnc.	spir.	50	533	(Bullington, 1925; Gilbert, 1994)
Kerona polyporum	imnc.	spir.	107	476.5	(Bullington, 1925)
Laboea strobila	imnc.	spir.	100	810	(Buskey et al., 1993)
Lacrymaria lagenula	imnc.	lit.	45	909	(Bullington, 1925)
Lembadion bullinum	imnc.	olig.	43	415	(Bullington, 1925)
Lembus velifer	imnc.	olig.	87	200	(Bullington, 1925)
Mesodinium rubrum	imnc.	lit.	38	7350	(Jonsson and Tiselius, 1990; Riisgård and Larsen, 2009; Crawford and Lindholm, 1997)
Metopides contorta	imnc.	armophorea	115	359	(Bullington, 1925)
Nassula ambigua	imnc.	nassophorea	143	2004	(Bullington, 1925)
Nassula ornata	imnc.	nassophorea	282	750	(Bullington, 1925)
Opalina ranarum	placidozoa (heterokont)	opalinea	350	50	(Blake, 1975; Sleigh and Blake, 1977)
Ophryoglena sp.	imnc.	olig.	325	4000	(Machemer, 1974)
Opisthonecta henneg	imnc.	olig.	126	1197	(Machemer, 1974; Jahn and Hendrix, 1969)
Oxytricha bifara	imnc.	spir.	282	1210	(Bullington, 1925)
Oxytricha ferruginea	imnc.	spir.	150	400	(Bullington, 1925)
Oxytricha platystoma	imnc.	spir.	130	520	(Bullington, 1925)
Paramecium aurelia	imnc.	olig.	244	1650	(Bullington, 1925; Bullington, 1930)
Paramecium bursaria	imnc.	olig.	130	1541.5	(Bullington, 1925; Bullington, 1930)
Paramecium calkinsii	imnc.	olig.	124	1392	(Bullington, 1930; Bullington, 1925)
Paramecium caudatum	imnc.	olig.	225.5	2489.35	(Bullington, 1930; Jung et al., 2014)
Paramecium marinum	imnc.	olig.	115	930	(Bullington, 1925)
Paramecium multimicronucleatum	imnc.	olig.	251	3169.5	(Bullington, 1930)
Paramecium polycaryum	imnc.	olig.	91	1500	(Bullington, 1930)
Paramecium spp.	imnc.	olig.	200	975	(Jahn and Bovee, 1967; Sleigh and Blake, 1977; Roberts, 1981)
Paramecium tetraurelia	imnc.	olig.	124	784	(Funfak et al., 2015)
Paramecium woodruffi	imnc.	olig.	160	2013.5	(Bullington, 1930)
Porpostoma notatum	imnc.	olig.	107.7	1842.2	(Fenchel and Blackburn, 1999)
Prorodon teres	imnc.	prostomatea	175	1066	(Bullington, 1925)
Spathidium spathula	imnc.	lit.	204.5	526	(Bullington, 1925)
Spirostomum ambiguum	pcdph.	hettr.	1045	810	(Bullington, 1925)
Spirostomum sp.	pcdph.	hettr.	1000	1000	(Sleigh and Blake, 1977)
Spirostomum teres	pcdph.	hettr.	450	640	(Bullington, 1925)
Stenosemella steinii	imnc.	spir.	83	190	(Buskey et al., 1993)
Stentor caeruleus	pcdph.	hettr.	528.5	1500	(Bullington, 1925)
Stentor polymorphus	pcdph.	hettr.	208	887	(Bullington, 1925; Sleigh and Aiello, 1972; Sleigh, 1968)
Strobilidium spiralis	imnc.	spir.	60	330	(Buskey et al., 1993)
Strobilidium velox	imnc.	spir.	43	150	(Gilbert, 1994)
Strombidinopsis acuminatum	imnc.	spir.	80	390	(Buskey et al., 1993)
Strombidium claparedi	imnc.	spir.	69.5	3740	(Bullington, 1925)
Strombidium conicum	imnc.	spir.	75	570	(Buskey et al., 1993)
Strombidium sp.	imnc.	spir.	33	360	(Buskey et al., 1993)
Strombidium sulcatum	imnc.	spir.	32.5	995	(Fenchel and Jonsson, 1988; Fenchel and Blackburn, 1999 Fenchel and Blackburn, 1999)
Stylonichia sp.	imnc.	spir.	167	737.5	(Bullington, 1925; Machemer, 1974)
Tetrahymena pyriformis	imnc.	olig.	72.8	475.6	(Sleigh and Blake, 1977; Roberts, 1981; Brennen and Winet, 1977)
Tetrahymena thermophila	imnc.	olig.	46.7	204.5	(Wood et al., 2007)
Tillina magna	imnc.	colpodea	162.5	2000	(Bullington, 1925)
Tintinnopsis kofoidi	imnc.	spir.	100	400	(Buskey et al., 1993)
Tintinnopsis minuta	imnc.	spir.	40	60	(Buskey et al., 1993)
Tintinnopsis tubulosa	imnc.	spir.	95	160	(Buskey et al., 1993)
Tintinnopsis vasculum	imnc.	spir.	82	250	(Buskey et al., 1993)
Trachelocerca olor	pcdph.	karyorelictea	267.5	900	(Bullington, 1925)
Trachelocerca tenuicollis	pcdph.	karyorelictea	432	1111	(Bullington, 1925)
Uroleptus piscis	imnc.	spir.	203	487	(Bullington, 1925)
Uroleptus rattulus	imnc.	spir.	400	385	(Bullington, 1925)
Urocentrum turbo	imnc.	olig.	90	700	(Bullington, 1925)
Uronema filificum	imnc.	olig.	25.7	1372.7	(Fenchel and Blackburn, 1999)
Uronema marinum	imnc.	olig.	56.9	1010	(Fenchel and Blackburn, 1999)
Uronema sp.	imnc.	olig.	25	1175	(Sleigh and Blake, 1977; Roberts, 1981)
Uronychia transfuga	imnc.	spir.	118	6406	(Leonildi et al., 1998)
Uronychia setigera	imnc.	spir.	64	7347	(Leonildi et al., 1998)
Uronemella spp.	imnc.	olig.	28	250	(Petroff et al., 2015)

https://doi.org/10.7554/eLife.44907.014

Data availability

All data generated or analysed during this study are included in the manuscript.

References

1. Adam RD
(2001) Biology of giardia lamblia
Clinical Microbiology Reviews 14:447–475.
https://doi.org/10.1128/CMR.14.3.447-475.2001
- PubMed
- Google Scholar
1. Allen NS
2. Allen RD
(1978) Cytoplasmic streaming in green plants
Annual Review of Biophysics and Bioengineering 7:497–526.
https://doi.org/10.1146/annurev.bb.07.060178.002433
- PubMed
- Google Scholar
1. Bargul JL
2. Jung J
3. McOdimba FA
4. Omogo CO
5. Adung'a VO
6. Krüger T
7. Masiga DK
8. Engstler M
(2016) Species-Specific adaptations of trypanosome morphology and motility to the mammalian host
PLOS Pathogens 12:e1005448.
https://doi.org/10.1371/journal.ppat.1005448
- PubMed
- Google Scholar
(2016) Swimming patterns of the quadriflagellate Tetraflagellochloris mauritanica (Chlamydomonadales, chlorophyceae)
Journal of Phycology 52:209–218.
https://doi.org/10.1111/jpy.12384
- PubMed
- Google Scholar
(1986) Application of laser Doppler spectroscopy (LDS) in determining swimming velocities of motile phytoplankton
Marine Biology 93:323–327.
https://doi.org/10.1007/BF00401099
- Google Scholar
(1995) Estimating the distribution of a sum of independent lognormal random variables
IEEE Transactions on Communications 43:2869.
https://doi.org/10.1109/26.477480
- Google Scholar
(2010) Mechanisms of temperature-dependent swimming: the importance of physics, physiology and body size in determining protist swimming speed
Journal of Experimental Biology 213:4223–4231.
https://doi.org/10.1242/jeb.045435
- PubMed
- Google Scholar
Book
1. Blake JR
(1975)
Hydromechanical aspects of ciliary propulsion

In: Wu T, Brokaw C. J, Brennen C, editors. Swimming and Flying in Nature. New York: Plenum. pp. 185–209.
- Google Scholar
(2011) Analysis and modelling of swimming behaviour in Oxyrrhis marina
Journal of Plankton Research 33:641–649.
https://doi.org/10.1093/plankt/fbq136
- Google Scholar
Book
1. Bray D
(2001)
Cell Movements

New York: Garland Science.
- Google Scholar
1. Brennen C
2. Winet H
(1977) Fluid mechanics of propulsion by cilia and flagella
Annual Review of Fluid Mechanics 9:339–398.
https://doi.org/10.1146/annurev.fl.09.010177.002011
- Google Scholar
Book
1. Buetow DE
(2011) Euglena
American Cancer Society.
https://doi.org/10.1002/9780470015902.a0001964.pub3
- Google Scholar
1. Bullington WE
(1925)
A study of spiral movement in the ciliate infusoria

Archiv für Protistenkunde 50:219–274.
- Google Scholar
1. Bullington WE
(1930) A further study of spiraling in the ciliate Paramecium, with a note on morphology and taxonomy
Journal of Experimental Zoology 56:423–449.
https://doi.org/10.1002/jez.1400560404
- Google Scholar
(1993)
Locomotory patterns of microzooplankton: potential effects on food selectivity of larval fish

Bulletin of Marine Science 53:29–43.
- Google Scholar
(2002) Video-microscopy observations of fast dynamic processes in the protozoon Giardia lamblia
Cell Motility and the Cytoskeleton 51:213–224.
https://doi.org/10.1002/cm.10026
- PubMed
- Google Scholar
Book
1. Cappuccinelli P
(1980) The Movement of Eukaryotic Cells
Netherlands, Dordrecht: Springer.
https://doi.org/10.1007/978-94-009-5812-8_4
- Google Scholar
Conference
(2012) Analysis of dynamics and planar motion strategies of a swimming microorganism - Giardia lamblia
IEEE International Conference on Robotics and Automation. pp. 4204–4209.
https://doi.org/10.1109/ICRA.2012.6225014
- Google Scholar
1. Christensen-Dalsgaard KK
2. Fenchel T
(2004) Complex flagellar motions and swimming patterns of the flagellates Paraphysomonas vestita and Pteridomonas danica
Protist 155:79–87.
https://doi.org/10.1078/1434461000166
- PubMed
- Google Scholar
1. Crawford DW
2. Lindholm T
(1997) Some observations on vertical distribution and migration of the phototrophic ciliate Mesodinium rubrum (= Myrionecta rubra) in a stratified brackish inlet
Aquatic Microbial Ecology 13:267–274.
https://doi.org/10.3354/ame013267
- Google Scholar
(2017) Swimming and feeding of mixotrophic biflagellates
Scientific Reports 7:39892.
https://doi.org/10.1038/srep39892
- PubMed
- Google Scholar
1. Fenchel T
(2001) How dinoflagellates swim
Protist 152:329–338.
https://doi.org/10.1078/1434-4610-00071
- PubMed
- Google Scholar
1. Fenchel T
2. Blackburn N
(1999) Motile chemosensory behaviour of phagotrophic protists: mechanisms for and efficiency in congregating at food patches
Protist 150:325–336.
https://doi.org/10.1016/S1434-4610(99)70033-7
- PubMed
- Google Scholar
1. Fenchel T
2. Jonsson PR
(1988) The functional biology of Strombidium sulcatum, a marine oligotrich ciliate (Ciliophora, oligotrichina)
Marine Ecology Progress Series 48:1–15.
https://doi.org/10.3354/meps048001
- Google Scholar
1. Freedman D
2. Diaconis P
(1981)
Zeitschrift Fü Wahrscheinlichkeitstheorie Und Verwandte Gebiete

453–476, On the histogram as a density estimator: L2 theory, Zeitschrift Fü Wahrscheinlichkeitstheorie Und Verwandte Gebiete, 57, Springer.
- Google Scholar
(2015) Paramecium swimming and ciliary beating patterns: a study on four RNA interference mutations
Integrative Biology 7:90–100.
https://doi.org/10.1039/c4ib00181h
- PubMed
- Google Scholar
(2007) Flagellar and ciliary beating in trypanosome motility
Cell Motility and the Cytoskeleton 64:629–643.
https://doi.org/10.1002/cm.20210
- PubMed
- Google Scholar
1. Gilbert JJ
(1994) Jumping behavior in the oligotrich ciliates Strobilidium velox and Halteria grandinella, and its significance as a defense against rotifer predators
Microbial Ecology 27:189–200.
https://doi.org/10.1007/BF00165817
- PubMed
- Google Scholar
(1974) Locomotion in the marine dinoflagellate Amphidinium carterae (Hulburt)
Transactions of the American Microscopical Society 93:101–105.
https://doi.org/10.2307/3225224
- Google Scholar
1. Gittleson SM
2. Jahn TL
(1968) Flagellar activity of Polytomella agilis
Transactions of the American Microscopical Society 87:464–471.
https://doi.org/10.2307/3224220
- Google Scholar
1. Gittleson SM
2. Noble RM
(1973) Locomotion in Polytomella agilis and Polytoma uvella
Transactions of the American Microscopical Society 93:101–105.
https://doi.org/10.2307/3225176
- Google Scholar
1. Goldstein RE
(2015) Green algae as model organisms for biological fluid dynamics
Annual Review of Fluid Mechanics 47:343–375.
https://doi.org/10.1146/annurev-fluid-010313-141426
- PubMed
- Google Scholar
1. Goldstein RE
(2018) Are theoretical results 'Results'?
eLife 7:e40018.
https://doi.org/10.7554/eLife.40018
- PubMed
- Google Scholar
(2010) Oscillatory flows induced by microorganisms swimming in two dimensions
Physical Review Letters 105:168102.
https://doi.org/10.1103/PhysRevLett.105.168102
- PubMed
- Google Scholar
(1965) The effects of temperature and salinity change on swimming rate in the dinoflagellates, Gonyaulax and Gyrodinium
The Biological Bulletin 128:90–101.
https://doi.org/10.2307/1539392
- Google Scholar
1. Hand WG
2. Schmidt JA
(1975) Phototactic orientation by the marine dinoflagellate Gyrodinium dorsum kofoid. ii. flagellar activity and overall response mechanism
The Journal of Protozoology 22:494–498.
https://doi.org/10.1111/j.1550-7408.1975.tb05217.x
- Google Scholar
Book
(2009)
The Chlamydomonas Sourcebook (2nd edition)

London: Academic Press.
- Google Scholar
1. Hinchliff CE
2. Smith SA
3. Allman JF
4. Burleigh JG
5. Chaudhary R
6. Coghill LM
7. Crandall KA
8. Deng J
9. Drew BT
10. Gazis R
11. Gude K
12. Hibbett DS
13. Katz LA
14. Laughinghouse HD
15. McTavish EJ
16. Midford PE
17. Owen CL
18. Ree RH
19. Rees JA
20. Soltis DE
21. Williams T
22. Cranston KA
(2015) Synthesis of phylogeny and taxonomy into a comprehensive tree of life
PNAS 112:12764–12769.
https://doi.org/10.1073/pnas.1423041112
- PubMed
- Google Scholar
Book
1. Holwill MEJ
(1974)
Hydrodynamic aspects of ciliary and flagellar movement

In: Sleigh M. A, editors. Cilia and Flagella. London: Academic Press. pp. 143–176.
- Google Scholar
Book
1. Holwill MEJ
(1975)
The role of body oscillation in the propulsion of microorganisms

In: Wu T, Brokaw C. J, Brennen C, editors. Swimming and Flying in Nature. New York: Plenum. pp. 133–141.
- Google Scholar
1. Holwill ME
2. Peters PD
(1974) Dynamics of the hispid flagellum of Ochromonas danica. The role of mastigonemes
The Journal of Cell Biology 62:322–328.
https://doi.org/10.1083/jcb.62.2.322
- PubMed
- Google Scholar
1. Horstmann U
(1980) Observations on the peculiar diurnal migration of a red tide dinophyceae in tropical shallow waters
Journal of Phycology 16:481–485.
https://doi.org/10.1111/j.1529-8817.1980.tb03064.x
- Google Scholar
Book
1. Jahn TL
2. Bovee EC
(1967) Motile behavior of protozoa
In: Chen T. T, editors. Research in Protozoology. New York: Pergamon. pp. 41–200.
https://doi.org/10.1016/B978-0-08-011846-8.50005-0
- Google Scholar
1. Jahn TL
2. Fonseca JR
(1963)
Mechanisms of locomotion of flagellates: v: Trypanosoma lewisi and T. cruzi

The Journal of Protozoology 11:.
- Google Scholar
1. Jahn TL
2. Hendrix EM
(1969)
Locomotion of the telotrich ciliate Opisthonecta henneguyi

Revista De La Sociedad Mexicana De Historia Natural 30:103–131.
- Google Scholar
1. Jahn TL
2. Votta JJ
(1972) Locomotion of protozoa
Annual Review of Fluid Mechanics 4:93–116.
https://doi.org/10.1146/annurev.fl.04.010172.000521
- Google Scholar
(2006) Hydromechanical signaling between the ciliate Mesodinium pulex and motile protist prey
Aquatic Microbial Ecology 44:197–206.
https://doi.org/10.3354/ame044197
- Google Scholar
1. Jonsson PP
2. Tiselius P
(1990) Feeding behaviour, prey detection and capture efficiency of the copepod Acartia tonsa feeding on planktonic ciliates
Marine Ecology Progress Series 60:35–44.
https://doi.org/10.3354/meps060035
- Google Scholar
(2014) Evidence for two extremes of ciliary motor response in a single swimming microorganism
Biophysical Journal 106:106–113.
https://doi.org/10.1016/j.bpj.2013.11.3703
- PubMed
- Google Scholar
(1992)
Comparison of sinking velocity, swimming velocity, rotation and path characteristics among six marine dinoflagellate species

Marine Biology 113:319–328.
- Google Scholar
1. Kamykowski D
2. Zentara S-J
(1977) The diurnal vertical migration of motile phytoplankton through temperature gradients1
Limnology and Oceanography 22:148–151.
https://doi.org/10.4319/lo.1977.22.1.0148
- Google Scholar
(2005) What accounts for the wide variation in life span of genetically identical organisms reared in a constant environment?
Mechanisms of Ageing and Development 126:439–443.
https://doi.org/10.1016/j.mad.2004.09.008
- PubMed
- Google Scholar
1. Koch AL
(1966) The logarithm in biology. 1. Mechanisms generating the log-normal distribution exactly
Journal of Theoretical Biology 12:276–290.
https://doi.org/10.1016/0022-5193(66)90119-6
- PubMed
- Google Scholar
1. Kullback S
2. Leibler RA
(1951) On information and sufficiency
The Annals of Mathematical Statistics 22:79–86.
https://doi.org/10.1214/aoms/1177729694
- Google Scholar
1. Lauga E
2. Powers TR
(2009) The hydrodynamics of swimming microorganisms
Reports on Progress in Physics 72:096601.
https://doi.org/10.1088/0034-4885/72/9/096601
- Google Scholar
1. Lee JW
(1954)
The effect of pH on forward swimming in Euglena and Chilomonas

Transactions of the American Microscopical Society 84:395–406.
- Google Scholar
1. Lenaghan SC
2. Davis CA
3. Henson WR
4. Zhang Z
5. Zhang M
(2011) High-speed microscopic imaging of flagella motility and swimming in Giardia lamblia trophozoites
PNAS 108:E550–E558.
https://doi.org/10.1073/pnas.1106904108
- PubMed
- Google Scholar
1. Leonildi A
2. Erra F
3. Banchetti R
4. Ricci N
(1998) The ethograms of Uronychia transfuga and Uronychia setigera (ciliata, hypotrichida): a comparative approach for new insights into the behaviour of protozoa
European Journal of Protistology 34:426–435.
https://doi.org/10.1016/S0932-4739(98)80011-4
- Google Scholar
Book
1. Levandowsky M
2. Kaneta PJ
(1987)
Behaviour in dinoflagellates

In: Taylor F. J. R, editors. The Biology of Dinoflagellates (Botanical Monographs), 21. Blackwell Scientific. pp. 360–397.
- Google Scholar
1. Lewis NI
2. Xu W
3. Jericho SK
4. Kreuzer HJ
5. Jericho MH
6. Cembella AD
(2006) Swimming speed of three species of Alexandrium (Dinophyceae) as determined by digital in-line holography
Phycologia 45:61–70.
https://doi.org/10.2216/04-59.1
- Google Scholar
(2001) Log-normal distributions across the sciences: keys and clues
BioScience 51:341–352.
https://doi.org/10.1641/0006-3568(2001)051[0341:LNDATS]2.0.CO;2
- Google Scholar
1. Lowndes AG
(1941) On flagellar movement in unicellular organisms
Proceedings of the Zoological Society of London A111:111–134.
https://doi.org/10.1111/j.1469-7998.1941.tb08476.x
- Google Scholar
1. Lowndes AG
(1944) The swimming of Monas stigmatica pringsheim and peranema Trichophorum (Ehrbg.) Stein. and Volvox sp. Additional experiments on the working of a flagellum
Proceedings of the Zoological Society of London 114:325–338.
https://doi.org/10.1111/j.1096-3642.1944.tb00228.x
- Google Scholar
(2006) The reproductive significance of human fallopian tube cilia
Human Reproduction Update 12:363–372.
https://doi.org/10.1093/humupd/dml012
- PubMed
- Google Scholar
Book
1. Machemer H
(1974)
Ciliary activity and metachronism in protozoa

In: Sleigh M. A, editors. Cilia and Flagella. London: Academic Press. pp. 199–287.
- Google Scholar
(1995) Temperature effects on motion parameters and the phototactic reaction of the marine ciliate Fabrea salina
Journal of Photochemistry and Photobiology B: Biology 30:123–127.
https://doi.org/10.1016/1011-1344(95)07160-4
- Google Scholar
1. Metzner P
(1929)
Bewegungsstudien an Peridineen

Z.Bot 22:225–265.
- Google Scholar
(2013) Impact of swimming behaviour and nutrient limitation on predator–prey interactions in pelagic microbial food webs
Journal of Experimental Marine Biology and Ecology 446:29–35.
https://doi.org/10.1016/j.jembe.2013.04.015
- Google Scholar
1. Miyasaka I
2. Nanba K
3. Furuya K
4. Nimura Y
(1998) High-speed video observation of swimming behavior and flagellar motility of Prorocentrum minimum (Dinophyceae)
Protoplasma 204:38–46.
https://doi.org/10.1007/BF01282292
- Google Scholar
(2014) Eukaryotic flagella: variations in form, function, and composition during evolution
BioScience 64:1103–1114.
https://doi.org/10.1093/biosci/biu175
- Google Scholar
(2005) 3D structure of eukaryotic flagella in a quiescent state revealed by cryo-electron tomography
PNAS 102:15889–15894.
https://doi.org/10.1073/pnas.0508274102
- PubMed
- Google Scholar
1. Peters N
(1929)
Über Orts- und Geisselbewegung bei marinen Dinoflagellaten

Archiv für Protistenkunde 67:291–321.
- Google Scholar
1. Petroff AP
2. Pasulka AL
3. Soplop N
4. Wu XL
5. Libchaber A
(2015) Biophysical basis for convergent evolution of two veil-forming microbes
Royal Society Open Science 2:150437.
https://doi.org/10.1098/rsos.150437
- PubMed
- Google Scholar
1. Purcell EM
(1977) Life at low Reynolds number
American Journal of Physics 45:3–11.
https://doi.org/10.1119/1.10903
- Google Scholar
(1997) The effects of 2 μm Hg++ on the ethogram of Euplotes vannus (Ciliata, Hypotrichida)
European Journal of Protistology 33:63–71.
https://doi.org/10.1016/S0932-4739(97)80021-1
- Google Scholar
1. Riisgård HU
2. Larsen PS
(2009) Ciliary-propelling mechanism, effect of temperature and viscosity on swimming speed, and adaptive significance of ‘jumping’ in the ciliate Mesodinium rubrum
Marine Biology Research 5:585–595.
https://doi.org/10.1080/17451000902729704
- Google Scholar
Book
1. Roberts AM
(1981)
Hydrodynamics of protozoan swimming

In: Levandowski M, Hunter S, editors. Biochemistry and Physiology of Protozoa. Academic Press. pp. 5–66.
- Google Scholar
1. Rodríguez JA
2. Lopez MA
3. Thayer MC
4. Zhao Y
5. Oberholzer M
6. Chang DD
7. Kisalu NK
8. Penichet ML
9. Helguera G
10. Bruinsma R
11. Hill KL
12. Miao J
(2009) Propulsion of african trypanosomes is driven by bihelical waves with alternating chirality separated by kinks
PNAS 106:19322–19327.
https://doi.org/10.1073/pnas.0907001106
- PubMed
- Google Scholar
(2006) Flows driven by flagella of multicellular organisms enhance long-range molecular transport
PNAS 103:8315–8319.
https://doi.org/10.1073/pnas.0600566103
- PubMed
- Google Scholar
(1974) Density dependent vertical migration in the freshwater dinoflagellate Peridinium penardii (lemm.) lemm. fo. Californicum javorn
Journal of Phycology 10:475–476.
https://doi.org/10.1111/j.1529-8817.1974.tb02743.x
- Google Scholar
1. Sleigh MA
(1968)
Patterns of ciliary beating

Symposia of the Society for Experimental Biology 22:131–150.
- PubMed
- Google Scholar
Book
1. Sleigh MA
(1975)
Cilia and Flagella

London: Academic Press.
- Google Scholar
1. Sleigh MA
2. Aiello E
(1972)
The movement of water by cilia

Archiv für Protistenkunde 50:219–274.
- Google Scholar
Book
1. Sleigh MA
2. Blake JR
(1977)
Methods of ciliary propulsion and their size limitations

In: Pedley T. J, editors. Scale Effects in Animal Locomotion. Academic Press. pp. 243–256.
- Google Scholar
1. Sournia A
(1982) Form and function in marine phytoplankton
Biological Reviews 57:347–394.
https://doi.org/10.1111/j.1469-185X.1982.tb00702.x
- Google Scholar
1. Szyszkowicz S
2. Yanikome H
(2009) Limit theorem on the sum of identically distributed equally and positively correlated joint lognormals
IEEE Transactions on Communications 57:3538–3542.
https://doi.org/10.1109/TCOMM.2009.12.070539
- Google Scholar
1. Tam D
2. Hosoi AE
(2011) Optimal kinematics and morphologies for spermatozoa
Physical Review E 83:.
https://doi.org/10.1103/PhysRevE.83.045303
- Google Scholar
1. Throndsen J
(1973)
Motility in some marine nanoplankton flagellates

Norwegian Journal of Zoology 21:193–200.
- Google Scholar
(1997) Production of anisogametes and gamete motility dimorphism in Monostroma angicava
Sexual Plant Reproduction 10:261–268.
https://doi.org/10.1007/s004970050096
- Google Scholar
1. Visser AW
2. Kiørboe T
(2006) Plankton motility patterns and encounter rates
Oecologia 148:538–546.
https://doi.org/10.1007/s00442-006-0385-4
- Google Scholar
(1971) Nature of the flagellar beat in Trachelomonas volvocina, Rhabdomonas spiralis, Menoidium cultellus, and Chilomonas paramecium
Transactions of the American Microscopical Society 90:404–412.
https://doi.org/10.2307/3225455
- PubMed
- Google Scholar
1. Wang W
2. Shor LM
3. LeBoeuf EJ
4. Wikswo JP
5. Taghon GL
6. Kosson DS
(2008) Protozoan migration in bent microfluidic channels
Applied and Environmental Microbiology 74:1945–1949.
https://doi.org/10.1128/AEM.01044-07
- PubMed
- Google Scholar
1. Wheeler B
(1966) Phototactic vertical migration in Exuviaella baltica
Botanica Marina 9:15–17.
https://doi.org/10.1515/botm.1966.9.1-2.15
- Google Scholar
Book
1. Wichterman R
(1986)
The Biology of Paramecium

Springer.
- Google Scholar
(2007) Targeted gene disruption of dynein heavy chain 7 of Tetrahymena thermophila results in altered ciliary waveform and reduced swim speed
Journal of Cell Science 120:3075–3085.
https://doi.org/10.1242/jcs.007369
- PubMed
- Google Scholar

Decision letter

Arup K Chakraborty

Senior and Reviewing Editor; Massachusetts Institute of Technology, United States
Matthew Herron

Reviewer; Georgia Tech, United States

In the interests of transparency, eLife includes the editorial decision letter and accompanying author responses. A lightly edited version of the letter sent to the authors after peer review is shown, indicating the most substantive concerns; minor comments are not usually included.

Thank you for submitting your article "Swimming eukaryotic microorganisms exhibit a universal speed distribution" for consideration by eLife. Your article has been reviewed by two peer reviewers, and the evaluation has been overseen by Arup Chakraborty as the Senior and Reviewing Editor. The following individual involved in review of your submission has agreed to reveal his identity: Matthew Herron (Reviewer #1).

The reviewers have discussed the reviews with one another and the Reviewing Editor has drafted this decision to help you prepare a revised submission.

Summary:

This study analyzes published data on swimming speed among flagellated and ciliated microorganisms. Data from both groups fit similar, but separate, log normal distributions, leading the authors to infer a "universal way for ecological niches to be populated by abundant microorganisms." This short report is well-written, well-sourced, and has sufficient detail provided by the authors to replicate the findings. This methodology has the potential to enrich parallel genetics-based studies and provide deeper insights into the connections between ecology and evolution. However, we feel that the main claims of the paper need to be toned down or further substantiated in a revised submission.

Essential revisions:

1) The ecological implication of the results, expressed in the Abstract and at the end of the Discussion, concern how ecological niches are partitioned by microorganisms. The limitation of the analyzed data is that it is per species, and all species are treated equally, with no information about abundance. It is undoubtedly an important result that aquatic ecosystems contain many species that swim slowly and far fewer that swim quickly, at least within each category. However, without information about abundances, this result says very little about the distribution of swimming speeds within a given ecosystem; that is, the data are equally compatible with slow swimmers being rare (but diverse) and fast swimmers being common (but homogenous) or the opposite. So, the distribution of swimming speeds among all individuals is unclear. Similarly, flagellates could be common and ciliates rare or vice versa. The point that the distributions of swimming speeds among species do not necessarily reflect the distributions of swimming speeds among individuals needs to be addressed.

2) Can universality really be claimed with a set of just two distributions? Figures 2, 3, and 4 well-illustrate the authors' point, but comprise fitting and rescaling of just these two sets of data. The demonstration in Figure 4 that two log-normal distributions may be collapsed onto one another is not too surprising. It seems with such a data set on hand, there is much potential to explore a variety of questions that can enrich or explain the authors conclusions. In particular, addressing a few of the following points will enhance the paper:

• Did the authors examine any other distributions, such as cell size?

• Did the authors attempt a physical rescaling of the distributions, e.g. in terms of the Reynolds or Peclet numbers?

• Is there sufficient resolution in the data set (i.e. number of samples) to explore/compare subsets of the data such as uniflagellates versus multiflagellates and ciliates?

• Similar to the above comment, examining the swimming speed distributions of other taxonomic groups from the data set – i.e. corresponding to an early bifurcation in Figure 1 – may provide additional insights and strengthen the authors' argument for universality in the swimming speed distribution.

• While perhaps outside of the scope of the present short report, the potential implications of the authors' observation abound for other organismal systems. Did they consider examining other dataset, e.g. for higher organisms as in Gazzola et al., Nature Physics 10 (2014)?

https://doi.org/10.7554/eLife.44907.016

Author response

Essential revisions:

1] The ecological implication of the results, expressed in the Abstract and at the end of the Discussion, concern how ecological niches are partitioned by microorganisms. The limitation of the analyzed data is that it is per species, and all species are treated equally, with no information about abundance. It is undoubtedly an important result that aquatic ecosystems contain many species that swim slowly and far fewer that swim quickly, at least within each category. However, without information about abundances, this result says very little about the distribution of swimming speeds within a given ecosystem; that is, the data are equally compatible with slow swimmers being rare (but diverse) and fast swimmers being common (but homogenous) or the opposite. So, the distribution of swimming speeds among all individuals is unclear. Similarly, flagellates could be common and ciliates rare or vice versa. The point that the distributions of swimming speeds among species do not necessarily reflect the distributions of swimming speeds among individuals needs to be addressed.

This is a very interesting point and we agree with the reviewers. Alas, since there is little or no available data for abundance, it is difficult to make claim concerning particular ecosystems. We are implicitly assuming in our paper that the sampling of the underlying distributions was random. Of course, in real ecosystems there are interactions (symbiotic, mutualistic, etc.) among species so they are not necessarily “independent”. To state our point clearly, we have added a sentence in the last paragraph of the main text.

2] Can universality really be claimed with a set of just two distributions? Figures 2, 3, and 4 well-illustrate the authors' point, but comprise fitting and rescaling of just these two sets of data. The demonstration in Figure 4 that two log-normal distributions may be collapsed onto one another is not too surprising. It seems with such a data set on hand, there is much potential to explore a variety of questions that can enrich or explain the authors conclusions. In particular, addressing a few of the following points will enhance the paper:

• Did the authors examine any other distributions, such as cell size?

Yes we have. We have now added to the paper the available data on cell sizes that we believe helps present a broader picture. Figure 2—figure supplement 2 contains histogram of cell sizes, produced using values given in the revised tables in Appendix 1. The cell sizes represent the 'characteristic' size for each cell (largest of the available sizes if different width/length were given in literature). The size distributions are distinct and no apparent similarity between them is visible. We have then used them to calculate the Reynolds and Péclet numbers, as suggested below.

• Did the authors attempt a physical rescaling of the distributions, e.g. in terms of the Reynolds or Peclet numbers?

Rescaled distributions have been added as Figure 2—figure supplement 3. We have plotted there the Reynolds number for each organism. Due to the paucity of reported viscosities in the analysed works, we assumed the viscosity to be that of water at standard conditions in each case. The distributions are different, since ciliates are generally larger and faster swimmers compared to flagellates. The Péclet number is proportional to the Reynolds number, since both contain the product of cell size and swimming velocity. Therefore, we choose one (Re) as a measure of the character of the fluid transport. We have modified the paper to indicate these points.

• Is there sufficient resolution in the data set (i.e. number of samples) to explore/compare subsets of the data such as uniflagellates versus multiflagellates and ciliates?

Unfortunately, the resolution of the subsets is not sufficient for the proposed comparison. The listed data was the information available in literature on the swimming problem. Our focus was to collect it and analyse it together. Moreover, some of the values given represent averages over samples analyzed in the papers, given with no further information on the uncertainty. In the plots, we have included the fitting errors and estimated the errors associated to the binning procedures. Within the available data, this statistical uncertainty was the only accessible measure.

• Similar to the above comment, examining the swimming speed distributions of other taxonomic groups from the data set – i.e. corresponding to an early bifurcation in Figure 1 – may provide additional insights and strengthen the authors' argument for universality in the swimming speed distribution.

Pointing back to the previous remark, we think the statistics for individual groups would not be sufficient to justify potential conclusions and thus we refrained from this analysis in the paper.

• While perhaps outside of the scope of the present short report, the potential implications of the authors' observation abound for other organismal systems. Did they consider examining other dataset, e.g. for higher organisms as in Gazzola et al., Nature Physics 10 (2014)?

The paper by Gazzola et al. concerns the relation that can be established between the Reynolds number for the flow created by a swimming organism and its swimming number, which involves the temporal details of the actuation (frequency of the periodic body motion which gives rise to the flow). In our study, we focus on unicellular microscale swimmers, and thus the Reynolds numbers rarely exceed 1 (see Figure 2—figure supplement 3), so the realm of higher Re is outside of the scope of the report.

https://doi.org/10.7554/eLife.44907.017

Article and author information

Author details

Maciej Lisicki
1. Department of Applied Mathematics and Theoretical Physics, University of Cambridge, Cambridge, United Kingdom
2. Institute of Theoretical Physics, Faculty of Physics, University of Warsaw, Warsaw, Poland
Contribution
Conceptualization, Data curation, Software, Formal analysis, Validation, Investigation, Methodology, Writing—original draft, Writing—review and editing

Contributed equally with
Marcos F Velho Rodrigues

For correspondence
maciej.lisicki@fuw.edu.pl

Competing interests
No competing interests declared

"This ORCID iD identifies the author of this article:" 0000-0002-6976-0281
Marcos F Velho Rodrigues

Department of Applied Mathematics and Theoretical Physics, University of Cambridge, Cambridge, United Kingdom

Contribution
Data curation, Software, Formal analysis, Investigation, Visualization, Methodology, Writing—original draft, Writing—review and editing

Contributed equally with
Maciej Lisicki

Competing interests
No competing interests declared

"This ORCID iD identifies the author of this article:" 0000-0002-8744-6966
Raymond E Goldstein

Department of Applied Mathematics and Theoretical Physics, University of Cambridge, Cambridge, United Kingdom

Contribution
Investigation, Methodology, Writing—review and editing

Competing interests
Reviewing editor, eLife

"This ORCID iD identifies the author of this article:" 0000-0003-2645-0598
Eric Lauga

Department of Applied Mathematics and Theoretical Physics, University of Cambridge, Cambridge, United Kingdom

Contribution
Conceptualization, Formal analysis, Supervision, Funding acquisition, Validation, Investigation, Methodology, Writing—original draft, Project administration, Writing—review and editing

For correspondence
e.lauga@damtp.cam.ac.uk

Competing interests
No competing interests declared

"This ORCID iD identifies the author of this article:" 0000-0002-8916-2545

Funding

H2020 European Research Council (682754)

Eric Lauga

Engineering and Physical Sciences Research Council (EP/M017982/)

Raymond E Goldstein

Gordon and Betty Moore Foundation (7523)

Raymond E Goldstein

The funders had no role in study design, data collection and interpretation, or the decision to submit the work for publication.

Acknowledgements

This project has received funding from the European Research Council (ERC) under the European Union's Horizon 2020 research and innovation program (grant agreement 682754 to EL), and from Established Career Fellowship EP/M017982/1 from the Engineering and Physical Sciences Research Council and Grant 7523 from the Gordon and Betty Moore Foundation (REG).

Senior and Reviewing Editor

Arup K Chakraborty, Massachusetts Institute of Technology, United States

Reviewer

Matthew Herron, Georgia Tech, United States

Publication history

Received: January 5, 2019
Accepted: June 29, 2019
Version of Record published: July 16, 2019 (version 1)

Copyright

This article is distributed under the terms of the Creative Commons Attribution License, which permits unrestricted use and redistribution provided that the original author and source are credited.

Metrics

1,528

Page views
191

Downloads
21

Citations

Article citation count generated by polling the highest count across the following sources: Crossref, PubMed Central, Scopus.

Download links

A two-part list of links to download the article, or parts of the article, in various formats.

Downloads (link to download the article as PDF)

Open citations (links to open the citations from this article in various online reference manager services)

Mendeley

Cite this article (links to download the citations from this article in formats compatible with various reference manager tools)

Maciej Lisicki
Marcos F Velho Rodrigues
Raymond E Goldstein
Eric Lauga

(2019)

Swimming eukaryotic microorganisms exhibit a universal speed distribution

eLife 8:e44907.

https://doi.org/10.7554/eLife.44907

Share this article

Cite this article

The tree of life (cladogram) for unicellular eukaryotes encompassing the phyla of organisms analyzed in the present study.

Histograms of swimming speed for ciliates and flagellates demonstrate a similar character but different scales of velocities.

Probability distribution functions of swimming speeds for flagellates (a) and ciliates (b) with the fitted log-normal distributions.

Test of rescaling hypothesis.

Author details

Maciej Lisicki

Contribution

Contributed equally with

For correspondence

Competing interests

Marcos F Velho Rodrigues

Contribution

Contributed equally with

Competing interests

Raymond E Goldstein

Contribution

Competing interests

Eric Lauga

Contribution

For correspondence

Competing interests

Downloads (link to download the article as PDF)

Open citations (links to open the citations from this article in various online reference manager services)

Cite this article (links to download the citations from this article in formats compatible with various reference manager tools)

Categories and tags

Further reading