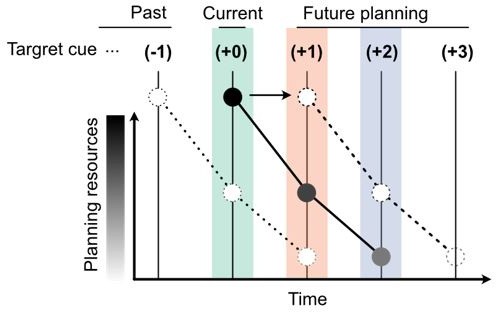
Future movement plans interact in sequential arm movements
- Western Institute for Neuroscience, Western University, Canada
- School of Exercise and Nutritional Sciences, San Diego State University, United States
- Department of Physiology and Pharmacology, Western University, Canada
- Department of Computer Science, Western University, Canada
- Department of Statistical and Actuarial Sciences, Western University, Canada
Figures
Figure 1 with 1 supplement
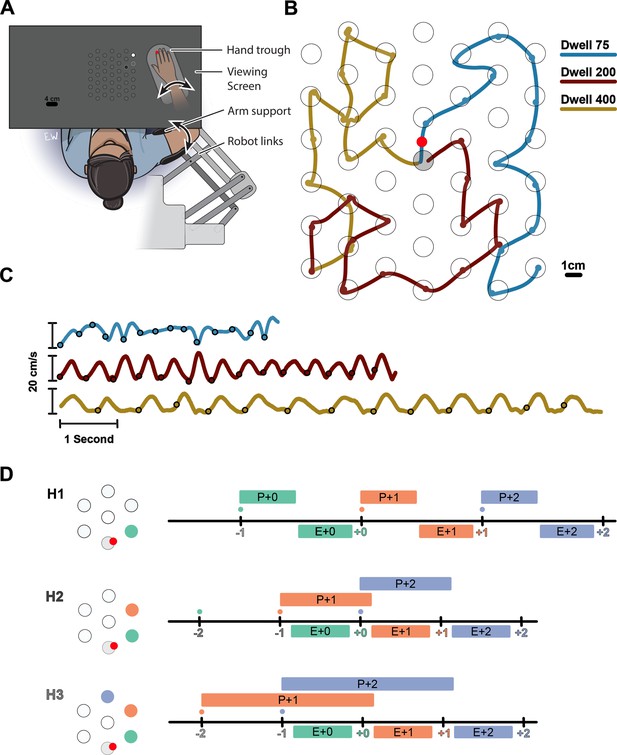
Experimental paradigm.
(A) Participants performed reaches in an exoskeleton robot. Their hand was occluded, and hand position was indicated by a red dot. The full grid of possible targets not shown to participants. The targets and their order were shown with decreasing brightness (an H3 trial is shown). (B) Movement trajectory in three example trials (Horizon 2; Dwell 75, 200, 400). Trials always started from a fixed home target in center (gray target). The small circles on the traces show the time point in which the target was captured. (C) Speed profiles for the example trials shown in (B). (D) Timeline of the task for Horizon 1–3 conditions. Ticks show the time when the target was captured (colored number) and a new target was shown on the screen (colored small dot). The boxes above the line show the available time for planning each movement, the time from when the target first shown to the beginning of the execution of the movement. The boxes below the line show the execution of each movement, the time interval in which the hand was moving from one target to another.
Figure 1—figure supplement 1
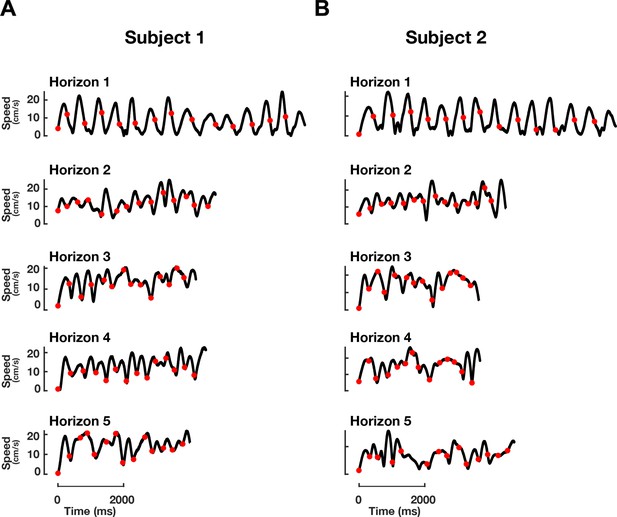
Speed profile for two participants.
(A) From one participant, for each horizon, one trial is selected randomly. Black traces show the speed profile, red dots show the time point that hand entered a new target. (B) Same as (A) but for a different participant.
Figure 2
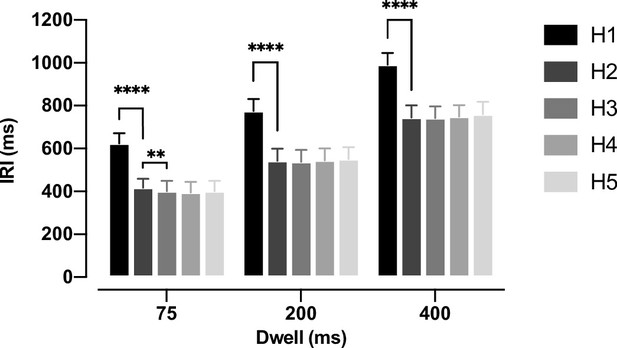
Inter-reach interval (IRI) for three dwell times and five horizons.
IRI was averaged across all trials, all session, for each participant. The error bars show a 95% confidence interval accross participants (n = 11), ** signifies p<0.01, **** signifies p<0.0001.
Figure 3
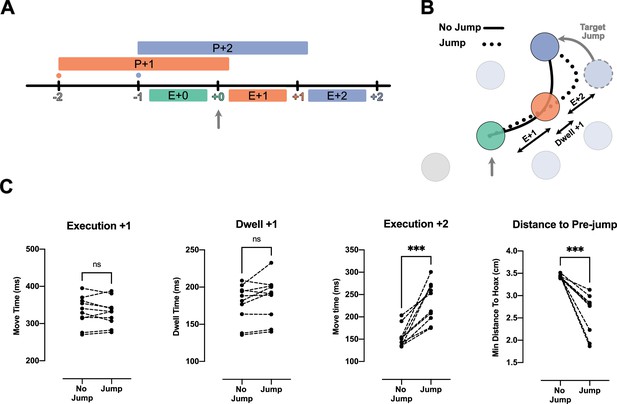
Jump of the +2 target reveals existence of planning processes for the reach toward +2 target during the execution of +0 reach.
(A) Timeline of the jump experiment in Horizon 3, Dwell time 75 ms condition. The jump occurred at the capture of +0 target (vertical gray arrow). (B) Reach trajectory for an example no-jump trial (solid line) in which the pre-jump target (light purple) was not shown, and a +2 jump sample trial (dotted line) in which the pre-jump target moved to a new position (dark purple) at the time the +0 target was captured (vertical gray arrow). (C) The time for Execution +1 (E+1), Dwell +1, and Execution +2 (E+2), and the minimum distance of reach trajectory to the center of pre-jump target for no-jump and jump conditions. Each dot represents one participant (n = 10), *** shows p-value<0.001.
Figure 4
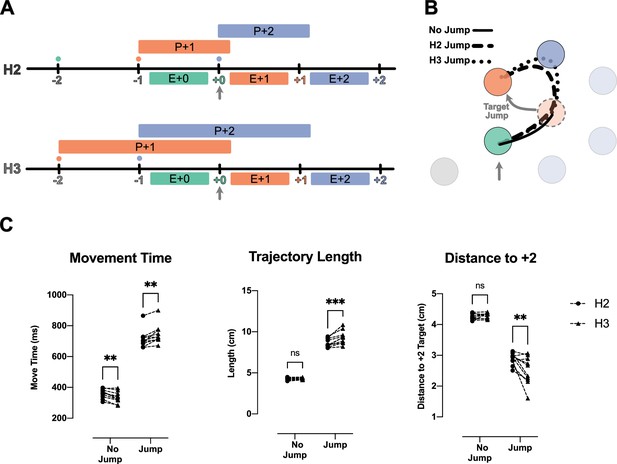
Correction for jump of the +1 target is delayed when more future movements are planned.
(A) Timeline of the jump experiment in the H2 and H3 conditions. In both conditions, the jump of the +1 target (orange) occurred when the +0 target was captured (vertical gray arrow). (B) Example trials for a no-jump condition (solid line) and for jump conditions for H2 (dashed line) and H3 (dotted line). In the latter two conditions, the +1 target (orange dotted circle) jumped to a new position (curved gray arrow), when the +0 target was captured (vertical gray arrow). (C) Movement time, trajectory length, and minimum distance of the trajectory to the center of +2 target for the reach to the new position of the +1 target. Dots and triangles show mean values for each subject (n = 10) in H2 and H3 conditions respectively. ** and *** signify p-value<0.01 and p-value<0.001, respectively.
Figure 5
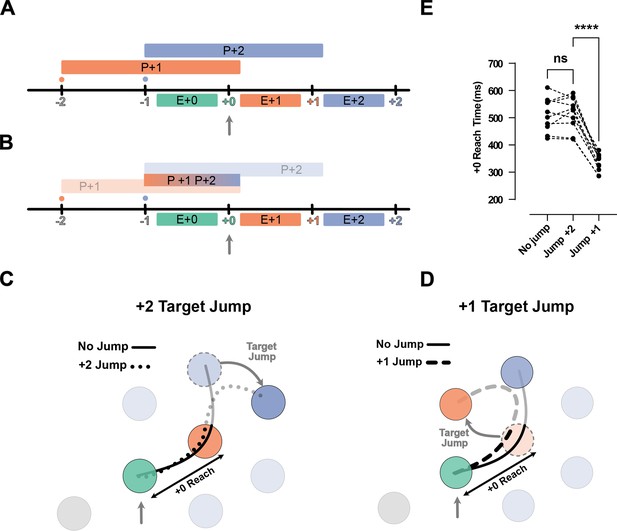
Jump of the +2 target or +1 rejects chunked planning of the reaches to the +1 and +2 targets.
(A) Timeline of the task in the jump experiments. Ticks show the time when the target was captured (colored number) and a new target was shown on the screen (colored small dot). The boxes above the line show the available time for planning each movement, and the boxes below the line show the execution of each planned movement. (B) Same as (A), but for the chunked planning hypothesis, here one chunked planning controls both Execution +1 (E+1) and Execution +2 (E+2) reaches. (C) Reach trajectories for +2 target jump experiment. Reach trajectory for one example no-jump trial (solid line) in which the pre-jump target (light purple) was not shown, and a +2 jump sample trial (dotted line) in which the pre-jump target moved to a new position (dark purple) at the time the +0 target was captured (vertical gray arrow). (D) Example trials for a no-jump condition (solid line) and for jump conditions for H2 (dashed line) in which the +1 target (orange dotted circle) jumped to a new position (curved gray arrow) when the +0 target was captured (vertical gray arrow). (E) +0 Reach time measured from when the cursor entered the +0 target (green circle) to when it exits the +1 target (orange circle) for each participant (n = 10). *** signify p-value<0.01 and p-value<0.001.
Figure 6
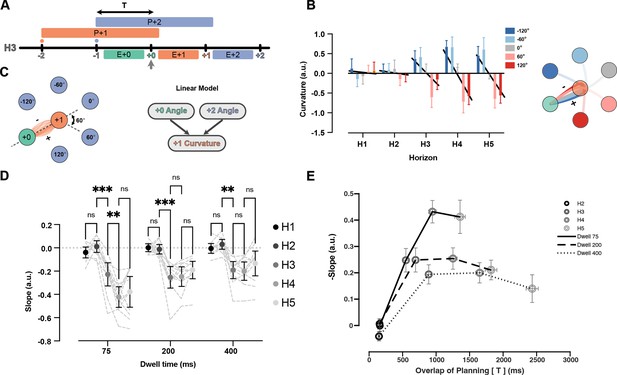
Curvature of the reach to +1 target is modulated by position of +2 target.
(A) Timeline of planning and execution for a Horizon 3 trial. Amount of time overlap (T) between planning processes for reach to +1 and +2 target is represented by the black arrow. (B) Effect of +2 target angle on the curvature of +1 reach for Dwell time 75, all horizons, one participant. Positive value of curvature indicates downward curve and vice versa. The overall effect is captured by the slope of the line relating average curvature to the five angles (slope of black lines). (C) All the reaches are aligned to one start point and one direction. Then, the angle at the start of the movement to the +2 target can be −120, –60, 0, 60, 120 degrees (60 is shown with dotted line). A linear model is used to predict the signed curvature based on the position of last target (+0 angle) and the +2 target (+2 angle). (D) Each dot represents the average summary statistics of the curvature across participants. Individual participant values are shown with shadowed dotted lines in the background. (E) Average curvature effect across participants vs overlaps of planning time (T) for each condition. Shades of gray show different horizons and solid, dashed, and dotted lines represent different dwell time conditions. Error bars are SEM across participants (n = 11), and ** signifies p-value<0.001.
Figure 7
Saccade position is shifted toward the future target.
(A) One sample trial from a representative participant. The blue and black trace shows the hand and eye position, respectively. The blue dots are when hand first entered the target, yellow dots show when hand exited the target, the red × shows the fixation location. (B) Timing of capturing the current target (gray), saccade to the next target (red), hand out of the captured target (yellow), and hand in the next target (blue), averaged across participants, black lines show 95% confidence interval. (C) Saccade angles of a representative participants for each possible +2 target and three horizons. (D) Average saccade angle for all participants (n = 19) and each horizon. Error bars show 95% confidence interval.
Author response image 1
Basic diagram showing a soft distribution of a limited planning resource.
Additional files
Download links
A two-part list of links to download the article, or parts of the article, in various formats.
Downloads (link to download the article as PDF)
Open citations (links to open the citations from this article in various online reference manager services)
Cite this article (links to download the citations from this article in formats compatible with various reference manager tools)
Future movement plans interact in sequential arm movements
eLife 13:RP94485.
https://doi.org/10.7554/eLife.94485.3
