Dendritic trafficking faces physiologically critical speed-precision tradeoffs
- University of California, San Diego, United States
- Salk Institute for Biological Studies, United States
- Stanford University, United States
- University of Bristol, United Kingdom
- Brandeis University, United States
- University of Cambridge, United Kingdom
Figures
Figure 1 with 1 supplement
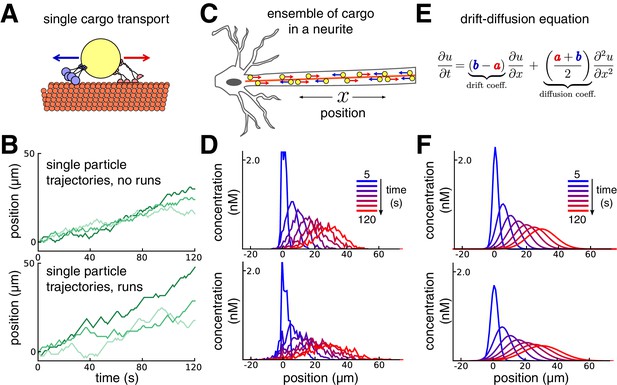
Constructing a coarse-grained model of intracellular transport.
(A) Cartoon of a single cargo particle on a microtubule attached to opposing motor proteins. (B) Three example biased random walks, representing the stochastic movements of individual cargoes. (Top …
Figure 1—figure supplement 1
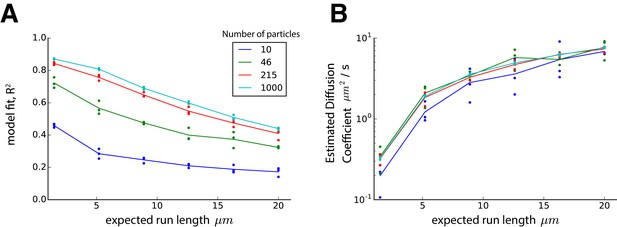
The effect of cargo run length on mass-action model fit and diffusion coefficient.
The model of stochastic particle movement (Equation 7, Materials and methods) was simulated with equal transition probabilities () for various values of and particle numbers in an infinite …
Figure 2 with 1 supplement
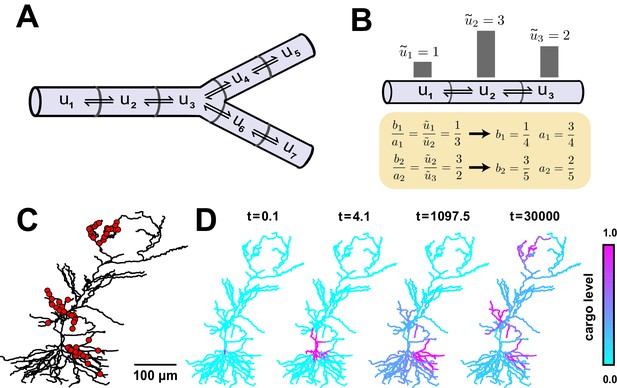
Local trafficking rates determine the spatial distribution of biomolecules by a simple kinetic relationship.
(A) The mass action transport model for a simple branched morphology. (B) Demonstration of how trafficking rates can be tuned to distribute cargo to match a demand signal. Each pair of rate …
Figure 2—figure supplement 1
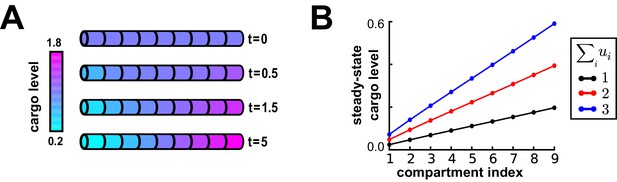
Equation 4 specifies the relative distribution of cargo, changing the total amount of cargo scales this distribution.
(A) Inspired by ion channel expression gradients observed in hippocampal cells (Hoffman et al., 1997; Magee, 1998), we produced a linear gradient in cargo distribution in an unbranched cable. By Equa…
Figure 3
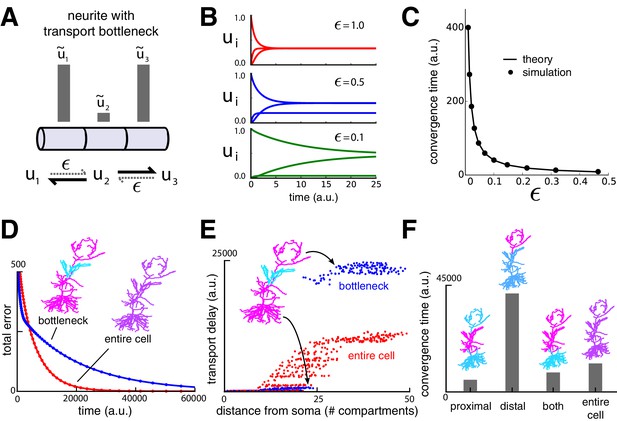
Transport bottlenecks caused by cargo demand profiles.
(A) A three-compartment transport model, with the middle compartment generating a bottleneck. The vertical bars represent the desired steady-state concentration of cargo in each compartment. The …
Figure 4
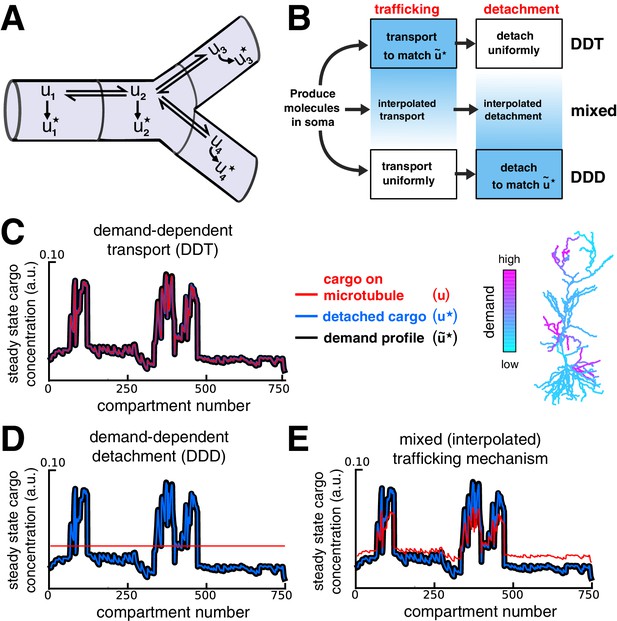
Multiple strategies for transport with trafficking and cargo detachment controlled by local signals.
(A) Schematic of microtubular transport model with irreversible detachment in a branched morphology. (B) Multiple strategies for trafficking cargo to match local demand (demand = ). (Top) The …
Figure 5
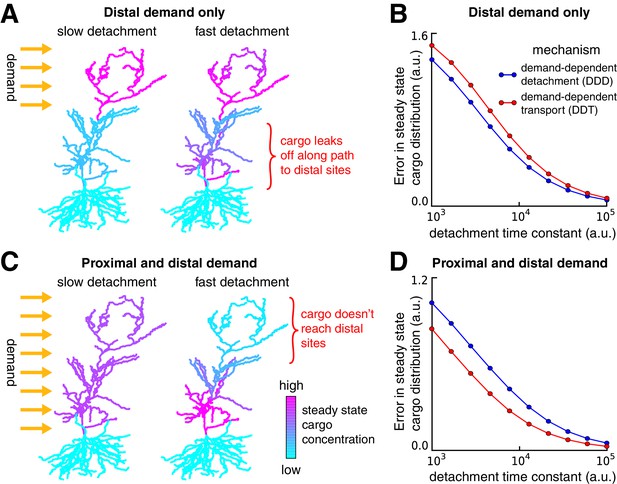
Tradeoffs in the performance of trafficking strategies depends on the spatial pattern of demand.
(A) Delivery of cargo to the distal dendrites with slow (left) and fast detachment rates (right) in a reconstructed CA1 neuron. The achieved pattern does not match the target distribution when …
Figure 6 with 1 supplement
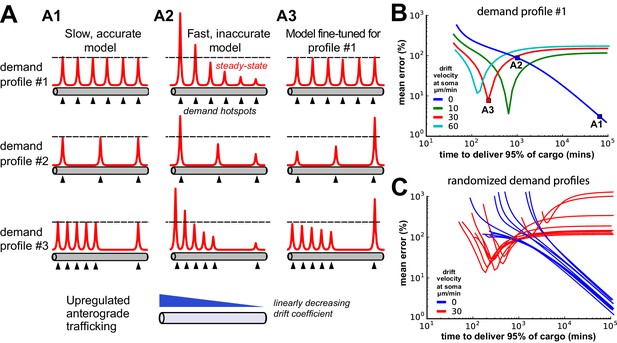
Tuning the DDD model for speed and specificity results in sensitivity to the target spatial distribution of cargo.
(A) Cargo begins on the left end of an unbranched cable to be distributed equally amongst several demand ‘hotspots’. Steady-state cargo profiles (red) are shown for three different models (A1, A2, …
Figure 6—figure supplement 1
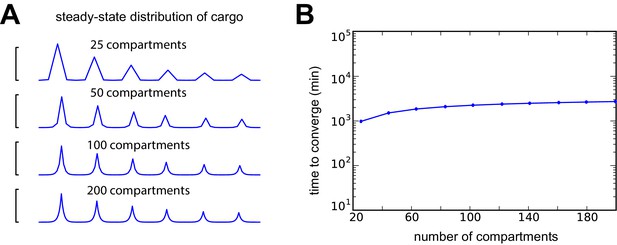
Changing compartment size over an order of magnitude leads to insignificant changes in model behavior when trafficking rates are appropriately scaled (i.e. and are inversely scaled to the squared compartment length; the diffusion coefficient converges to 10 μm2 s−1 as the compartment size shrinks to zero).
(A) A DDD model with six evenly spaced demand hotspots along a 800 µm cable and a fixed detachment rate constant of 5 × 10−4 s−1 converges to the same qualitative distribution of cargo at …
Figure 7
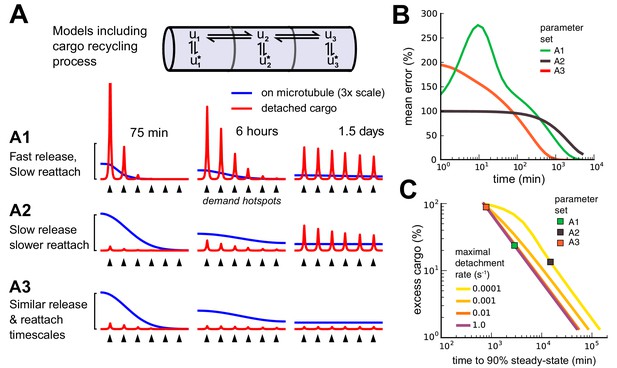
Adding a mechanism for cargo reattachment produces a further tradeoff between rate of delivery and excess cargo.
(A) Simulations of three models (A1, A2, A3) with cargo recycling. As in Figure 6, cargo is distributed to six demand hotspots (black arrows). The distributions of cargo on the microtubules (, …
Figure 8
Effect of morphology on trafficking tradeoffs.
(A) Representative morphologies from four neuron types, drawn to scale. The red dot denotes the position of the soma (not to scale). (B) Distribution of cargo on the microtubles () and delivered …
Videos
Video 1
Distribution of trafficked cargo over logarithmically spaced time points in a CA1 pyramidal cell model adapted from (Migliore and Migliore, 2012).
Cargo was trafficked according to Equation 4 to match a demand signal established by stimulated synaptic inputs (see Figure 2C). Time and cargo concentrations are reported in arbitrary units.
Video 2
A model with slow detachment rate accurately distributes cargo to six demand hotspots in an unbranched cable.
The spatial distribution of detached cargo (bottom subplot) and cargo on the microtubules (top subplot) are shown over logarithmically spaced timepoints. Compare to Figure 6A1 (top row).
Video 3
A model with a fast detachment rate misallocates cargo to six demand hotspots in an unbranched cable.
The spatial distribution of detached cargo (bottom subplot) and cargo on the microtubules (top subplot) are shown over logarithmically spaced timepoints. Proximal demand hotspots receive too much …
Video 4
Fine-tuning the trafficking rates in a model with fast detachment produces fast and accurate deliver of cargo to six demand hotspots in an unbranched cable.
The spatial distribution of detached cargo (bottom subplot) and cargo on the microtubules (top subplot) are shown over logarithmically spaced timepoints. Compare to Figure 6A3 (top row).
Video 5
The model fine-tuned for fast and accurate deliver of cargo to six demand hotspots misallocates cargo to three demand hotspots.
The spatial distribution of detached cargo (bottom subplot) and cargo on the microtubules (top subplot) are shown over logarithmically spaced timepoints. Compare to Figure 6A3 (middle row).
