Figures and data in Quantitative dissection of transcription in development yields evidence for transcription-factor-driven chromatin accessibility | eLife

Figures
Videos
Additional files

23 figures, 7 videos and 2 additional files

Figures

Figure 1

Download asset Open asset

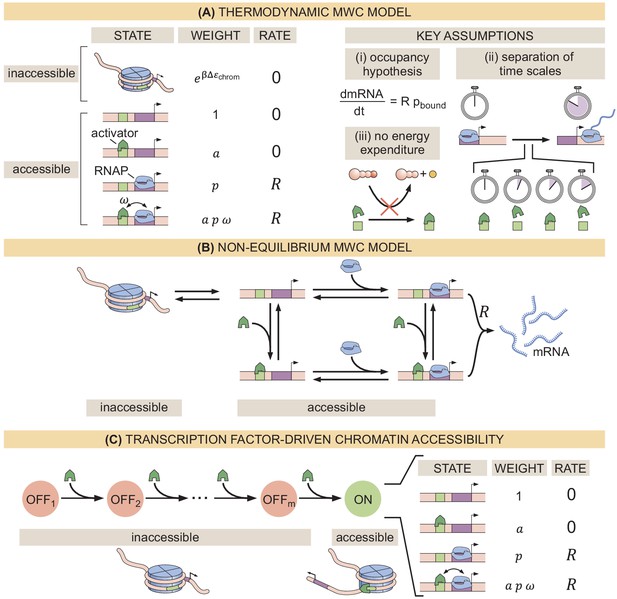
Three models of chromatin accessibility and transcriptional regulation.

(A) Thermodynamic MWC model where chromatin can be inaccessible or accessible to transcription factor binding. Each state is associated with a statistical weight given by the Boltzmann distribution and with a rate of transcriptional initiation. $Δ ε_{chrom}$ is the energy cost associated with making the DNA accessible and ω is an interaction energy between the activator and RNAP. $a = [activator] / K_{a}$ and $p = [RNAP] / K_{p}$ with $K_{a}$ and $K_{p}$ being the dissociation constants of the activator and RNAP, respectively. This model assumes the occupancy hypothesis, separation of time scales, and lack of energy expenditure described in the text. (B) Non-equilibrium MWC model where no assumptions about separation of time scales or energy expenditure are made. Transition rates that depend on the concentration of the activator or RNAP are indicated by an arrow incorporating the respective protein. (C) Transcription-factor-driven chromatin accessibility model where the activator catalyzes irreversible transitions of the DNA through m silent states before it becomes accessible. Once this accessible state is reached, the system is in equilibrium.

Figure 2

Download asset Open asset

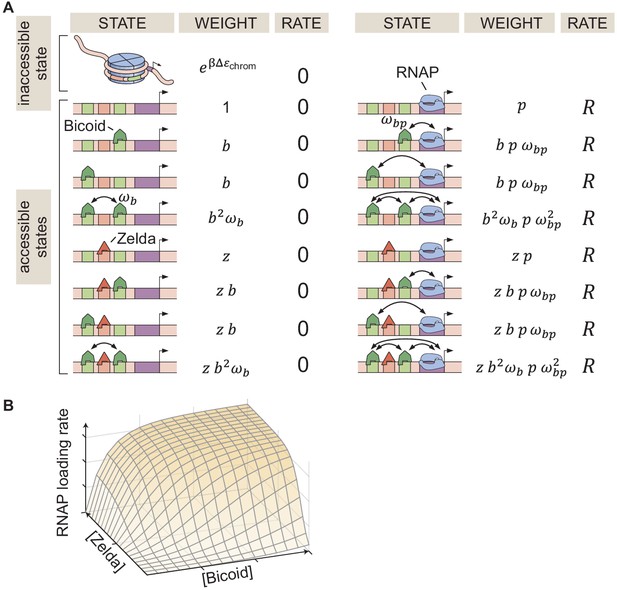
Thermodynamic MWC model of transcriptional regulation by Bicoid and Zelda.

(A) States and statistical weights for a simplified version of the hunchback P2 enhancer. In this model, we assume that chromatin occluded by nucleosomes is not accessible to transcription factors or RNAP. Parameters are defined in the text. (B) 3D input-output function predicting the rate of RNAP loading (and of transcriptional initiation) as a function of Bicoid and Zelda concentrations for a given set of model parameters.

Figure 3

Download asset Open asset

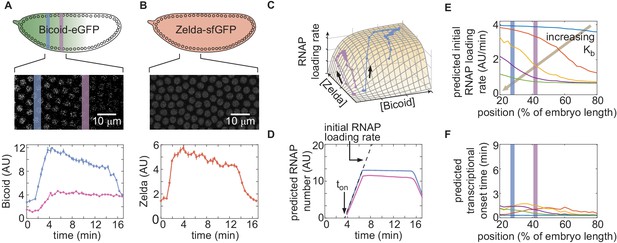
Prediction and measurement of dynamical input-output functions.

(A) Measurement of Bicoid concentration dynamics in nuclear cycle 13. Color denotes different positions along the embryo and time is defined with respect to anaphase. (B) Zelda concentration dynamics. These dynamics are uniform throughout the embryo. (C) Trajectories defined by the input concentration dynamics of Bicoid and Zelda along the predicted input-output surface. Each trajectory corresponds to the RNAP loading-rate dynamics experienced by nuclei at the positions indicated in (A). (D) Predicted number of RNAP molecules actively transcribing the gene as a function of time and position along the embryo, and calculation of the corresponding initial rate of RNAP loading and the time of transcriptional onset, $t_{o n}$ . (E, F) Predicted hunchback (E) initial rate of RNAP loading and (F) $t_{o n}$ as a function of position along the embryo for varying values of the Bicoid dissociation constant $K_{b}$ . (A, B, error bars are standard error of the mean nuclear fluorescence in an individual embryo, averaged across all nuclei at a given position; D, the standard error of the mean predicted RNAP number in a single embryo, propagated from the errors in A and B, is thinner than the curve itself; E, F, only mean predictions are shown so as to not obscure differences between them; we imaged n=6 Bicoid-GFP and n=3 Zelda-GFP embryos.)

Figure 4

Download asset Open asset

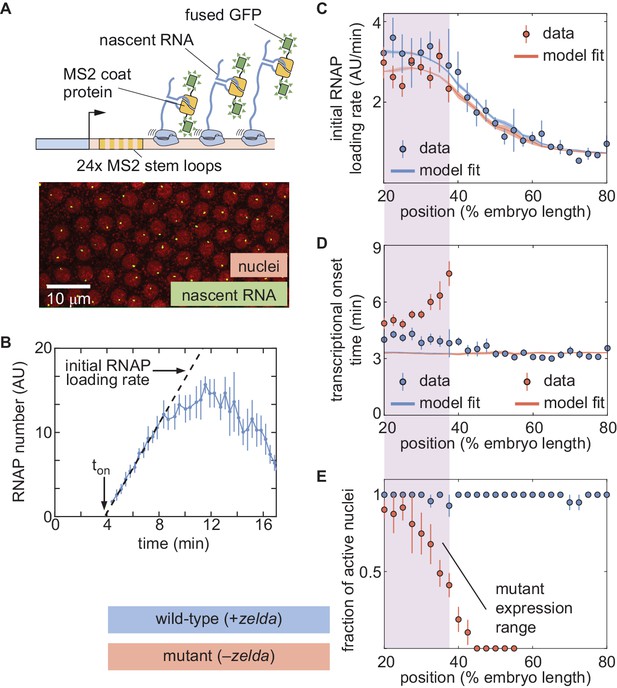
The thermodynamic MWC model can explain hunchback transcriptional dynamics in wild-type, but not zelda⁻, embryos.

(A) The MS2 system measures the number of RNAP molecules actively transcribing the hunchback reporter gene in live embryos. (B) Representative MS2 trace featuring the quantification of the initial rate of RNAP loading and $t_{o n}$ . (C) Initial RNAP loading rate and (D) $t_{o n}$ for wild-type (blue points) and zelda⁻ (red points) embryos, compared with best fit to the thermodynamic MWC model (lines). The red and blue fit lines are close enough to overlap substantially. (E) Fraction of transcriptionally active nuclei for wild-type (blue) and zelda⁻ (red) embryos. Active nuclei are defined as nuclei that exhibited an MS2 spot at any time during the nuclear cycle. Purple shading indicates the spatial range over which at least 30% of nuclei in the zelda⁻ background display transcription. (B, error bars are standard error of the mean observed RNAP number, averaged across nuclei in a single embryo; C, D solid lines indicate mean predictions of the model, shading represents standard error of the mean; C, D, E, error bars in data points represent standard error of the mean over 11 wild-type embryos (blue) or 12 zelda⁻ embryos (red)).

Figure 5

Download asset Open asset

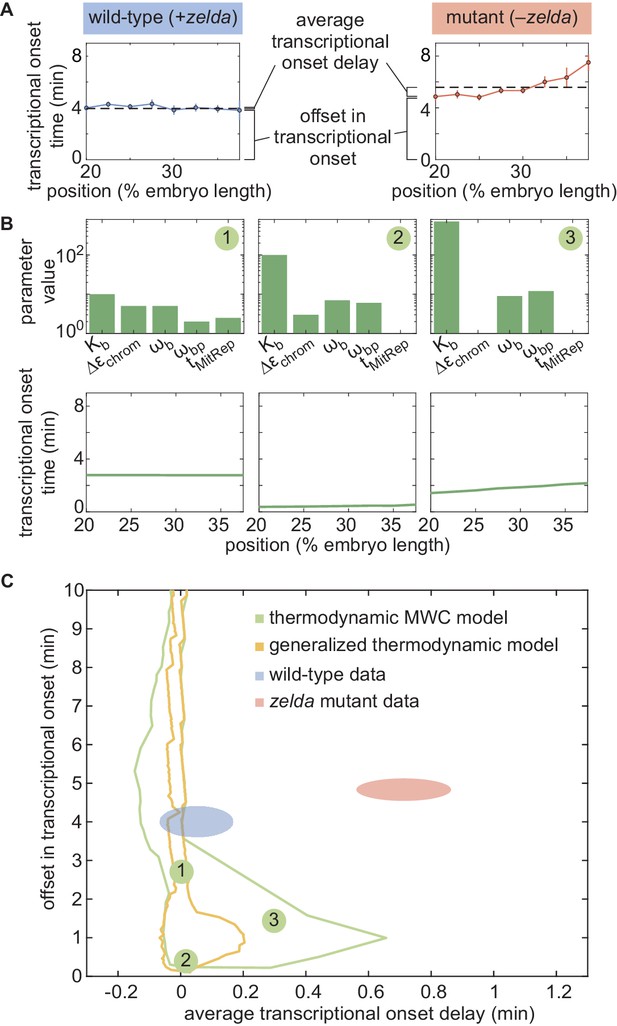
Failure of thermodynamic models to describe Bicoid-dependent activation of hunchback.

(A) Experimentally determined $t_{o n}$ with offset and average delay. Horizontal dashed lines indicate the average $t_{o n}$ delay with respect to the offset in $t_{o n}$ at 20% along the embryo for wild-type and zelda⁻ data sets. (B) Exploration of $t_{o n}$ offset and average $t_{o n}$ delay from the thermodynamic MWC model. Each choice of model parameters predicts a distinct $t_{o n}$ profile of along the embryo. (C) Predicted range of $t_{o n}$ offset and average $t_{o n}$ delay for the three cases featured in B (green points), for all possible parameter choices of the thermodynamic MWC model (green region), as well as for all thermodynamic models considering 12 Bicoid-binding sites (yellow region), compared with experimental data (red and blue regions). (A, C, error bars/ellipses represent standard error of the mean over 11 and 12 embryos for the wild-type and zelda⁻ datasets, respectively; B, solid lines indicate mean predictions of the model).

Figure 6

Download asset Open asset

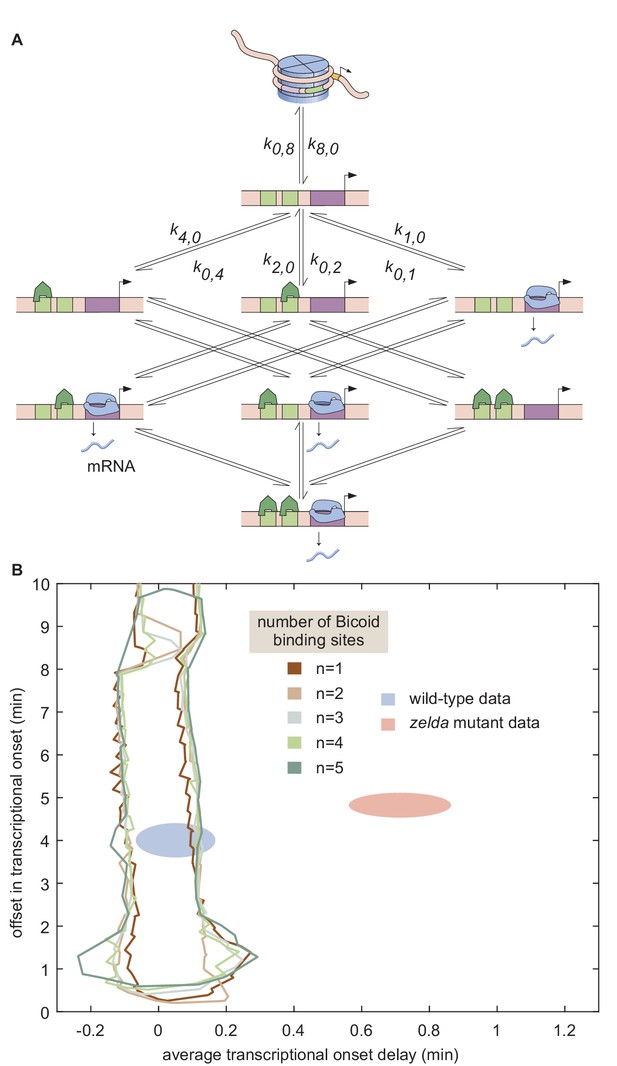
Non-equilibrium MWC model of transcriptional regulation cannot predict the observed $t_{o n}$ delay.

(A) Model that makes no assumptions about the relative transition rates between states or about energy expenditure. Each transition rate $i, j$ represents the rate of switching from state i to state j. See Appendix section 7.1 for details on how the individual states are labeled. (B) Exploration of $t_{o n}$ offset and average $t_{o n}$ delay attainable by the non-equilibrium MWC models as a function of the number of Bicoid-binding sites compared to the experimentally obtained values corresponding to the wild-type and zelda⁻ mutant backgrounds. While the non-equilibrium MWC model can explain the wild-type data, the exploration reveals that it fails to explain the zelda⁻ data, for up to five Bicoid-binding sites. (B, ellipses represent standard error of the mean over 11 and 12 embryos for the wild-type and zelda⁻ datasets, respectively).

Figure 7

Download asset Open asset

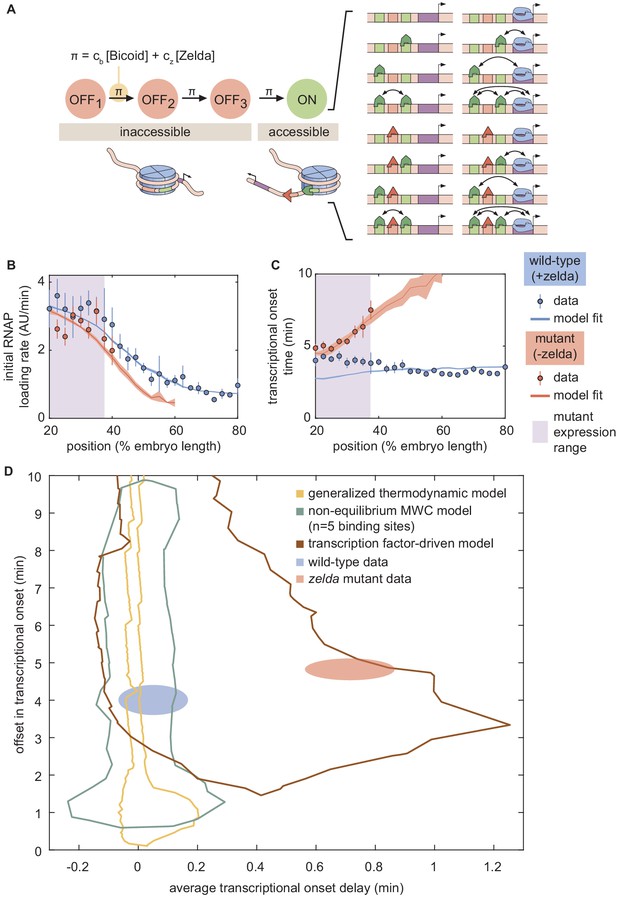
A model of transcription-factor-driven chromatin accessibility is sufficient to recapitulate hunchback transcriptional dynamics.

(A) Overview of the proposed model, with three ( $m = 3$ ) effectively irreversible Zelda and/or Bicoid-mediated intermediate transitions from the inaccessible to the accessible state. (B, C) Experimentally fitted (B) initial RNAP loading rates and (C) $t_{o n}$ for wild-type and zelda⁻ embryos using a single set of parameters and assuming six Bicoid binding sites. (D) The domain of $t_{o n}$ offset and average $t_{o n}$ delay covered by this transcription-factor-driven chromatin accessibility model (brown) is much larger than those of the generalized thermodynamic model (yellow) and the non-equilibrium MWC models (green), and easily encompasses both experimental datasets (ellipses). (B-D, error bars/ellipses represent standard error of the mean over 11 and 12 embryos for the wild-type and zelda⁻ datasets, respectively).

Appendix 1—figure 1

Download asset Open asset

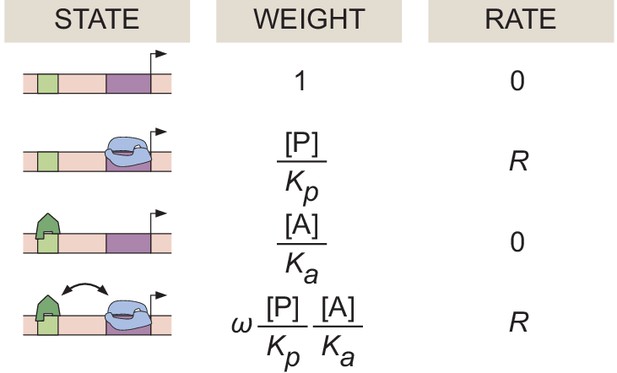
Equilibrium thermodynamic model of simple activation.

A promoter region with one binding site for an activator molecule has four possible microstates, each with its corresponding statistical weight and rate of RNAP loading.

Appendix 1—figure 2

Download asset Open asset

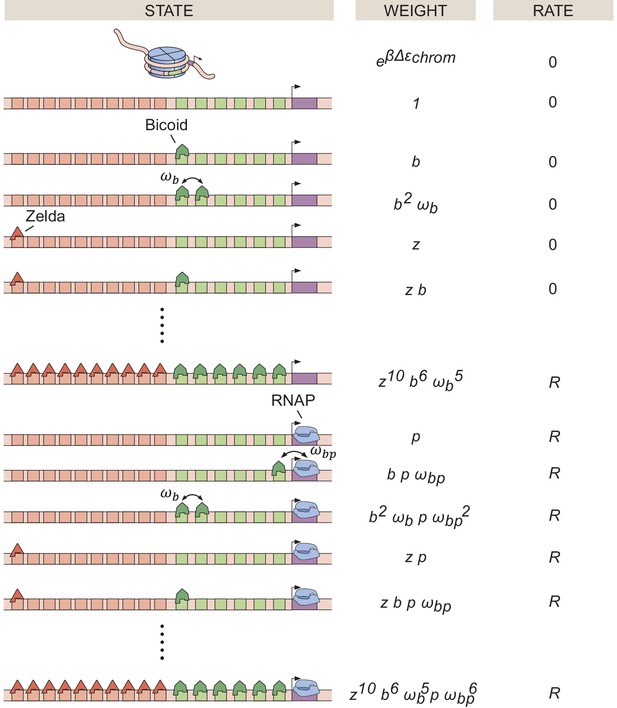
States, weights, and rate of RNAP loading diagram for the thermodynamic MWC model, containing 6 Bicoid-binding sites, 10 Zelda-binding sites, and a promoter.

Appendix 1—figure 3

Download asset Open asset

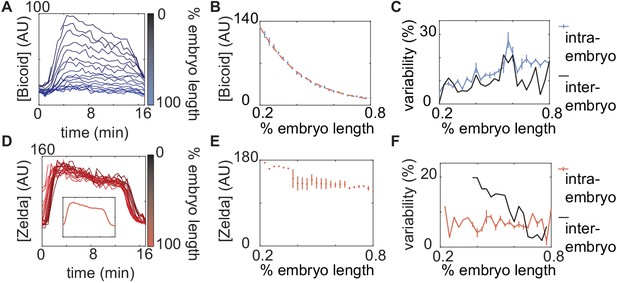
Measurements of input transcription-factor concentration dynamics .

(A) Nuclear eGFP-Bicoid concentration as a function of time into nuclear cycle 13 across various positions along the anterior-posterior axis of a single embryo. (B) eGFP-Bicoid concentration at 8 min into nuclear cycle 13 as a function of position along the embryo averaged over all measured embryos (n = 6). The fit of the concentration profile to an exponential function (dashed line) results in a decay length of $23 % \pm 0.6 %$ embryo length. (C) Intra- and inter-embryo variability in eGFP-Bicoid nuclear fluorescence along the anterior-posterior axis. (D) Zelda-sfGFP concentration as a function of time into nuclear cycle 13 across various anterior-posterior positions of a single embryo. (D, inset) Zelda-sfGFP concentration averaged over the data shown in D. (E) Zelda-sfGFP concentration at 8 min into nuclear cycle 13 as a function of position along the anterior-posterior axis of the embryo averaged over all measured embryos (n = 3). Note that anterior of 40% and posterior of 77.5% only a single embryo was measured; no error bars were calculated. (F) Intra- and inter-embryo variability in Zelda-sfGFP nuclear fluorescence along the anterior-posterior axis. (B,E, error bars represent standard error of the mean nuclear fluorescence, measured across embryos; C,F, error bars represent standard error of the mean intra-embryo variability, measured across embryos.).

Appendix 1—figure 4

Download asset Open asset

MS2 fluorescence calculation protocol.

RNAP molecules load onto the reporter gene at a time-dependent rate $R (t)$ , after which they elongate at a constant velocity v. Upon reaching the end of the gene after a length $L$ has been transcribed, they are assumed to terminate and disappear instantly, given by the time-shifted rate $R (t - \frac{L}{v})$ . The time-dependent MS2 fluorescence is calculated by summing the contributions of RNAP molecules that are located before, within, or after the MS2 stem loop sequence (i, ii, and iii, respectively).

Appendix 1—figure 5

Download asset Open asset

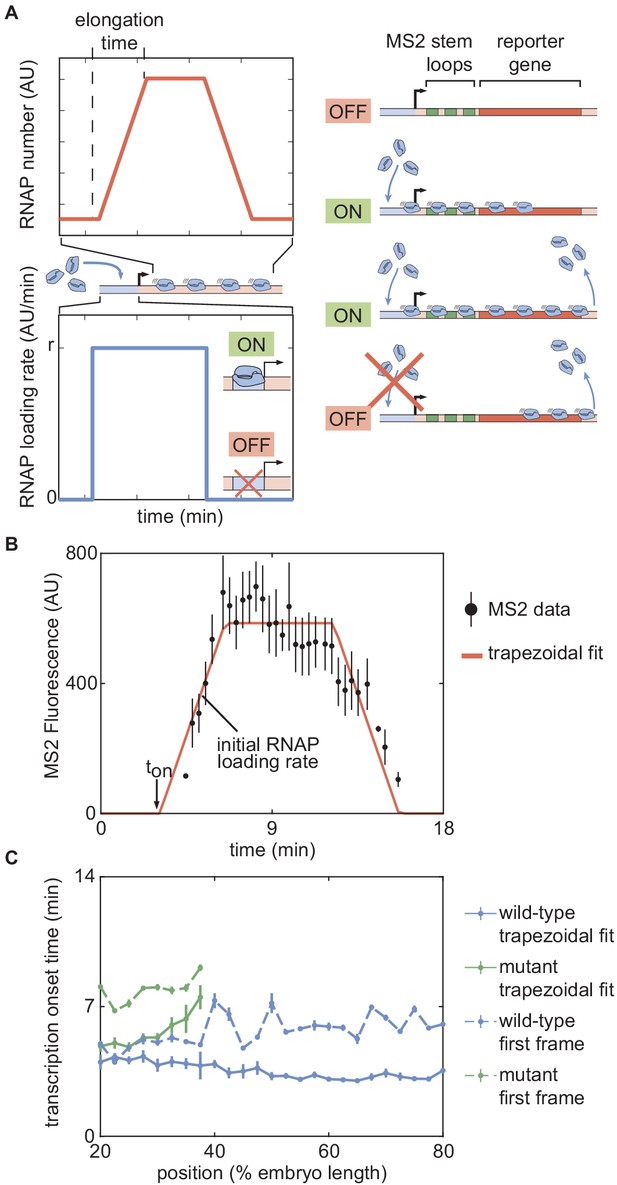
Outline of fitting to the trapezoidal model of transcription.

(A) The trapezoidal model of transcription, where transcription begins at an onset time $t_{o n}$ and loads RNAP molecules with a constant rate r. (B) Results of fitting the MS2 fluorescence data from a position in a single embryo to the trapezoidal model to extract $t_{o n}$ and the initial rate of RNAP loading. (C) Comparison of inferred $t_{o n}$ values between the trapezoidal model (solid lines) and using the time of first detection of signal in a fluorescence spot (dashed lines) for both wild-type and zelda⁻ backgrounds. (B, error bars are standard error of the mean averaged over multiple nuclei within the embryo, for data in a wild-type background at 50% along the embryo length; C, error bars are standard error of the mean, averaged across embryos).

Appendix 1—figure 6

Download asset Open asset

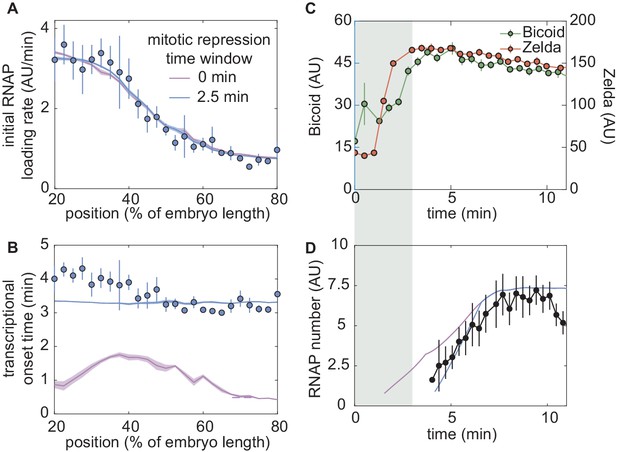
A thermodynamic MWC model including mitotic repression can recapitulate hunchback regulation by Bicoid and Zelda.

(A) Measured initial rates of RNAP loading and (B) $t_{o n}$ (blue points) across the length of the embryo, compared to fits to the thermodynamic MWC model with and without accounting for mitotic repression (blue and purple curves, respectively). (C) Nuclear concentration dynamics of Bicoid and Zelda with proposed mitotic repression window (gray shading). (D) Predicted MS2 dynamics with no mitotic repression term or a 3 min mitotic repression window compared to experimental measurements. (A,B, solid lines indicate mean predictions of the model and shading represents standard error of the mean, while points indicate data and error bars represent the standard error of the mean, across 11 embryos; C, D, data from single embryos at 45% of the embryo length with error bars representing the standard error of the mean across nuclei, errors in model predictions in D were negligible and are obscured by the prediction curve; fitted parameter values for a 3 min mitotic repression window were $Δ ϵ_{c h r o m} = 10 k_{B} T$ , $K_{b} = 34 A U$ , $K_{z} = 500 A U$ , with different arbitrary fluorescent units for Bicoid and Zelda, $ω_{b} = 10$ , $ω_{b p} = 0.4$ , for a model assuming six Bicoid-binding sites.).

Appendix 1—figure 7

Download asset Open asset

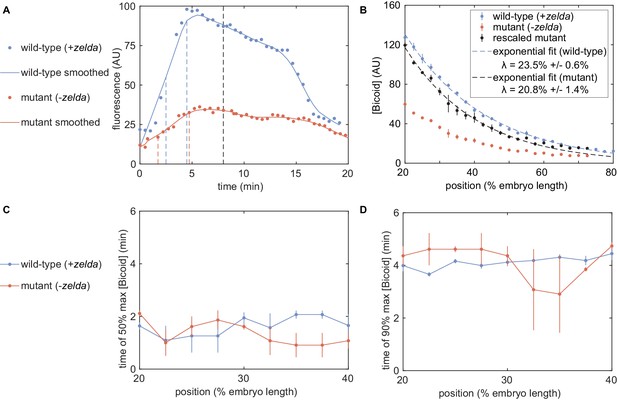
Comparison of eGFP-Bicoid measurements in wild-type and zelda⁻ embryos.

(A) Example mean nuclear eGFP-Bicoid concentrations for a single embryo at 30% along the embryo’s length, for wild-type (blue) and zelda⁻ (red) backgrounds. Datapoints are raw values and lines are smoothed results. The raw fluorescence at 8 min into the nuclear cycle, indicated by the black dashed line, is calculated to yield (B). The blue and red dashed lines correspond to the times to reach 50% and 90% of the maximum fluorescence for the smoothed wild-type and zelda⁻ signals, respectively. (B) eGFP-Bicoid measurements in wild-type (blue) and zelda⁻ mutant embryos (red), along with rescaled mutant profiles (black). Fits to an exponentially decaying function yield decay lengths in each background (blue and black dashed curves). (C, D) Time to reach 50% and 90% of maximum nuclear eGFP-Bicoid fluorescence for wild-type (blue) and zelda⁻ (red) backgrounds. A total of n = 3 embryos were measured in the zelda⁻ background, compared to n = 6 for wild-type. All error bars are standard error of mean across embryos.

Appendix 1—figure 8

Download asset Open asset

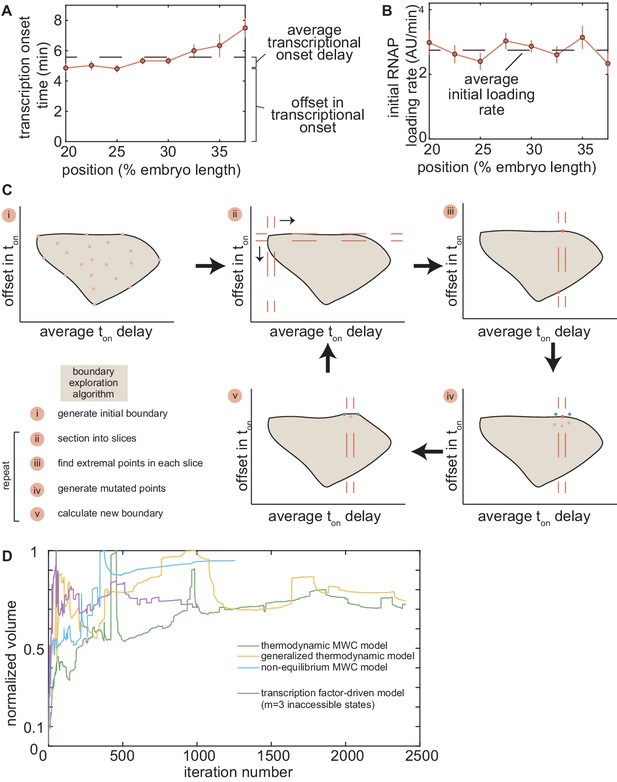
Description of state-space metrics and boundary-exploration algorithm.

(A) Representative average $t_{o n}$ delay (black dashed line) and $t_{o n}$ offset for the zelda⁻ background data in Figure 4D. (B) Average initial RNAP loading rate for the zelda⁻ background data in Figure 4C. (C) Overview of the boundary-exploration algorithm for an example state space containing two dimensions. (i) A set of 50 points with random input parameters generates an initial state space of the investigated model. (ii) The space is sectioned into 10 horizontal and 10 vertical slices. (iii) The extremal points of each slice are found. (iv) For each extremal point, five new points are generated with input parameters in a small neighborhood around the parameters of this extremal point. (v) The new space is plotted with these new points, and steps (ii) - (iv) are repeated. (D) Normalized volume of state-space domain of each model investigated in this work as a function of algorithm iteration number. All volumes approach a steady value, indicating convergence.

Appendix 1—figure 9

Download asset Open asset

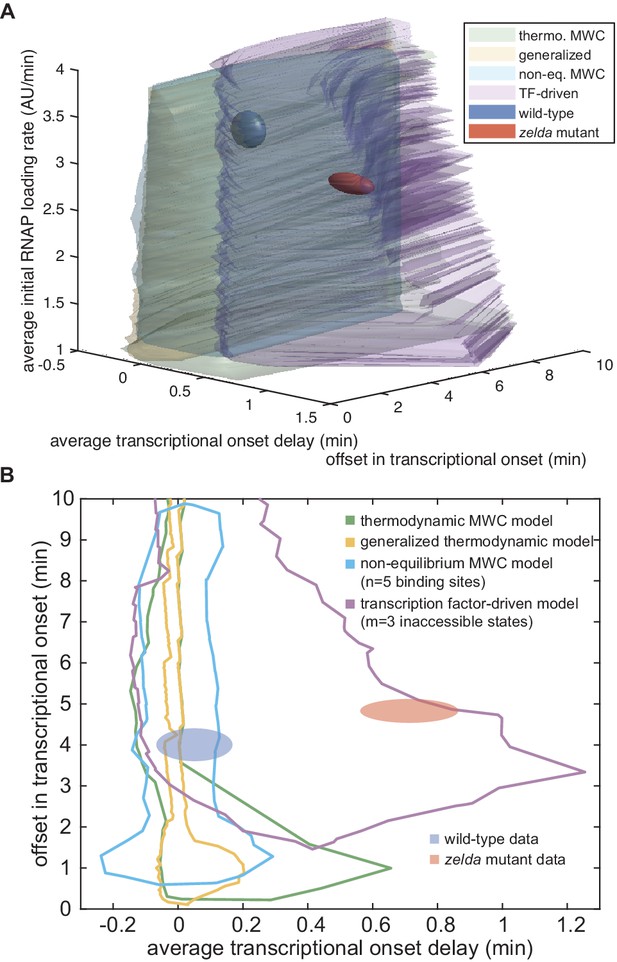
Exploration of state space.

(A) Three-dimensional state-space exploration, showing the extents of state space of the wild-type (blue) and zelda⁻ (red) data as well as of various models explored in the main text. See also Appendix 1—video 1 for an more comprehensive representation of our results. (B) Two-dimensional state-space exploration, created by projecting the three-dimensional state space in (A) for average initial loading rate values between 2.5 and 3.6 AU/min onto the xy-plane corresponding to the average $t_{o n}$ delay and $t_{o n}$ offset. Volumes (A) and areas (B) covered by the experimental data represent the standard error of the mean.

Appendix 1—figure 10

Download asset Open asset

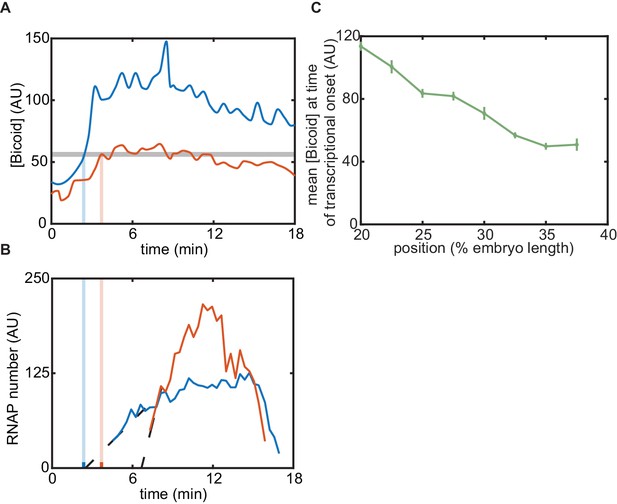
Intuition for failure of equilibrium models.

(A) Mean Bicoid concentrations for two positions along the embryo (blue, red), with a ‘threshold’ chosen to to attempt to match the corresponding $t_{o n}$ in (B). (B) MS2 fluorescence signal for the two positions shown in (A) for the zelda⁻ experiment. Note that no single threshold value of Bicoid can match the timings in (A) with the transcriptional onset times in (B). (C) Mean Bicoid concentration at $t_{o n}$ as a function of position for the zelda⁻ data.

Appendix 1—figure 11

Download asset Open asset

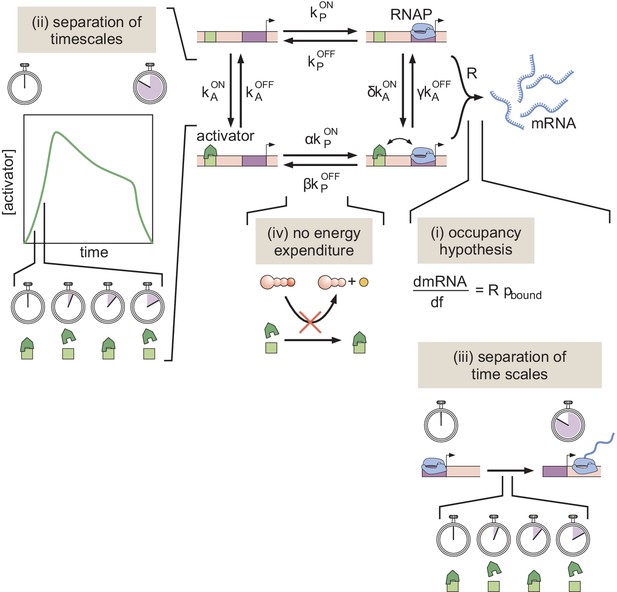
A simple kinetic model of transcriptional activation in which activator molecules influence RNAP binding kinetics.

The assumptions that make it possible to turn this kinetic model into a thermodynamic one are (i) the occupancy hypothesis, (ii, iii) a separation of time scales between binding and unbinding rates, and activator and mRNA production dynamics, respectively, and (iv) no energy expenditure (detailed balance).

Appendix 1—figure 12

Download asset Open asset

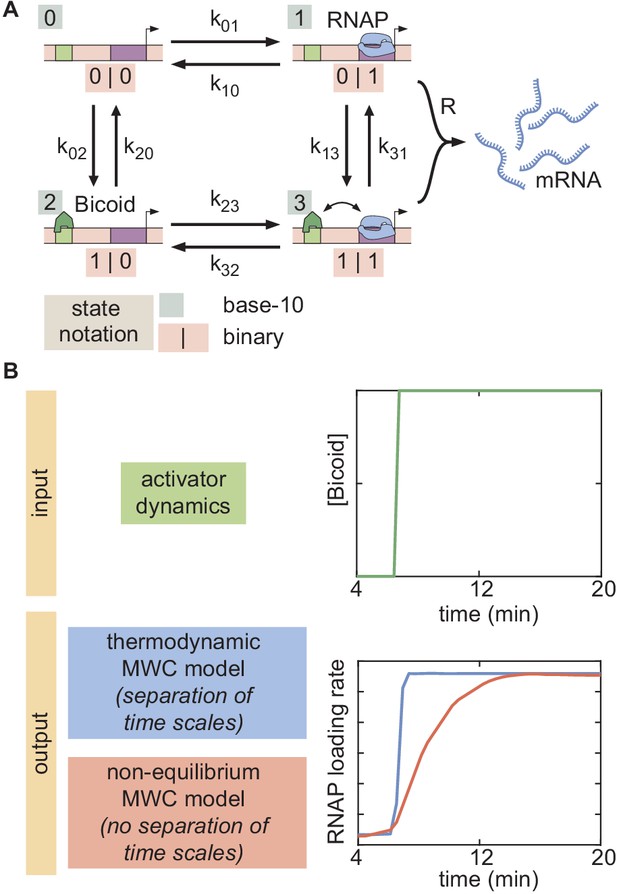
Example of a four-state time-dependent model with one Bicoid binding site and no closed chromatin state.

(A) The binary label for each state (light pink) can be converted into a base-10 label for each state (light teal). The transition rates $k_{i j}$ are defined as the transition rate from state i into state j using this labeling system. (B) For an example input activator concentration temporal profile that is a step function, the time-dependent response is compared for the cases of separation of time scales and lack thereof. In the former, the transcriptional initiation rate responds instantaneously to the increase in activator input, while the response is slower in the latter.

Appendix 1—figure 13

Download asset Open asset

State space exploration for non-equilibrium MWC model with strong mitotic repression for up to five Bicoid-binding sites.

Appendix 1—figure 14

Download asset Open asset

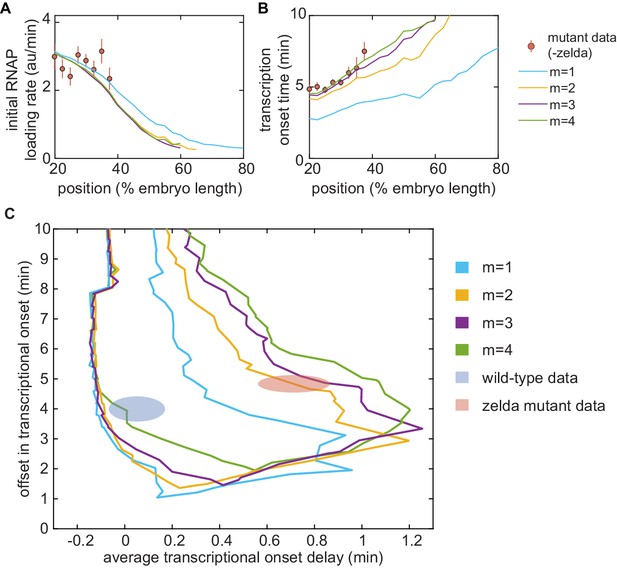
Testing the transcription-factor-driven model of chromatin accessibility.

(A,B) Best-fit results of the transcription-factor-driven model to the mutant zelda⁻ data. (A) initial RNAP loading rates, and (B) $t_{o n}$ , for varying numbers m of transcriptionally silent states. (C) Parameter exploration in average $t_{o n}$ delay and $t_{o n}$ offset state space for increasing values of m.

Appendix 1—figure 15

Download asset Open asset

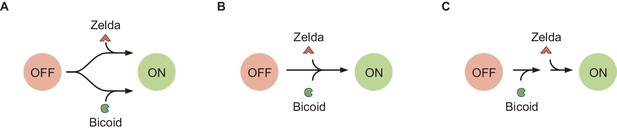
Different potential schemes of Bicoid- and Zelda-mediated transition into the accessible state, for a model with $m = 1$ transcriptionally silent state.

(A) The model used in the main text, where Bicoid and Zelda provide independent pathways for chromatin to transition into the accessible state. (B) A scheme where Bicoid and Zelda act simultaneously on the transition. (C) A scheme where Bicoid acts first, and then Zelda, on the same pathway.

Author response image 1

Download asset Open asset

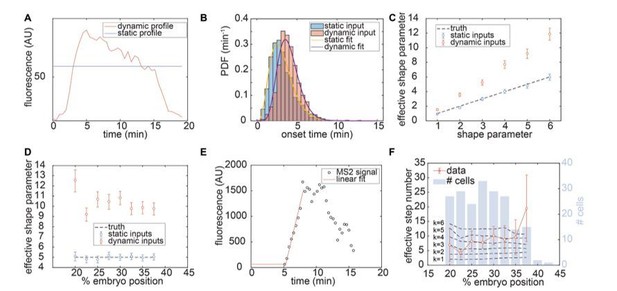
Single cell analysis of transcription onset times for zelda^- data.

(A) Comparison of Bicoid input profiles for dynamic (red) or static (blue) cases at 22.5% along the embryo length. Static profile was obtained for each position along the embryo length by computing the mean of the dynamic profile between 0 and 8 minutes. (B) Simulated transcription onset times of the transcription factor-driven model for k=5 intermediary states using dynamic (red) or static (blue) Bicoid inputs, along with Gamma-distribution fits (purple and yellow, respectively). (C) Effective shape parameter for simulated transcription onset times of the transcription factor-driven model for k=1 to k=6 intermediary transitions using dynamic (red) or static (blue) Bicoid inputs. (D) Effective shape parameter for k=5 intermediary transitions as a function of embryo position for dynamic (red) or static (blue) Bicoid inputs. (E) Extraction of single-cell transcription onset times using linear fit (red) from a single-cell MS2 signal (black). (F) Effective step number computed from single-cell transcription onset times as a function of embryo position, for zelda^- data (red) as well as for predictions of the transcription factor-driven model with k=1 to k=6 intermediary steps and dynamic Bicoid inputs (black dashed lines). The total number of cells per position bin is shown in light blue bars, for a total of n=12 datasets. (C, D, error bars reflect 95% confidence interval; F, error bars reflect bootstrapped standard error).

Videos

Video 1

Download asset

posterframe for video — Measurement of eGFP-Bicoid.

Movie of eGFP-Bicoid fusion in an embryo in nuclear cycle 13. Time is defined with respect to the previous anaphase.

Video 2

Download asset

posterframe for video — Measurement of Zelda-sfGFP.

Movie of Zelda-sfGFP fusion in an embryo in nuclear cycle 13. Time is defined with respect to the previous anaphase.

Video 3

Download asset

posterframe for video — Measurement of MS2 fluorescence in a wild-type background.

Movie of MS2 fluorescent spots in a wild-type background embryo in nuclear cycle 13. Time is defined with respect to the previous anaphase.

Video 4

Download asset

posterframe for video — Measurement of MS2 fluorescence in a zelda⁻ background.

Movie of MS2 fluorescent spots in a zelda⁻ background embryo in nuclear cycle 13. Time is defined with respect to the previous anaphase.

Video 5

Download asset

posterframe for video — Transcriptionally active nuclei in a wild-type background.

Movie of MS2 fluorescent spots in a wild-type background embryo in nuclear cycle 13, with transcriptionally active nuclei labeled with an overlay. Time is defined with respect to the previous anaphase.

Video 6

Download asset

posterframe for video — Transcriptionally active nuclei in a zelda⁻ background.

Movie of MS2 fluorescent spots in a zelda⁻ background embryo in nuclear cycle 13, with transcriptionally active nuclei labeled with an overlay. Time is defined with respect to the previous anaphase.

Appendix 1—video 1

Download asset

posterframe for video — Exploration of three-dimensional space consisting of average initial RNAP loading rate and offset and average delay in transcriptional onset time.

The models explored in the main text inhabit domains in this space, whereas the wild-type and zelda⁻ data inhabit ellipsoids of uncertainty. Whereas the thermodynamic MWC, generalized thermodynamic, and non-equilibrium MWC model with up to five Bicoid-binding sites cannot explain the zelda⁻ data, the transcription-factor-driven model with three inaccessible states can adequately encompass both datasets.

Additional files

Source code 1 mRNADynamics image analysis software.: https://cdn.elifesciences.org/articles/56429/elife-56429-code1-v2.zip
Download elife-56429-code1-v2.zip
Transparent reporting form: https://cdn.elifesciences.org/articles/56429/elife-56429-transrepform-v2.docx
Download elife-56429-transrepform-v2.docx

Download links

A two-part list of links to download the article, or parts of the article, in various formats.

Downloads (link to download the article as PDF)

Article PDF

Open citations (links to open the citations from this article in various online reference manager services)

Mendeley

Cite this article (links to download the citations from this article in formats compatible with various reference manager tools)

Elizabeth Eck
Jonathan Liu
Maryam Kazemzadeh-Atoufi
Sydney Ghoreishi
Shelby A Blythe
Hernan G Garcia

(2020)

Quantitative dissection of transcription in development yields evidence for transcription-factor-driven chromatin accessibility

eLife 9:e56429.

https://doi.org/10.7554/eLife.56429

Sign up for email alerts

Privacy notice