Investigating molecular crowding within nuclear pores using polarization-PALM
- The Texas A&M University Health Science Center, United States
- University of Toronto, Canada
Figures
Figure 1 with 2 supplements
p-PALM imaging.
The fluorescent protein mEos3 was photoactivated by UV illumination (405 nm), and excited by linearly or circularly polarized 561 nm light. The mEos3 fluorescence emission was separated by a 50:50 polarizing beam splitter and detected on two halves of an EMCCD camera. The images show four successive frame-pairs in which two molecules (red and blue boxes) of Pom121-mEos3 (see Figure 1—figure supplements 1,2 for all mEos3 fusion protein constructs used in this work) were detected at the bottom of the nucleus in a permeabilized HeLa cell using circular excitation (see Video 1). Fluctuating emission intensities (Ip and Is) result from changes in the molecules’ average orientation during the image integration time (t = 10 ms). The λ/2 and λ/4 waveplates were used to rotate the angle of linear polarization and to adjust the ellipticity, respectively, of the excitation beams. A 300 µm pinhole was used to reduce the illumination area to ~7 µm (narrow-field epifluorescence [Yang et al., 2004]). Scale bar: 1 µm.
Figure 1—figure supplement 1
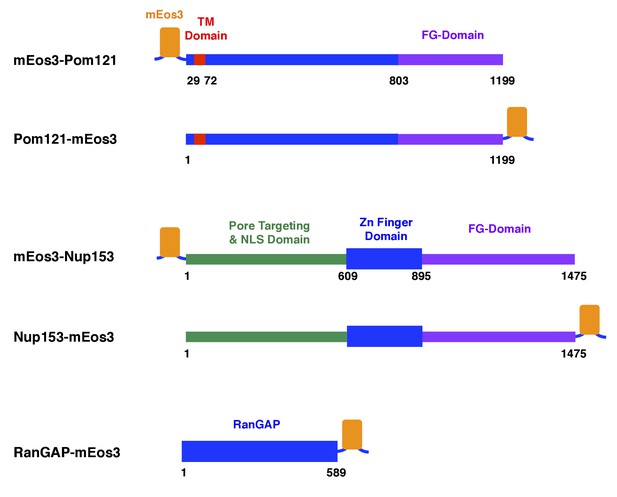
mEos3 fusion protein constructs with Pom121, Nup153, and RanGAP.
Domain structures for Pom121 and Nup153 were adapted from (Söderqvist et al., 1997) and (Nakielny et al., 1999), respectively.
Figure 1—figure supplement 2
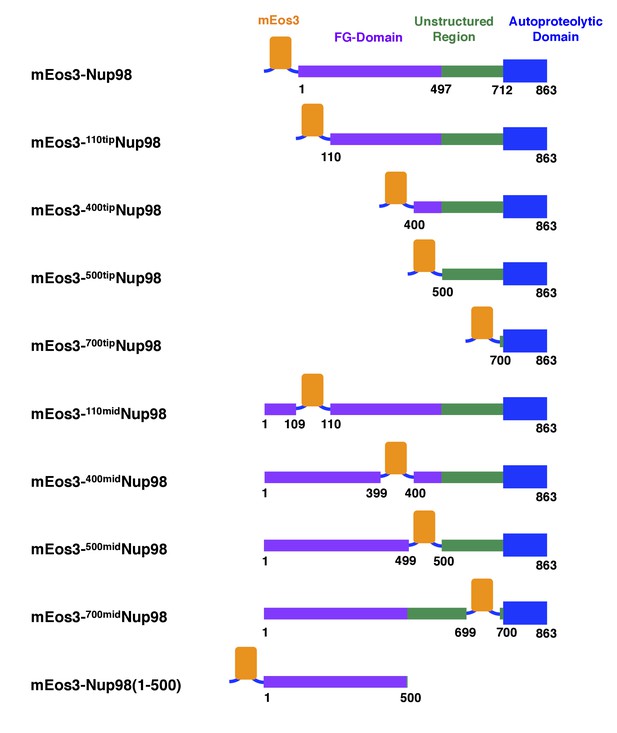
mEos3 fusion protein constructs with Nup98.
Domain structure adapted from (Ren et al., 2010).
Figure 2 with 7 supplements
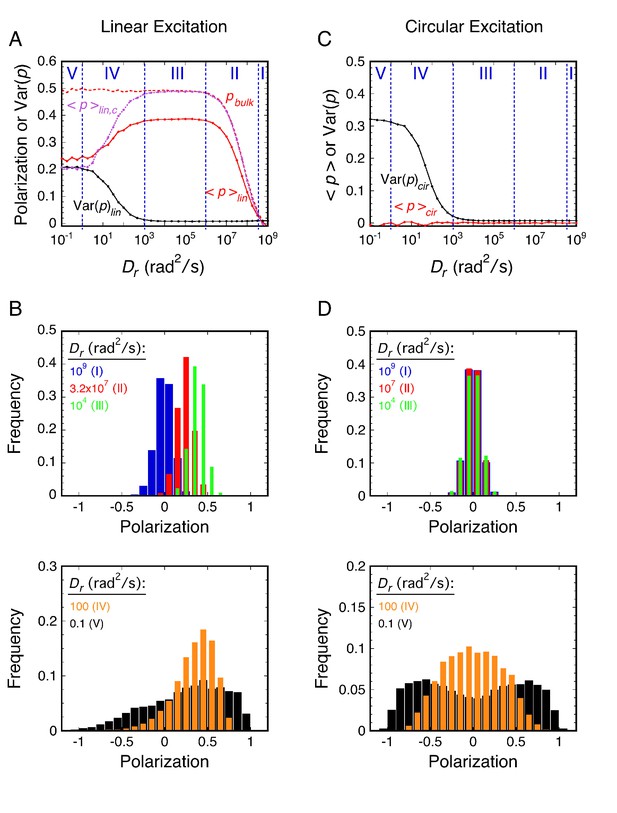
Principles of rotational mobility analysis by the p-PALM method.
Rotational random walk simulations were used to obtain polarization histograms, and the corresponding mean polarization, <p>, and its variance, Var(p), for different Dr values using linearly (A and B) and circularly (C and D) polarized excitation. The values predicted for single molecule measurements (solid) in A and C were calculated from polarization histogram data, such as that shown in B and D. The bulk polarization (pbulk) using linear excitation (A) was calculated assuming that the photons from all molecules (N = 10,000 per Dr value) were collected simultaneously in the two polarization channels. <p>lin,c is the mean polarization collected under p-PALM conditions corrected for the polarization mixing by the microscope objective. See text for discussion and Materials and methods for simulation details and definitions. In all panels: Dr = Dx = Dy = Dz = (Dx + Dy + Dz)/3 (i.e., a spherical particle); the noise (p photons, s photons) was (22, 15) and (15, 15) using linear and circular excitation, respectively; 1400 (circular) or 2800 (linear) rotational random walk steps per simulation, yielding an average of ~350 photons at high Dr values (see Figure 2—figure supplement 5); 10,000 initial values per simulation; t = 10 ms; τ (fluorescence lifetime) = 3.5 ns; θobj = 74.1°. The five rotational diffusion regimes (identified in A and C) are described in Figure 2—figure supplement 1. Figure 2—figure supplements 1–5 illustrate the influence of fluorescence lifetime, integration time, threshold, intensity shape factor, noise, ellipticity, and the number of collected photons on <p> and Var(p). Figure 2—figure supplement 6 illustrates the relationship between the number of measurements and the statistical measurement uncertainty. The anisotropy values, <r>bulk and <r>lin,c, are shown in Figure 2—figure supplement 7 and described in Materials and methods.
Figure 2—figure supplement 1
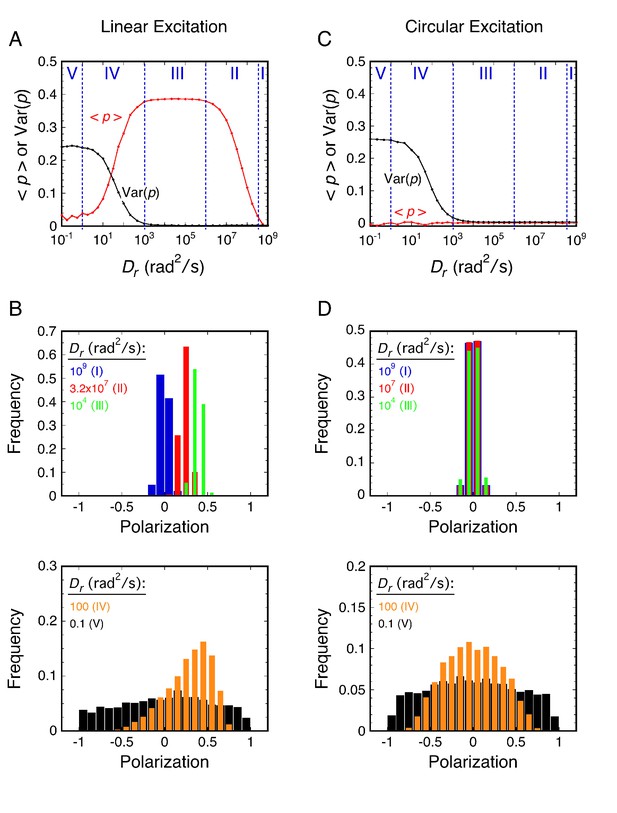
Principles of rotational mobility analysis by the p-PALM method – no threshold, intensity broadening, or background noise.
Results of the same rotational random walk simulations used for Figure 2 using the photons collected in each channel with no corrections – no threshold selection was used, the intensity distribution was not broadened, and no noise was added (see Materials and methods). The contributions of each of these experimentally determined conditions on <p> and Var(p) are more explicitly illustrated in Figure 2—figure supplement 4. The five rotational diffusion regimes (identified in (A) and (C)) are characterized as follows. (Regime I – very fast rotation) During the excited state of the fluorophore, the transition dipole orientation is virtually completely randomized. All measurements of p from collecting hundreds of photons are therefore close to 0 for each molecule, and hence, the polarization histograms are narrowly centered around 0 for both excitation modes. (Regime II – fast rotation) The fluorescent particle rotates significantly during its fluorescence lifetime, but the dipole orientation when excited influences the direction (polarization) of the observed emission. Thus, emission is preferentially observed toward the direction of linearly polarized excitation. However, the transition dipole is completely randomized between excitation events. Therefore, polarizations measured using circularly polarized excitation remain closely centered around 0. (Regime III – moderate rotation) The fluorescent particle does not rotate significantly during its fluorescence lifetime, and thus, linear excitation leads to strongly biased emission, with <p>lin ≈ 0.4. However, the transition dipole is essentially completely randomized between excitation events, and therefore, measured polarizations using circular excitation are closely centered around 0. (Regime IV – slow rotation) The transition dipole rotates between excitation events, but does not get completely randomized. Thus, individual polarization measurements are broadly distributed, with a width determined by the relationship between τ (is this the correct greek letter "tau"?) and Dr. (Regime V – very slow rotation) The transition dipole does not rotate significantly between excitation events. This time regime corresponds to particles that are essentially ‘stuck’, and returns all polarizations for a particle randomly oriented in three-dimensions. The threshold significantly affects <p> and Var(p) under conditions where the molecules are essentially immobile (regime V). Under circularly polarized excitation, dipoles having a large axial component are largely invisible when there is a 100 photon threshold due to the low excitation probability by the beam propagating in the z-direction. This causes a central dip in the histogram for Dr = 0.1 rad2/s with circular excitation (observed in Figure 2D, black, but not here in (D), due to the absence of a threshold), which increases Var(p)cir (compare (C) with Figure 2C). With linearly x-polarized excitation under low Dr conditions, molecules with negative polarizations are largely undetectable when there is a 100 photon threshold due to inefficient excitation (compare (B), black, with Figure 2B). The consequences of this threshold are an increase in <p> and a decrease in Var(p) (compare (A) with Figure 2A).
Figure 2—figure supplement 2
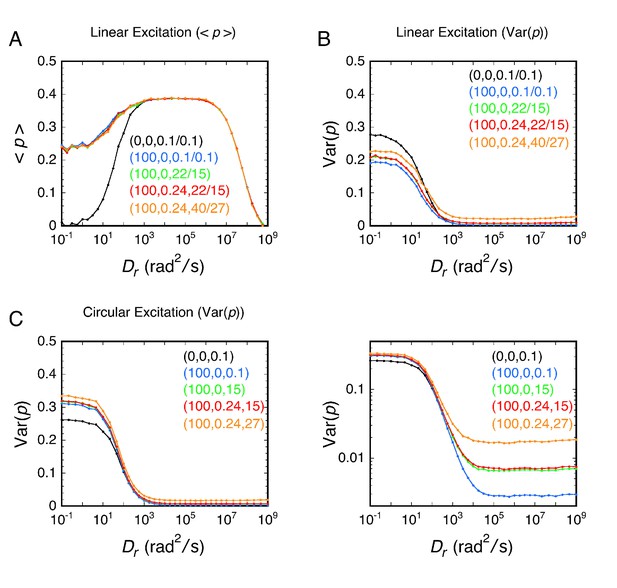
Effect of threshold, intensity shape factor, and background noise on <p> and Var(p).
Effects on <p> and Var(p) are shown for the indicated parameter values (threshold, σ*, p-noise/s-noise) for a spherical particle. The <p>cir was always 0, and therefore is not shown. Note that in (C), both linear (left) and log (right) ordinate scales are shown to illustrate the effect of thresholding and noise at low and high Dr values, respectively. Threshold and noise values are given in photons. σ* is the shape parameter of the log-normal intensity distribution (see Materials and methods). The threshold has a major impact on <p>lin (and a lesser impact on Var(p)cir) at low Dr values and noise primarily influences the Var(p)cir baseline at high Dr values. The value of the shape parameter has no significant effect.
Figure 2—figure supplement 3
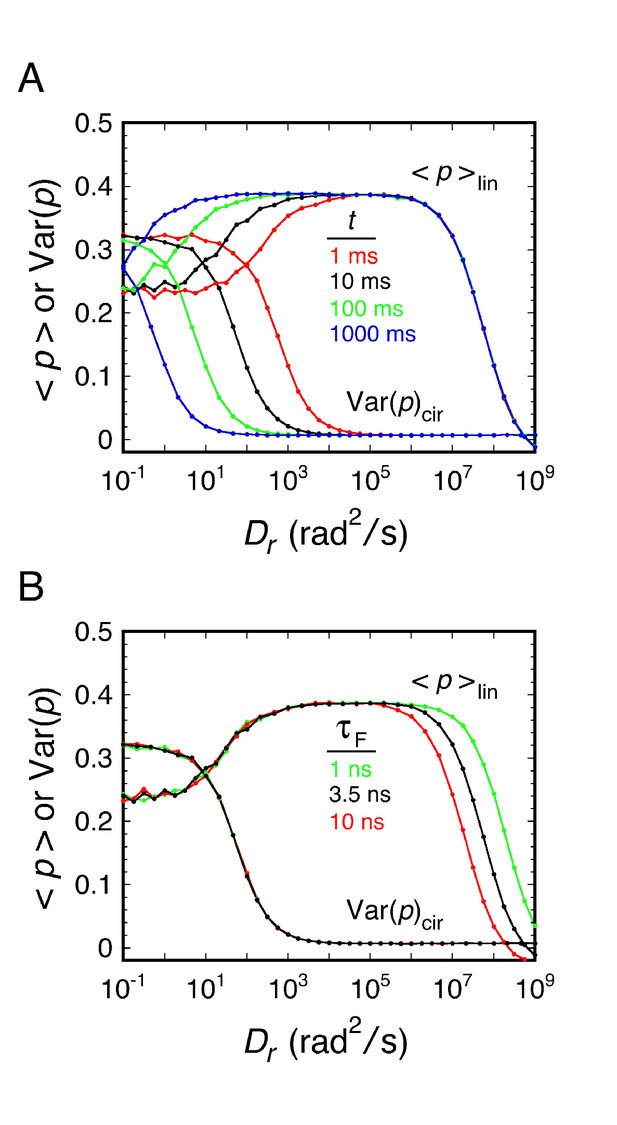
Effects of image integration time and fluorescence lifetime on <p>lin and Var(p)cir.
(A) Effect of image integration time (t). (B) Effect of fluorescence lifetime (τF). These simulations were performed under the conditions of Figure 2 , unless otherwise noted. These simulations demonstrate that τF determines the position of Regime II and that t determines the position of Regime IV (see Figure 2—figure supplement 1 for the definition of the rotational mobility regimes).
Figure 2—figure supplement 4
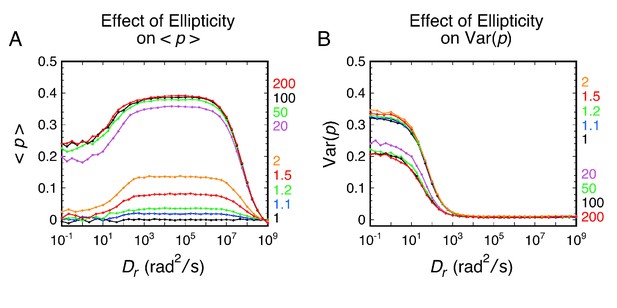
Effect of excitation ellipticity on <p> and Var(p).
(A) Effect of ellipticity on <p>. (B) Effect of ellipticity on Var(p). Ellipticity (ε) values of ≤ 1.2 represent close to circular excitation; while ε = 1 represents true circular excitation, optical effects or misalignment cause deviations from the ideal case, which is difficult to achieve. For ε ≥ 20, the excitation beam is p-polarized. 1400 (ε ≤ 2) or 2800 (ε ≥ 20) rotational random walk steps (Nsteps) were used per simulation. Other parameters are as in Figure 2.
Figure 2—figure supplement 5
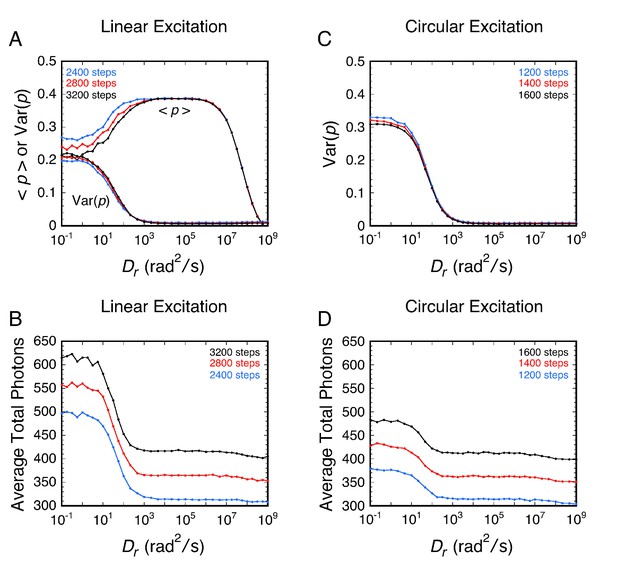
Influence of the total photons collected on <p> and Var(p).
In the case of circular excitation, the expected total number of photons recovered at the limit of high Dr is approximately given by Nphotons = Nsteps (1-cos(θobj))/3. The expected total photons recovered using linear excitation is 2-fold lower, which is why double the number of steps were used for (A) and (B) relative to (C) and (D). The graph values at the high Dr limits are higher than the formula predicts due to the baseline noise and the threshold (loss of information from < 0.5% of the molecules). The higher average total photons at low Dr values occurs because the threshold eliminates low emitting molecules.
Figure 2—figure supplement 6
Estimating the error in datasets used to calculate Var(p)cir – the number of data points required for reasonable estimates.
(A–D) Variations in the Var(p)cir for different sized datasets. Using the dataset in Figure 2C, estimates of Var(p)cir were obtained by dividing the data into four equivalently sized subsets of n = 100–500 data points. For each 4 x n dataset, the mean and standard deviations (SD) of the four individual means were calculated. The black points are means calculated under the indicated conditions, the red points are the means calculated for the entire N = 10,000 dataset for each set of conditions, and the red curves are fits to the total dataset using Equation 25. (E and F) Absolute and percent errors under the indicated conditions. These calculations suggest that the Var(p)cir value estimated from a p-PALM dataset of ~2000 points (4 × 500 points) differs from the ‘true’ value by an average of ~2–3%. The ‘true’ value was included in the range defined by the mean ± SD approximately 85% of the time, and thus this range defines the 85% confidence interval. In contrast, for a normal distribution, the mean ± SD should include the ‘true’ value approximately 68% of the time. Var(p)cir is therefore not normally distributed, which violates the assumption of most statistical tests.
Figure 2—figure supplement 7
Comparison of bulk anisotropy with the corrected anisotropy calculated from p-PALM experiments.
Bulk anisotropy (rbulk; green dashed line) and the corrected anisotropy from p-PALM experiments (<r>lin,c; green dotted line) are defined in Equations 22 and 24, respectively. The anisotropy calculated from the Perrin equation (r = r0/(1 + 6DrτF; red) is shown for comparison (r0 is the fundamental anisotropy attained as DrτF → 0) (Lakowicz, 2006). The deviation between r (from the Perrin equation) and rbulk (from simulations) at high Dr values is a consequence of the large step sizes at the highest Dr values, which can be corrected by using smaller time steps (black dashed line; N = ~20,000 photons, time step = 0.01 ns).
Figure 3 with 1 supplement
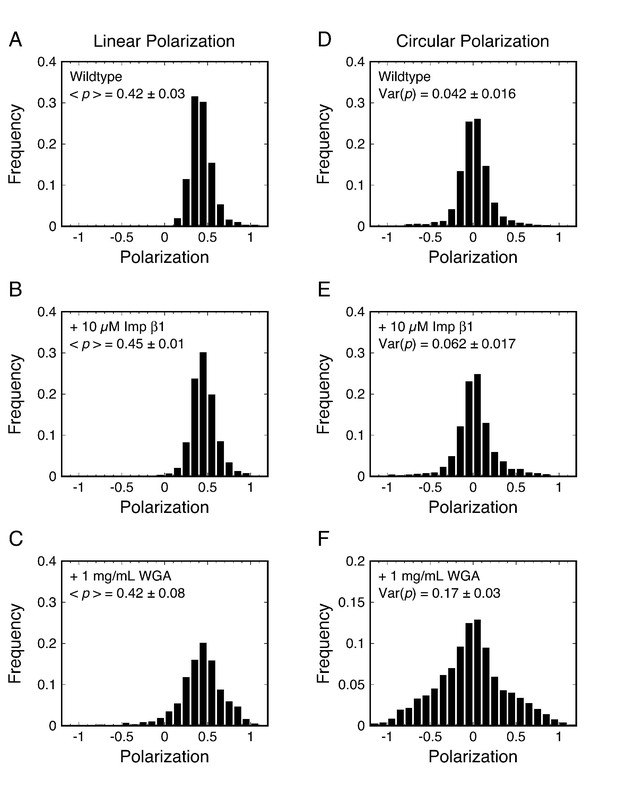
p-PALM polarization histograms for Pom121-mEos3.
Linearly (A–C) and circularly (D–F) polarized excitation was used to obtain experimental polarization histograms for mEos3 attached to the C-terminus of Pom121 (Pom121-mEos3) under the indicated conditions. t = 10 ms. See text for discussion.
Figure 3—figure supplement 1
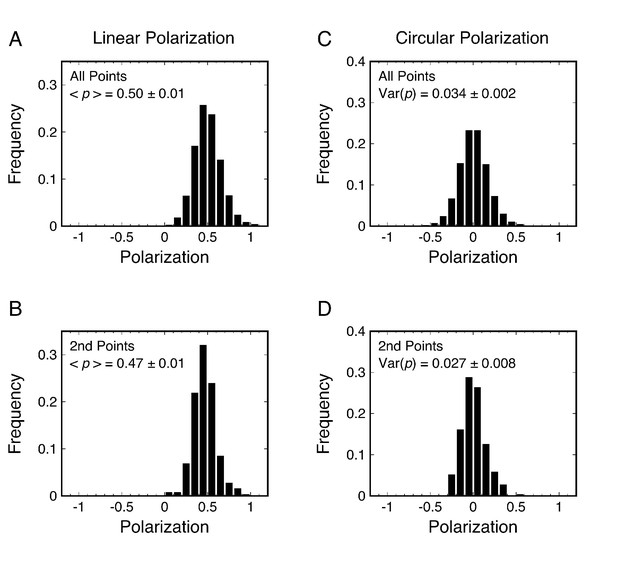
p-PALM polarization histograms for mEos3 in 92% glycerol.
Linearly (A–B) and circularly (C–D) polarized excitation was used. For (A) and (C), all observed spots were included. For (B) and (D), only the second point in trajectories with ≥ 3 points were used – this process weights trajectories equivalently and insures that activation of the probe does not occur during the observation window (see Supplementary file 1). t = 10 ms.
Figure 4
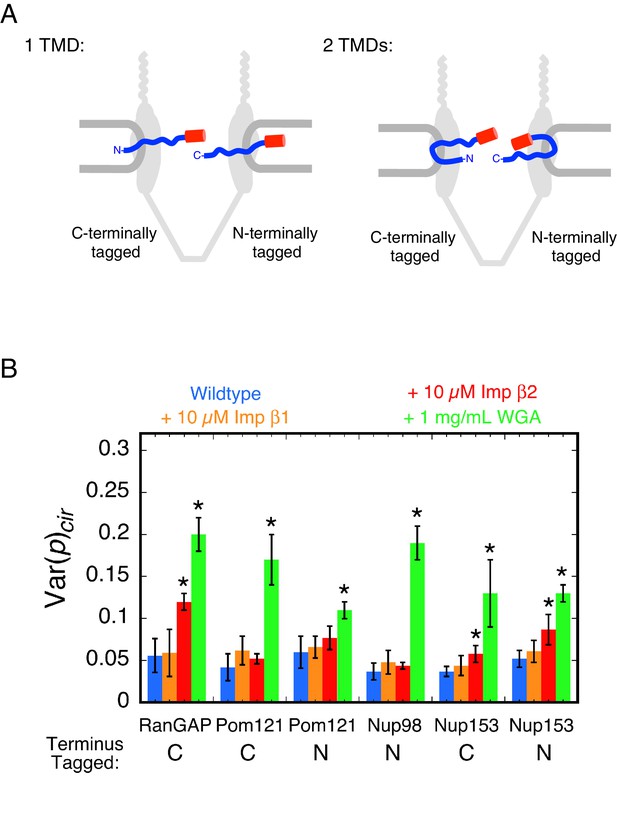
Pom121 membrane topology and rotational mobility of the mEos3 probe within the FG-network under various conditions.
(A) Possible membrane topologies of Pom121. The N-terminus of Pom121 is predicted to be in the lumen of the ER (1 TMD) or in the central pore (2 TMDs). TMD = transmembrane domain. (B) Var(p)cir values for various proteins under the indicated conditions. p-PALM data were obtained under the same conditions as in Figure 3. The stars (*) indicate significantly different values from the wildtype (blue) condition within the same group according to a two-sided Welch’s t-test (95%). Note that the actual significance level is expected to be higher than indicated since the error bars shown here are wider than a typical standard deviation (see Figure 2—figure supplement 6). A significant effect of WGA on the probe attached to the N-terminus of Pom121 suggests that this part of Pom121 is located within the central pore, and not in the ER lumen, and thus, that Pom121 has two TMDs (see (A)). 10 µM NTR ≈ 1 mg/mL.
Figure 5 with 1 supplement
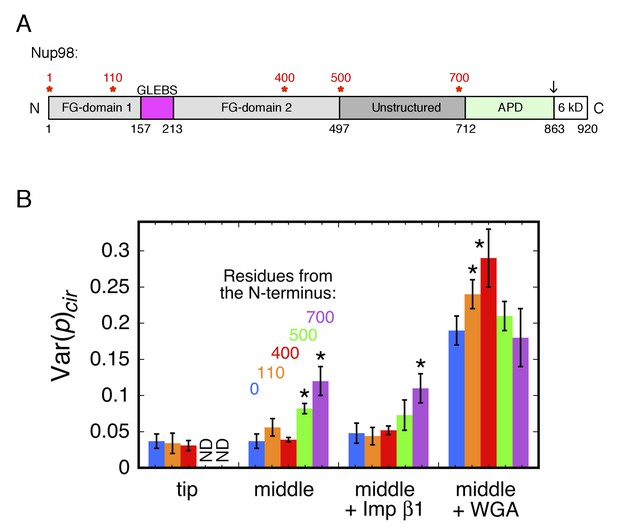
Rotational mobility of mEos3 at different positions within the Nup98 disordered domains.
(A) Human Nup98 domain structure. The GLEBS domain is a binding motif for RNA export factors. The autoproteolytic domain (APD) co-translationally cleaves the C-terminal 6 kDa domain after residue 863 (arrow) (Rosenblum and Blobel, 1999). Amino acids numbers are indicated on the bottom and stars (*) indicate the positions at which the mEos3 probe was incorporated (0, 110, 400, 500, 700 residues from the N-terminus). The regions from residues 1–157 and 213–712 are considered disordered. Adapted from (Ren et al., 2010). (B) Var(p)cir values obtained under the indicated conditions for the mEos3 probe at different positions within the Nup98 disordered domains (see A). p-PALM data were obtained as in Figure 3. The stars (*) indicate significantly different values from the wild-type (blue) condition within the same group according to a two-sided Welch’s t-test (95%). Note that the actual significance level is expected to be higher than indicated since the error bars are wider than a typical standard deviation (see Figure 2—figure supplement 6).
Figure 5—figure supplement 1
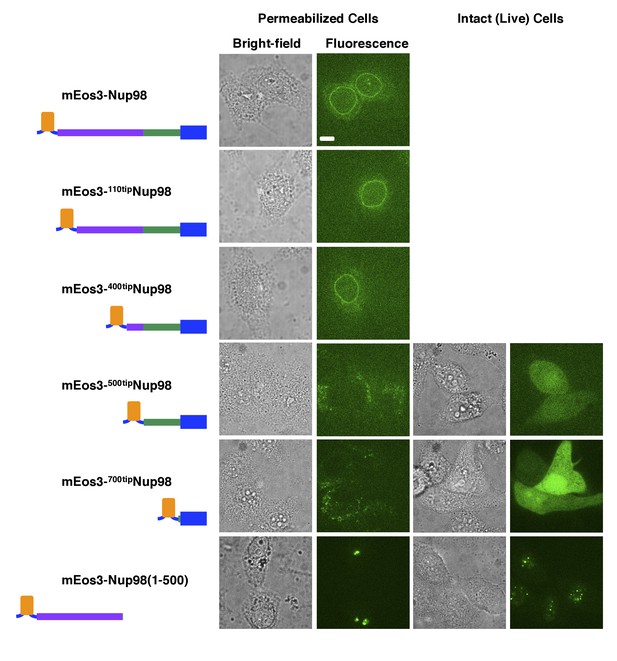
NE binding of Nup98 tip mutants.
The mEos3-Nup98, mEos3-110tipNup98, and mEos3-400tipNup98 proteins localize to the NE. The mEos3-500tipNup98, mEos3-700tipNup98, and mEos3-Nup98(1-500) proteins do NOT localize to the NE. These data indicate that both the FG-domain (purple) and the C-terminal domain (green + blue) are required for Nup98 to bind to the NPC. Fusion protein schematics are colored as in Figure 1—figure supplement 1. For the proteins indicated here, stable cell lines were only generated for the mEos3-Nup98 fusion protein (see Materials and methods) – the remainder were examined after transient transfections. Bar = 10 µm.
Figure 6 with 8 supplements
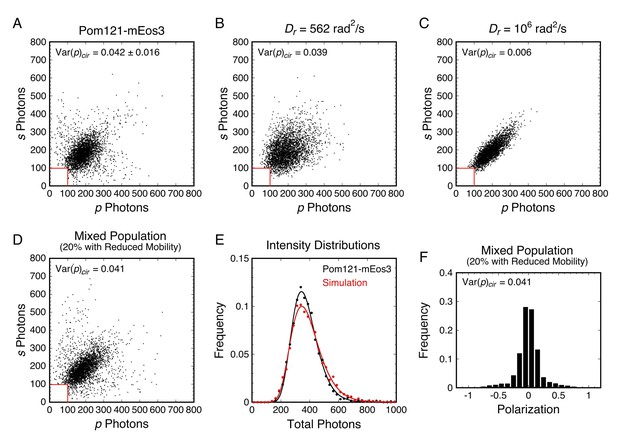
Analysis of rotational mobility within mixed populations.
(A) Scatterplot of the numbers of photons collected in the p- and s-polarization channels for Pom121-mEos3 (data from Figure 3D; N = 3463). Each dot corresponds to one molecule. (B and C) Simulated photon scatterplots under the indicated conditions, assuming the NAeff = 1.02 in water (θobj = 50°; see Appendix 1—figure 4). The results in (B) were simulated with the value of Dr corresponding approximately to the Var(p)cir in (A), whereas (C) corresponds to a higher rotational mobility at the high end of Regime III. The scatter is significantly wider in (A) than either (B) or (C), suggesting a mixed population. (D) Simulated photon scatterplot for a mixed population consisting of 80% of molecules with Dr,1 = 3160 rad2/s and 20% with Dr,2 = 100 rad2/s. Despite a relatively flat Var(p)cir curve in Regime III, the scatterplots become narrower as Dr increases from 103 to 106 rad2/s (Figure 6—figure supplement 3). The prevalent rotational mobility in the population was chosen guided by the width of the central scatter in (A) compared with the scatterplots in Figure 6—figure supplement 3. (E) Total photon intensity histograms of the experimental results in (A) and the mixed population simulation in (D), fit to a log-normal distribution. (F) Polarization histogram from the results in (D) (compare with Figure 3D). These results support the hypothesis that the p-PALM data for Pom121-mEos3 arise from a mixed population. For appropriate visual comparison with the experimental dataset in (A), N ≈ 3500 for all simulations. The red box near the origin identifies the region eliminated by the 100 photon threshold. Figure 6—figure supplements 1–8 show additional experimental and simulated photon scatterplots, and the effect of γ under highly anisotropic conditions on polarization histograms.
Figure 6—figure supplement 1
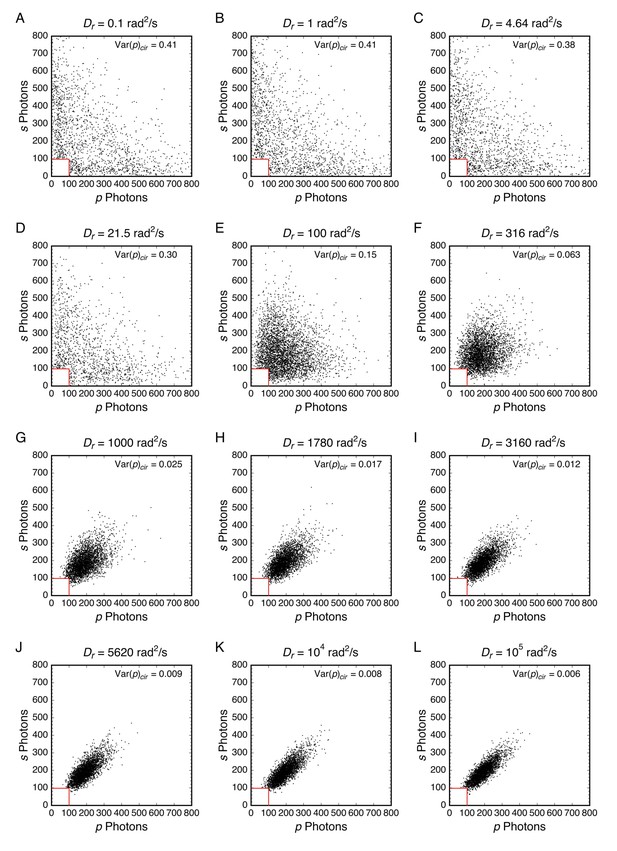
Simulated photon scatterplots using circular excitation.
Over a range in Var(p)cir values that are difficult to distinguish experimentally (~103–105 rad2/s), the widths of photon scatterplot distributions clearly narrow as Dr increases (see also Figure 6B and C). As the width of the scatter for Dr = 3160 rad2/s (I) reasonable approximates the central part of the distribution in Figure 6A, this rotational diffusion constant was assumed to be the dominant rotational mobility for the mixed population in Figure 6D. Data from Appendix 1—figure 4 for θeff ≈ 50° (NAeff = 1.02).
Figure 6—figure supplement 2
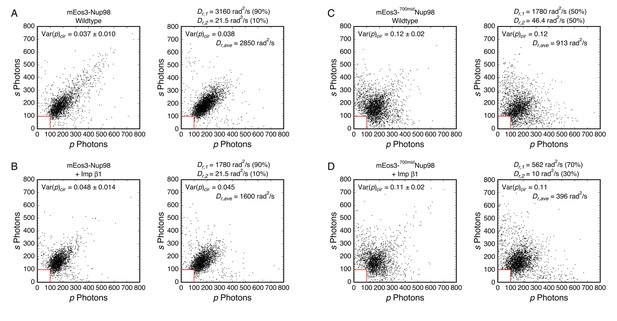
Mixed rotational mobility populations for mEos3-Nup98 and mEos3-700midNup98.
Experimental (left) and simulated (right) photon scatterplots were generated under the indicated conditions. (A) Wild-type mEos3-Nup98 (N = 2242) and simulated scatterplot (N = 2455; 2846 steps → Nphotons ≈ 400). (B) mEos3-Nup98 + 10 µM Imp β1 (N = 2180) and simulated scatterplot (N = 2416; 2439 steps → Nphotons ≈ 350). (C) Wild-type mEos3-700midNup98 (N = 2411) and simulated scatterplot (N = 2396; 2439 steps → Nphotons ≈ 350). (D) mEos3-700midNup98 + 10 µM Imp β1 (N = 2840) and simulated scatterplot (N = 2800; 2439 steps → Nphotons ≈ 350). For all simulations, θobj = 50° (NAeff = 1.02; see Appendix 1—figure 4). The average weighted rotational diffusion constant was defined as Dr,ave = f1Dr,1 + f2Dr,2, where f1 and f2 are the fractional sub-populations.
Figure 6—figure supplement 3
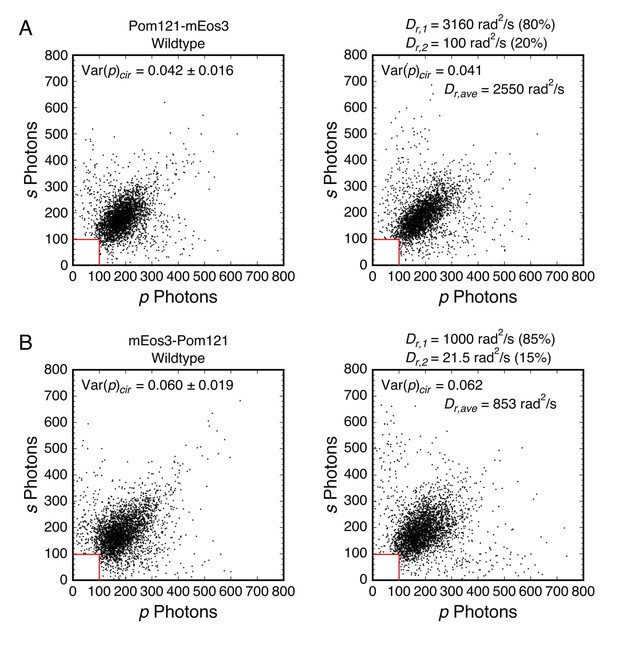
Mixed rotational mobility populations for mEos3-tagged Pom121.
Experimental (left) and simulated (right) photon scatterplots were generated under the indicated conditions. (A) Wild-type Pom121-mEos3 (N = 3464) and simulated scatterplot (N = 3461). (B) Wild-type mEos3-Pom121 (N = 3561) and simulated scatterplot (N = 2913). For easier comparison between the two fusion proteins, the plots in (A) are from Figure 6A and D. For all simulations, θobj = 50° (NAeff = 1.02; see Appendix 1—figure 4). See Figure 6—figure supplement 2 for the definition of Dr,ave.
Figure 6—figure supplement 4
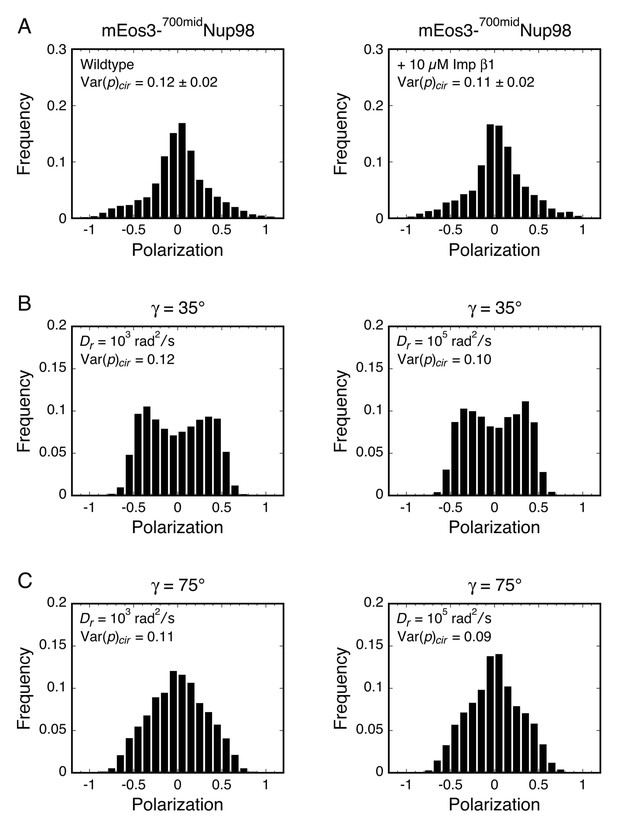
Polarization histograms for γ far from the magic angle for Dz/Dxy = 105.
(A) Polarization histograms for mEos3-700midNup98 under wt and +Imp β1 conditions (see Figure 5B). (B and C) Simulated polarization histograms for γ = 35° and 75° for Dz/Dxy = 105 at low and high Dr values (see Appendix 1—figure 2). Despite the similar Var(p)cir values for the histograms in (B) and (C) to those in (A), the shapes are significantly different, indicating that the γ and Dz/Dxy constraints are inconsistent with the experimental results.
Figure 6—figure supplement 5
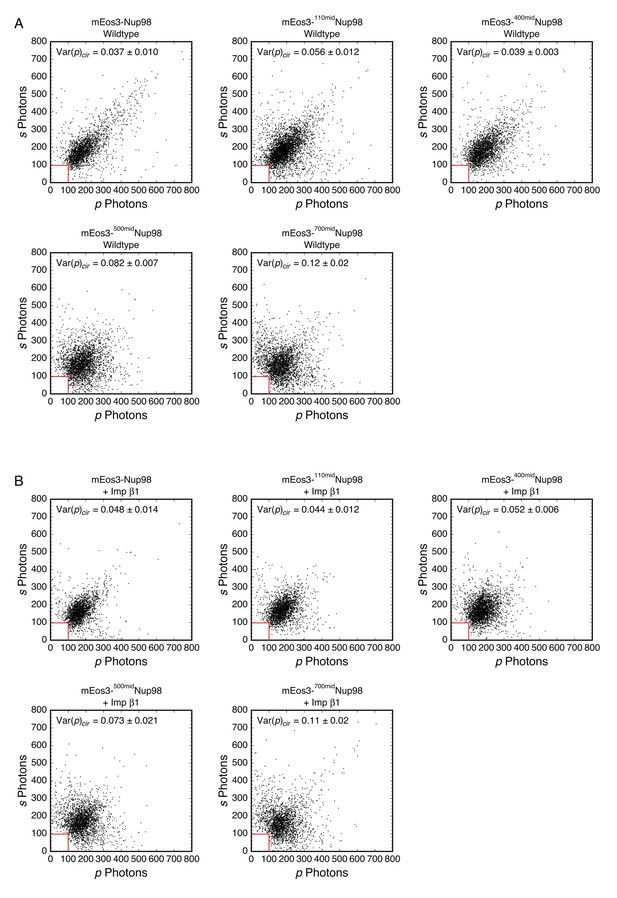
Photon scatterplots for data in Figure 5B (wt and +Imp β1).
(A) mEos3-Nup98 middle mutants with no additions. (B) mEos3-Nup98 middle mutants + 10 µM Imp β1.
Figure 6—figure supplement 6
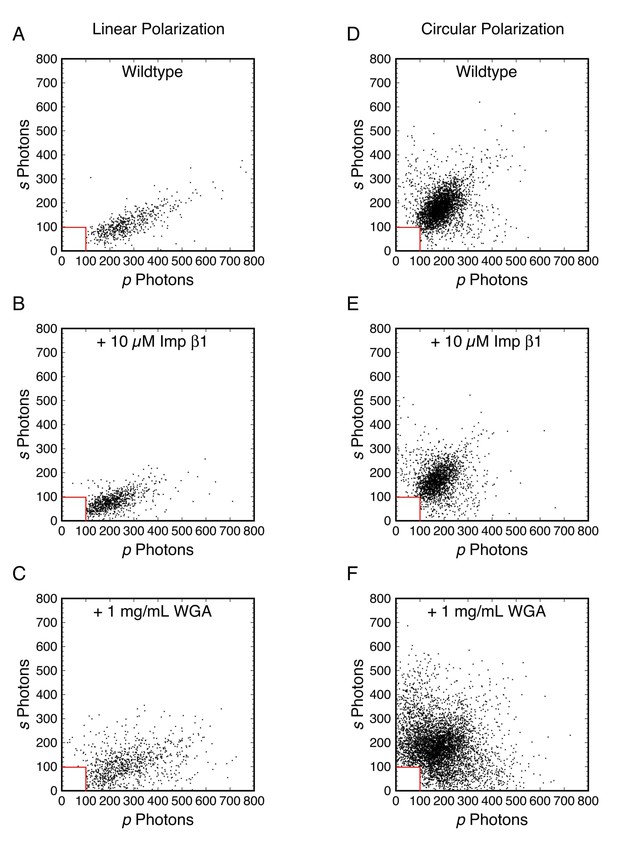
Photon scatterplots for the data in Figure 3.
(A)-(F) correspond to Figure 3A–3F.
Figure 6—figure supplement 7
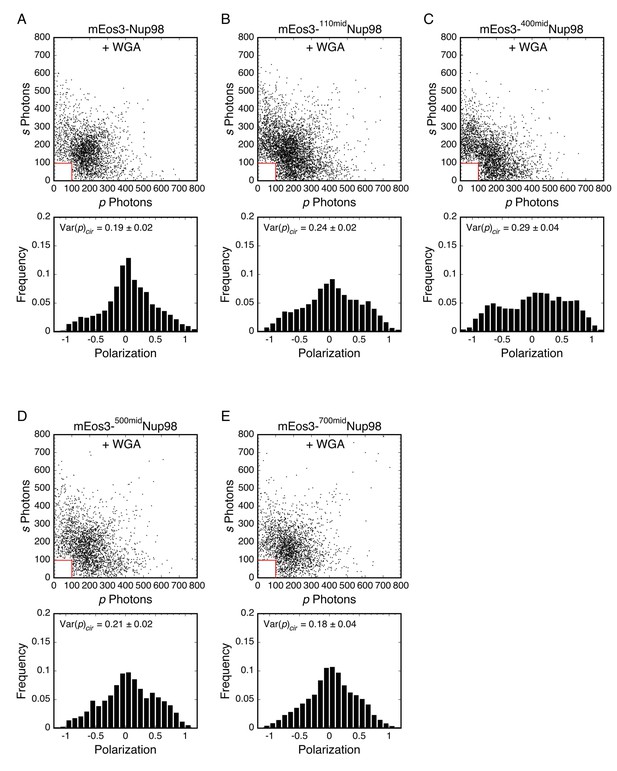
Photon scatterplots and polarization histograms for data in Figure 5B (+WGA).
mEos3-Nup98 middle mutants + 1 mg/mL WGA.
Figure 6—figure supplement 8
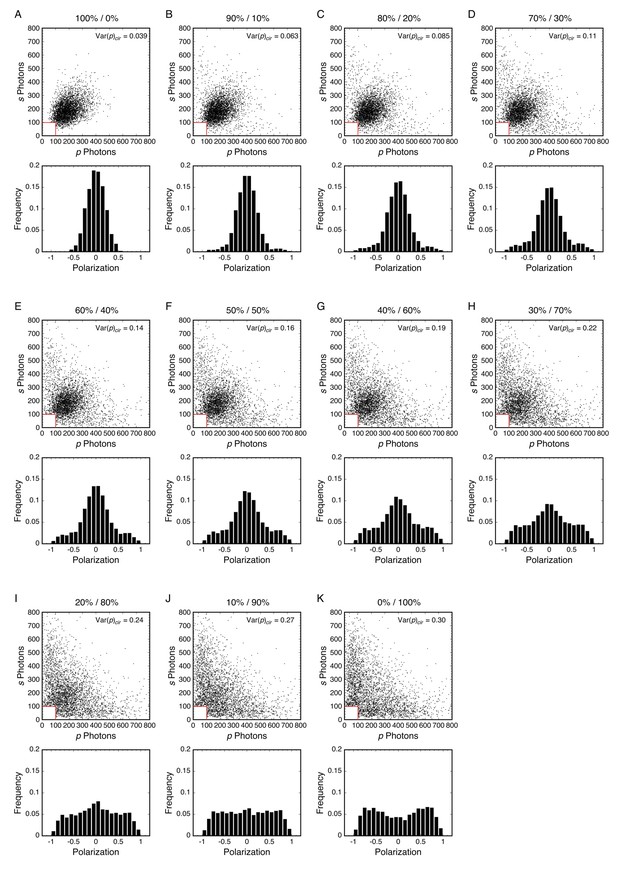
Mixed rotational mobility populations modeling mEos3-Nup98 middle mutants + WGA.
Simulated photon scatterplots and polarization histograms are shown for mixed populations, assuming Dr,1 = 562 rad2/s (first %) or Dr,2 = 21.5 rad2/s (second %). Comparison with Figure 6—figure supplement 7 suggests that the experimental p-PALM data for the mEos3-Nup98 middle mutants with 1 mg/mL WGA can be explained by a mixture of two populations with different rotational mobilities. For all simulations, θobj = 50° (NAeff = 1.02; see Appendix 1—figure 4). N = 3369–3959; Nphotons = 389–437.
Figure 7 with 1 supplement
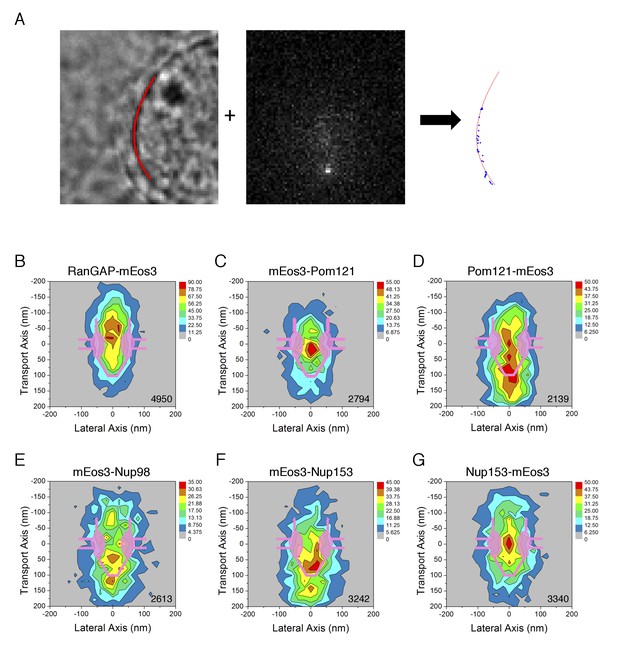
PALM 2D distribution maps of the mEos3 probe within the FG-Network attached to various proteins.
(A) PALM imaging approach. (left) Nuclear envelope (NE) position. The position of the NE (red) was determined from a bright-field image at the nuclear equator, as described previously (Yang and Musser, 2006b). (middle) PALM imaging using circular excitation. In this example, the fluorescence from RanGAP-mEos3 was determined as in p-PALM imaging, except that a polarizer was not used and a single image per timepoint was collected (see Video 2). (right) mEos3 locations vis-à-vis the NE. The position of mEos3 in PALM spots was determined by 2D Gaussian fitting, and mEos3 positions (blue) were overlaid onto the NE position (red). (B)-(G) 2D particle distribution maps generated from PALM data. Spots/trajectories from different NPCs in PALM images (A) were aligned and overlaid, and two-dimensional (2D) distribution maps were generated by quantifying the number of localizations in a 400 nm x 400 nm area (20 nm x 20 nm ‘pixels’; see Materials and methods for details). The total number of spots/map is indicated in the bottom right corner (from 3 to 6 cells; 6–10 NPCs/cell).
Figure 7—figure supplement 1
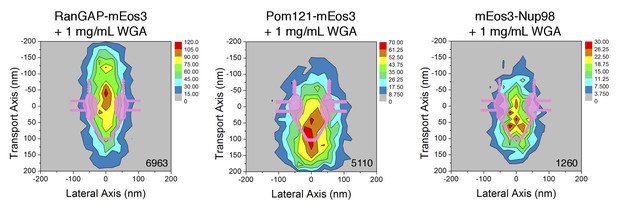
2D distribution maps of the mEos3 probe within the FG-Network in the presence of WGA.
PALM data were collected as in Figure 7, except in the presence of 1 mg/mL WGA. The total number of spots/map is indicated in the bottom right corner (from 3 to 6 cells; 6–10 NPCs/cell).
Figure 8
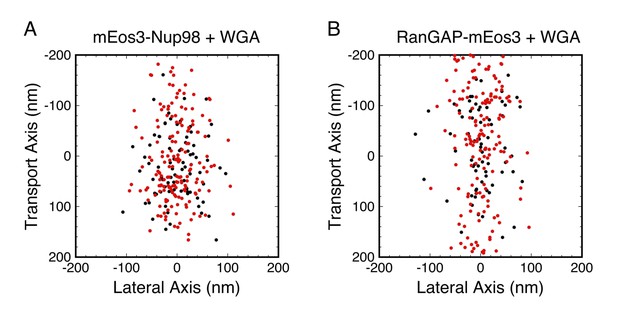
Combined PALM and p-PALM measurements of mEos3-Nup98 and RanGAP-mEos3 in the presence of WGA.
Data were collected as in Figure 7, except that the emission was divided into p- and s-polarization channels. Each dot corresponds to one image from one probe molecule. (red) 1.2 > |pcir| > 0.3, which is only expected for slowly rotating molecules with Dr < ~103 rad2/s; (black) |pcir| < 0.3, which could be observed for molecules with any Dr (see Figure 2C and D). The two color-coded populations have similar broad distributions in each panel, suggesting that WGA influences rotational mobility throughout most, if not all, of the FG-network. Total number of molecules and distribution widths (mean ± SD along the transport axis): (A) N = 359, (black) 17 ± 65 nm, (red) 5 ± 77 nm; (B) N = 386, (black) −8 ± 84 nm, (red) 21 ± 117 nm.
Appendix 1—figure 1
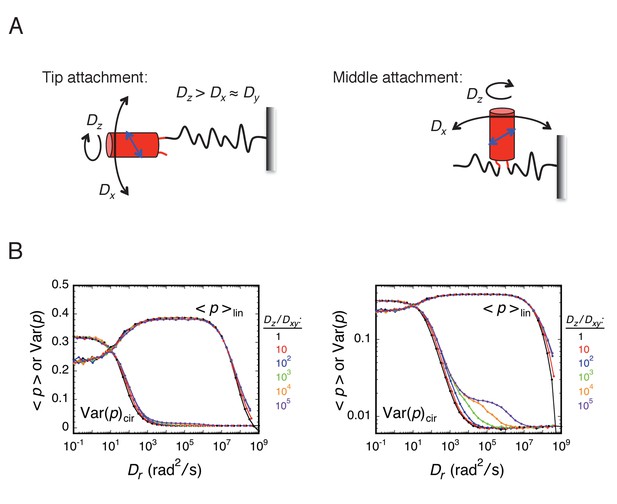
Effect of rotational anisotropy, expressed through the Dz/Dxy ratio, on <p>lin and Var(p)cir.
(A) Rotational modes for an mEos3 protein attached to the tip or middle of an FG-polypeptide. For 'tip' attachment, free rotation of mEos3 (red) is expected around the bonds in the tether (polypeptide link). 'Middle' attachment could potentially inhibit z-axis rotation because this would require the linked FG-polypeptides to twist in a coordinated fashion. Rotational motion about the three rotational axes could potentially be differentially influenced by local crowding and the motion of the FG-polypeptide domain(s) to which the probe is attached. The transition dipole (blue) is assumed to be within the yz-plane at an angle of ~60° to the rotational z-axis (assumed to be approximately equivalent to the β-barrel axis). (B) Effect of the Dz/Dxy ratio. Rotational random walk simulations were used to estimate <p>lin and Var(p)cir for different Dz/Dxy ratios, assuming t = 10 ms. Under these conditions, the effect of the Dz/Dxy ratio is relatively minor (but see Appendix 1—figures 2 and 3). The panel on the right shows the same information as the one on the left, except that the ordinate is log-scaled to more easily reveal the biphasic relationship of Var(p)cir with Dr at high Dz/Dxy ratios.
Appendix 1—figure 2
Effect of γ on the relationship between Var(p)cir and Dr.
Rotational random walk simulations were used to estimate Var(p)cir for different γ values (t = 10 ms). (A) Dz/Dxy = 100. (B) Dz/Dxy = 100,000.
Appendix 1—figure 3
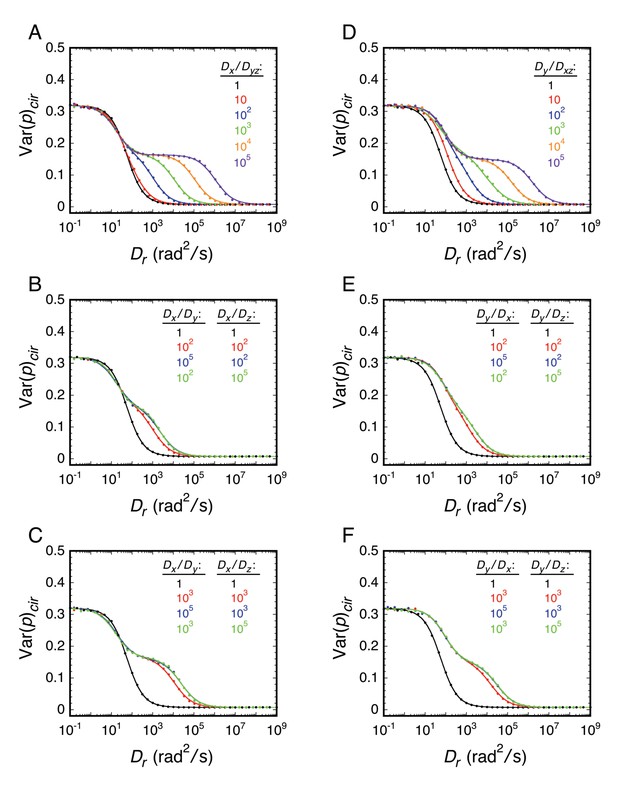
Effect of rotational diffusion constant anisotropy on the relationship between Var(p)cir and Dr.
For all results in this figure, γ = 60° and the transition dipole was in the yz-plane. (A) Effect of the Dx/Dyz ratio, where Dyz = Dy = Dz. (B and C) Effect of Dx > Dy, Dx > Dz, and Dy ≠ Dz. Since the transition dipole was in the yz-plane, Dx = 100Dy = 100Dz is equivalent to γ = 90° in Appendix 1—figure 2A. (D) Effect of the Dy/Dxz ratio, where Dxz = Dx = Dz. (E and F) Effect of Dy > Dx, Dy > Dz, and Dx ≠ Dz. Since the transition dipole was in the yz-plane 60° from the z-axis, Dy = 100Dx = 100Dz is equivalent to γ = 30° in Appendix 1—figure 2A. In all cases, Dr = (Dx + Dy + Dz)/3 and t = 10 ms. Results were fit using Equations 25 and 27. In (B), (C), (E), and (F), the green and blue curves overlap. The large effects of diffusion constant anisotropy shown here contrast with the small effects shown in Appendix 1—figure 1. The latter results from the assumption that γ = 60°, which is near the magic angle of 54.7° (see Appendix 1—figure 2).
Appendix 1—figure 4
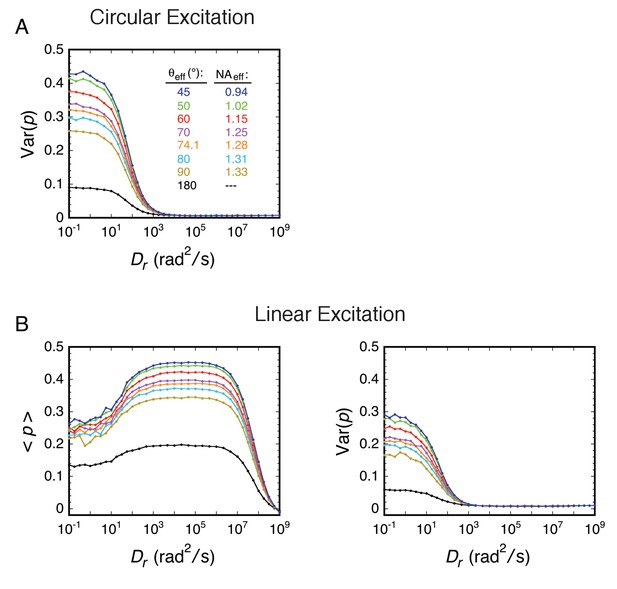
Effects of the numerical aperture.
(A) Effect of the NA on Var(p)cir. (B) Effect of the NA on <p>lin and Var(p)lin. For most of the rotational random walk simulations reported in this paper, we have assumed an angular semi-aperture of θobj = 74.1°, where θobj is the half-angle of the cone of light recovered by the microscope objective. This θobj was calculated in the normal way (Ha et al., 1999), assuming an immersion oil refractive index of n = 1.518 and NA = n sin(θobj) where NA = 1.46 is the value inscribed on the objective. This approach assumes that the sample is embedded in a medium with refractive index equivalent to that of immersion oil. The NAeff for an aqueous sample depends on a variety of factors, including distance from the surface, spherical aberrations, and scattering. Our p-PALM data are most consistent with θeff ≈ 50–60° (see text for details). In the figure, NAeff = 1.33 sin(θeff), where n = 1.33 is the refractive index for water. θeff = 180° describes a perfect objective (all light collected), and is compared with an analytical calculation in Appendix 2—figure 3 (see Appendix 2). θeff = 90° represents the theoretical maximum with current objective designs. The color key is the same for all graphs.
Appendix 1—figure 5
Effect of spherical aberration on NAeff.
Refractive index mismatch causes spherical aberration. (A) Effect of refractive index mismatch on the point spread function (PSF). When an oil-immersion objective is used to illuminate a sample in identical refractive index medium (n = 1.518), all rays converge upon a single focal point (dashed lines). When illuminating an aqueous sample, the rays are refracted (red), and the PSF is elongated along the optical axis. (B) Effect of refractive index mismatch on image quality. (top) Imaging an aqueous sample (n = 1.33) with an oil-immersion objective leads to a poorly focused image with loss of photons emitted at high angles to the optical axis, thus reducing the NAeff (refracted rays in red). (bottom) Imaging a sample in glycerol (n = 1.47) with the same objective leads to a more tightly focused image (refracted rays in green). If the objective is corrected for spherical aberrations introduced by aqueous samples, the opposite will be true – the PSF will be smaller for aqueous samples and a sample in glycerol will be poorly focused and have a low NAeff.
Appendix 1—figure 6
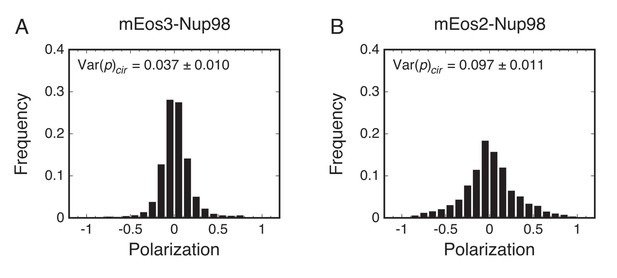
Reduced rotational mobility of the mEos2 probe.
p-PALM measurements were made on mEos3 (A) and mEos2 (B) attached to the N-terminus of Nup98. The rotational mobility of mEos2 was significantly lower, likely due to the tendency of mEos2 to dimerize (Hoi et al., 2010; McKinney et al., 2009).
Appendix 2—figure 1
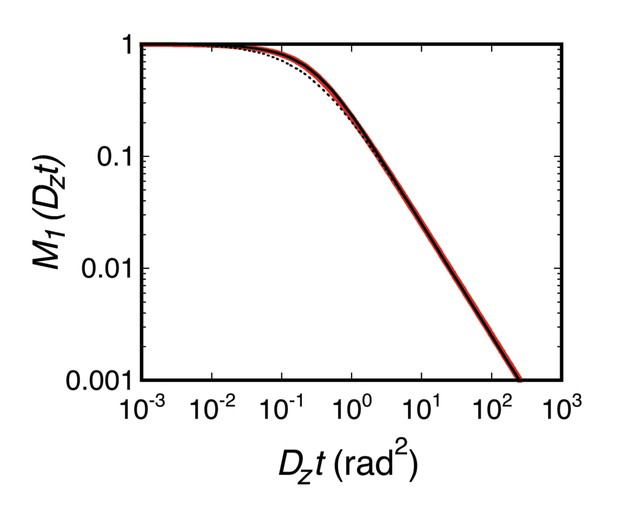
M1(Dzt).
Equation 48 was evaluated by numerical integration (red). The dashed black curve is Equation 49, and the solid black curve is Equation 50.
Appendix 2—figure 2
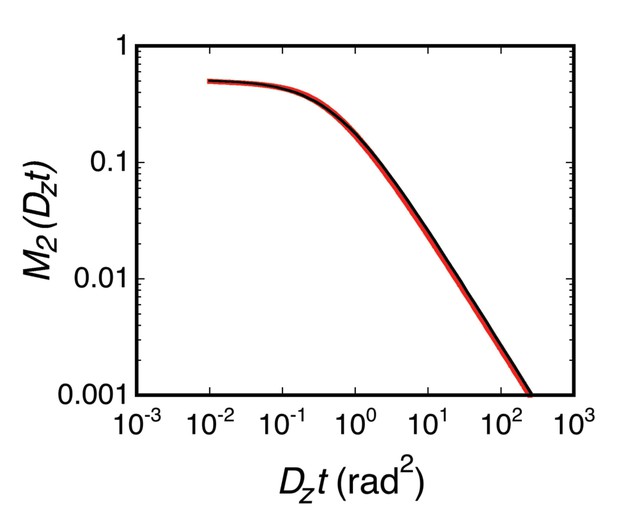
M2(Dzt).
Equation 55 was evaluated by numerical integration (red). The solid black curve is the best fit, given in Equation 56 as M2(Dzt) = <p2>(t)/H2 (see Equation 54).
Appendix 2—figure 3
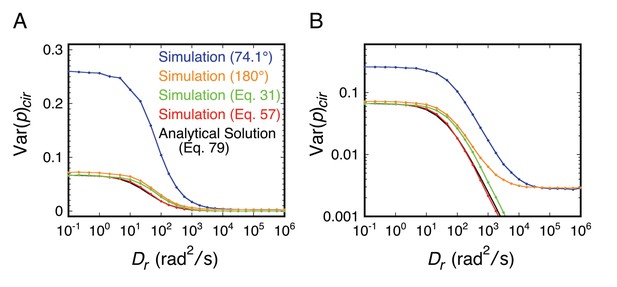
Comparison of the relationship between <p2>cir (=Var(p)cir) and Dr as determined analytically and from rotational random walk simulations for an isotropically rotating spherical particle.
Results are plotted on both linear (A) and log (B) ordinate scales. The red curve reproduces the black curve, providing confirmation of the simulation algorithm. As noted earlier, Equation 57 requires isotropic excitation and B = 1. The relatively small difference between the red and green curves indicates that the error introduced by reducing Equation 31 to Equation 57 is not very high. The blue and orange curves reach a minimum of ~0.003 at high Drt values due to the limited photons recovered from the random walk simulations (shot-noise limited). (black) Equation 79; (red) simulation algorithm using Equation 57 to calculate p; (green) simulation algorithm using Equation 31 to calculate p; (orange) rotational random walk simulation (no noise, no threshold) for a perfect objective (θobj = 180°); (blue) simulation data from Figure 2—figure supplement 1C (θobj = 74.1°). t = 10 ms; Ns = 1400; N = 10,000.
Videos
Video 1
p-PALM imaging of Pom121-mEos3 at the bottom of the nucleus.
The top half is the p-channel and the bottom half is the s-channel. The round illumination area created by the narrow-field epifluorescence imaging is clearly detectable within the center of the fields. Fluorescent spots that appear and disappear arise from single mEos3 molecules and are clearly correlated between the two channels. t = 10 ms; 240 nm square pixels (see Figure 1).
Video 2
PALM imaging of RanGAP-mEos3 at the nuclear equator.
Imaging conditions were the same as for p-PALM, except that there was no polarizer and only a single image was collected per time interval. Fluorescent spots that appear and disappear arise from single mEos3 molecules. t = 10 ms; 240 nm square pixels (see Figure 7A).
Additional files
-
Supplementary file 1
Table S1: <p>cir and <p2>cir Values, Table S2: <p>lin and Var(p)lin Values.
- https://doi.org/10.7554/eLife.28716.033
-
Transparent reporting form
- https://doi.org/10.7554/eLife.28716.034
Download links
A two-part list of links to download the article, or parts of the article, in various formats.
Downloads (link to download the article as PDF)
Open citations (links to open the citations from this article in various online reference manager services)
Cite this article (links to download the citations from this article in formats compatible with various reference manager tools)
Investigating molecular crowding within nuclear pores using polarization-PALM
eLife 6:e28716.
https://doi.org/10.7554/eLife.28716
