Statistical context dictates the relationship between feedback-related EEG signals and learning
- Brown University, United States
- Freie Universität Berlin, Germany
- Max Planck Institute for Human Development, Germany
- International Max Planck Research School on the Life Course (LIFE), Germany
Figures
Figure 1
Measuring learning in different statistical contexts with a predictive inference task.
(A) Participants were trained to place the center of a shield (green tick; prediction phase [left]) at the aim location of a cannon (training task [top]) in order to block a cannonball shot from it …
Figure 2 with 1 supplement
Participants scale learning according to surprise differently in changepoint and oddball contexts as would be expected for normative learning rate adjustment.
(A) In the changepoint condition, surprising events (changepoints) signaled a transition in the aim of the cannon whereas (B) in the oddball condition, surprising events (oddballs) were unrelated to …
Figure 2—figure supplement 1
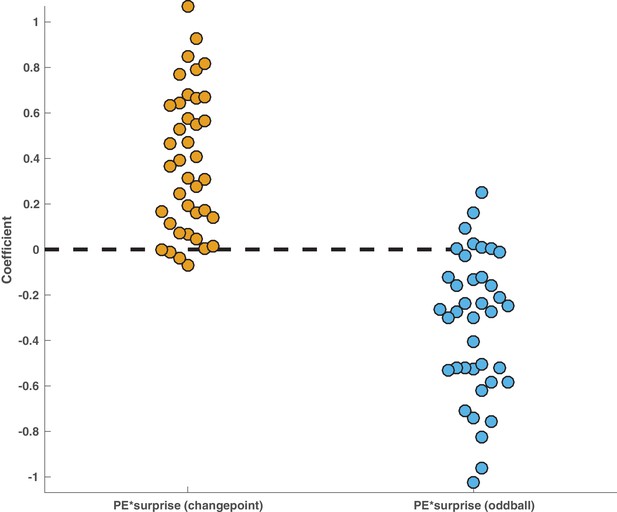
Behavioral interaction was driven by positive effects of surprise on learning in the changepoint condition and negative effects of surprise on learning in the oddball condition.
Model described in Figure 2E was altered such that PE*Surprise and PE*Surprise*Condition terms were replaced with two terms that separately modeled the effect of surprise on updating behavior in the …
Figure 3 with 2 supplements
Outcome-locked central positivity reflects surprise irrespective of context.
(A) Trial-series of EEG data for a given electrode and timepoint was regressed onto an explanatory matrix that contained separate binary regressors for changepoint and oddball trials (left). A …
Figure 3—figure supplement 1
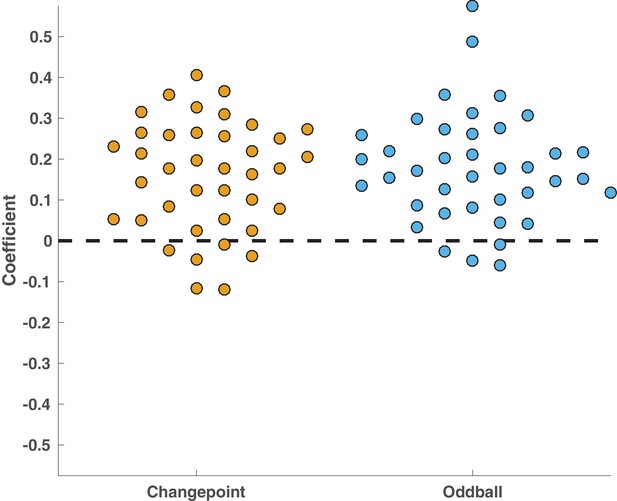
P300 spatiotemporal cluster reflected surprise in both changepoint and oddball conditions.
Raw regression coefficients computed per subject (points) averaged across electrode/timepoints for P300 component. Within these clusters, both changepoint (yellow) and oddball (blue) coefficients …
Figure 3—figure supplement 2
The difference between changepoint and oddball events was not reflected by any event related EEG signal.
(A) Trial-series of EEG data for a given electrode and timepoint was regressed onto an explanatory matrix that contained separate binary regressors for changepoint and oddball trials (left). A …
Figure 4 with 1 supplement
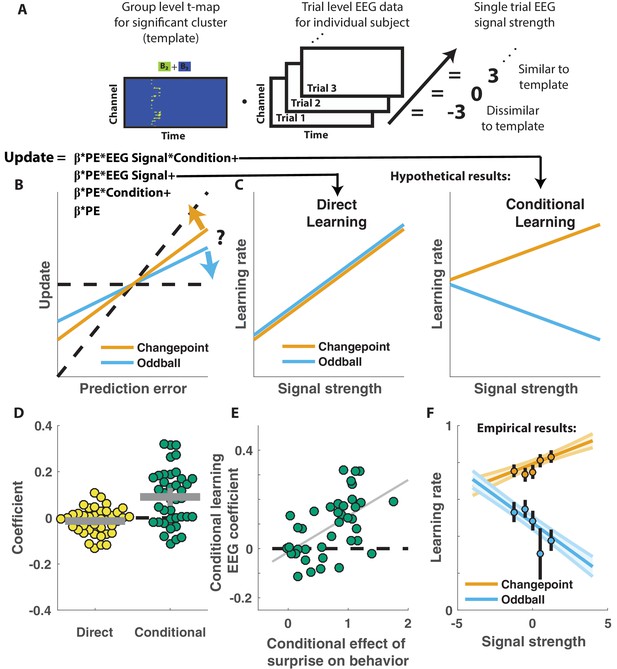
Central positivity predicts learning in opposite directions for changepoint and oddball contexts.
(A) T-maps corresponding to significant spatiotemporal clusters were used as templates to estimate trial-by-trial signal strength. (B) Single trial updates for each participant were fit with a …
Figure 4—figure supplement 1
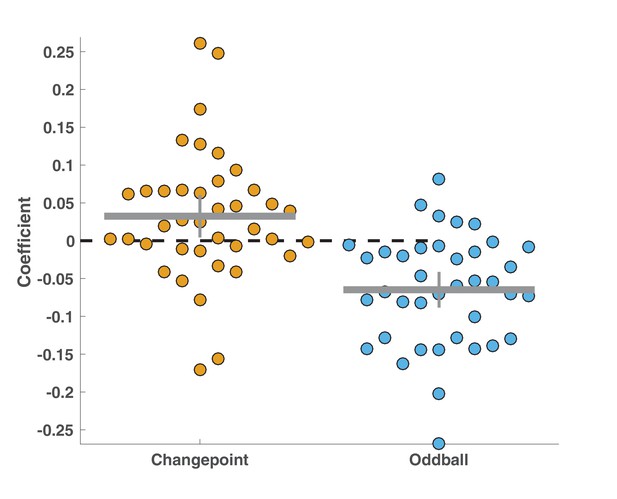
Conditional learning effect was driven by positive effects of EEG signals on learning in the changepoint condition and negative effects of EEG signals on learning in the oddball condition.
Points reflect best-fitting coefficients for individual subjects in an alternative model of update behavior that included separate PE*EEG signal terms for the two different task conditions. Bar and …
Figure 5
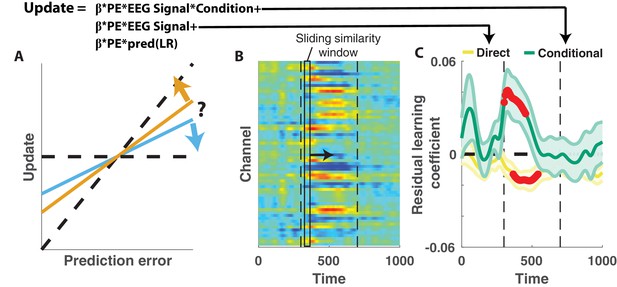
Central positivity explains trial-to-trial learning behavior that could not be otherwise captured through behavioral modeling.
(A) Single trial updates for each participant were fit with a regression model that included the best estimates of learning rate provided by our behavioral regression model (β times PE times …
Appendix 1—figure 1
Graphical generative model for changepoint (left) and oddball (right) task conditions.
Subscripts denote time, colored arrows depict the causal influence of an unlikely event (oddball or changepoint) on current and future outcomes. Note that changepoints (left) affect both current and …
Appendix 1—figure 2
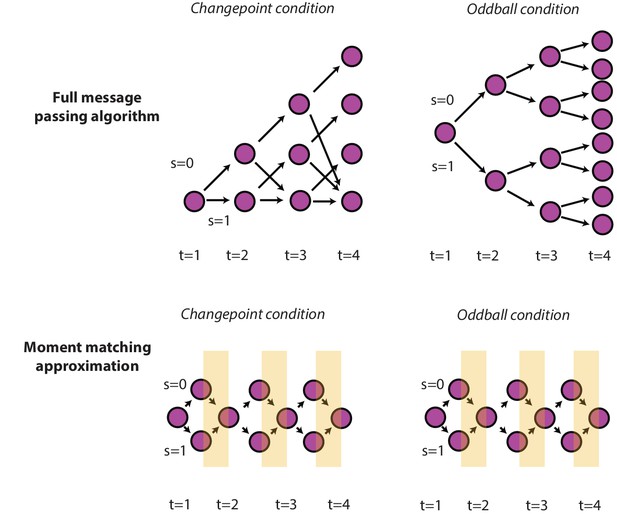
Optimal and approximate inference through message passing algorithms.
Exact parametric solutions to inference in the changepoint (left) and oddball (right) are possible for a given event history (e.g., S is known at all timesteps). Exact solutions can be approximated …
Author response image 1
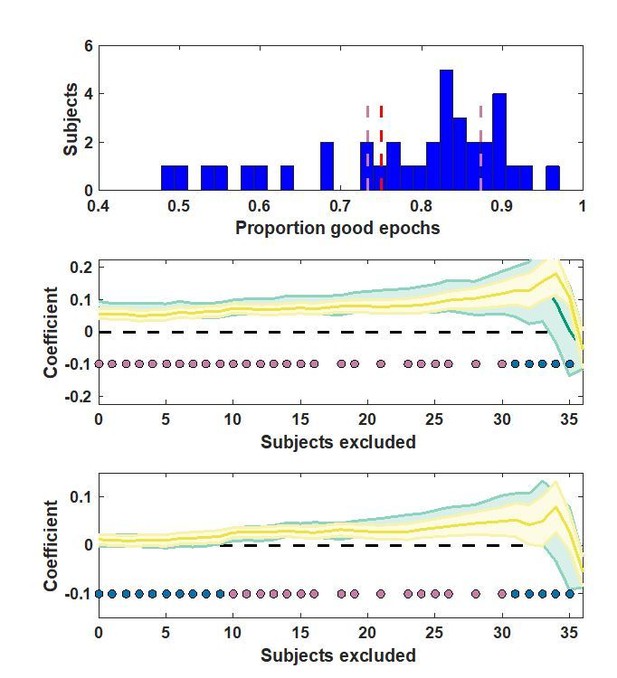
Robustness check on exclusion crierion value.
Top: proportion of good epochs for each participant. Middle/bottom: analysis results for different exclusion criteria. Lines/shading reflect mean/SEM conditional learning coefficients in the base …
Additional files
-
Transparent reporting form
- https://doi.org/10.7554/eLife.46975.011
