Intrinsic mechanisms in the gating of resurgent Na+ currents
Abstract
The resurgent component of the voltage-gated sodium current (INaR) is a depolarizing conductance, revealed on membrane hyperpolarizations following brief depolarizing voltage steps, which has been shown to contribute to regulating the firing properties of numerous neuronal cell types throughout the central and peripheral nervous systems. Although mediated by the same voltage-gated sodium (Nav) channels that underlie the transient and persistent Nav current components, the gating mechanisms that contribute to the generation of INaR remain unclear. Here, we characterized Nav currents in mouse cerebellar Purkinje neurons, and used tailored voltage-clamp protocols to define how the voltage and the duration of the initial membrane depolarization affect the amplitudes and kinetics of INaR. Using the acquired voltage-clamp data, we developed a novel Markov kinetic state model with parallel (fast and slow) inactivation pathways and, we show that this model reproduces the properties of the resurgent, as well as the transient and persistent, Nav currents recorded in (mouse) cerebellar Purkinje neurons. Based on the acquired experimental data and the simulations, we propose that resurgent Na+ influx occurs as a result of fast inactivating Nav channels transitioning into an open/conducting state on membrane hyperpolarization, and that the decay of INaR reflects the slow accumulation of recovered/opened Nav channels into a second, alternative and more slowly populated, inactivated state. Additional simulations reveal that extrinsic factors that affect the kinetics of fast or slow Nav channel inactivation and/or impact the relative distribution of Nav channels in the fast- and slow-inactivated states, such as the accessory Navβ4 channel subunit, can modulate the amplitude of INaR.
Editor's evaluation
After more than 20 years of intensive research the molecular machinery of Resurgent Currents (INaR), a non-canonical identity of currents mediated by voltage-activated sodium channels is still a mystery. In this paper, Ransdell and colleagues advance the conceptual framework with new experimental insight and a new kinetic model of INaR.
https://doi.org/10.7554/eLife.70173.sa0Introduction
Voltage-gated sodium (Nav) channels open rapidly on membrane depolarization and underlie the generation of action potentials in many excitable cells, including skeletal and cardiac muscle, as well as central and peripheral neurons. The pore-forming (α) subunits of Nav channels, Nav1.1 to Nav1.9, belong to the ‘S4’ superfamily of voltage-gated ion channel genes (Catterall, 2010). Each Nav α subunit comprises four homologous domains (DI–DIV) with six transmembrane spanning segments (S1–S6) (Noda et al., 1984). The S1–S4 segments in each domain form the four voltage sensing domains (VSDs) that activate on membrane depolarization (Noda et al., 1984; Guy and Seetharamulu, 1986), resulting in channel opening and Na+ influx. Nav channels conduct Na+ when the VSDs of domains I, II, and III move outwardly to an activated conformation (Bezanilla, 2000). Following opening, fast inactivation occurs (Bezanilla and Armstrong, 1977), mediated by a hydrophobic (IFM) motif in the cytosolic DIII–DIV linker that binds to a site near the pore that is revealed on activation of the DIII and DIV VSDs (Bosmans et al., 2008; Capes et al., 2013; Hsu et al., 2017; Cha et al., 1999). Thus, fast inactivation of open Nav channels occurs when all four VSDs are in the outward/activated position (Capes et al., 2013). If domains I, II, and III are activated and DIV is in the deactivated position, however, the IFM motif does not bind, which results in the generation of a non-inactivating or persistent Nav current (INaP) component (Chanda and Bezanilla, 2002; Horn et al., 2000).
An additional Nav current component, that is observed on membrane hyperpolarizations following brief depolarizing voltage steps and referred to as the resurgent component of Nav current (INaR), was first described in isolated, postnatal day 8–14 rat cerebellar Purkinje neurons (Raman and Bean, 1997). Although linked to the regulation of the spontaneous firing of action potentials in cerebellar neurons (Raman and Bean, 1997; Raman and Bean, 1999), INaR was subsequently identified in more than 20 types of neurons in the central and peripheral nervous systems, only some of which are spontaneously active (Lewis and Raman, 2014) suggesting that INaR likely plays diverse functional roles in regulating neuronal excitability. Although flowing through the same Nav channels as the transient (INaT) and persistent (INaP) sodium current components, the time- and voltage-dependent properties of INaR are distinct (Lewis and Raman, 2014). In addition to being revealed on membrane hyperpolarizations presented after brief (~5 ms) depolarizing voltage steps that evoke INaT (Khaliq et al., 2003), for example, the time courses of INaR activation and decay are much slower than INaT. These experimental observations were interpreted as suggesting a Nav channel gating model with two distinct mechanisms contributing to inactivation: a conventional, fast inactivation mechanism in which channels recover from inactivation without passing through an open conducting state; and, a second mechanism, favored by brief depolarizations, in which channels recover from inactivation by passing through an open, conducting state (Raman and Bean, 2001). It was further suggested that the second mechanism was consistent with a voltage-dependent process whereby Nav channels, opened on depolarization, are blocked by an endogenous ‘blocking’ particle that occludes the pore, driving channels into an ‘open-blocked’ (OB) state (Raman and Bean, 2001). On subsequent membrane hyperpolarization, the blocking particle is displaced, and Na+ flows through unblocked/open Nav channels, generating the resurgent Nav current (Raman and Bean, 2001). Importantly, in this gating scheme, blocked Nav channels do not inactivate and inactivated channels are not blocked, that is, a Nav channel cannot be blocked and inactivated simultaneously (Lewis and Raman, 2014).
Studies focused on defining the molecular mechanism(s) underlying the generation of INaR revealed that proteases (e.g., trypsin/chymotrypsin) that act at positively charged and aromatic/hydrophobic amino acid residues eliminate INaR, while increasing INaT, observations interpreted as supporting the blocking particle model and suggesting that the putative blocker was a protein within the Nav channel complex (Grieco et al., 2002). Attention focused quickly on the transmembrane accessory Navβ4 subunit, which has a short cytosolic tail with several positive charges and multiple aromatic/hydrophobic residues (Grieco et al., 2005). Clear support for a role for Navβ4 was provided in experiments in which intracellular application of a synthetic Navβ4 peptide containing the tail sequence (β4154–167), following elimination of the resurgent Nav current by trypsin or chymotrypsin, rescued INaR in isolated cerebellar Purkinje neurons (Grieco et al., 2005). In addition, experiments on CA3 pyramidal neurons, which lack INaR and Navβ4, demonstrated that the application of the β4154–167 peptide generated resurgent Nav currents (Grieco et al., 2005). Further support for a critical role for Navβ4 was provided with the demonstration that treatment of mouse cerebellar granule neurons with small interfering RNAs (siRNAs) targeting Scn4b (Navβ4) resulted in the loss of INaR and that the subsequent exposure of Scn4b-siRNA-treated cells to the β4154–167 peptide rescued INaR (Grieco et al., 2005; Bant and Raman, 2010).
In experiments designed to test directly the hypothesis that Navβ4 is required for the generation of INaR, however, we found that INaR was reduced (by ~50%), but not eliminated, and that the time- and voltage-dependent properties of the currents were unaffected in cerebellar Purkinje neurons in (Scn4b-/-) mice lacking Navβ4 (Ransdell et al., 2017). It was subsequently reported that INaR densities in cerebellar Purkinje neurons isolated from another Scn4b-/- mouse line were not significantly different from the currents measured in wild type cells (White et al., 2019). In addition, it has been reported that INaR is readily measured in Scn4b-/- striatal neurons (Miyazaki et al., 2014). These observations clearly suggest that additional mechanisms contribute to the generation of INaR. One possibility is that there are other open channel blocking molecules expressed in Purkinje (and other) neurons. Support for this hypothesis was provided in studies showing that INaR is decreased in neonatal mouse cerebellar Purkinje neurons isolated from animals harboring a targeted disruption in the Fgf14 (which encodes intracellular fibroblast growth factor 14 [iFGF14]) locus (White et al., 2019), as well as in wild type neonatal Purkinje cells following exposure to an interfering RNA targeting the Fgf14b variant (Yan et al., 2014). Interestingly, however, it was also reported that INaR was readily detected in Purkinje neurons isolated from neonatal animals lacking both Scn4b and Fgf14 (White et al., 2019). It is certainly possible and that there are additional, yet to be discovered, endogenous open channel blockers that contribute to the generation of INaR. Alternatively, it seemed possible to us that there is an intrinsic gating mechanism(s) by which Nav channels can produce resurgent current. Here, we present the results of experimental and modeling efforts designed to explore the latter hypothesis directly, and we provide evidence for a ‘blocking-particle independent’ mechanism in the generation of INaR in cerebellar Purkinje neurons.
Results
The amplitude of INaR depends on the duration, but not the voltage, of the prior membrane depolarization
In isolated neonatal (P12–P16) mouse cerebellar Purkinje neurons, the fast transient (INaT), persistent (INaP), and resurgent (INaR) Nav current components can be distinguished using voltage-clamp protocols that take advantage of the unique time- and voltage-dependent properties of the three current components (Figure 1A). On membrane depolarization, for example, INaT activates fast and subsequently decays rapidly to a steady-state (persistent) level of inward current, INaP (Figure 1A). INaR , in contrast, is revealed on membrane hyperpolarizations from the depolarized membrane potentials that evoke INaT (Figure 1A). In addition, the time courses of INaR activation and decay are much slower than INaT activation and decay (Figure 1A). Additional experiments revealed that, in response to membrane hyperpolarizations following brief (5 ms) depolarizing steps (to 0 mV), the amplitude of INaR varies as a function of the membrane potential of the hyperpolarizing voltage step (Figure 1B). The maximal amplitude of INaR is observed at approximately –45 mV (Figure 1C).
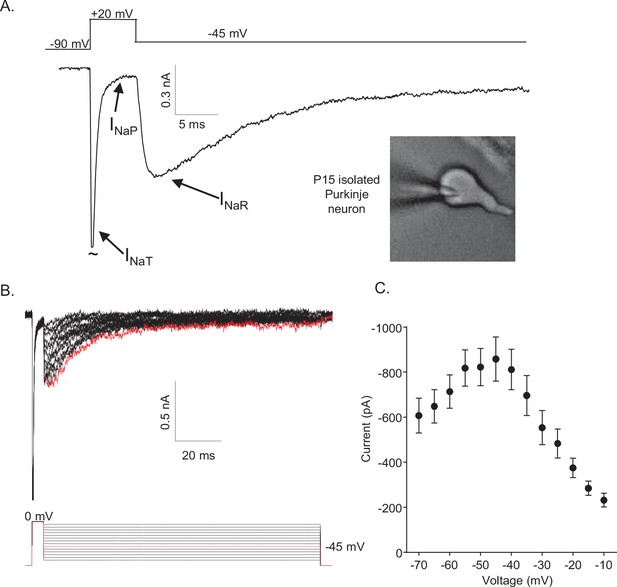
Mouse cerebellar Purkinje neurons express three voltage-gated sodium (Nav) current components.
(A) Representative recording of the transient (INaT), persistent (INaP), and resurgent (INaR) components of the Nav currents in an isolated neonatal mouse cerebellar Purkinje neuron. The voltage-clamp paradigm is displayed above the current record, and the labelled arrows indicate the three Nav current components. (B) INaR waveforms, recorded during hyperpolarizing voltage steps to various potentials ranging from –70 to –10 mV, following 5 ms depolarizing voltage steps to 0 mV from a holding potential (HP) of –80 mV; the voltage-clamp paradigm is shown below the current records. The current record highlighted in red was recorded during the –45 mV hyperpolarizing voltage step (also indicated in red in the illustrated voltage-clamp paradigm). (C) Mean ± SEM (n = 15) peak INaR amplitudes are plotted as a function of the hyperpolarizing test potential; the peak INaR is recorded at approximately –45 mV.
To determine how the duration and the voltage of the depolarizing voltage step (that evokes INaT) affect the amplitudes and waveforms of INaR, voltage-clamp paradigms were designed in which either the duration or the voltage of the depolarizing step was varied (Figure 2). In initial experiments, the external and internal Na+ concentrations were 151 and 8 mM, respectively, resulting in a Na+ reversal potential of +75 mV. These experiments revealed that prolonging the duration of the +20 mV depolarizing voltage step resulted in the marked attenuation of the peak amplitudes of INaR measured during hyperpolarizing voltage steps to –45 mV (Figure 2A). The time course of the attenuation of INaR was well described by a single exponential characterized by a mean ± SEM (n = 12) time constant of 15.5 ± 0.5 ms (Figure 2B).
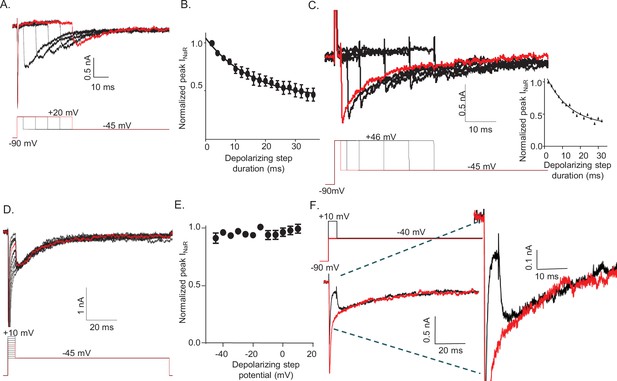
The amplitude of resurgent voltage-gated sodium current (INaR) is determined by the duration of the prior membrane depolarization.
(A) In a neonatal mouse cerebellar Purkinje neuron, INaR was revealed on membrane hyperpolarizations following depolarizing voltage steps to +20 mV of varying durations; the voltage-clamp paradigm is shown below the current records. (B) Peak INaR amplitudes, evoked at –45 mV following each depolarizing voltage step to +20 mV, were measured and normalized to the maximal peak INaR (measured in the same cell). The mean ± SEM (n = 12) normalized peak INaR amplitudes are plotted as a function of the duration of the depolarizing voltage step. The attenuation of peak INaR as a function of the duration of the depolarizing voltage step was well described by a single exponential with a mean ± SEM time constant of 15.5 ± 0.5 ms (n = 12). (C) The dependence of INaR on the duration of the depolarizing voltage step was also measured with reduced (50 mM) extracellular and increased intracellular (15 mM) sodium, resulting in a Na+ reversal potential of +30 mV. Under these conditions, depolarizing voltage steps to +46 mV evoked outward INaT. Peak INaR amplitudes, revealed during hyperpolarizing voltage steps to –45 mV, however, were also found to vary as a function of the duration of the depolarizing voltage step, revealing that INaR and the time-dependent attenuation of INaR are not affected by the direction (inward versus outward) of Na+ flux during the depolarizing voltage step. The peak amplitudes of INaR, evoked at –45 mV following each depolarizing voltage step, were measured in each cell and normalized to the maximal INaR amplitude (in the same cell). As is evident from the representative records and the plot of normalized peak INaR amplitudes (on the right), the attenuation of INaR as a function of the duration of the depolarizing voltage steps to +46 mV is also well described by a single exponential with a mean ± SEM time constant of 13.8 ± 1.1 ms (n = 6), a value similar to that observed when INaT was inward (B). (D) Representative INaR waveforms, recorded directly on repolarizations to –45 mv following 5 ms depolarizations to various membrane potentials from a –90 mV HP, are shown; the voltage-clamp protocol is shown below the current records. (E) The mean ± SEM (n = 6) normalized peak INaR amplitudes are plotted as a function of potential of the depolarizing voltage step. (F) Representative voltage-clamp recordings of Nav currents evoked (in the same cell) on direct depolarization to –40 mV from an HP of –90 mV (red) and on hyperpolarization to –40 mV following a 5 ms depolarizing voltage step to +10 mV (black) from the same HP; the voltage-clamp protocols are shown above the current records and the currents are shown on an expanded scale on the right. In panels A, C, D, and F, the currents in red were recorded during the voltage-clamp paradigms (shown below or above) depicted in red.
Subsequent experiments explored the effect of the driving force on Na+ on the time-dependent attenuation of INaR. In these experiments, the extracellular Na+ was reduced to 50 mM and the Na+ concentration in the internal solution was increased to 15 mM, resulting in a Na+ reversal potential of approximately +30 mV. Under these recording conditions, depolarizations to +46 mV resulted in outward INaT (Figure 2C). Similar to the results obtained with inward INaT (Figure 2A and B), prolonging the depolarizing (+46 mV) voltage step when INaT is outward results in the rapid attenuation of the amplitudes of INaR evoked during the subsequent hyperpolarizations to –45 mV (Figure 2C). Under these conditions (outward INaT), the time course of the attenuation of INaR was also well described by a single exponential with a mean ± SEM (n = 6) time constant of 13.8 ± 1.1 ms (Figure 2C), a value that is very similar to that observed when INaT is inward (Figure 2B). Taken together, these combined results demonstrate that, following brief depolarizations, there is a time-dependent accumulation of Nav channels (which underlie INaR) in a non-conducting state, and that this accumulation occurs independent of the direction of the movement of permeating Na+ ions.
To determine how the voltage of the depolarizing step affects the amplitudes and kinetics of INaR, the currents recorded at –45 mV after 5 ms depolarizing steps to various membrane potentials (Figure 2D) were measured. These experiments revealed that hyperpolarizations to –45 mV following brief (5 ms) depolarizations to various membrane potentials (ranging from –45 to +10 mV) resulted in identical INaR amplitudes (Figure 2E). Additionally, varying the voltage of the depolarizing step did not affect the kinetics of the decay of INaR (Figure 2D). This is clearly illustrated in Figure 2F, in which Nav currents recorded (in the same cell) at –40 mV during a sustained voltage step (red) and following a 5 ms depolarizing voltage step to +10 mV are superimposed. The opening of Nav channels that conduct INaR, therefore, is not affected by the voltage of the prior membrane depolarization. Taken together, these observations suggest that there are two parallel, and kinetically distinct, Nav channel inactivation pathways: a fast inactivating pathway that is responsible for INaT; and, a second, slower inactivation pathway that underlies INaR.
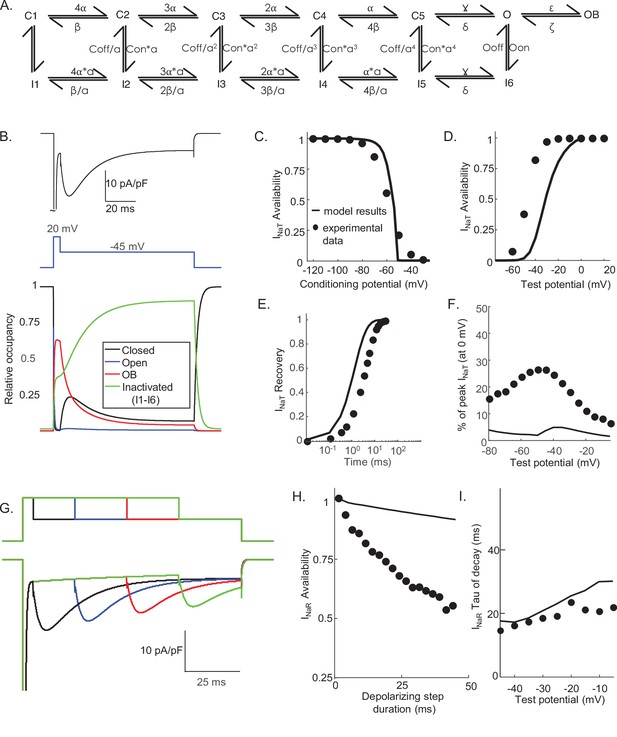
A novel Markov model, with parallel inactivation pathways, for Nav channel gating in Purkinje neurons
The results of the voltage-clamp experiments described above suggest that there are (at least) two distinct inactivation pathways that contribute to the gating of the Nav channels expressed in mouse cerebellar Purkinje neurons, that is, one that is populated quickly on channel opening and inactivates rapidly (fast inactivation), and a second that is populated and decays much more slowly (slow inactivation). To explore this hypothesis, we developed a Markov kinetic state model that, after numerical optimization (see Materials and methods), recapitulates the range of time- and voltage-dependent properties observed experimentally for the Nav currents in mouse cerebellar Purkinje neurons. The optimized Markov model (Figure 3A) includes parallel fast (IF1, IF2) and slow inactivation (IS) pathways to reconcile the experimental findings (Figure 2) that the duration of the depolarizing voltage step that underlie Nav channel activation, and not the potential of the depolarizing voltage step or the direction (i.e., inward or outward) of the Na+ flux through open channels, determines the amplitudes of INaR recorded during subsequent membrane hyperpolarizations. The model was constrained to fit the experimental data derived from multiple voltage-clamp protocols designed to detail the properties of INaT, including those to determine the voltage dependence of INaT activation and steady-state inactivation, the time course of INaT recovery from inactivation (Figure 3B–D), and the time constant (tau) of decay of the peak INaT. The model was further constrained by the experimental data obtained using protocols designed to detail the properties of INaR, including the voltage dependence of the ratio of the amplitudes of INaR and INaT (INaR:INaT), the time-dependent attenuation of INaR amplitudes, observed as a function of the duration of the depolarizing voltage step (Figure 2A and B), and the time constants (tau) of INaR decay determined for the currents recorded during hyperpolarizing voltage steps to various membrane potentials (Figure 3E–G). Consistent with the experimental results presented in Figure 2, simulations using this gating model reveal that the amplitude of INaR is dependent on the duration, but not on the potential, of the depolarizing voltage step that evokes INaT (Figure 3H,I).
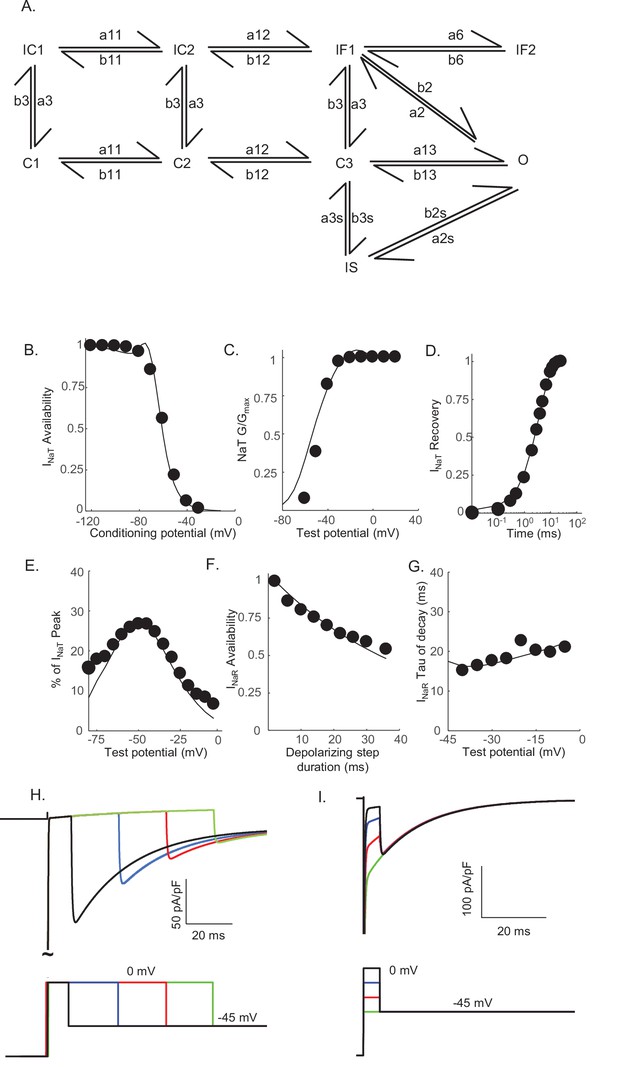
Novel Markov kinetic state model of voltage-gated sodium (Nav) channel gating in cerebellar Purkinje neurons.
A novel Markov kinetic state model was developed with parallel fast inactivating (IF1, IF2) and slow inactivating (IS) gating pathways (A). The model was numerically optimized (see Materials and methods) by simulating the data generated using voltage-clamp protocols identical to those used in the experiments to determine the detailed time- and voltage-dependent properties of the Nav currents in cerebellar Purkinje neurons. The various rate constants in the model were numerically optimized to recapitulate the measured properties of INaT, INaP, and INaR including the voltage dependences of steady-state inactivation (B) and activation (C) of INaT, and the kinetics of INaT recovery from inactivation (D). The model also reproduces the measured properties of INaR, including the magnitude of INaR relative to INaT (E), the attenuation of the peak INaR amplitude as a function of the duration of the depolarizing voltage steps (F), and the kinetics of the decay (inactivation) of peak INaR amplitudes (G). Filled circles represent the mean experimental data and the lines represent the results of the simulation. The model successfully reproduces the observed, time-dependent attenuation of peak INaR amplitudes that is evident experimentally on membrane hyperpolarizations following depolarizing voltage steps of varying durations (H), and the finding that the peak amplitude of INaR is not affected by the potential of the depolarizing voltage step (I).
One potential benefit of computational modeling is the ability to dissect out possible mechanisms of channel gating by examining the occupancy of the individual channel states as a function of voltage and time. Taking advantage of this benefit, we examined the proportion of Nav channels populating each gating state during a simulated voltage-clamp protocol that evoked INaR at –45 mV after a 5 ms depolarizing voltage step to 0 mV (Figure 4). As illustrated, fast inactivation of INaT (during the 0 mV step) reflects (simulated) channels exiting the open state and accumulating into the IF1/IF2 states. The activation of INaR (at –45 mV) reflects channel transitioning from IF1/IF2 back into the open state, and the decay of INaR during the –45 mV step reflects the time-dependent accumulation of (simulated) Nav channels in the secondary, slow-inactivated state, IS (see Figures 3A and 4A). The distinct pathways of inactivation are separated in time, but are not distinguished by differing voltage dependences. Additionally, Figure 4—figure supplement 1 shows that simulated channels follow a similar inactivation pathway during a sustained depolarization to 0 mV, with an initial accumulation in the IF1/IF2 states and subsequent accumulation in the IS state, a property of the model that reveals why INaR amplitudes are reduced as the duration of the depolarizing voltage step (that evokes INaT) is increased (Figure 3F and H).
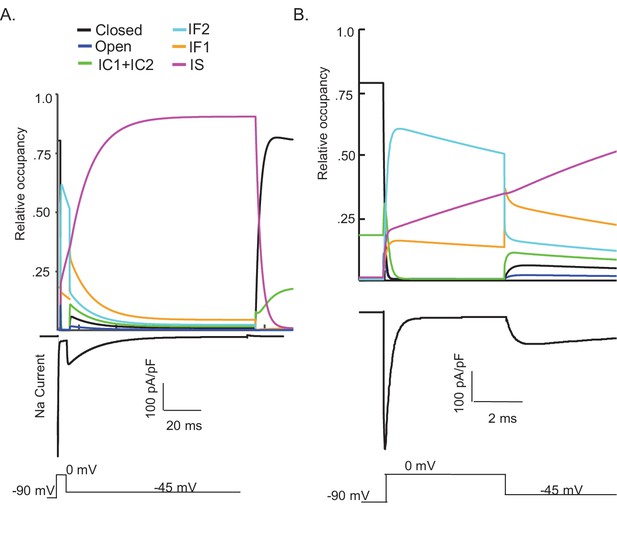
Kinetic state transitions during voltage-clamp simulations that evoke voltage-gated sodium current (INaR).
There are two parallel pathways of voltage-gated sodium (Nav) channel inactivation (IF1/IF2 and IS) in the novel Markov kinetic state model developed here (Figure 3). The occupancies of these states and of the other (i.e., closed, open, etc.) channel gating states during a simulated voltage-clamp protocol, in which INaR is revealed on membrane hyperpolarization to –45 mV following a brief (5 ms) depolarizing voltage step to 0 mV from a holding potential of –90 mV, are plotted as a function of time in (A). The simulated voltage-clamp records and the experimental paradigm are illustrated below the gating state occupancy plot. Expanded (in time) views of the gating state occupancies and the simulated Nav currents are presented in (B). In the gating state occupancy plots, black represents the closed state, blue represents the open state, green represents the IC1+ IC2 states, aqua represents the IF2 state, orange represents the IF1 state, and purple represents the IS state.
INaT and INaR are differentially sensitive to entry into the slow-inactivated state
The simulations (Figure 4) indicate the fast decay of INaT and the much slower decay of INaR reflect separate, that is, fast and slow, pathways of Nav channel inactivation and, in addition, that the decay of INaR reflects Nav channels accumulating in an absorbing, slow-inactivated state (i.e., the IS state in Figure 3), suggesting there may be discrete Nav channel populations that accumulate in the ‘IS’ state during prolonged depolarizations. To test this hypothesis directly, we measured peak INaT and INaR amplitudes, recorded at 0 and –45 mV, respectively, during sequential voltage-clamp protocols separated by a brief (20 ms) interval at –90 mV; the paradigm is illustrated in Figure 5A below the current records. The 20 ms interval at –90 mV between the sequential protocols was determined to be sufficient for the near complete recovery of INaT from fast inactivation (Ransdell et al., 2017; Aman and Raman, 2007). However, if the channels that underlie INaR have accumulated in the second, slow-inactivated (IS) state during the first –45 mV voltage step and recovery from this state is also slow, one would expect to see differential effects on peak INaT and peak INaR amplitudes when the time interval at –90 mV is sufficient to allow complete recovery of INaT, but too short to allow the complete recovery of INaR. As illustrated in the representative records shown in Figure 5A, this voltage-clamp paradigm revealed that, when the time interval at –90 mV was reduced to 20 ms, the amplitude of INaR was indeed reduced to a greater extent than the amplitude of INaT. Recordings from five additional Purkinje neurons using this voltage-clamp paradigm yielded similar results. Plotting the relative peak amplitudes of INaT and INaR measured during the second protocol, compared with the first, reveals that the 20 ms hyperpolarizing voltage step to –90 mV was sufficient to provide nearly complete (0.95 ± 0.01; n = 6) recovery of INaT (Figure 5B), whereas there was a marked reduction in the amplitude of INaR (0.63 ± 0.05; n = 6), measured during the second, compared with the first, protocol (Figure 5B).
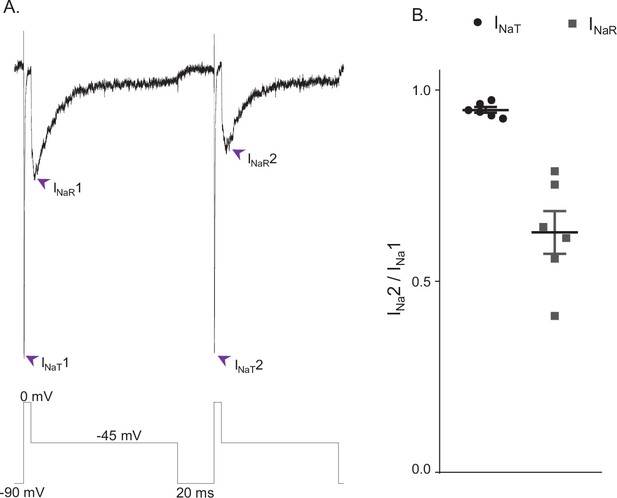
INaR and INaT display distinct rates of recovery from inactivation.
(A) Representative voltage-gated sodium (Nav) currents recorded in a mouse cerebellar Purkinje neuron during a voltage-clamp paradigm designed to determine if the relative rates of recovery from inactivation of INaT and INaR are distinct. Inward Nav currents were recorded during sequential and identical voltage-clamp steps (to 0 mV for 5 ms and to –45 mV for 100 ms), separated by a brief (20 ms) hyperpolarizing step to –90 mV; the voltage-clamp paradigm is shown below the current records. As is evident, the amplitude of INaR (at –45 mV) during the second voltage-clamp step to –45 mV was attenuated more than INaT (during the second step to 0 mV). Similar results were obtained in five additional Purkinje neurons using the voltage-clamp paradigm shown. (B) Plot of the relative peak INaT (circles) and peak INaR (squares) amplitudes measured during the second voltage-clamp steps (to 0 and –45 mV), compared with the first. As is evident, the relative amplitude of INaR is reduced (0.63 ± .05; n = 6) to a greater extent (paired Student’s t-test; p = .00039) than INaT (0.95 ± 0.01; n = 6). The mean ± SEM (n = 6) relative INaT and INaR amplitudes are also indicated.
INaR reflects the transitioning of fast-inactivated Nav channels into an open conducting state
The results presented in Figure 5 indicate that Nav channels open during membrane hyperpolarizations from depolarized potentials and that these (open) channels recover from inactivation at a rate that is distinct from the complement of Nav channels responsible for INaT. To determine the relationship between INaR and the channels that give rise to the persistent component of the sodium current, INaP, we used two voltage-clamp protocols, designed to allow direct measurements of INaP alone or INaP plus INaR. In the first protocol, a slow (dV/dt = 0.12 mV/ms) depolarizing voltage ramp (from –100 to 0 mV) was presented and inward currents, reflecting only INaP, were recorded (Figure 6A, blue). In the same cell, we also recorded Nav currents evoked during a slow (dV/dt = 0.12 mV/ms) hyperpolarizing (from 0 to –100 mV) voltage ramp (Figure 6A, red). In the latter case, the measured inward currents reflect both INaP and INaR, that is, channels capable of recovering from a fast-inactivated state into an open (conducting) state on membrane repolarization. It should be noted that INaR decays (see Figure 2B) during the hyperpolarizing voltage-ramp and that the relative amplitudes of INaR and INaP to the measured currents vary as function of time during the ramp. In addition, we recorded the currents evoked during depolarizing voltage steps to various test potentials between –75 and +10 mV from a holding potential of –100 mV, and we measured the amplitudes of INaP directly, at 25 ms after the onset of each depolarizing voltage step (Figure 6A, green). The current-voltage plots, derived from the data obtained in these experiments, are presented in Figure 6B; the colors correspond to those used to illustrate the current records presented in Figure 6A. As is evident, the voltage dependences and the magnitudes of the Nav currents recorded using the three voltage-clamp protocols (in the same cell) are indeed indistinguishable. Similar results were obtained in recordings from four additional Purkinje neurons (see Discussion).
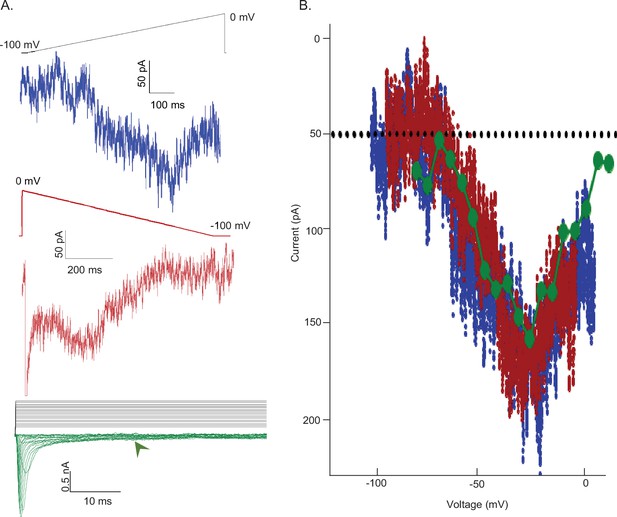
Voltage dependences of activation of INaR and INaP are indistinguishable.
(A) To test the hypothesis that non-inactivating voltage-gated sodium (Nav) channels underlie INaR, a depolarizing voltage ramp (blue) protocol (from –100 to 0 mV at 0.12 mV/ms) and a steady-state voltage step (green) protocol (with depolarizations from a holding potential of –100 mV to test potentials ranging from –75 to 10 mV in 5 mV increments) were used to reveal the magnitude and voltage-dependent properties of the non-inactivating (persistent) component of the Nav current, INaP. In addition, a hyperpolarizing voltage ramp (from 0 to –100 mV at 0.12 mV/ms or dV/dt) was used to reveal both INaR and INaP. Note that as INaR decays (see Figure 2B) during the hyperpolarizing voltage-ramp, the relative amplitudes of INaR and INaP vary during the ramp; the sum of the two current components, not the amplitudes of the individual components, therefore, are measured. The three representative records shown were obtained from the same Purkinje neuron. (B) The current-voltage relationships, derived from the records presented in (A) are plotted (in the corresponding color). From the records shown in the lowest panel of (A), the amplitudes of the steady-state inward currents at 25 ms at each test potential are plotted as points (green); the current amplitudes determined (at 2 ms intervals) from the ramp protocols (red and blue traces) are also plotted. As is evident, the current-voltage relations of the Nav currents recorded using the three different voltage-clamp protocols overlap; the magnitudes of the inward Nav currents are also indistinguishable. Similar results were obtained in four additional Purkinje neurons.
Nav channel gating model with an OB state does not reproduce the voltage-clamp data
The novel Markov model developed here (Figure 3A) is quite different from the previously proposed model of Nav channel gating in mouse cerebellar Purkinje neurons (Raman and Bean, 2001). In this earlier model (illustrated in Figure 7A), there are two distinct competing pathways that depopulate the open state, one of which involves fast Nav channel inactivation and results in the population of the I6 state (Figure 7A) and the other, competing, pathway involves the blockade of open Nav channels and the generation of the OB state (Figure 7A). The isolation of the OB state from all of the other kinetic states except the open state is a distinctive feature in the model of Raman and Bean (Figure 7A). This configuration means that channels that have entered the OB state can only exit this state (i.e., become unblocked) by transitioning into the open/conducting state to generate resurgent Na+ influx, that is, INaR (Raman and Bean, 2001). Although it was suggested that the blocking particle responsible for producing the OB state was a protein, specifically the Nav channel accessory subunit Navβ4 (Grieco et al., 2002; Grieco et al., 2005; Bant and Raman, 2010), it was subsequently demonstrated that INaR is reduced, but is not eliminated, in cerebellar Purkinje neurons in (Scn4b-/-) mice lacking Navβ4 (Ransdell et al., 2017; White et al., 2019) (see Discussion).
Simulations using the Raman-Bean model of voltage-gated sodium (Nav) channel gating do not recapitulate the acquired voltage-clamp data.
(A) The previously described Markov kinetic state model of Nav channel gating in mouse cerebellar Purkinje neurons (Raman and Bean, 2001) is illustrated. (B) Representative simulated inward Nav current waveforms, produced by this (A) model using the voltage-clamp paradigm shown below the current records, are presented. The time-matched normalized occupancies of the combined closed (C1–C5, shown in black), open (O, shown in blue), open-blocked (OB, shown in red), and combined inactivated (I1–I6, shown in green) gating states are plotted below the voltage protocol. (C–E) Comparisons of the time- and voltage-dependent properties of INaT derived from simulations using the model in (A) with (our) experimental data obtained in recordings from mouse cerebellar Purkinje neurons (the same data as were used to generate the results in Figure 3); filled circles represent the mean experimental data and the lines represent the results of the simulations. (F) Relative INaR amplitudes (normalized to peak INaT at 0 mV) are plotted as a function of the hyperpolarizing test potential. (G) Simulated INaR waveforms, produced on membrane hyperpolarizations to –45 mV following depolarizing voltage steps to +20 mV of varying durations, are shown. (H) Peak normalized INaR amplitudes (at –45 mV), derived from the simulations in (G), are plotted as a function of the duration of the prior +20 mV depolarizing voltage step (solid line), together with the mean experimental data (filled circles) obtained in recordings from mouse cerebellar Purkinje neurons (the same data as used to generate the results in Figure 3). (I) The kinetics of INaR decay, derived from single exponential fits to the decay phases of the currents recorded at various membrane potentials, are presented in (I); the solid lines indicate the results of the simulations, and the filled circles are the mean experimental data obtained in recordings from mouse cerebellar Purkinje neurons (the same data as used to generate the results in Figure 3).
Subsequent efforts here were focused on determining directly whether the Nav channel gating model in which INaR is generated by the OB mechanism (Figure 7A) could/would also reliably reproduce the detailed time- and voltage-dependent properties of the Nav currents determined experimentally in mouse cerebellar Purkinje neurons (and presented in Figures 1, 2, 5,, 6). As illustrated in Figure 7B, simulations with the OB model for INaR generation (Figure 7A) provided transient and resurgent Nav current components (Figure 7B, upper panel) that resemble those measured experimentally (Figure 1A). A time-locked plot of gating state occupancies with this model reveals that INaR activation occurs as simulated channels exit the OB state and into the open (conducting) state (Figure 7B, lower panel). Additional simulations revealed that this model also recapitulates the voltage and time dependences of INaT activation, inactivation and recovery from inactivation (Figure 7C–E) in mouse cerebellar Purkinje neurons, although the modeled INaT activates at more hyperpolarized voltages than native INaT. Although recapitulating the voltage dependence of INaR activation (Figure 7F), the model also predicts that the magnitude of INaR evoked at all hyperpolarized membrane potentials, relative to the peak amplitude of INaT (evoked at 0 mV), is much smaller than observed experimentally (Figure 7F). It should be noted, however, that the relative INaR amplitudes measured here were obtained in experiments conducted with a much higher (151 mM) extracellular Na+ concentration than the concentration (of 50 mM) used in earlier studies (Raman and Bean, 2001), which likely accounts for some (if not all) of the difference in the relative magnitudes of INaR measured here and previously (Raman and Bean, 2001).
In the open-channel block model of INaR gating (Figure 7A), more positive depolarizing voltage steps promote entry into the OB state, whereas channels are favored to undergo fast (conventional) inactivation at more hyperpolarized membrane potentials (Raman and Bean, 2001; Lewis and Raman, 2014). When the voltage-clamp protocols used to generate the data presented in Figure 2 were used in simulations with the open-channel block model (Figure 7A), however, substantial differences between the predictions of this model and our experimental data were revealed. In contrast to what is observed experimentally (Figure 2), for example, increasing the duration of the brief (5 ms) depolarizing voltage step (to 0 mV) in the open channel block model resulted in very little time-dependent attenuation of the amplitude of INaR recorded on membrane hyperpolarization to –45 mV (Figure 7G and H). In addition, the time course of the decay of the resurgent currents predicted by the open channel block model (Figure 7A) are slower than we observed experimentally for INaR in mouse cerebellar Purkinje neurons (Figure 7I).
The fast- and slow-inactivated Nav channel states are populated separately and at different rates
The experimental data and the simulations using the novel Nav channel gating model developed here (Figure 3A) suggest that INaR is mediated by Nav channels transiting from a fast-inactivated state (IF1) into the open state, and subsequently accumulating in an absorbing, slow-inactivated state (IS). It is also possible, however, that the absorbing slow-inactivated state that underlies INaR decay reflects Nav channels accumulating into a long-term inactivated state, that is, a state in which channels are non-conducting for hundreds of ms, for example, by a blocking particle(s) that competes on a time scale similar to conventional fast inactivation (Dover et al., 2010; Venkatesan et al., 2014). To test this possibility directly, a voltage-clamp protocol was developed to allow direct comparison in the same cell of INaR recorded during a single (80 ms) hyperpolarizing voltage step to –45 mV, presented following a brief (5 ms) depolarization to 0 mV, with INaR recorded at –45 mV during successive brief (2 ms) hyperpolarizing voltage steps interspersed with brief (5 ms) depolarizations to 0 mV (Figure 8A). If a competing extrinsic blocking particle has fast-onset, competes with conventional inactivation and is absorbing, one would expect to see reductions in the amplitudes of INaR recorded during each successive hyperpolarizing voltage step to –45 mV compared with INaR recorded during a sustained hyperpolarizing voltage step to –45 mV. As is evident in the experimental records shown in Figure 8A, however, INaR waveforms evoked (in the same cell) using these two voltage-clamp protocols were quite similar.
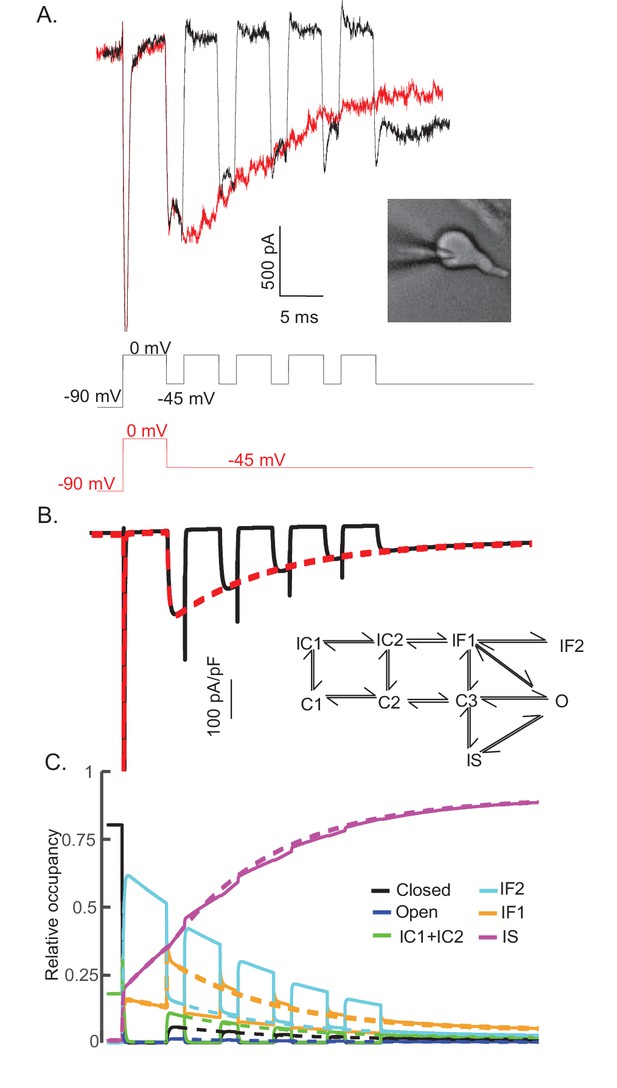
The time course and amplitude of INaR are recapitulated during repetitive brief depolarizing steps.
To determine if two competing inactivation states underlie the observed differences in INaT and INaR recovery from inactivation (illustrated in Figure 5), a protocol was developed to allow direct comparison of INaR recorded during a single (80 ms) hyperpolarizing voltage step to –45 mV (red), presented following a brief (5 ms) depolarization to 0 mV, with INaR recorded (in the same cell) at –45 mV during successive brief (2 ms) hyperpolarizing voltage steps interspersed with brief (5 ms) depolarizations to 0 mV (black). Representative records are shown in (A); the voltage-clamp paradigms are illustrated below the current records. Similar results were obtained in four additional Purkinje neurons. As is evident (A), the envelope of the currents generated using these two protocols superimpose, suggesting that the inactivation pathway responsible for INaR decay does not compete with fast inactivation. (B) Simulated current waveforms, generated using the same two voltage-clamp protocols illustrated in (A) with the novel kinetic state model presented in Figure 3A, are shown. (C) Gating state occupancies for simulated current traces are shown with black representing the closed state, blue representing the open state, green representing the IC1+ IC2 states, aqua representing the IF2 state, orange representing the IF1 state, and purple representing the IS state. For direct comparison of the results of the simulations using the voltage-clamp protocols illustrated in (A) with the Raman-Bean gating model (2001), see Figure 8—figure supplement 1.
The representative traces presented in Figure 8A were recapitulated in simulations (Figure 8B) using the novel gating state model (Figure 3A) developed here. The current waveforms generated by the model are indistinguishable from the experimental results (compare Figure 8A and B). In addition, and without tuning any of the model parameters, the kinetic state occupancy plots generated using the two voltage-clamp protocols were also very similar (Figure 8C). This voltage-clamp protocol was also applied in simulations using the previously described (Figure 7A) open channel block model (Raman and Bean, 2001). In this case, in marked contrast with the results presented in Figure 8, the model does not reproduce the experimental data (Figure 8—figure supplement 1A). The simulations revealed that, in this model, channels did not maximally enter into the OB state on depolarizations to 0 mV and the 2 ms hyperpolarizations were not sufficient to fully activate INaR (Figure 8—figure supplement 1B). Additionally, in this model, during each of the successive depolarizations (to 0 mV), transient Nav currents were revealed (Figure 8—figure supplement 1A), reflecting channels exiting the OB state on membrane hyperpolarization and re-entering the OB state on membrane depolarization.
Simulating INaR in Scn4b-/- cerebellar Purkinje neurons
We previously reported that the targeted deletion of Scn4b in mice results in a marked (~50%) reduction in INaR amplitudes in cerebellar Purkinje neurons (Figure 9A) without measurable effects on INaR kinetics or voltage dependence (Ransdell et al., 2017). In subsequent studies conducted using a different Scn4b-/- mouse line, it was reported that the targeted deletion of Scn4b had no effects on INaR kinetics, voltage dependences, or amplitudes (relative to INaT) (White et al., 2019; Ransdell et al., 2017). To explore the ability of the novel model of Nav gating, developed and presented here (Figure 3A), to scale the amplitude of INaR while leaving the time- and voltage-dependent properties of the currents unaffected, we optimized the parameters of the model (see Materials and methods) to reproduce the experimentally determined reduction in INaR amplitudes with the loss of Navβ4 (Ransdell et al., 2017; White et al., 2019). As illustrated in Figure 9B, although reduced in amplitude, the voltage dependence of INaR generated by the Scn4b-/- Nav channel gating model is very similar to wild type INaR. In Figure 9C, representative INaR waveforms generated by the gating models of wild type and Scn4b-/- Nav currents are superimposed; the time courses of wild type and Scn4b-/- INaR are indistinguishable. Time-locked with the wild type and Scn4b-/- Nav current traces are plots of the Nav channel gating state occupancies as a function of time (Figure 9D) in the wild type (solid lines) and the Scn4b-/- (dashed lines) INaR models. The gating state occupancy plots (Figure 9D) reveal that the attenuation of the amplitude of INaR in the Scn4b-/- model is the result of the reduced accumulation of channels in the IF2 state during the initial depolarization and increased occupancy in the IS state. Together, these data suggest that Navβ4 delays entry into the slow-inactivated state (IS), allowing for greater recovery from conventional inactivation and thus, larger INaR amplitudes (see Discussion).
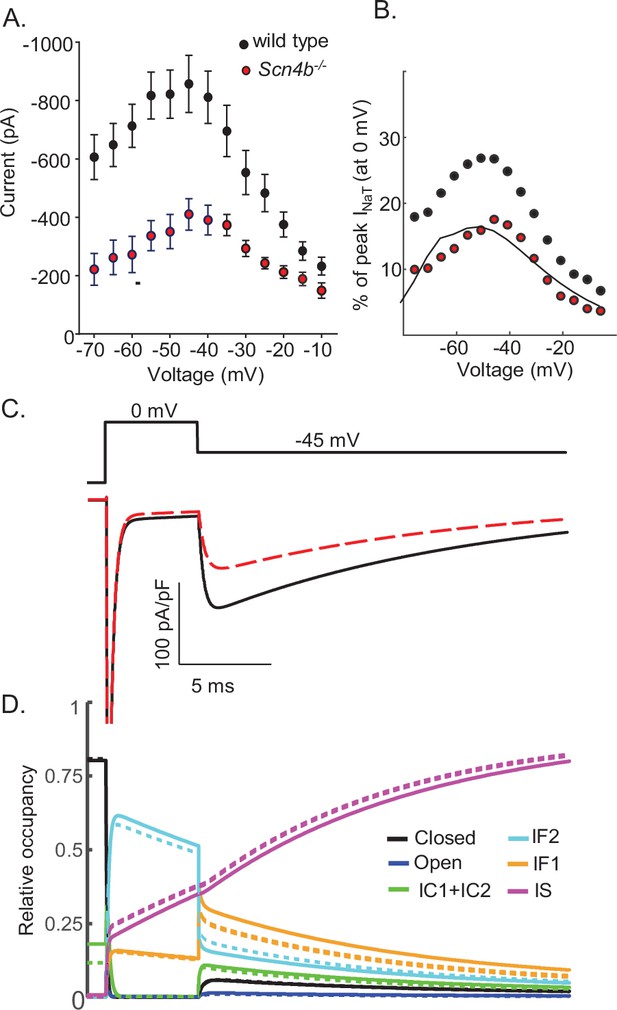
Promoting entry into the slow-inactivated state reduces voltage-gated sodium current (INaR) amplitudes.
(A) Mean ± SEM peak INaR amplitudes, measured on membrane hyperpolarizations following brief depolarizing voltage steps to +10 mV, in wild type (black) and Scn4b-/- (red) mouse cerebellar Purkinje neurons are plotted as a function of membrane voltage are shown (data were reproduced with permission from Ransdell et al., 2017). Peak INaR amplitudes in individual wild type and Scn4b-/- cells were also normalized to peak INaT measured (at 0 mV) in the same cell, and the mean INaR as a percentage of peak INaT in wild type (black) and Scn4b-/- (red) cells are plotted (as points) in (B); the solid line is the normalized relative INaR/INaT generated by the Scn4b-/- model. (C) Consistent with the experimental data, the kinetics of INaR are not affected measurably by the loss of Scn4b (Navβ4) in the model, whereas INaR amplitudes are reduced to ~50% of wild type INaR levels (C). A time-locked plot of the gating state transitions (D) indicates that INaR amplitudes are reduced in the Scn4b-/- model (dashed lines) due to a decrease in IF2 occupancy and an increase in IS occupancy. In this gating state occupancy plot, black represents the closed state, blue represents the open state, green represents the IC1+ IC2 states, aqua represents the IF2 state, orange represents the IF1 state, and purple represents the IS state.
Discussion
Using a combined experimental and modeling approach, we describe here a novel mechanism for INaR gating that reflects two kinetically distinct (fast and slow) pathways of Nav channel inactivation. Importantly, the model reliably recapitulates the detailed time- and voltage-dependent properties of INaR determined experimentally in mouse cerebellar Purkinje neurons. The model, for example, accounts for the experimental finding that the peak amplitude of INaR (recorded on membrane hyperpolarizations following brief depolarizations that evoke INaT) is sensitive to the duration of the preceding depolarizing voltage step, with longer depolarizations resulting in lower INaR amplitudes. Importantly, the dependence of INaR amplitudes on the duration of the depolarizing voltage step was also observed when the inward driving force of Na+ was reduced or eliminated. In addition, the model reproduces the experimental finding that INaR amplitudes are not dependent on the voltage of the initial depolarizing voltage step (that evokes INaT). Finally, and also consistent with the experimental data, the voltage dependence of INaR (revealed on membrane hyperpolarizations) mirrors the voltage dependence of INaP (see Figure 6). Interestingly, this last observation, that is, that the voltage dependence of INaP is identical to the voltage dependence of INaR, was previously reported (Kay et al., 1998).
The model developed here (Figure 3A) is distinct from a previously proposed model (Figure 7A) of Nav channel gating in mouse cerebellar Purkinje neurons (Raman and Bean, 2001) that involves two competing pathways out of the open state, that is, channels transitioning into either an OB state or an inactivated state. In this earlier model (Figure 7A) of INaR gating, a blocking particle occludes the Nav channel pore that is opened on membrane depolarization, functionally competing with conventional, fast inactivation, and, in addition, the OB state is isolated off the open/conducting state (Raman and Bean, 2001). In this model, entry into the OB state is promoted by membrane depolarizations to positive potentials and, on subsequent membrane hyperpolarization, the blocking particle is expelled by the Na+ driven through the unblocked Nav channel pore (Aman and Raman, 2010). In addition, the larger the driving force on Na+, the more rapidly the blocker is displaced (Aman and Raman, 2010). This process, while intrinsically voltage-independent, therefore, is tied to the driving force on Na+, consistent with the experimental observation that the peak amplitude of INaR is observed at relatively hyperpolarized (−45 to –30 mV) membrane potentials (Aman and Raman, 2010; Lewis and Raman, 2014). At more negative membrane potentials, closed state inactivation begins to dominate, and INaR is reduced (Raman and Bean, 2001; Lewis and Raman, 2014). The experimental results presented here, however, reveal that the peak amplitude of INaR is not affected by the voltage of the initial membrane depolarization (Figure 2D), but rather is affected by the duration of the prior depolarizing voltage step (Figure 2A and B). The finding that the magnitude of INaR is substantially reduced when the initial depolarizing voltage step is increased in duration was previously reported (Raman and Bean, 2001). In addition, experimental results presented here demonstrate that the time-dependent accumulation of Nav channels into the slow-inactivated state occurs independent of the direction of the movement of permeating Na+ ions.
Recovery from conventional Nav channel inactivation into an open/conducting state
The experiments and the simulations presented here suggest that INaR reflects the transitioning of Nav channels that have undergone conventional fast inactivation, into an open/conducting state on membrane hyperpolarizations. Consistent with the slow decay of INaR, the model includes two parallel, but distinct, inactivation pathways: the fast (i.e., IF1 and IF2) inactivation pathway and the slow (i.e., IS) inactivation pathway, which satisfies the key experimental finding that the amplitude of INaR is insensitive to the voltage of the initial depolarization (Figure 2). Although it was previously reported that the slower component of the biexponential decay of INaT (measured at –30 mV) in Purkinje neurons is identical to the decay rate of INaR (also measured at –30 mV), this observation was interpreted as suggesting that all Nav channels initially undergo open channel block (Raman and Bean, 2001). The results of the experiments here involving prolonged depolarizations (Figure 2A–C), however, are not consistent with this model. In the experiments here, we found that there is still a time-dependent attenuation of INaR amplitudes on membrane hyperpolarizations following depolarizations to positive membrane potentials, with little or no inward driving force on Na+. These findings are inconsistent with the open-channel block hypothesis, in which positive membrane potentials are thought to promote and stabilize open-channel block (Raman and Bean, 2001; Aman and Raman, 2010; Lewis and Raman, 2014).
One assumption usually made when considering the gating of Nav channels is that deactivation must occur prior to recovery from inactivation. Or, to state another way, Nav channels cannot exit the conventional inactivated state directly into an open or conducting state. This idea is based on voltage-clamp experiments conducted on CA1 hippocampal neurons (Kuo and Bean, 1994) and the squid giant axon (Armstrong, 2006), which revealed that recovery from inactivation occurs with a delay and is voltage-dependent. Indeed, if recovery from inactivation requires all four of the VSDs of domains I–IV to move into the deactivated position, the model presented here is not possible because channels that have undergone conventional, fast inactivation would not be able to move directly into an open/conducting state without first transiting through an intermediate closed state. However, if deactivation of VSD IV is sufficient to release the cytosolic DIII–DIV linker peptide from the channel pore, while VSD I, II, and III remain in the activated conformation, Nav channels could transit from an inactivated state directly into an open/conducting state. There are several reports suggesting that this is possible. Schiavon et al., 2012, for example, demonstrated that scorpion beta toxins induce INaR in heterologously (in HEK-293 cells) expressed Nav channels. These toxins were previously shown to cause a negative shift in the voltage dependence of DI-VSD, DII-VSD, and DIII-VSD activation by trapping the DII-VSD in the activated position after depolarizing prepulses (Cestèle et al., 1998; Schiavon et al., 2006). Thus, on repolarization, the channel recovers from inactivation, allowing resurgent Na+ influx.
Resurgent Nav currents have also been induced in heterologously expressed (in HEK-293 cells) Nav1.5-encoded channels following application of the classical type II pyrethroid, deltamethrin. Similar to our model for the generation of INaR, the deltamethrin-modified Nav1.5 channels were found to recover from inactivation prior to deactivation (Thull et al., 2020). In addition, it has also been reported (Cummins et al., 2004) that heterologously expressed (in HEK-293 cells) mutant Nav1.7-encoded channels have prolonged deactivation time constants at the hyperpolarized membrane potentials associated with the activation of INaR. These mutant Nav1.7 channels were also reported to display an increase in the relative amplitude of the non-inactivating Nav current (i.e., INaP). These two observations are clearly consistent with the INaR gating model proposed here in which Nav channels can recover from conventional, fast inactivation directly into an open/conducting state. It is, however, worth noting that in this report (Cummins et al., 2004), INaR was never measured directly, instead the membrane was repolarized prior to the completion of INaT inactivation. As a result, it is not possible to conclude, with certainty, that the mutant Nav1.7 channels were recovering from inactivation into an open/conducting state, or simply displaying prolonged deactivation at hyperpolarized membrane potentials.
In developing the model (Figure 3A), we found that two fast-inactivated states, that is, IF1 and IF2, were required to recapitulate the experimental data. It is certainly possible, however, that IF1 and IF2 are substrates of the same channel state. We also note here that, initially, the model was developed with two slow-inactivated states (IS, IS2) for symmetry. The simulations revealed, however, that the two slow-inactivated states were redundant and that we were able to fit the experimental data reliably with only one of these slow-inactivated states.
It is important to note that we appreciate that our model topology and rate constants, while reliably recapitulating the time- and voltage-dependent properties of the currents determined experimentally, are not necessarily ‘unique’ or potentially the ‘most simple’. Indeed, in using Markov kinetic state modeling, it is not possible to tell the uniqueness or the ambiguity of the model (both with regard to the parameters and the model topology). Following the approach of Menon et al., 2009, using a state mutating genetic algorithm to vary topologies in a Markov model, Mangold et al., 2021, recently reported the development of an algorithm to enumerate all possible model structures distinctly using rooted graph theory (e.g., all possible combinations of models, rooted around a single open state). These efforts revealed that there are many model structures and parameter sets that can adequately fit some experimental datasets.
Molecular determinants of INaR
Soon after the discovery of INaR, it was reported that the targeted deletion of Scn8a (which encodes the Nav1.6 α subunit) in mouse cerebellar Purkinje neurons resulted in a 90% reduction in the amplitude of INaR, suggesting that, of the α subunits (Nav1.1 and Nav1.6) expressed in Purkinje neurons (Vega-Saenz de Miera et al., 1997; Xiao et al., 2013), Nav channels formed by Nav1.6 are the major contributors to INaR (Raman et al., 1997). However, it was later reported that the targeted deletion of Scn1a (which encodes the Nav1.1 α subunit) also results in ~65% reduction in INaR, suggesting that multiple Nav α subunits contribute to INaR in cerebellar Purkinje neurons and, in addition, that the contributions are non-linear. To date, all but one (Nav1.3) of the nine Nav channel α subunits have been shown to mediate, or can be induced to mediate, INaR in native or heterologous cells (Lewis and Raman, 2014; Jarecki et al., 2010, Do and Bean, 2004; Tan et al., 2014). It is unclear whether Nav1.3-encoded Nav channels also generate INaR, as it appears that voltage-clamp studies focused on exploring this possibility have not been conducted to date.
In the model proposed here, the amplitude of INaR is dependent on, and ultimately regulated by, two factors: the proportion of Nav channels that are non-inactivating at hyperpolarized voltages, that is, the number of channels that recover from inactivation into an open state on repolarization; and, the proportion of these non-inactivating channels that accumulate into a slow-inactivated state. In the simulations presented in Figure 9, we show that by promoting the occupancy of the IS (slow-inactivated) state, we can recapitulate the experimental observation that INaR amplitudes are reduced in Scn4b-/-, compared with wild type, cerebellar Purkinje neurons, suggesting the possibility that Navβ4 expression regulates INaR by influencing slow inactivation. The hypothesis that INaR is directly affected by Nav channel slow inactivation is also consistent with previous work (Hampl et al., 2016), showing that mutations in the Nav1.6 and Nav1.7 α subunits that enhance or inhibit slow inactivation also result in reduced or increased, respectively, INaR amplitudes. Interestingly, it has also been reported that mutation of the IFM motif (to QQQ) in Nav1.4, in addition to completely eliminating fast inactivation, increases the proportion of channels that enter the slow inactivated state (Featherstone et al., 1996), suggesting that the model developed here (Figure 3A) in which the fast and slow components of inactivation are exclusive, may be applicable to diverse Nav channels in different cell types and encoded by different α subunits.
There are a number of additional factors, intrinsic and extrinsic, to Nav α subunits, as well as additional pre- and post-translational mechanisms, that regulate persistent Nav currents (Aman et al., 2009; Hammarstrom and Gage, 1998; Paul et al., 2016; Lin and Baines, 2015) and slow inactivation (Chen et al., 2008; Silva, 2014; Chen et al., 2006; Carr et al., 2003). In the model developed and presented here, the combined effects of these factors/mechanisms will regulate/modulate the amplitudes and the time- and voltage-dependent properties of INaR. In this context, it is interesting to note that the experiments presented in Figure 5 indicate that the Nav channels underlying INaR, compared to the sum of the Nav channels underlying INaT, are differentially sensitive to slow inactivation. This observation clearly suggests that there is functional (and perhaps molecular) heterogeneity in the population of Nav channels underlying the Nav currents in mouse cerebellar Purkinje neurons, with channels underlying INaR having properties distinct from at least a portion of the Nav channels that participate in the generation of INaT, but that do not produce INaR. This possibility is consistent with results presented by White et al., 2019, in which INaR was found to have a greater sensitivity to tetrodotoxin block than INaT in mouse cerebellar Purkinje neurons. It should also be noted that the Markov model developed here was created to reflect a single channel population, that is, Nav channels capable of generating both INaT and INaR. As such, the developed Markov model does not reproduce the differential recovery from inactivation presented in Figure 5.
Functional implications
Based on the experimental data and the computational modeling presented here, we propose a novel, blocking particle-independent, gating mechanism for the generation of INaR that involves two, kinetically distinct inactivation pathways. The modeling results suggest that two parameters are critical in determining the magnitude and the time- and voltage-dependent properties of INaR: (1) the relative amplitude of the persistent Nav current, INaP, component; and, (2) the proportion of the persistent Nav channels (channels that fail to undergo fast inactivation) that undergo slow inactivation. Interestingly, INaR has now been identified in over 20 types of neurons, many of which do not display the high rates of repetitive firing that are characteristics of cerebellar Purkinje neurons, suggesting that the role(s) of INaR in the regulation of membrane excitability are diverse, and likely distinct, in different neuronal cell types (Lewis and Raman, 2014). Recent studies conducted on serotonergic raphe neurons, for example, suggest that the accumulation of Nav channels in a slow-inactivated state functions as a homeostatic brake on repetitive firing (Navarro et al., 2020). In addition, INaR has been implicated in several inherited and acquired neurological diseases (Lewis and Raman, 2014), including paroxysmal extreme pain disorder, paramyotonia congenita (Jarecki et al., 2010), and chemotherapy-induced neuropathy (Lewis and Raman, 2014; Sittl et al., 2012), as well as epilepsy (Hargus et al., 2013). Given the implications of these findings, it will be of considerable interest to detail the properties of INaR in different types of neurons and to explore directly the hypothesis that the rate of decay of INaR on membrane repolarization plays a role in determining how much persistent sodium current is available to contribute to repetitive firing, as well as to define the molecular mechanisms that control INaR amplitudes, kinetics, and functioning in diverse neuronal cell types.
Materials and methods
Animals
All animal experiments were performed in accordance with the guidelines published in the National Institutes of Health Guide for the Care and Use of Laboratory Animals. Protocols were approved by the Washington University Institutional Animal Care and Use Committee (IACUC). Postnatal day 12–16 (P12–P16) male and female C57BL6/J (Jackson laboratories) mice were used in the experiments reported here.
Isolation of neonatal cerebellar Purkinje neurons
Request a detailed protocolFor the preparation of isolated cerebellar Purkinje neurons, postnatal day 12–16 (P12–P16) animals were anesthetized with 1.25% Avertin and brains were rapidly removed and placed in ice-cold isolation medium containing (in mM): 82 Na2SO4, 30 K2SO4, 5 MgCl2, 10 HEPES, 10 glucose, and 0.001% phenol red (at pH 7.4). Using a scalpel, the cerebellum was removed, minced into small chunks and incubated in isolation medium containing 3 mg/ml protease XXIV at 33°C for 10–15 min. Following this incubation period, the tissue pieces were washed with enzyme-free isolation medium containing 1 mg/ml bovine serum albumin and 1 mg/ml trypsin inhibitor. The tissue pieces were transferred to artificial cerebral spinal fluid (ACSF) that was continuously bubbled with 95% oxygen/5% carbon dioxide and contained (in mM): 125 NaCl, 2.5 KCl, 1.25 NaH2PO4, 25 NaHCO3, 2 CaCl2, 1 MgCl2, and 25 dextrose (310 mosmol/l) at 22–23°C. Tissue pieces were triturated with a fire-polished glass pipette. An aliquot of the cell suspension was placed on a coverslip in the recording chamber and superfused with fresh ACSF (at a rate of 0.5 ml/min), saturated with 95% O2/5% CO2, for 25 min before beginning electrophysiological experiments.
Electrophysiological recordings
Request a detailed protocolWhole-cell voltage-clamp recordings were obtained at room temperature from visually identified cerebellar Purkinje neurons using differential interference contrast optics. Data were collected using a Multiclamp 700B patch clamp amplifier interfaced to a Dell PC with a Digidata 1332 and pCLAMP 10 software (Axon Instruments, Union City, CA). In all recordings, tip potentials were zeroed prior to forming a giga-ohm membrane–pipette seal. Pipette capacitances were compensated using the pCLAMP software. Signals were acquired at 50–100 kHz and filtered at 10 kHz prior to digitization and storage.
In the experiments here, INaR was routinely recorded in ACSF (containing ~151 mM Na+; see above) with 5 mM tetraethylammonium chloride (TEA-Cl) and 250 μM cadmium chloride (CdCl2) added. To decrease Nav currents, improve the spatial control of the membrane voltage (space-clamp) and enable reliable recordings of INaT or INaP, INaR was also recorded in some experiments (see Figure 6) in bath solution containing (in mM): 25 NaCl, 100 TEACl, 2.5 KCl, 1.25 NaH2PO4, 25 NaHCO3, 2 CaCl2, 1 MgCl2, 25 dextrose, and 0.25 CdCl2. Recording pipettes routinely contained (in mM): 110 CsCl, 15 TEACl, 5 4AP, 1 CaCl2, 2 MgCl2, 10 EGTA, 4 Na2-ATP, and 10 HEPES, pH 7.25 300 mosmol/l. Alternative bath and internal solutions were used in experiments presented in Figure 2; these solutions are described in the Results section and in the legend to Figure 2. In all experiments, recording pipettes had resistances of 2–3 MΩ.
Membrane capacitances were determined by analyzing the decays of capacitive currents elicited by short (25 ms) voltage steps (±10 mV) from the holding potential (−70 mV). Whole-cell membrane capacitances (Cm) were calculated for each cell by dividing the integrated capacitive transients by the voltage. Consistent with the short time in culture and lack of extensive processes, the capacitive transients of recorded cells had single-exponential decay phases. Input resistances were calculated from the steady-state currents elicited by the same ±10 mV steps (from the holding potential). For each cell, the series resistance was calculated by dividing the time constant of the decay of the capacitive transient (fit by a single exponential) by the Cm. Series resistances were routinely compensated ≥80%. Voltage errors resulting from the uncompensated series resistance were always ≤4 mV and were not corrected. Only data obtained from cells with input resistances >50 MΩ and capacitive transients well described by single exponentials were included in the cumulative data analyses presented.
Measurements of INaT, INaR, and INaP
Request a detailed protocolIn the standard voltage-clamp studies, involving step depolarizations, peak transient Nav conductances (in each cell at each test potential) were calculated (using Ereversal = +75 mV) from measurements of peak INaT following digital subtraction of the non-inactivating, persistent Nav current component (INaP), measured during the same voltage step. These peak transient Nav conductances were then normalized to the maximal peak transient Nav conductance determined in the same cell. Mean ± SEM normalized peak transient Nav conductances were then determined, plotted as a function of the test potential, and fitted with a single (Equation 1) Boltzmann function:
where Vh is the membrane potential of half-maximal activation and k is the slope factor.
To determine the voltage dependence of steady-state inactivation of INaT, a two-step voltage-clamp protocol was used. From a holding potential of –70 mV, brief (20 ms) voltage steps to various conditioning voltages, between –120 and –35 mV, were presented prior to depolarizations to 0 mV to evoke INaT. Peak INaT amplitudes at 0 mV, evoked from each conditioning potential in each cell, were measured following digital subtraction of INaP, measured during the same voltage step, and subsequently normalized to the peak INaT amplitude evoked (at 0 mV) from the –120 mV conditioning step in the same cell. Mean ± SEM normalized peak INaT amplitudes were plotted as a function of the conditioning voltage and fitted with a single (Equation 2) Boltzmann function:
where Vh is the membrane potential of half-maximal inactivation and k is the slope factor.
The peak amplitudes of INaR, evoked on hyperpolarizations to various membrane potentials, following brief (5 ms) depolarizing voltage steps to 0 mV, were determined following digital subtraction of INaP, measured during the same voltage step (in the same cell). The time constants (tau) of the decay of INaR were determined from first-order exponential fits to the decay phases of these subtracted records. The amplitudes of INaP were routinely determined by direct measurement of the steady-state inward currents remaining at the end of depolarizing (or hyperpolarizing) voltage steps. INaP (or INaP plus INaR) amplitudes were also determined in additional experiments from analyses of the currents recorded during depolarizing (or hyperpolarizing) voltage ramps (see Figure 6).
The peak amplitudes of INaT and INaR, generated using our Nav channel gating model or the Raman-Bean model, were routinely determined directly from the simulated current records, that is, without subtraction of INaP. Determinations of INaT and INaR amplitudes following subtraction of INaP yielded indistinguishable results. The time constants of decay of INaR simulated using our model or the Raman-Bean model were determined from single exponential fits to the inactivating component of the current (i.e., INaR uncontaminated by INaP).
Sample sizes were determined based on mean data (and associated standard deviations) obtained from analyses of voltage-clamp recordings of Nav currents obtained from mouse cerebellar Purkinje neurons (Ransdell et al., 2017). As in this previous study, all of the mean data presented here reflect results obtained from biological replicates, that is, analyses of Nav current recordings, evoked using identical voltage-clamp protocols, obtained from individual isolated Purkinje neurons. Technical replicates, that is, the presentation of identical voltage-clamp protocols to the same Purkinje neuron, were occasionally acquired to ensure that the properties of the Nav currents did not change during prolonged whole-cell recordings. Technical replicates were not included in the data analysis or reported results.
Data were analyzed using ClampFit (Molecular Devices), MATLAB (Mathworks), Microsoft Excel, and Prism (GraphPad Software Inc).
Simulations using the Raman-Bean model
Request a detailed protocolThe Raman-Bean model of Nav channel gating (Raman and Bean, 2001) was coded in MATLAB using the equations and schematic of the Markov model structure presented in Figure 7 of Raman and Bean, 2001; no changes were made to the published model or parameters (Raman and Bean, 2001). The model simulations were run as described below using the matrix exponential technique (Teed and Silva, 2016).
Development of a computational model of Nav channel gating
Request a detailed protocolA Markov kinetic state model of Nav channel gating in mouse cerebellar Purkinje neurons was formulated based on our acquired experimental data that led to a hypothesized structure of channel gating states (see: Figure 3A). The rate constants in the model were all single exponentials derived from the acquired experimental data and were optimized numerically using described methods (Moreno et al., 2016; Teed and Silva, 2016). All simulations, numerical optimization, and data visualization were done in MATLAB 2017B. Detailed methods are below. Model definition files and MATLAB scripts used for the simulations are available at https://github.com/morenomdphd/Resurgent_INa; Moreno, 2022. The equations were verified by one of the authors (DB) who was not involved in the creation of the model.
The Nav channel gating model developed (see Figure 3) consists of nine states: three closed states (C1, C2, C3), a single open state (O), two closed-inactivated states (IC1, IC2), two inactivated states, resulting from fast channel inactivation (IF1, IF2), and an additional, slowly populated inactivated state (IS). The model accurately simulates the experimentally observed kinetics and voltage dependences of Nav channel gating, including activation, inactivation (closed and open state), deactivation, recovery from fast inactivation, as well as the voltage dependence of activation and the proportion (relative to INaT) of INaR.
Numerical optimization procedure
Request a detailed protocolAll computations were done in MATLAB 2017B. We coded all voltage protocols and used the matrix exponential technique, which is described in Teed and Silva, 2016, for simulations. A modified Nelder-Mead simplex method that allows for constrained optimization (only positive rate constants) was used for simultaneous optimization of the protocols listed below. A cost function for each protocol was defined as the sum of squared differences between the experiments and the simulations. The total cost function (sum of the individual protocol errors) was then minimized and converged when a tolerance of 0.01 for the change of the cost function and 0.01 for the change in parameters was achieved. For further details about this numerical optimization method, see Moreno et al., 2016.
The protocols were as follows:
Steady-state availability: For each voltage between –120 and –10 mV, the steady-state probabilities of the channel were found. The channel was then depolarized to 0 mV, and the open-state probability was determined. The value of the open-state probability was then normalized to the open-state probability at –120 mV.
Steady-state activation: Channel steady state was found at –80 mV. The channel was then depolarized to voltages between –77 and 0 mV (in 3 mV increments). For each voltage, the maximum open probability of the channel was calculated, and the conductance, GNa, at each voltage was determined. The calculated values were then normalized to GNa at 0 mV.
Tau of inactivation: Channel steady state was found at a holding potential of –90 mV. From steady state, the channel was depolarized to voltages between –50 and 0 mV (in 5 mV increment), and the time constant (tau) corresponding to 64% decay (1/e) of the peak current was calculated for each voltage.
Recovery from fast inactivation: From a steady state of –90 mV, the channel was depolarized to 0 mV, and the peak current determined. The channel was then allowed to recover at –90 mV for variable time intervals, before being depolarized again to 0 mV. The peak currents during the depolarizations to 0 mV following the various recovery times were determined and normalized to the initial peak current.
Persistent component of the Nav current: From a steady state of –90 mV, the channel was depolarized to 0 mV for 5 ms, and subsequently hyperpolarized to –45 mV for 100 ms. The persistent current recorded after the 100 ms hyperpolarizing step was measured and normalized to the initial peak current.
Voltage dependence of the ratio of the peak resurgent to peak transient Nav current amplitude: From a steady state of –90 mV, the channel was depolarized to 0 mV for 5 ms, and subsequently hyperpolarized to potentials between –5 and –80 mV (in 5 mV increments). The peak resurgent current at each hyperpolarized voltage step was determined and normalized to the peak transient inward current evoked at 0 mV.
Dependence of the peak INaR amplitude on the duration of the depolarizing voltage step duration: From a steady state of –90 mV, the channel was depolarized to +20 mV for varying times (2 to 36 ms) prior to hyperpolarization to –45 mV. The amplitude of INaR at –45 mV following each depolarizing voltage step to +20 mV (of varying durations) was measured and normalized to the peak INaR evoked following the 2 ms depolarizing voltage step.
Tau of decay of the resurgent Nav current: From a steady state of –90 mV, the channel was depolarized to 0 mV for 5 ms, and subsequently hyperpolarized to potentials ranging from –5 to –45 mV (in 5 mV increments). The time constant (tau) of the exponential decay (1/e) of the resurgent current at each hyperpolarized test potential was determined.
Voltage independence of peak resurgent current: Consistent with the experimental data presented in Figure 2D and E, the peak resurgent current was independent of the potential of the depolarizing voltage step. From a steady state of –90 mV, the channel was depolarized to membrane potentials ranging from –5 to –35 mV (in 5 mV increments) prior to a hyperpolarizing voltage step to –45 mV. The difference between the peak INaR measured at –45 mV from each depolarized potential was determined, and the mean resurgent peak current determined across all depolarized potentials was minimized. This ensured the peak resurgent current was constant without specifying the magnitude of the resurgent peak a priori.
Optimization of the Scn4b-/- Nav current model
Request a detailed protocolTo develop the model for Nav channels lacking Scn4b, the optimization routine was restarted from the optimized wild type rate constants (initial conditions). The ‘Voltage dependence of the ratio of the peak resurgent to peak transient Nav current amplitude’ protocol described above was fitted to the experimental data acquired from isolated cerebellar Purkinje neurons from (Scn4b-/-) animals harboring a targeted disruption in the Scn4b locus (Ransdell et al., 2017); the data are presented in Figure 9A. All of the other protocols used in the optimization procedure for Scn4b-/- were the same as those described above for the wild type Nav channel to ensure no other changes to the model.
Model parameters
Request a detailed protocol| Rate Parameter | Optimized Rate (WT) | Optimized rate (Scn4b-/-) | Rate Equations |
|---|---|---|---|
| a11_variable1 | 2.3989e-02; | 2.0761e-02; | Where the transition rates are of the form: |
| a11_variable2 | 9.6108e + 02; | 9.7685e + 02; | |
| a12 | 8.5613e + 02; | 8.3340e + 02; | a11= Tfactor*1/(Input(1)*exp(-V/Input(2))); |
| a13 | 7.2682e + 01; | 6.1087e + 01; | a12= Input(3)*a11; |
| b11_variable1 | 1.7233e-01; | 1.6995e-01; | a13= Input(4)*a11; |
| b11_variable2 | 1.9691e + 01; | 1.8560e + 01; | b11= Tfactor*1/(Input(5)*exp(V/Input(6))); |
| b12 | 8.8549e + 01; | 9.3042e + 01; | b12= Input(7)*b11; |
| b13 | 1.4841e + 02; | 1.7914e + 02; | b13= Input(8)*b11; |
| a3_variable1 | 3.6734e-01; | 3.9273e-01; | a3= Tfactor*Input(9)*exp(-V/Input(10)); |
| a3_variable2 | 9.8034e + 02; | 9.8807e + 02; | b3= Tfactor*Input(11)*exp((V)/Input(12)); |
| b3_variable1 | 5.3241e + 01; | 4.7402e + 01; | a2= Tfactor*(Input(13)*exp(V/Input(14))); |
| b3_variable2 | 1.4204e + 01; | 1.3469e + 01; | b2= ((a13*a2*a3)/(b13*b3)); |
| a2_variable1 | 8.7852e + 01; | 8.1085e + 01; | a6= Tfactor*(Input(15)*exp(V/Input(16))); |
| a2_variable2 | 9.9972e + 02; | 9.9984e + 02; | b6= Tfactor*Input(17)*exp(-V/Input(18)); |
| a6_variable1 | 6.0921e + 02; | 6.0756e + 02; | |
| a6_variable2 | 8.6490e + 01; | 7.8591e + 01; | a2s = Tfactor*(Input(19)*exp(V/Input(20))); |
| b6_variable1 | 1.5645e + 02; | 1.6214e + 02; | b2s = Tfactor*(Input(21)*exp(-V/Input(22))); |
| b6_variable2 | 3.0317e + 01; | 4.3656e + 01; | a3s = Tfactor*Input(23)*exp(-V/Input(24)); |
| a2s_variable1 | 1.5817e + 01; | 2.3130e + 01; | b3s = (a2s*a3s*a13)/(b2s*b13); |
| a2s_variable2 | 9.9982e + 02; | 9.9969e + 02; | |
| b2s_variable1 | 1.0010e-03; | 1.0208e-03; | Q10 = 3; T = 295; |
| b2s_variable2 | 1.1963e + 01; | 1.1383e + 01; | Tfactor = 1.0/(Q10^((37.0-(T-273))/10.0)); |
| a3s_variable1 | 4.4773e-03; | 2.8677e-03; | |
| a3s_variable2 | 9.9993e + 02; | 9.8330e + 02; | Note: b2, b3s are constrained by microscopic reversibility. |
Of note, in the MATLAB script, the Nav channel model developed here contains 24 parameters; these are inputted as a matrix ‘Input’. For example, Input(1) corresponds to a11_variable1, and Input(2) corresponds to a11_variable2, Input(3) corresponds to a12, and Input(4) corresponds to a13, etc. The transition rate constants are of the form denoted in the right-hand column of the table above.
Data availability
Model definition files and Matlab scripts used for the simulations are available at https://github.com/morenomdphd/Resurgent_INa, (copy archived at swh:1:rev:07202f3d8c299b3b918fd9ae91b005a07d34c095).
References
-
Regulation of persistent Na current by interactions between beta subunits of voltage-gated Na channelsThe Journal of Neuroscience 29:2027–2042.https://doi.org/10.1523/JNEUROSCI.4531-08.2009
-
Inwardly permeating Na ions generate the voltage dependence of resurgent Na current in cerebellar Purkinje neuronsThe Journal of Neuroscience 30:5629–5634.https://doi.org/10.1523/JNEUROSCI.0376-10.2010
-
Inactivation of the sodium channel. I. Sodium current experimentsThe Journal of General Physiology 70:549–566.https://doi.org/10.1085/jgp.70.5.549
-
The voltage sensor in voltage-dependent ion channelsPhysiological Reviews 80:555–592.https://doi.org/10.1152/physrev.2000.80.2.555
-
Domain IV voltage-sensor movement is both sufficient and rate limiting for fast inactivation in sodium channelsThe Journal of General Physiology 142:101–112.https://doi.org/10.1085/jgp.201310998
-
Tracking voltage-dependent conformational changes in skeletal muscle sodium channel during activationThe Journal of General Physiology 120:629–645.https://doi.org/10.1085/jgp.20028679
-
Functional properties and differential neuromodulation of Na(v)1.6 channelsMolecular and Cellular Neurosciences 38:607–615.https://doi.org/10.1016/j.mcn.2008.05.009
-
Electrophysiological properties of mutant Nav1.7 sodium channels in a painful inherited neuropathyThe Journal of Neuroscience 24:8232–8236.https://doi.org/10.1523/JNEUROSCI.2695-04.2004
-
Sodium currents in subthalamic nucleus neurons from Nav1.6-null miceJournal of Neurophysiology 92:726–733.https://doi.org/10.1152/jn.00186.2004
-
Long-term inactivation particle for voltage-gated sodium channelsThe Journal of Physiology 588:3695–3711.https://doi.org/10.1113/jphysiol.2010.192559
-
Interaction between fast and slow inactivation in Skm1 sodium channelsBiophysical Journal 71:3098–3109.https://doi.org/10.1016/S0006-3495(96)79504-8
-
A role for phosphorylation in the maintenance of resurgent sodium current in cerebellar purkinje neuronsThe Journal of Neuroscience 22:3100–3107.https://doi.org/10.1523/JNEUROSCI.22-08-03100.2002
-
Inhibition of oxidative metabolism increases persistent sodium current in rat CA1 hippocampal neuronsThe Journal of Physiology 510 (Pt 3):735–741.https://doi.org/10.1111/j.1469-7793.1998.735bj.x
-
Sodium channel slow inactivation interferes with open channel blockScientific Reports 6:25974.https://doi.org/10.1038/srep25974
-
Evidence for a role of Nav1.6 in facilitating increases in neuronal hyperexcitability during epileptogenesisJournal of Neurophysiology 110:1144–1157.https://doi.org/10.1152/jn.00383.2013
-
Immobilizing the moving parts of voltage-gated ion channelsThe Journal of General Physiology 116:461–476.https://doi.org/10.1085/jgp.116.3.461
-
Regulation of Na+ channel inactivation by the DIII and DIV voltage-sensing domainsThe Journal of General Physiology 149:389–403.https://doi.org/10.1085/jgp.201611678
-
Human voltage-gated sodium channel mutations that cause inherited neuronal and muscle channelopathies increase resurgent sodium currentsThe Journal of Clinical Investigation 120:369–378.https://doi.org/10.1172/JCI40801
-
Kinetic and stochastic properties of a persistent sodium current in mature guinea pig cerebellar Purkinje cellsJournal of Neurophysiology 80:1167–1179.https://doi.org/10.1152/jn.1998.80.3.1167
-
Resurgent current of voltage-gated Na(+) channelsThe Journal of Physiology 592:4825–4838.https://doi.org/10.1113/jphysiol.2014.277582
-
Identification of structures for ion channel kinetic modelsPLOS Computational Biology 17:e1008932.https://doi.org/10.1371/journal.pcbi.1008932
-
SoftwareResurgent_INa, version swh:1:rev:07202f3d8c299b3b918fd9ae91b005a07d34c095Software Heritage.
-
Resurgent sodium current and action potential formation in dissociated cerebellar Purkinje neuronsThe Journal of Neuroscience 17:4517–4526.https://doi.org/10.1523/JNEUROSCI.17-12-04517.1997
-
Ionic currents underlying spontaneous action potentials in isolated cerebellar Purkinje neuronsThe Journal of Neuroscience 19:1663–1674.https://doi.org/10.1523/JNEUROSCI.19-05-01663.1999
-
Resurgent current and voltage sensor trapping enhanced activation by a beta-scorpion toxin solely in Nav1.6 channel. Significance in mice Purkinje neuronsThe Journal of Biological Chemistry 281:20326–20337.https://doi.org/10.1074/jbc.M600565200
-
BookSlow inactivation of Na(+) channelsIn: Silva J, editors. Handbook of Experimental Pharmacology. Elesvier. pp. 33–49.https://doi.org/10.1007/978-3-642-41588-3_3
-
Mechanism underlying hooked resurgent-like tail currents induced by an insecticide in human cardiac Nav1.5Toxicology and Applied Pharmacology 397:115010.https://doi.org/10.1016/j.taap.2020.115010
-
Effects of FGF14 and NaVβ4 deletion on transient and resurgent Na current in cerebellar Purkinje neuronsThe Journal of General Physiology 151:1300–1318.https://doi.org/10.1085/jgp.201912390
-
FGF14 localization and organization of the axon initial segmentMolecular and Cellular Neurosciences 56:393–403.https://doi.org/10.1016/j.mcn.2013.07.008
Article and author information
Author details
Funding
National Institute of Neurological Disorders and Stroke (NS065761)
- Jeanne M Nerbonne
National Heart, Lung, and Blood Institute (HL136553)
- Jonathan R Silva
National Institute of Neurological Disorders and Stroke (NS090765)
- Joseph L Ransdell
The funders had no role in study design, data collection and interpretation, or the decision to submit the work for publication.
Acknowledgements
The authors thank Mr Richard Wilson for expert technical assistance. The financial support provided by the NIH (R01 NS065761 to JMN, R01 HL136553 to JRS, and F32 NS090765 to JLR) is also gratefully acknowledged; JDM was supported by an NIH institutional training grant (T32 HL007081) and a grant from the Foundation for Barnes Jewish Hospital. The authors declare no competing financial interests.
Ethics
This study was performed in strict accordance with the recommendations in the Guide for the Care and Use of Laboratory Animals of the National Institutes of Health. All of the animals were handled according to an approved institutional animal care and use committee (IACUC) protocol (20180045) of Washington University in St. Louis (Animal Welfare Assurance # A-3381-01).
Copyright
© 2022, Ransdell et al.
This article is distributed under the terms of the Creative Commons Attribution License, which permits unrestricted use and redistribution provided that the original author and source are credited.
Metrics
-
- 1,967
- views
-
- 288
- downloads
-
- 11
- citations
Views, downloads and citations are aggregated across all versions of this paper published by eLife.
Citations by DOI
-
- 11
- citations for umbrella DOI https://doi.org/10.7554/eLife.70173






