Ionic mechanisms underlying history-dependence of conduction delay in an unmyelinated axon
Abstract
Axonal conduction velocity can change substantially during ongoing activity, thus modifying spike interval structures and, potentially, temporal coding. We used a biophysical model to unmask mechanisms underlying the history-dependence of conduction. The model replicates activity in the unmyelinated axon of the crustacean stomatogastric pyloric dilator neuron. At the timescale of a single burst, conduction delay has a non-monotonic relationship with instantaneous frequency, which depends on the gating rates of the fast voltage-gated Na+ current. At the slower timescale of minutes, the mean value and variability of conduction delay increase. These effects are because of hyperpolarization of the baseline membrane potential by the Na+/K+ pump, balanced by an h-current, both of which affect the gating of the Na+ current. We explore the mechanisms of history-dependence of conduction delay in axons and develop an empirical equation that accurately predicts this history-dependence, both in the model and in experimental measurements.
https://doi.org/10.7554/eLife.25382.001Introduction
Recent years have seen a growing appreciation of the role of axons in neural signaling. Rather than serving solely as faithful transmission lines of the neural code, axons shape neuron output through activity- and neuromodulator-dependent changes in propagation fidelity (Debanne, 2004; Alle and Geiger, 2008; Kress and Mennerick, 2009; Bucher and Goaillard, 2011; Debanne et al., 2011; Sasaki, 2013; Bucher, 2015). Repetitive activity alters axonal membrane excitability and conduction velocity, which can substantially change the temporal pattern of spikes during propagation from proximal initiation sites to distal presynaptic sites. Apart from the more extreme cases of spike failures or ectopic spike initiation, changes in conduction velocity across consecutive spikes result in varying conduction delays, and therefore in altered spike intervals. Temporal coding schemes, in which information is contained in spike intervals, latencies, or firing phase (Panzeri et al., 2010), may be exquisitely sensitive to such changes.
Many of the activity-dependent effects on spike propagation are owed to the fact that membrane excitability in most axons is substantially more complex than often credited. In addition to the basic ionic mechanisms necessary for spike propagation, i.e., fast sodium and delayed rectifier potassium conductances (Hodgkin and Huxley, 1952a, 1952b), axons can have a large variety of voltage-gated ion channels and ion pumps (Krishnan et al., 2009; Bucher and Goaillard, 2011; Debanne et al., 2011). As the activation and gating of these currents can be associated with a wide range of time constants, conduction velocity can change depending on the history of activity at timescales far exceeding refractory effects occurring in the millisecond range (George, 1977; Raymond, 1979; Weidner et al., 2002; Bucher and Goaillard, 2011; Ballo et al., 2012). However, the ionic mechanisms underlying history-dependence of spike propagation are not well understood, and the common approach to test excitability changes with simple paired conditioning and test pulses (Bostock et al., 1998; Krishnan et al., 2009; Bucher and Goaillard, 2011) is insufficient to capture slow dynamics and transformation of complex temporal patterns (Weidner et al., 2002; Ballo et al., 2012).
Conduction velocity in unmyelinated axons depends on membrane and cytosol properties, and axon diameter (Hodgkin and Huxley, 1952a; Hodgkin, 1954; Del Castillo and Moore, 1959; Waxman, 1975; Bucher and Goaillard, 2011). Total conductance change during the spike (Matsumoto and Tasaki, 1977; Tasaki and Matsumoto, 2002; Tasaki, 2004), as well as sodium channel gating (Muratov, 2000), can be used to accurately predict conduction velocity of isolated spikes. It is unclear, however, how well those descriptions apply to complex excitability changes during highly repetitive activity. Additionally, theoretical investigations of the history-dependence of conduction delay have considered only minimal sets of ionic currents (Miller and Rinzel, 1981; Kepler and Marder, 1993; Moradmand and Goldfinger, 1995; Faisal and Laughlin, 2007).
Here we study the ionic mechanisms underlying history-dependence of conduction delay at different timescales, using a biophysical axon model with relatively complex excitability. The model was inspired by experimental findings from the pyloric dilator (PD) motor axon of the lobster Homarus americanus stomatogastric nervous system. This several-centimeters long axon shows large changes in conduction delay during repetitive stimulation (Ballo and Bucher, 2009; Ballo et al., 2012). Delays depend on spike intervals, but this dependence changes substantially over the course of several minutes of stimulation. Furthermore, axon excitability critically depends on modulation by dopamine, which drastically alters temporal fidelity (Ballo et al., 2010, 2012).
Our model replicates this behavior well. We find that delay changes are mostly because of the effect of membrane potential changes on a subset of variables describing sodium channel gating. Other currents contribute via their effect on the membrane potential. Equations developed to predict conduction velocity of isolated spikes fail to capture activity-dependent changes. We therefore develop empirical equations that allow good predictions of delay changes from either sodium channel gating variables or voltage trajectories. The latter also perform well on experimental data.
Results
The history-dependence of conduction delay in the model axon is similar to experimental observations in the PD axon
To examine dependence of a discrete process on the history of prior activity it is common to use Poisson train stimuli (Krausz and Friesen, 1977; Sen et al., 1996; Stern et al., 2009), which by definition include a large range of inherent frequencies. Poisson trains have been used before in Hodgkin-Huxley model axons to characterize spike delay and velocity (George, 1977; Moradmand and Goldfinger, 1995), but not commonly in model axons with more complex excitability. We therefore examined the history-dependence of conduction delay in the PD neuron model axon by stimulating one end of the axon and measuring conduction delay between two positions along its length (Figure 1).
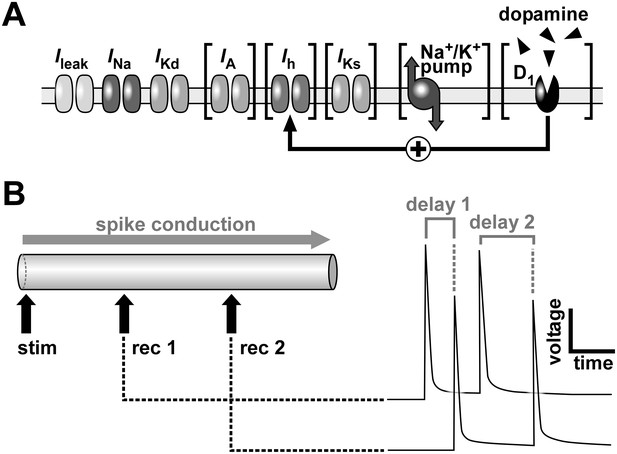
Schematic of the model axon and conduction delay.
(A) Schematic of ion channels, the Na+/K+ pump and dopamine receptors in the model axon. Brackets indicate components that were omitted in some simulations. (B) The model axon was stimulated at one end and the conduction delay was measured between two positions at 0.3 and 0.7 times the length of the axon. Right panel shows conduction delays between the recording sites for two consecutive action potentials.
Experimental data from Poisson stimulation of the PD axon show a slow hyperpolarization that is accompanied by overall slowing of propagation (Ballo et al., 2012). History-dependence of delay is manifest in several ways. At the slow timescale (STS) of hyperpolarization, both the mean value and variability of conduction delay increase over a timescale of minutes following the onset of the stimuli (Figure 2A).
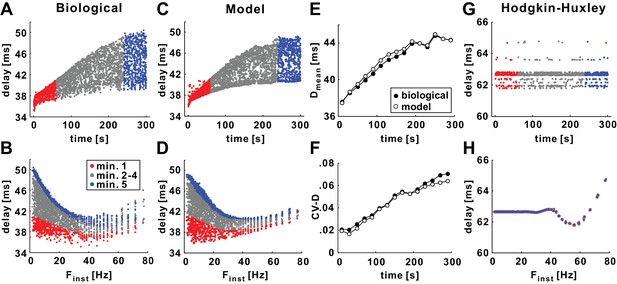
History-dependence of conduction delay in the model axon (with gh = 0).
(A) Delay shown as a function of stimulus time for a 10 Hz (mean rate), 5 min Poisson stimulation of the axon of the biological PD neuron. Red and blue, respectively, mark delay values for the first and fifth minute of stimulation. (B) The data in panel A, plotted as a function of the instantaneous stimulus frequency (Finst). Colors as in panel A. (C–D) The same Poisson stimulation as in panel A, applied to the model axon produces results that are quantitatively similar to the experimental data. (E–F) The mean (Dmean) and the coefficient variation (CV-D) of the data in panels A and C, measured in 20 s bins. (G–H) The same Poisson stimulation of panels C-D, applied to the standard Hodgkin-Huxley model axon.
At a fast timescale (FTS), a nonlinear and non-monotonic relationship between delay and the instantaneous stimulus frequency (Finst) is observed: conduction delay of the PD axon has a minimum value for Finst around 40 Hz but higher values for lower or higher Finst (Figure 2B). Note that the FTS effect compares the conduction delay of each spike only to that of the previous one. The FTS effect, that is, the nonlinear and non-monotonic frequency-dependence, changes with the STS effect, as can be seen in Figure 2B in the changing frequency-dependence at different times of the stimulation. These effects are strongest during pharmacological block of Ih, presumably because the axon hyperpolarizes more in the absence of inward rectification (Ballo et al., 2012, see below). The data shown in Figure 2A and B were obtained under these conditions. To match these conditions, the simulations shown in Figure 2C and D were performed without Ih in the model (=0), but with Ipump and IA in addition to ILeak, INa and IKd. We note that both the STS and the FTS effects were present with or without Ih in the model and, later on, we will show how Ih influences these effects in the model and experiments. The model captured, qualitatively and quantitatively, both the STS and the FTS history-dependence of conduction delay observed in the experiments (Figure 2C and D). To compare the experimental and model STS effect, we calculated the mean (Dmean) and coefficient of variation (CV-D) of conduction delay in 20 s time bins. The model provided an excellent match to the changes in Dmean and the increase in variability over the 5 min stimulation period (Figure 2E and F).
To test to which degree the STS and FTS history-dependence are the result of more complex membrane excitability, we applied the same Poisson stimulation to an axon modeled using the classical Hodgkin-Huxley equations (not including IA, Ih, and Ipump). The results of this simulation indicated that the Hodgkin-Huxley model axon showed no slow history-dependence (Figure 2G). It did, however, show a weak FTS history-dependence for Finst values larger than 40 Hz (Figure 2H). This effect was qualitatively similar to the FTS effect seen in the PD model axon (Figure 2D).
The STS effect is determined by the activity level of the Na+/K+ pump
The STS effect occurred over a timescale of minutes and should therefore be related to a slow activity-dependent process of the PD axon. In many axons, slowing of conduction is thought to be because of hyperpolarization caused by the Na+/K+ pump (Bucher and Goaillard, 2011), and our model did not produce any STS effect without the pump (not shown).
The activity level of Ipump is determined by the fast sodium current (see Materials and methods). Therefore, both the increase of Ipump with time, and the steady state level it reached, depended on the mean rate of stimulation (Figure 3A). Figure 3B and C show that both Dmean and CV-D also increased depending on the stimulation rate, and with time courses very similar to Ipump. With increased stimulation, Ipump produced a hyperpolarization of the baseline membrane potential (Figure 3D). When Ipump was modeled without dynamics and instead set to different constant values during Poisson stimulation, the baseline membrane potential (trough voltage, VT) showed a linear dependence on Ipump, while the peak voltage of spikes (VP) was fairly unaffected (Figure 3E). Both Dmean and CV-D appeared to be determined by the level of Ipump, as both showed a positive and linear dependence (Figure 3F). We therefore concluded that the dominant cause of the STS effect is the hyperpolarization mediated by Ipump.
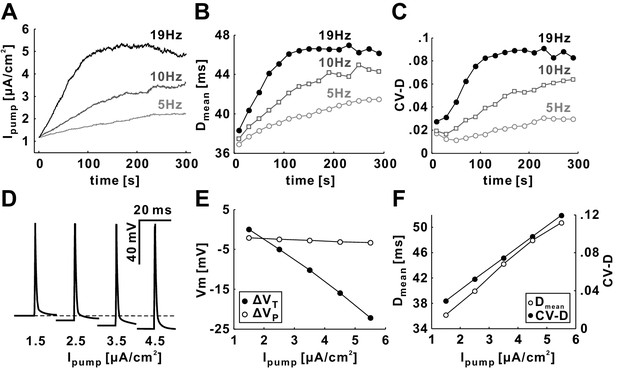
Dmean and CV-D strongly depend on the activity level of Ipump at all Poisson stimulation frequencies.
(A) The value of Ipump for Poisson stimulations with different mean rates (5, 10 and 19 Hz). (B–C) Dmean and CV-D increase with time as well as with the mean rate of Poisson stimulation. (D) The baseline membrane potential is hyperpolarized as the level of Ipump is increased. (E) The trough voltage (VT) linearly decreases with Ipump, but the peak voltage (VP) does not change much when Ipump is increased. (F) Dmean and CV-D linearly increase with the level of Ipump (set to a constant value in each simulation run).
A slow K+ current fails to produce the STS effect
Alternatively, slow hyperpolarization could be because of cumulative activation of slow K+ currents. We therefore tested if a slow conductance can produce a similar STS effect as Ipump. To address this possibility, we replaced the Na+/K+ pump with a slowly-activating potassium current (IKs) which was tuned to produce a slow increase of conductance on a timescale of several minutes. As before, was set to zero.
The voltage activity of the model axon with IKs showed a slow decrease in VP but little change in VT (Figure 4A). This was in contrast to the effect of Ipump, which had a strong effect in hyperpolarizing VT but little effect on VP (Figure 3D and E). Although gKs increased with a similar time course as Ipump in the model version described in Figure 3, the behavior of IKs was quite different. Unlike the slow continuous increase of Ipump (Figure 3A), IKs increased with each action potential and was reset back to almost 0 between action potentials (Figure 4B, expanded in 4C) and only the peak value of IKs increased with a similar time course as gKs. As a result, IKs was only effective during each spike (reducing VP) and had no cumulative effect in between spikes, and therefore could not change the baseline membrane potential. The lack of effect of IKs on VT is because of the small driving force of this current during the inter-spike intervals. Interestingly, Dmean increased with a similar time course as seen in the decrease of VP (Figure 4D and E), and this increase was only slightly smaller than in the model including Ipump. However, the CV-D stayed relatively constant throughout Poisson stimulation (Figure 4D and F). These results suggest that the STS effect on the variability of delay strongly depends on the baseline membrane potential, while increase in mean delay can be because of baseline hyperpolarization or decreasing peak voltage.
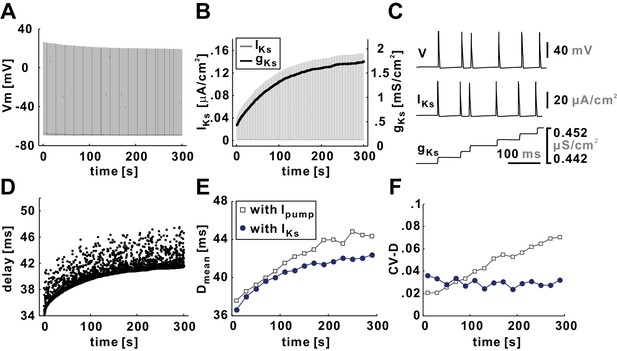
A slow potassium current (IKs) fails to capture the slow timescale effects on conduction delay.
(A) Voltage trace of the model axon, with IKs substituted for Ipump, for a 5 min, 10 Hz Poisson stimulation. (B) Both gKs and the peak values of IKs increase with time during the stimulation process. (C) A small time-window shows that, although gKs accumulates with time, IKs vanishes after each action potential because of the drop in the driving force. (D) Conduction delay of the model axon in response to a 5 min, 10 Hz Poisson stimulation. (E) Dmean increases with time but does not quite match the results of the model axon with Ipump. (F) CV-D does not increase with time as it does in the model axon with Ipump.
We conclude that the STS effect on variability of delay in the case of the PD axon could not easily be captured with IKs, possibly because the minimal driving force between spikes did not allow for a substantial cumulative hyperpolarization.
The dependence of conduction delay on Ih
In the PD axon, inward rectification by Ih plays an important role during repetitive firing. It is modulated by dopamine (DA), which increases gh 2–3 fold in the range of normal resting membrane potentials (Ballo et al., 2010). DA prevents hyperpolarization and drastically reduces history-dependence of conduction delay, while pharmacological block of Ih increases both hyperpolarization and history-dependence (Ballo et al., 2012). Here, we wanted to examine whether the experimentally-observed effects of DA and CsCl on Ih levels are sufficient to explain their effects on the history-dependence of conduction delay.
We approximated the effects of DA enhancement or pharmacological block of Ih by adjusting the model parameters governing this current (see Materials and methods and Tables 1 and 2), and we will refer to these respective model parameter sets as DA or Ih block. We examined how the STS and FTS effects were influenced by DA or Ih block. Such a comparison is shown in Figure 5 for the Poisson stimulation with a mean rate of 10 Hz. Blocking Ih increased both Dmean and CV-D in the model, an effect that qualitatively matched the experimental application of CsCl (Figure 5A,B). Consistent with experimental data, DA had the opposite influence on the STS effect.
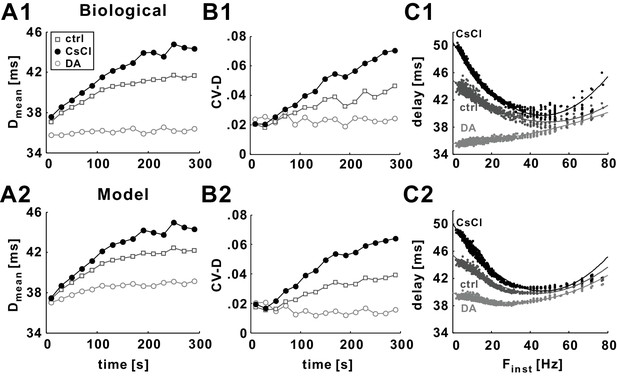
Changing the level of Ih in the model mimics the experimental effects of CsCl and DA.
(A1–B1) At the slow timescale, DA results in an increase of gh, which in turn causes Dmean and CV-D stay to remain relatively constant in response to a 5 min 10 Hz Poisson stimulation. In contrast, blocking Ih with CsCl results in an increase in Dmean and CV-D (Ballo et al., 2012). (A2–B2) This effect is mimicked by changing gh levels and the activation curve of Ih in the model. (C1) Changes in gh levels by DA or CsCl result in changes in the minimum delay (Dmin) and curvature (κmin) values in the delay vs. Finst plots (for the 5th minute of the Poisson stimulation). (C2) These effects are mimicked by the models with different activity level of Ih (see Materials and methods and Tables 1 and 2 for details).
Model parameters.
| Ionic Curr | [mS/cm2] | [mV] |
|---|---|---|
| INa | 14 | Dynamic |
| IKd | 3 | −70 |
| ILeak | 0.125 | −65 |
| IA | 5 | −70 |
| Ih (ctrl) | 0.05 | −32 |
| Ih (DA) | 0.1 | −25 |
| IKs | 3 | −70 |
| Other | Value | |
| Imax,pump | 2 mA/cm2 | |
| [Na+]1/2 | 78 mM | |
| [Na+]S | 2 mM | |
| α | 7.4 | |
| Vol | 7850 µm3 | |
| F | 96485 C/mol | |
Parameters of ionic current steady-state activation and inactivation and their time constants.
| Ionic Curr | m, h | [ms] | |
|---|---|---|---|
| INa | m3 | ||
| h | |||
| IKd | m4 | ||
| IA | m3 | ||
| h | 50 | ||
| Ih (ctrl) | m | 3700 | |
| Ih (DA) | m | 3800 | |
| IKs | m |
To quantify the influence of Ih on the FTS effect, we focused on the data in the fifth minute of the Poisson stimulation (Figure 5C). We fit the nonlinear relationship between delay and Finst with a quadratic function and determined κmin, the curvature at the frequency at which delay was smallest, as a measure of nonlinearity. Blocking Ih increased the nonlinearity in the Finst-delay relationship (Figure 5C2; control: κmin=0.0059; = 0: κmin=0.0091), whereas DA decreased the nonlinearity (2 x control : κmin=0.0032). These effects mimic the influence of CsCl and DA on the FTS effect in experiments (Figure 5C1; control: κmin=0.0051; CsCl: κmin=0.01; DA: κmin=0.001).
Although the biophysical model qualitatively captured the STS and FTS effects of Ih changes seen in biological experiments, there were small quantitative differences between the model and the experiments. These were most notable for the DA effect when the simulation Dmean values were larger than the experimental values (Figure 5A1–A2), and there was a small increase in Dmean that was not observed in the biological data. Additionally, the nonlinearity of the FTS effect was reduced but not removed as in the experiments (Figure 5C1–C2).
The FTS effects can be predicted by paired-pulse stimulation protocols
Activity-dependent changes in axon excitability and conduction velocity are often gauged with paired-pulse stimulations (Bucher and Goaillard, 2011), particularly for diagnostic purposes in the context of peripheral neuropathies (Bostock and Rothwell, 1997; Bostock et al., 1998; Burke et al., 2001; Krishnan et al., 2009). Such stimulation protocols consist of a single conditioning pulse followed by a test pulse delivered at different intervals. The change in spike threshold, conduction velocity, or conduction delay between conditioning and test pulses can then be analyzed as a function of inter-stimulus interval (ISI). Typically, excitability changes as a function of ISI in a sequence of relative refractory, supernormal, and sometimes subnormal intervals, collectively referred to as the ‘recovery cycle’. Recovery cycle measurements gauge excitability changes occurring at a similar timescale as the FTS effect seen in Poisson stimulations. We predicted that employing paired-pulse stimulations to test conduction velocity changes is equivalent to analyzing the FTS effect on conduction delay at the beginning of the Poisson stimulation.
Instead of conditioning trains of different duration or frequency, we used simple paired-pulse stimulations and mimicked different excitability states of the axon by setting Ipump to different constant values. These values were obtained from the 10 Hz Poisson stimulation results described in Figure 3A, the relatively low mean value during minute 1 (Ipump,LO), and the relatively high mean value during minute 5 (Ipump,HI). Figure 6A shows recovery cycle measurements for both levels of Ipump. Consistent with the STS effects of Ipump, the conduction velocities of the conditioning (line) and test spikes were higher with Ipump,LO than with Ipump,HI. For both levels of Ipump, test spikes showed reduced velocity at small ISIs compared to the conditioning spike (relative refractory period), and increased velocities at larger ISIs (supernormal period), before converging back to the velocity of the conditioning pulse at even larger ISIs. However, note that with different levels of Ipump, the peak conduction velocity of the test pulse corresponded to different ISIs and that the difference between the velocity of conditioning pulse and the peak velocity of the test pulse was larger with Ipump,HI.
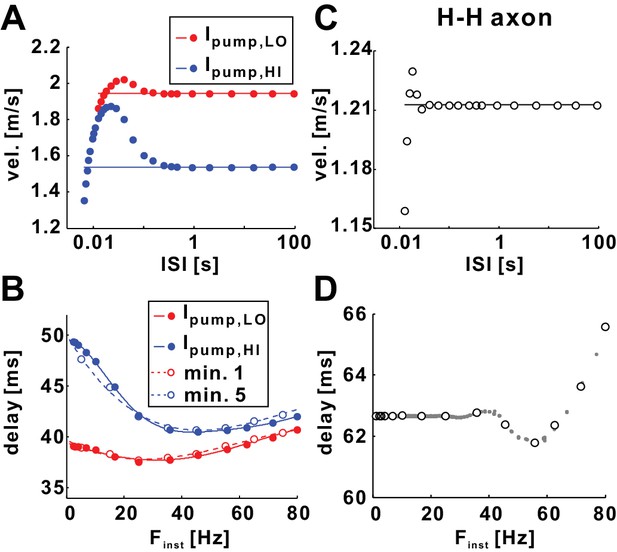
The fast timescale effect can be predicted by paired-pulse stimulations.
(A) Conduction velocity of conditioning (horizontal line) and test (filled circles) pulses plotted as a function of interspike interval (ISI). The graph shows the refractory and supernormal phases, where the velocity of the test pulse is, respectively, less or more than that of the conditioning pulse. Simulations were done with two constant pump currents: Ipump,LO and Ipump,HI, respectively equal to the mean value of Ipump during minute 1 and minute 5 of the 10 Hz Poisson stimulation (Figure 2). Horizontal lines show the velocity of the conditioning spikes. (B) Data of panel A plotted as conduction delay vs. Finst (=1/ISI), together with the Poisson stimulation results of minutes 1 and 5 (from Figure 2D). Solid curves are polynomial fits for the Ipump,LO and Ipump,HI datasets. (C) Paired-pulse stimulations of the Hodgkin-Huxley model axon show similar refractory and supernormal phases. (D) The data from the panel C compared with the Poisson stimulation data (from Figure 2H) of the Hodgkin-Huxley model axon show a perfect match.
In order to compare the paired-pulse data with the FTS effect seen in the delay vs. Finst relationship of the Poisson stimulation (Figure 2D), we plotted the data from Figure 6A as delay vs. Finst and fit these data with a cubic polynomial function. Figure 6B shows plots of these functions together with the data for the first and the fifth minute of the Poisson stimulation (the latter in 10 Hz bins). This comparison showed that the fits of the paired-pulse data provided very good predictions of the FTS effect seen in the Poisson stimulation (R2 = 0.939 for minute 1 and Ipump,LO; R2 = 0.937 for minute 5 and Ipump,HI). We can therefore conclude that at the fast timescale, delay is primarily determined by the ISI, that is, the timing of the last preceding spike. The STS effect influences the dependence of velocity or delay on ISI or Finst, but this effect is slow enough that the FTS effect exposed by Poisson stimulation can still be accurately predicted by simple paired-pulse or train-pulse stimulation methods.
We also compared the effect of paired-pulse and Poisson stimulations in the Hodgkin-Huxley model axon. As shown in Figure 2, this model exhibits no STS effect. We therefore predicted that recovery cycle measurements should perfectly match data from Poisson stimulations, even when repetitive activity is used as a conditioning paradigm. To test this, we used a single pulse as conditioning stimulus. The recovery cycle measurement in Figure 6C shows that relative refractory and supernormal periods were restricted to relatively small ISIs. Figure 6D shows that when plotted as delay vs. Finst, results from paired-pulse stimulations are a near perfect match to results from Poisson stimulations.
The FTS effects of the PD axon during ongoing bursting can be predicted by paired-pulse stimulation
The PD neuron is a member of the pacemaker group of the pyloric network and its natural ongoing activity consists of bursts with a cycle frequency of ~1 Hz and a parabolic interval structure within each burst (Szucs et al., 2003; Ballo and Bucher, 2009; Ballo et al., 2012). During ongoing bursting activity, different spikes of each burst have different conduction delays and there is a highly nonlinear relationship between the conduction delay and the spike number in the burst (Ballo and Bucher, 2009; Ballo et al., 2012). We used the natural activity pattern in our model to address two questions. First, we examined whether the natural activity changes the STS and FTS effects or whether these effects remain the same as during Poisson stimulations. Second, we asked if the FTS effect for the natural bursting activity could be as accurately predicted from paired-pulse stimulations as for Poisson stimulations.
Figure 7A shows traces of the model axon from the start and end of a 300 s stimulation with a burst frequency of 1 Hz. Action potentials were produced by stimulating the model with brief current pulses (see Materials and methods) and it captured the dynamics of voltage trajectories described in the experimental data (Ballo and Bucher, 2009). In particular, over the course of the entire stimulation, the baseline membrane potential between bursts slowly hyperpolarized by several millivolts. Within each burst, both the peak and the trough voltages changed. Peak voltages decreased with increasing Finst towards the middle of bursts and increased again with decreasing Finst towards the end, because of inactivation of INa. Trough voltages were more depolarized in the middle of bursts, because summation resulting from relatively slow spike repolarization is largest at high Finst.
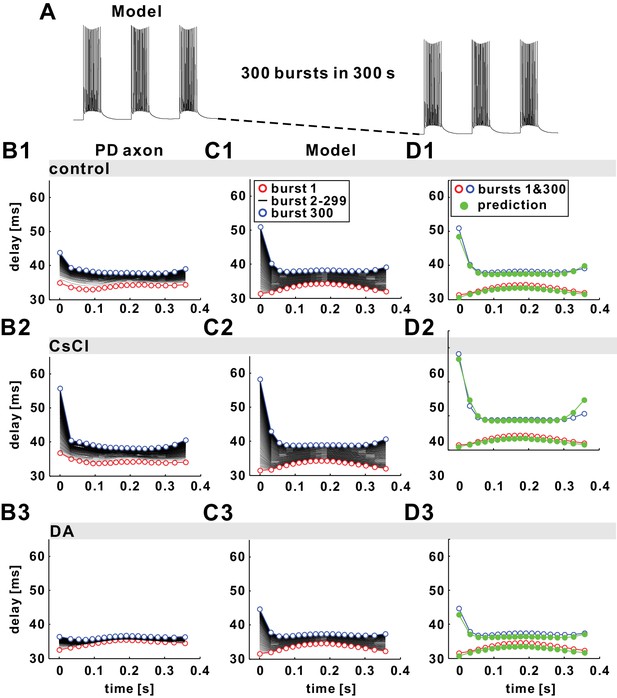
The fast timescale effect observed in the burst stimulations can be predicted by the paired-pulse stimulation results.
(A) The model responses to 300 parabolic burst stimuli (350 ms duration; 1 s period) over 300 s show a drop in baseline membrane potential. (B1–B3) Experimental results of conduction delay of spikes in each burst shown, superimposed as a function of time from the burst onset. Data of the first (red) and the 300th (blue) burst are colored. (C1–C3) Model replication of the experimental results, with different Ih levels (see Tables 1 and 2 for model parameters). (D1–D3) Simulation results of the first and the 300th burst in panel C1-C3 are predicted by estimating history-dependence using paired-pulse simulations (see Figure 6). Paired-pulse simulations were done with a constant low or high Ipump level, equal, respectively, to the Ipump mean value during the first and the 300th burst, fit with polynomial functions and used to obtain the plotted predictions (green).
Figure 7B1 shows PD axon conduction delays over the course of the burst duration for all 300 bursts in the 5 min stimulation, measured in one experiment. As in Poisson stimulations, Dmean and CV-D increased during the entire stimulation. Note that the delay of the first spikes in each burst, the ones occurring after an inter-burst interval of 650 ms, increases disproportionately. This corresponds to the large delays at small Finst as seen in Figure 2B for the FTS effect during Poisson stimulation. This increase in delay of the first spike in the burst was even more pronounced in the model (Figure 7C1).
In exploring spike conduction delay under natural bursting conditions, we also considered the effect of neuromodulation on history-dependence. As stated above, conduction delay in the PD axon is affected by the level of Ih, which is modulated by DA (Ballo et al., 2010, 2012). Figure 7B2 and B3 show that both the slow increase in mean delay and the variability of delay are more severe when Ih was blocked by CsCl, and less severe when Ih was increased by application of DA. In the model, these effects were mimicked when Ih was blocked (Figure 7C2) or increased (Figure 7C3). As with Poisson stimulations, the increase in the conduction delay of each subsequent burst was because of the slow increase of Ipump with stimulation (not shown), leading to a more hyperpolarized resting potential. This effect was counteracted to different degrees by different levels of Ih.
As with the FTS effect during Poisson stimulations, we examined whether the nonlinear relationships between conduction delay and time, seen in the burst stimulation of the biophysical model, can be predicted with paired-pulse stimulations at different levels of Ipump. A comparison of the paired-pulse estimates of these relationships for the first and last bursts (1 and 300) is shown in Figure 7D1–3 for the three conditions: control, Ih block and DA. The level of Ipump was set to the mean value of the dynamical Ipump at the time of burst 1 or the time of burst 300. The estimates for the paired-pulse stimulations shown in these panels were obtained from the polynomial fits (as in Figure 6B) and the ISIs of individual spikes. The nonlinear relationship between conduction delay and spike number was captured in all cases.
Predicting the history-dependence of conduction delay
In addition to exploring the history-dependence of conduction delay at a phenomenological level, we also investigated which ionic current dynamics could account for this history-dependence. In the PD axon, delay is not a simple linear function of spike trajectory measures like peak voltage, trough voltage, or duration (Ballo et al., 2012). A possible reason for this is the dependence of conduction velocity on changes in total membrane conductance, which because of opposing inward and outward currents are not necessarily apparent in voltage traces (Bucher and Goaillard, 2011). A number of theoretical studies have provided equations to describe conduction velocity of a single isolated spike. The Matsumoto-Tasaki equation provides an estimate of velocity as a function of the total conductance level at the peak of the spike (see Materials and methods), and has been verified experimentally for single spikes in the squid giant axon (Matsumoto and Tasaki, 1977; Tasaki and Matsumoto, 2002; Tasaki, 2004). If the history-dependence of conduction delay is because of changes in the total conductance level, the Matsumoto and Tasaki equation should be able to predict the STS and FTS effects seen in our simulations. We therefore used this equation to estimate the delay of each spike in the 300 s, 10 Hz Poisson stimulation of our model (as shown in Figure 2C,D).
Although the Matsumoto and Tasaki equation provided a good first-order estimate of conduction delay, it did not capture the STS effect in the model axon (Figure 8A1). Additionally, for the FTS effect, this equation only showed a decrease of delay at low Finst and did not capture the variability (Figure 8A2). In general, the overall ability of the Matsumoto and Tasaki equation to predict the conduction delay in our model was poor (Figure 8A3).
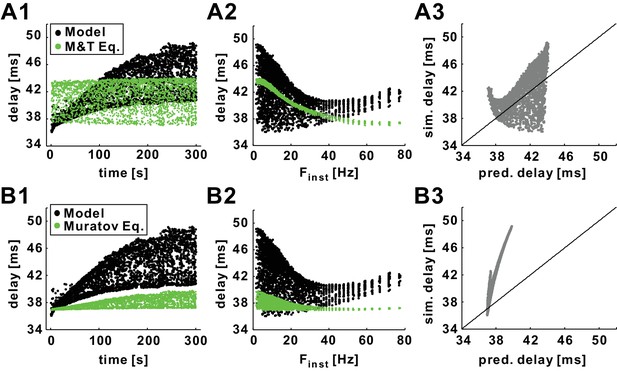
Predictions of history-dependence of conduction delay by previously published equations of action potential velocity.
(A1) Conduction delays of the biophysical model in response to 10 Hz Poisson stimulation and delays predicted by the Matsumoto and Tasaki equation (Tasaki and Matsumoto, 2002). (A2) Data in panel A1 plotted versus Finst. (A3) Simulation delay versus delay predicted by Matsumoto and Tasaki equation. (B1–B3) Comparison between simulation delays in the biophysical model (as in A1-C1) and the delays predicted by the Muratov equation (Muratov, 2000). Diagonal lines are y=x.
An alternative method for estimating conduction velocity in the Hodgkin-Huxley model axon is provided by the Muratov equation (Muratov, 2000). This estimate uses the values of the INa inactivation variable hNa and the INa activation rate , both evaluated at rest (see Materials and methods). Figure 8B shows a comparison of the delays in the Poisson stimulation of our model and the predicted delays using the Muratov equation. The predictions of delay by the Muratov equation were qualitatively similar to the simulation results for the STS effect, in that they showed an increase of Dmean and CV-D. However, the increases in these two factors were limited and the equation failed to provide a quantitatively accurate prediction (Figure 8B1). Additionally, for the FTS effect, the increase of delay with larger values of Finst was not captured by this equation (Figure 8B2). Therefore, the overall ability of this equation to predict the conduction delays in the model axon was also poor (Figure 8B3). In conclusion, although these equations provide good predictions for conduction delay of an isolated spike, neither could accurately predict the short- and long-term history-dependence of conduction delay of the model axon.
Sensitivity of the STS and FTS history-dependence of conduction delay to model parameters
Because previously published equations failed to predict accurately the history-dependence of conduction delay, we used an empirical approach to explore which model parameters contributed most to this history-dependence. Our model included five distinct ionic currents, each of which depended on a maximal conductance and reversal potential and, with the exception of ILeak, on parameters that determine the activation kinetics and, in the cases of INa and IA, inactivation kinetics. As a first step in understanding the contribution of these parameters to history-dependence of conduction delay, we examined the sensitivity of STS and FTS effects to changes in these parameters (see Materials and methods). The sensitivity was measured by examining how the different attributes that describe the STS and FTS effects (Figure 9A) depend on small changes in the parameter values. For the STS effect, these attributes were Dmean and CV-D. For the FTS effect, we used quadratic fits to determine the minimum delay (Dmin), the frequency (Fmin) at which Dmin occurred, and the curvature of the fit at this point (κmin).
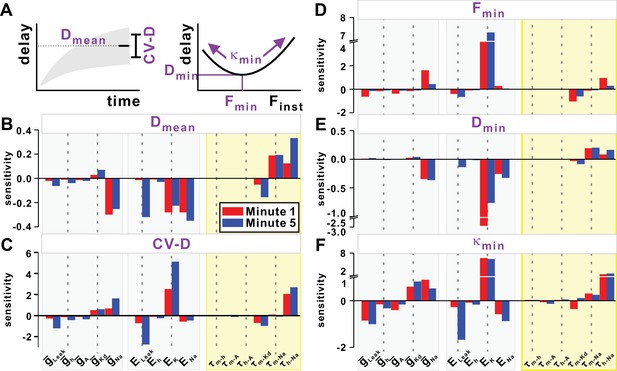
Sensitivity of the slow (STS) and fast timescale (FTS) effects to the model parameters.
(A) Top left inset schematically shows the mean (Dmean) and variability of conduction delay (CV-D = coefficient of variation), measured in the last 20 s of simulation. Top right inset schematically shows the minimum delay point (Fmin, Dmin) and the curvature κmin of the quadratic fit at this point. (B–C) STS effect. Sensitivity of Dmean and CV-D to model parameters in the first and five minute of a 10 Hz Poisson stimulation. (D–F) FTS effect. Sensitivity of Fmin, Dmin and κmin to model parameters in the first and fifth minute of a 10 Hz Poisson stimulation.
Note that a small sensitivity value does not imply that the model attribute is not dependent on the parameter, but rather that small changes in the parameter do not affect that attribute strongly or monotonically. The large effect of Ipump on conduction delay and history-dependence seen in Figure 3 was because of several-fold changes in pump activation over several minutes. In contrast, the comparatively small (±5% and±10%) changes of Ipump used here had little effect on sensitivity values (not shown). For all other parameters, we performed the sensitivity analysis at two different constant values of Ipump corresponding to the mean Ipump value at minute 1 (red) or 5 (blue) of the Poisson stimulation.
The primary result of the sensitivity analysis was that the parameters which produced the largest variations in any of the attributes of the STS (Figure 9B and C) or FTS (Figure 9D–9F) effect were those directly involved in spike generation, that is, the parameters of INa, IKd and ILeak. Although, as described above, Ih contributes greatly to the history-dependence of conduction delay, the different attributes of history-dependence were not greatly sensitive to the parameters of Ih, nor to that of the transient potassium current IA.
The value of Dmean (Figure 9B) strongly and negatively correlated with the equilibrium potential of K+ (EK) and the leak reversal potential (ELeak). This sensitivity was most likely because of the contribution of EK and ELeak to the resting membrane potential. Dmean also strongly and negatively depended on the equilibrium potential of sodium (ENa) and its maximum conductance (). This is consistent with the predictions of the Matsumoto-Tasaki equation, because an increase in either of these parameters results in an increase in gNa, and therefore total conductance. Additionally, Dmean strongly and positively depended on the activation and inactivation time constants of INa. The sensitivity of CV-D to model parameters was not as consistent as that observed for Dmean. Several parameters produced different (EK, ELeak, , ) effects on CV-D, when the Ipump value was low or high (Figure 9C).
Of the FTS attributes, Fmin was sensitive primarily to EK (Figure 9D). Although it is difficult to gain a clear intuition on how different parameters affect Fmin, this observation indicates that the fastest spikes can be obtained at a higher frequency if EK is shifted to a more depolarized value. Not surprisingly, the dependence of Dmin on the model parameters (Figure 9E) was similar to that of Dmean. The non-monotonic dependence of delay on the instantaneous stimulus frequency was captured primarily by the curvature of the quadratic fit. A larger curvature κmin implies a larger nonlinearity and therefore a larger difference between conduction delays at different Finst values. κmin is negatively dependent on ELeak, and ENa, but positively on , EK and (the time constant of INa inactivation, Figure 9F). These dependencies are consistent, but the reasons underlying their effect on κmin are not obvious.
The sensitivity analysis included two types of parameters: those with constant values (maximal conductances and reversal potentials), and those whose values depend on the membrane potential and can change with time (ionic current time constants). The latter parameters can change in value for each spike and therefore are the only ones that can potentially contribute to the history-dependence of conduction delay. Within this category of parameters, the FTS and STS effects showed the highest sensitivity to the time constants of INa.
History-dependence of conduction delay is dominated by two rate constants of the Na+ channel kinetics
The dependence of STS and FTS effects on the gating time constants of INa activation and inactivation, and , led us to explore whether the factors that determine these time constants were responsible for the history-dependence of conduction delay.
and are determined by four rates (Figure 10 inset): the opening and closing rates of activation, αm(V) and βm(V), and the opening and closing rate of inactivation, αh(V) and βh(V). We therefore examined how these rates changed for each spike during the Poisson stimulation. Because the opening rate is relevant at the onset of the spike and the closing rate during the spike itself, we evaluated αm and αh at the spike trough voltage (VT, the voltage minimum right before the spike), and βm and βh at the spike peak voltage (VP). In order to compare the changes in these parameters directly with those of conduction delay, we plotted the reciprocal values of the parameters, which have units of time.
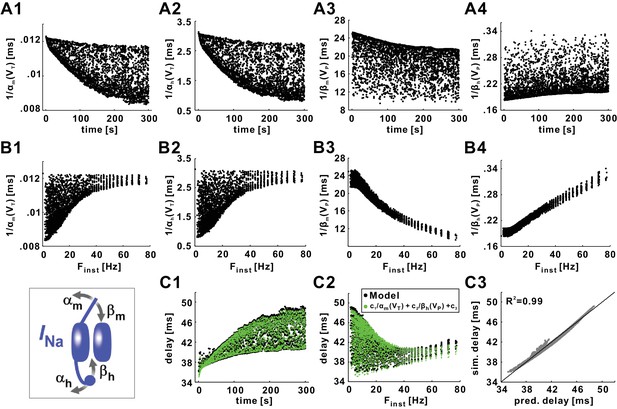
Conduction delay is determined by the INa activation and inactivation variables, evaluated at the action potential trough (VT) or peak (VP) voltage.
(A1–A2) Reciprocal of the INa activation rate, evaluated at VT. (A3–A4) Reciprocal of the INa inactivation rate, evaluated at VP. (B1–B4) Data in panel A plotted as a function of Finst. (C1) Conduction delay of the model compared with the predicted delay of c1/αm(VT)+c2/βh(VP)+c3 (c1 = −3361.48, c2 = 32.80, c3 = 70.14). (C2) Data in panel C1 plotted as a function of Finst. (C3) Simulation delays compared with predicted delays from the empirical equation (R2=0.99). The line is y=x. The inset is the schematic of the fast sodium channel.
All four parameters changed substantially during the Poisson stimulation and showed both STS and FTS effects (Figure 10A and B), but no single parameter followed exactly the STS and FTS effects on conduction delay. However, both 1/αm(VT) and 1/αh(VT), the (reciprocals of the) rates of activation and inactivation gates opening at the spike onset, showed an STS effect that was qualitatively similar to that of conduction delay (Figure 10A1 and A2); yet the STS effect for these parameters was decreasing rather than increasing, and neither parameter captured the non-monotonic FTS effect (Figure 10B1 and B2). In contrast, neither 1/βm(VP) nor 1/βh(VP), the (reciprocal) rates of activation and inactivation gates closing at the spike peak, qualitatively captured the increase in variance over time of the STS effect very well (Figure 10A3 and A4), and both these parameters showed a monotonic FTS effect (Figure 10B3 and B4). In summary, no single parameter could predict the overall time course of changes in conduction delay.
We next examined whether any combination of two parameters would better capture the STS and FTS effects. There are six possible combinations of two parameters out of these four. We fit the model STS and FTS effects with all six combinations. That is, for any combination of two parameters x and y out of the four, we fit the Poisson stimulation data of Figure 2C–D with the equation
The coefficients ci (i = 1,2,3) were determined with a Powell’s optimization method and the estimated delay was compared with the simulation results. We found that five of the combinations produced a fit to the model delay values that had a coefficient of determination R2 of >0.9, but only two of these qualitatively captured both the STS and FTS effects seen in Figure 2C–F (data not shown). These combinations were 1/αh(VT), 1/βh(VP), and 1/αm(VT), 1/βh(VP). Of these, the better fit was obtained from the combination that included both the activation and inactivation gating variables (R2 = 0.99 compared to 0.97). We therefore used the following empirical equation as the best estimate for the model conduction delay:
Figure 10C1 and C2 show that this equation captures both STS and FTS effects very well. Figure 10C3 shows the high accuracy with which this equation predicts the conduction delays in the model (R2 = 0.99). Although, for brevity, we do not show the dependence of conduction delay on αm(V) and βh(V), scaling these functions had a direct effect on the conduction delay. We therefore conclude that the opening rate of the activation gate of the sodium channel right before the spike, and the closing rate of the inactivation gate of the sodium channel at the peak of the spike are good proxies for, and likely important determinants of, the spike conduction velocity in axons.
History-dependence of conduction delay can be predicted from the action potential voltage trajectory
The equation to predict conduction delay from activation and inactivation variables served to gain insight into underlying mechanisms. However, it is of limited value for experimental work, because activation and inactivation variables cannot be measured during ongoing spiking. We therefore asked if there are good enough correlates of activation and inactivation variables to be found in the voltage trajectory of spikes that would allow the prediction of delay. Single spike measures like peak or trough voltages are poor predictors of delay in the PD axon (Ballo et al., 2012), but the dependence of delay on channel gating at both trough and peak shown here suggests that more than one measure has to be considered. We noticed that although αm and βh and are nonlinear functions, in the range of membrane potentials restricted to the trough or peak of the spike (VT and VP), both αm(VT) and βh(VP) were almost linear functions of their respective variables. We therefore treated VT and VP as linear approximations of 1/αm(VT) and 1/βh(VP), respectively, and used them instead in our equation:
With the same optimization process as before, the coefficients were determined for the Poisson stimulation data of the model axon. The resulting prediction of conduction delay captured the history-dependence of delays with 99% accuracy (Figure 11A1–A3). Furthermore, the equation with the same coefficient values predicted the delays in a novel set of Poisson stimulation data with the same accuracy (not shown).
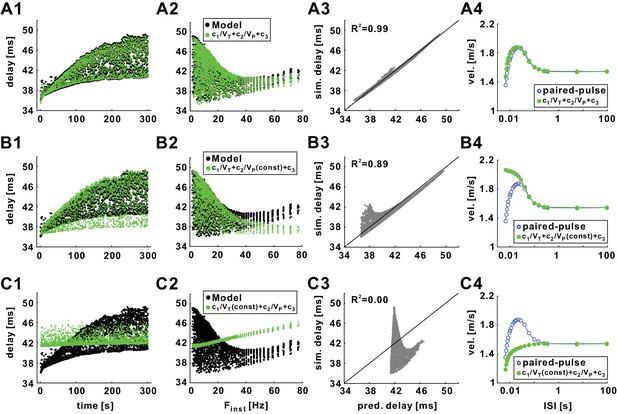
Conduction delay can be almost perfectly predicted by the trough and peak voltages of the action potentials.
(A1) Superimposed graph of conduction delays of the biophysical model (black) in response to 10 Hz Poisson stimulation and the predicted delays from the nonlinear regression equation: d = c1/VT +c2/VP +c3 (c1 = 4072.88, c2 = 302.36, c3 = 82.94; the same values of c1-c3 are used in all panels). (A2) Data in panel A1 plotted as a function of Finst. (A3) Simulation delays (training data) compared with predicted delays (R2 = 0.99). (A4) Conduction delays of the biological model (blue) in response to paired-pulse stimulation (same as in Figure 6A) and the predicted delays from the regression equation in panel A1. (B1–B3) Simulation results (same as in A1-A3) compared with predicted delays from the single variable regression: d = c1/VT +c2/VP (mean) +c3, where VP (mean) is the mean value of Vp. (B4) Simulation results (same as in A4) compared with predicted delays from the single variable regression: d = c1/VT +c2/VP (cond) +c3, where VP (cond) is the value of Vp of the conditioning pulse. (C1–C3) Simulation results (same as in A1-A3) compared with predicted delays from the single variable regression: d = c1/VT(mean)+c2/VP +c3, where VT (mean) is the mean value of VT. The lines in A3, B3, and C3 are y=x. (C4) Simulation results (same as in A4) compared with predicted delays from the single variable regression: d = c1/VT(cond)+c2/VP +c3, where VT (cond) is the value of VT of the conditioning pulse.
We also determined the contribution of each variable to the fit. To this end, we examined how well the simulated delays can be fit using only 1/VT or only 1/VP as the variable. In these fits, the other variable (VP or VT) was set to its mean value measured during the 300 s Poisson stimulation. The results of these fits are shown in Figure 11B and C. Neither variable perfectly captured the STS effect although this effect was better captured with fits using only 1/VT (Figure 11B1). This result is consistent with the sensitivity of Dmean to (see Figure 9B), because a larger results in a slower opening rate of INa activation variable at VT. Similarly, for the FTS effect, neither variable alone captured the non-monotonic relationship of delay with Finst; however, the decrease of delay with Finst was best approximated by 1/VT (Figure 11B2) whereas its increase was best approximated by 1/VP (Figure 11C2). Therefore, although neither 1/VT nor 1/VP can accurately predict conduction delay on their own, the multivariate linear regression using both1/VT and 1/VP provides an accurate prediction of history-dependence of conduction delay in the biophysical model.
Our description of delay changes suggests that they are dominated by the INa activation state before the spike and the inactivation state at the peak, and well reflected in the voltage values at these time points (Figure 10). We therefore predicted that, while other conductances can influence the gating state of INa through their effect on membrane potential, the recovery cycle should be accurately predicted by our equation describing the dependence of delay on trough and peak voltages. To test this prediction, we used the estimated delays from our equation to predict the results of the paired-pulse stimulations shown in Figure 6A (with Ipump,HI). As described above, the coefficients ci (i = 1,2,3) were independent of the stimulation pattern once the model axon was fixed. We therefore used coefficients obtained by a 1 min Poisson stimulation protocol to predict the conduction velocities of the spikes in the paired-pulse stimulation. With these coefficients and the VT and VP values, the conduction velocities were accurately predicted for both levels of Ipump (Figure 11A4).
The extent to which either VT or VP can separately predict delays during Poisson stimulation was discussed above (for Figure 11B and C). We also tested the extent to which VT and VP contributed to the different periods of the recovery cycle. We restricted this analysis to the paired-pulse simulation results for high levels of Ipump (Figure 11B4 and C4). In order to understand the contribution of VT to changed excitability at different intervals, we set VP to a constant value equal to the trough voltage of the conditioning spike in the paired-pulse stimulation. Using the coefficients from the Poisson stimulation and VT from the paired-pulse stimulation, the predicted conduction velocity decreased with increasing ISIs in the range of relative refractory and supernormal periods (Figure 11B4). Therefore, changes in VT captured the supernormal period. The decrease of velocity was consistent with the results for Poisson stimulations shown in Figure 11B2: predicted conduction delay decreased with Finst if only VT was used.
To capture the contribution of VP, we fixed the value of VT to a constant equal to the peak voltage of the conditioning spike in the paired-pulse stimulation. Again, using the same coefficients and the VP values from the paired-pulse stimulation, we found that the predicted conduction velocity monotonically increased with ISI (Figure 11C4). Thus the changes in VP captured the relative refractory period, which was consistent with the results shown in Figure 11C2: predicted conduction delay increased with Finst if only Vp was used.
History-dependence of experimentally measured conduction delay can be predicted from the voltage trajectory of spikes
Because both VT and VP are readily measured from experimental intracellular recordings (Ballo et al., 2012), we asked whether our equation can be used to predict the history-dependence of conduction delays in the PD axon. We used conduction delays measured with a Poisson stimulation at 10 Hz and fit the delay values with our equation. We then used this equation to predict the delay values of a novel dataset produced with the same stimulation rate. The results showed that we could predict both the STS and the FTS effects of the experimental data relatively accurately (Figure 12A–B). The fit produced a coefficient of determination of 0.87, indicating that 87% of the variance of the experimental delays can be predicted by this equation (Figure 12C). The history-dependence of conduction delay in experiments can therefore be predicted from the spike voltage trajectories without any need for computational modeling.
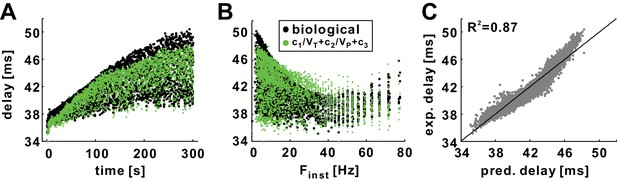
The history dependence of conduction delay in the biological PD axon can be predicted by the empirical equation without need for computational modeling.
(A) Conduction delays in the biological PD axon in response to a 5 min, 10 Hz, Poisson stimulation (black; same as in Figure 2A) compared with delays predicted by empirical equation (c1=4817.75, c2 = 500.5, c3 = 105) using VT and VP values from intracellular recordings (not shown). (B) Data in panel A plotted as a function of Finst. (C) Experimental delays plotted versus the predicted delays (R2 = 0.87). The line is y=x.
Discussion
The classic description of ionic mechanisms underlying spike generation and conduction was based on only two voltage-gated currents to describe the membrane behavior of the squid giant axon (Hodgkin and Huxley, 1952a, 1952b). This relatively simple model has dominated the common perception of axonal spike propagation, even though ionic mechanisms in most axons are more complex, as multiple currents with very different properties can be present (Krishnan et al., 2009; Bucher and Goaillard, 2011). Later theoretical work examined in detail the properties determining conduction velocity (Muratov, 2000; Tasaki, 2004), but only for single spikes and considering only a limited set of properties. We show that these models fail to capture the history-dependence of conduction velocity during repetitive activity. Such history-dependence is evident in findings that changes in axonal excitability and conduction velocity can occur at timescales far exceeding single inter-spike intervals, and can substantially alter the temporal pattern of spikes during propagation (Weidner et al., 2002; Ballo et al., 2012). It is important to understand the mechanisms underlying history-dependence of spike propagation, because changes in temporal patterns potentially play an important role in shaping the neural code (Izhikevich, 2006; Bucher and Goaillard, 2011; Bucher, 2015).
The dependence of conduction delay on slow activity-dependent hyperpolarization
We describe the history-dependence of conduction delay in a biophysically realistic model axon designed to match properties of the PD motor axon of the crustacean stomatogastric nervous system. The model captures well how delay changes as a function of prior spiking in this axon, at two different timescales (Ballo et al., 2012)(Figure 2).
At the slow timescale (STS effect), repetitive activity leads to cumulative hyperpolarization and conduction slowing. Over the course of minutes, mean delay and variability of delay increases. We show that the STS effect is due to the Na+/K+ pump (Figure 3). It has been proposed for many axons that slow hyperpolarization and slowing of conduction is caused by this pump, which is activated by the massive Na+ influx during repetitive spiking and causes a net deficit of positive charge (Van Essen, 1973; Gordon et al., 1990; Bostock and Bergmans, 1994; Robert and Jirounek, 1994; Vagg et al., 1998; Baker, 2000; Kiernan et al., 2004; Moldovan and Krarup, 2006; Scuri et al., 2007). Direct experimental evidence of the involvement of the pump in activity-dependent dynamics for the PD axon is missing because of the difficulty that pharmacological block of the pump also interferes with its overall role in maintaining a functional membrane potential (Ballo and Bucher, 2009). However, other slow outward currents have not been identified in the PD axon (Bucher, unpublished results).
We nevertheless considered a slowly activating outward current as an alternative mechanism because many axons express slow K+ channels, e.g. Kv7 channels that produce M-type currents (Dubois, 1981; Baker et al., 1987; Eng et al., 1988; Devaux et al., 2004; Schwarz et al., 2006; Vervaeke et al., 2006; Buniel et al., 2008). Such currents are thought to contribute to hyperpolarization and reduced excitability and may have cumulative effects during repetitive activity (Baker et al., 1987; Taylor et al., 1992; Stys and Waxman, 1994; Miller et al., 1995; Lin et al., 2000; Burke et al., 2001; Schwarz et al., 2006). An interesting difference between the pump current and currents due to ion channel conductances is that the pump current does not depend on a driving force. Equivalently, an ionic current would change the input conductance of the neuron, whereas the pump current does not. We found that even with a low activation threshold and slow kinetics, the slow voltage-gated K+ current cannot cause substantial activity-dependent hyperpolarization because an EK relatively close to the resting membrane potential rendered the driving force in between spikes too small for substantial summation of current (Figure 4). The same would be true for Cl- conductances and ECl. Yet, this slow K+ current reduced the peak voltage of action potentials and reproduced the effect of the pump current in increasing the mean delay Dmean, but not the variability CV-D.
It should be noted, however, that we used an EK value (−70 mV) in our axon model that is about 10 mV less negative than usually assumed for soma recordings of STG neurons (Golowasch and Marder, 1992). A sufficiently slow voltage-gated K+ with EK substantially lower than the resting potential could possibly reproduce the effect of the pump current in producing the STS effect. We chose the EK value near the resting potential because spikes in PD axon recordings show an absence of fast after-hyperpolarization (undershoot), and we could only replicate this in the model with a less negative EK. An alternative approach would have been to model EK as a dynamic variable and allow it to transiently change to more depolarized values over the course of a single spike. This type of dynamic EK was used in a model of the squid giant axon, which assumed a significant temporary extracellular K+ accumulation, and was successful in rendering a more realistic voltage trajectory of repolarization and undershoot (Clay, 1998). As the PD axon does not actually express slow K+ currents, we did not pursue this further. However, our results demonstrate that the increase of variability of delay was dependent on hyperpolarization, as a mere increase in conductance only increased the mean delay.
The dependence of the STS effect on hyperpolarization is further highlighted by the role of Ih (Figure 5), which is thought to play an important role in preventing spike failures in many axons because its inwardly rectifying properties can balance activity-dependent hyperpolarization (Baker et al., 1987; Grafe et al., 1997; Soleng et al., 2003; Kiernan et al., 2004; Baginskas et al., 2009; Tomlinson et al., 2010). In the PD axon, Ih is increased several-fold by dopamine (Ballo et al., 2010), which substantially reduces hyperpolarization and history-dependence of conduction delay (Ballo et al., 2012). Our model provides a mechanistic explanation of our previous experimental observation that the balance of Ih and pump current plays a dynamic role in setting how sensitive conduction is to the history of activity. This represents a level of importance in controlling temporal fidelity that goes beyond just preventing spike failures.
Previous studies have also used a modeling approach to explore the role of slow accumulation of ionic currents and concentrations in axons. The topics explored included explorations of Na+ accumulation and the effects of the Na+/K+ pump in the coupled left-shift of the voltage-dependence of Na+ channels due to axonal injury (Boucher et al., 2012; Yu et al., 2012; Lachance et al., 2014), the influence of Na+ channel clustering on spike conduction (Freeman et al., 2016) and activity-dependent slowing of spike conduction (Tigerholm et al., 2014).
The dependence of conduction delay on spike intervals
The model also captures well the PD axon’s nonlinear and non-monotonic dependence of delay on instantaneous frequency (FTS effect), the manifestation of which is influenced by the STS effect. It has long been known that, with increasing spike intervals even well beyond the refractory period, excitability and conduction velocity go through an oscillation of decreases and increases, (Bullock, 1951; Raymond, 1979), collectively termed the recovery cycle. Such activity-dependent changes are often gauged with paired-pulse stimulations (Bucher and Goaillard, 2011), particularly for diagnostic purposes in the context of peripheral neuropathies (Bostock and Rothwell, 1997; Bostock et al., 1998; Burke et al., 2001; Krishnan et al., 2009). The dependence on spike interval changes dramatically when axons are stimulated with trains of conditioning pulses instead of simple paired pulses. In our model, prior activity can be mimicked by setting the pump current to different values (Figure 6). This suggests that FTS and STS effects are completely separated in their time dependence, meaning that delay depends on the last interval and the mean rate of prior activity, but not on the exact temporal pattern of the last few preceding spikes. This independence of exact prior pattern is further demonstrated by our finding that paired-pulse stimulation results predict delays from both Poisson (Figure 6) and burst (Figure 7) stimulations. A previous modeling study on primary afferent C-fibers has also demonstrated an activity-dependent alteration of recovery cycles (Tigerholm et al., 2014).
The dependence of conduction delay on Na+ channel gating variables and other currents
Our results show that the dynamics of conduction delay can be accurately predicted from two parameters describing the state of the Na+ conductance at two time points, the opening rate of the activation gate at the onset of the spike, and the closing rate of the inactivation gate at the peak (Figure 10). In comparison, two equations that accurately describe conduction delay of single spikes fail to capture the dynamics. The Matsumoto-Tasaki equation predicts conduction delay dependent on the change in total membrane conductance elicited by a spike (Matsumoto and Tasaki, 1977; Tasaki and Matsumoto, 2002; Tasaki, 2004). The Muratov equation includes Na+ channel gating variables, but only at the rest state (Muratov, 2000). Both equations aim to predict absolute values of delay and include constant biophysical parameters such as diameter and membrane capacitance. In contrast, our empirical equation describes only how conduction delay changes as a function of activity. The magnitude of the delay changes and the absolute range of values are found by scaling the contribution of the two Na+ gating rates with two constants and adding a third. These three constants have to be found empirically and may represent stand-ins for actual biophysical parameters.
Interestingly, the gating parameters at the trough potential before a spike and the peak voltage of the spike correlate linearly with these voltages, so that the voltages can be treated as stand-ins for the gating parameters in the empirical fit, which allows us to predict delay solely from voltage trajectories (Figure 11). This illustrates the important point that, while both our sensitivity analysis (Figure 9) and the empirical prediction of delays seem to suggest that Na+ channel gating is the key factor, the rates used to predict delay are voltage-dependent and are therefore sensitive to the effect other ionic mechanisms have on the membrane potential trajectory.
It should be noted that the small level of sensitivity to the various currents is because sensitivity analysis only examines small changes in the parameters of these currents which would not greatly alter the membrane potential. However, some of these currents, as shown for the pump current and Ih, undergo large changes that have substantial effects on delay. Again, these mechanisms act at two different timescales. At the slow timescale, the balance of pump current and Ih determines the overall level of hyperpolarization and thus affects Na+ channel gating through the steady-state voltage-dependencies of the opening and closing rates. It should be noted that changes in baseline membrane potential overall can have ambiguous effects on conduction velocity. For example, slow hyperpolarization is often associated with slowing of conduction but can also increase removal of Na+ channel inactivation and therefore increase velocity (Vervaeke et al., 2006; De Col et al., 2008).
At the fast timescale, K+ currents play an important role in shaping the repolarization speed, after-potentials, and re-excitability, the extent of which critically depends on the gating time constants and temporal overlap with the Na+ current (Erisir et al., 1999; Baranauskas, 2007; Sengupta et al., 2010). K+ currents with different gating time constants may differentially affect both Na+ channel activation and its inactivation. Of note is the common presence of inactivating and low-threshold K+ currents in axons (Krishnan et al., 2009; Bucher and Goaillard, 2011; Debanne et al., 2011; Bucher, 2015), because their own activation and inactivation states will be critically dependent on slow timescale changes in baseline membrane potential and therefore change their effect on voltage trajectories and Na+ channel gating. In the PD axon, relatively slow spike repolarization leads to summation even at moderate frequencies, and spike duration depends on IA activation and inactivation, which vary substantially with the baseline membrane potential (Ballo and Bucher, 2009).
The relevance of the findings to other axons
The model presented here aimed to mimic, as faithfully as possible, the behavior of a well described unmyelinated invertebrate axon. However, it should be noted that the ionic mechanisms considered, like pump-mediated hyperpolarization, inward rectification through Ih, and the presence of both transient and sustained K+ currents, are found in many axons across different phyla (Bucher and Goaillard, 2011). In contrast, diversity of axon properties within a single species or even a single part of the nervous system can be very large, which makes the notion of a ‘typical’ axon appear not useful (Bucher, 2015). Nevertheless, spikes in the overwhelming majority of axons are driven by fast transient Na+ currents responsible for their regenerative nature. It is plausible as a general mechanism that the gating state of these channels at the onset and peak of the spike acts as a critical determinant of conduction velocity. Different complements of additional voltage-gated or electrogenic transporter currents across axon types may lead to very different dependence of conduction velocity on preceding activity. Yet, we expect that their influence on the Na+ channel gating variables, or trough and peak voltages as their proxies, would still serve to determine conduction velocity in each case. Consequently, once the peak and trough voltages of the action potential have been measured, the empirical equation introduced in this study would serve to accurately predict the conduction velocity, independent of the combinations of ionic mechanisms involved.
Materials and methods
The biophysical axon model
Request a detailed protocolWe constructed a conductance-based biophysical axon model to examine the role of different ionic currents in shaping the history-dependence of conduction delay. All simulations were done in NEURON (Carnevale and Hines, 2006). The model was based on standard cable equations for an unmyelinated axon, standard Hodgkin-Huxley type ionic conductances with different voltage- and time-dependencies, and a pump current representing the Na+/K+-ATPase:
The parameter a is the radius (=5 μm) of the model axon, Ri (=80 Ωcm) is the specific intracellular resistivity, V is voltage, x is the position along the axon, and Cm (=1 μF/cm2) is the membrane capacitance per unit area. The ionic currents (ΣIion), the Na+/K+ pump current (Ipump), and the stimulus current () are described below. A specific membrane resistivity Rm value of 8 KΩcm2 was used to calculate the leak conductance, resulting in a passive length constant λ = 1581 μm. Both a and λ were similar to the values found in the PD axon (Ballo and Bucher, 2009). The length of the model axon was set to 1 cm, and divided into 101 identical compartments for simulation. In order to apply the finite difference method to solve the model equations numerically, all compartments were assumed to be isopotential during the simulation process. All axon parameters are listed in Table 1.
Ionic currents
Request a detailed protocolIonic currents used in the model are shown in Figure 1A. Different versions of the model contained different combinations of ionic currents (currents not included in all versions are shown in brackets):
In the simplest version, it included only the standard Hodgkin-Huxley-like leak (ILeak), fast sodium (INa), and delayed-rectifier potassium (IKd) currents.
The PD axon expresses two additional voltage-gated ionic currents, a transient potassium current IA, and a hyperpolarization-activated inward current Ih (Ballo and Bucher, 2009; Ballo et al., 2010). Ih is increased by dopamine (DA, Figure 1A) through a D1-type receptor mechanism that increases cAMP levels (Ballo et al., 2010). We mimicked DA effects on Ih by using different values of voltage half-activation and slope (Table 2) that were described experimentally (Ballo et al., 2010). However, these changes alone were not sufficient to produce the experimentally-observed effect of Ih increase by DA on axonal delay, presumably because of differences in resting membrane potential between our model and the biological neuron. To match the biological results, we also assumed that DA increased (Table 1). To mimic block of Ih, was set to 0.
We also tested the effect of a slow potassium current (IKs). To this end, we introduced a conductance with the same voltage dependence as IKd, but a 5000 times slower time constant of activation.
All ionic currents were Hodgkin-Huxley type (Hodgkin and Huxley, 1952b) and were represented by equations of the following form:
where is the maximum conductance, Erev is the reversal potential, m and n are the activation and inactivation variables, respectively, and p and q are non-negative integers. As stated above, gating parameters and Erev for Ih were based on actual measurements in the PD axon (Ballo et al., 2010). INa and potassium current parameters were based on common values and tuned to produce spike shapes that matched those in intracellular PD axon recordings (Ballo and Bucher, 2009). Of note in these recordings is the absence of an undershoot following a spike. We only managed to replicate this by setting the K+ equilibrium potential EK to a fairly depolarized value of −70 mV (see Discussion). All gating parameters are listed in Table 2.
The Na+/K+ pump
Request a detailed protocolThe Na+/K+ pump current was modeled in a simplified form as described previously (Angstadt and Friesen, 1991), and was governed by the following equation:
where Imax,pump is the maximum current, [Na+]in the intracellular sodium concentration, [Na+]1/2 the concentration at which the pump is half active, and 1/[Na+]S describes the sensitivity of the pump to alterations of intracellular sodium concentrations. The rate of change for [Na+]in was governed by:
where INa is the level of sodium current, F is Faraday’s constant, Vol is the volume of one compartment of the model axon and α is a scale factor. According to this equation, at steady state, Ipump is equal to one third of INa. Because of their comparatively small magnitude, the contributions of ILeak and Ih to [Na+]in were ignored and changes in [Na+]in were modeled to depend solely on INa. The equilibrium potential of Na+ in the model axon was calculated according to the change in intracellular sodium concentration from the Nernst equation:
In our model, we made the simplifying assumption that the values of [Na+]out and the K+ concentrations inside and outside the axon were not changed by activity.
We also tried other, more descriptive, models of the Na+/K+ pump (Cressman et al., 2009; Yao et al., 2011) in our simulations. These provided somewhat different dynamics but produced very similar qualitative and quantitative results, which for brevity are not shown.
Axon stimulation
Request a detailed protocolSpikes were generated in the axon by applying a stimulus current in the first compartment:
where ti and dt (1 ms) are the stimulus time and duration, respectively. Spikes propagated along the length of the 1 cm axon and were recorded at two sites (0.3 and 0.7 cm; Figure 1B). The conduction delay was measured in NEURON using the time that the spike crossed the voltage threshold of −40 mV at the two recording sites. (Delays measured using action potential peak times produce practically identical results.) Delays produced along this 0.4 cm of axon were multiplied by a factor of 9.5 to mimic the conduction delays and velocities of the 4–5 cm biological axon shown in our results for comparison. Different conduction velocities of consecutive spikes lead to gradually changing intervals over distance. Because axonal excitability changes between spikes depend on interval, conduction delays during repetitive spiking do not scale linearly with distance (Kocsis et al., 1979; Bucher and Goaillard, 2011; Bucher, 2015). However, at initial spike frequencies smaller than about 100 Hz, these nonlinearities are relatively subtle and only differentially affect delays over distances of tens of centimeters (Moradmand and Goldfinger, 1995; Bucher and Goaillard, 2011). We confirmed that the conduction delay for a longer model axon, measured at the same relative points, scaled reasonably linearly with the length of the axon.
Stimulation patterns
Request a detailed protocolWe characterized the history-dependence of conduction delay with three different stimulus regimes that have been used before in the PD axon (Ballo et al., 2012). First, to describe delay as a function of time and stimulus frequency, we used 300 s Poisson stimulation protocols with different mean rates: 5, 10 and 19 Hz. The last value was chosen to match mean spike frequency in burst stimulation protocols (see below). It should be noted that the Poisson stimulation method is useful in producing a variety of inter-stimulus intervals, but this can be also achieved with other stimulation paradigms that incorporate such a variety, for example through rate adaptation.
Second, we used a paired-pulse protocol to obtain ‘recovery cycle’ measurements (Krishnan et al., 2009; Bucher and Goaillard, 2011). After a single conditioning stimulus, test stimuli were delivered at different intervals (between 10 ms and 10 s). The change of delay or conduction velocity was then analyzed as a function of stimulus interval.
Third, we used a burst stimulation protocol that mimicked normal ongoing rhythmic firing in the PD neuron (Ballo and Bucher, 2009; Ballo et al., 2012). It consisted of 300 bursts at 1 Hz burst frequency, and each burst consisted of 19 spikes (360 ms burst duration) in a parabolic instantaneous frequency structure, increasing from 32 Hz to 63 Hz and decreasing back to 32 Hz.
All simulations were run beginning with a 100 s interval of no stimulation to remove any transient and initial condition effects.
Equations for predicting conduction velocity
Request a detailed protocolTwo equations have been shown to provide good approximations for conduction velocity of a single spike in the squid giant axon and Hodgkin-Huxley model axon. We tested if these equations yielded good estimates of conduction velocities during repetitive spiking. The first is derived based on boundary matching principles which we will refer to as the Matsumoto-Tasaki equation (Matsumoto and Tasaki, 1977; Tasaki and Matsumoto, 2002; Tasaki, 2004):
In this equation, v is the conduction velocity, d is the diameter of the axon, Rtotal is the total resistance of the membrane of unit area in the excited state, Ri is the axial resistivity of the axon interior, Cm is the membrane capacitance per unit area and κ is defined as the ratio of Rtotal to Rrest, which is the resistance of the membrane of unit area at rest. In our evaluations, as in those done by Matsumoto and Tasaki, the value of κ was consistently close to 0 and did not make any demonstrable difference in the estimations. We therefore followed Matsumoto and Tasaki’s approach and used the simplified equation
The second equation is an explicit analytical expression developed for calculating conduction velocity in the Hodgkin-Huxley model (Muratov, 2000), which we will refer to here as the Muratov equation:
where Ri and Cm are as above, r is the radius of the axon, is the maximum fast sodium conductance, h0 is the value of the inactivation variable h of gNa at the rest state and
where ENa is the sodium equilibrium potential and Vrest is the resting membrane potential. To use this equation for repetitive spiking, we used the trough voltage before each spike (the local minimum voltage immediately before the spike) as a proxy for the resting membrane potential.
Sensitivity analysis
Request a detailed protocolThe sensitivity of conduction delay in the model to parameters was measured by examining how the different attributes that describe the slow- (STS) and fast-timescale (FTS) effects depend on small changes in the parameter values. For the STS effect, these attributes were the mean conduction delay and its coefficient of variation, measured for the final 20 s interval of simulation. For the FTS effect, we used quadratic fits to determine the minimum delay and the frequency at which it occurred, and the curvature of the quadratic fit at this point. To measure sensitivity, each parameter in the (reference) model was decreased or increased by 5% and 10% of its original value while other parameters were kept unchanged. It should be noted that ENa is a dynamic parameter, which is determined by the intracellular and extracellular concentration of Na+. Therefore, the scaling factors were applied to the Nernst equation for Na+ in order to rescale ENa.
The 5% and 10% changes in each direction produced four new models for each parameter. Each new model was subjected to the same Poisson stimulation as the original model and the attributes of the fast and slow timescale effects were measured. We then normalized these measures by their values in the reference model. The resulting four data points, together with the reference value (at the origin) were fit with a line. The sensitivity of an attribute to the parameter was defined as the slope of the linear fit. For example, a sensitivity value of 1 meant that a ± 5% and±10% change in the parameter resulted in a ± 5% or±10% change in the attribute.
Experimental data
Request a detailed protocolFor comparison of model results with experimental results, as well as for testing the empirical method of predicting conduction delay, we used experimental delay data from the PD axon obtained in our previous study (Ballo et al., 2012).
Optimization
Request a detailed protocolAll optimization was performed using Powell’s conjugate direction method (Powell's method; Powell, 1994), implemented in Matlab.
References
-
Analog signalling in mammalian cortical axonsCurrent Opinion in Neurobiology 18:314–320.https://doi.org/10.1016/j.conb.2008.08.006
-
Synchronized oscillatory activity in leech neurons induced by calcium channel blockersJournal of Neurophysiology 66:1858–1873.
-
The H-current secures action potential transmission at high frequencies in rat cerebellar parallel fibersEuropean Journal of Neuroscience 29:87–96.https://doi.org/10.1111/j.1460-9568.2008.06566.x
-
Axonal flip-flops and oscillatorsTrends in Neurosciences 23:514–519.https://doi.org/10.1016/S0166-2236(00)01645-3
-
Complex intrinsic membrane properties and dopamine shape spiking activity in a motor axonJournal of Neuroscience 29:5062–5074.https://doi.org/10.1523/JNEUROSCI.0716-09.2009
-
Dopamine modulates Ih in a motor axonJournal of Neuroscience 30:8425–8434.https://doi.org/10.1523/JNEUROSCI.0405-10.2010
-
Dopamine modulation of ih improves temporal fidelity of spike propagation in an unmyelinated axonJournal of Neuroscience 32:5106–5119.https://doi.org/10.1523/JNEUROSCI.6320-11.2012
-
Ionic channel function in action potential generation: current perspectiveMolecular Neurobiology 35:129–150.https://doi.org/10.1007/s12035-007-8001-0
-
Latent addition in motor and sensory fibres of human peripheral nerveThe Journal of Physiology 498 (Pt 1):277–294.https://doi.org/10.1113/jphysiol.1997.sp021857
-
Coupled left-shift of Nav channels: modeling the Na⁺-loading and dysfunctional excitability of damaged axonsJournal of Computational Neuroscience 33:301–319.https://doi.org/10.1007/s10827-012-0387-7
-
BookContribution of axons to short-term dynamics of neuronal communicationIn: Rockland K. S, editors. Axons and Brain Architecture (1st Edn). Boston: Elsevier Science. pp. 245–263.
-
Facilitation of conduction rate in nerve fibersThe Journal of Physiology 114:89–97.https://doi.org/10.1113/jphysiol.1951.sp004605
-
Distribution of voltage-gated potassium and hyperpolarization-activated channels in sensory afferent fibers in the rat carotid bodyThe Journal of Comparative Neurology 510:367–377.https://doi.org/10.1002/cne.21796
-
Excitability of human axonsClinical Neurophysiology 112:1575–1585.https://doi.org/10.1016/S1388-2457(01)00595-8
-
Excitability of the squid giant axon revisitedJournal of Neurophysiology 80:903–913.
-
The influence of sodium and potassium dynamics on excitability, seizures, and the stability of persistent states: I. single neuron dynamicsJournal of Computational Neuroscience 26:159–170.https://doi.org/10.1007/s10827-008-0132-4
-
Information processing in the axonNature Reviews Neuroscience 5:304–316.https://doi.org/10.1038/nrn1397
-
On increasing the velocity of a nerve impulseThe Journal of Physiology 148:665–670.https://doi.org/10.1113/jphysiol.1959.sp006315
-
KCNQ2 is a nodal K+ channelJournal of Neuroscience 24:1236–1244.https://doi.org/10.1523/JNEUROSCI.4512-03.2004
-
Evidence for the existence of three types of potassium channels in the frog ranvier node membraneThe Journal of Physiology 318:297–316.https://doi.org/10.1113/jphysiol.1981.sp013865
-
Development of 4-AP and TEA sensitivities in mammalian myelinated nerve fibersJournal of Neurophysiology 60:2168–2179.
-
Function of specific K(+) channels in sustained high-frequency firing of fast-spiking neocortical interneuronsJournal of Neurophysiology 82:2476–2489.
-
Mechanisms of sodium channel clustering and its influence on axonal impulse conductionCellular and Molecular Life Sciences 73:723–735.https://doi.org/10.1007/s00018-015-2081-1
-
Changes in interspike interval during propagation: quantitative descriptionBiological Cybernetics 26:209–213.https://doi.org/10.1007/BF00366592
-
Ionic currents of the lateral pyloric neuron of the stomatogastric ganglion of the crabJournal of Neurophysiology 67:318–331.
-
Function of the hyperpolarization-activated inward rectification in nonmyelinated peripheral rat and human axonsJournal of Neurophysiology 77:421–426.
-
Propagation of electrical signals along giant nerve fibersProceedings of the Royal Society B: Biological Sciences 140:177–183.https://doi.org/10.1098/rspb.1952.0054
-
A note on conduction velocityThe Journal of Physiology 125:221–224.https://doi.org/10.1113/jphysiol.1954.sp005152
-
Polychronization: computation with spikesNeural Computation 18:245–282.https://doi.org/10.1162/089976606775093882
-
Spike initiation and propagation on axons with slow inward currentsBiological Cybernetics 68:209–214.https://doi.org/10.1007/BF00224853
-
Differences in activity-dependent hyperpolarization in human sensory and motor axonsThe Journal of Physiology 558:341–349.https://doi.org/10.1113/jphysiol.2004.063966
-
The analysis of nonlinear synaptic transmissionThe Journal of General Physiology 70:243–265.https://doi.org/10.1085/jgp.70.2.243
-
Axonal ion channels from bench to bedside: a translational neuroscience perspectiveProgress in Neurobiology 89:288–313.https://doi.org/10.1016/j.pneurobio.2009.08.002
-
Stimulation-induced ectopicity and propagation windows in model damaged axonsJournal of Computational Neuroscience 37:523–531.https://doi.org/10.1007/s10827-014-0521-9
-
A study of conduction velocity in nonmyelinated nerve fibersBiophysical Journal 20:1–13.https://doi.org/10.1016/S0006-3495(77)85532-X
-
Evaluation of Na+/K+ pump function following repetitive activity in mouse peripheral nerveJournal of Neuroscience Methods 155:161–171.https://doi.org/10.1016/j.jneumeth.2005.12.015
-
Computation of long-distance propagation of impulses elicited by Poisson-process stimulationJournal of Neurophysiology 74:2415–2426.
-
Sensory neural codes using multiplexed temporal scalesTrends in Neurosciences 33:111–120.https://doi.org/10.1016/j.tins.2009.12.001
-
BookA direct search optimization method that models the objective and constraint functions by linear interpolationIn: Gomez S, Hennart J. -P, editors. Advances in Optimization and Numerical Analysis. Dordrecht: Springer. pp. 51–67.
-
Effects of nerve impulses on threshold of frog sciatic nerve fibresThe Journal of Physiology 290:273–303.https://doi.org/10.1113/jphysiol.1979.sp012771
-
Uptake of potassium by nonmyelinating schwann cells induced by axonal activityJournal of Neurophysiology 72:2570–2579.
-
The axon as a unique computational unit in neuronsNeuroscience Research 75:83–88.https://doi.org/10.1016/j.neures.2012.12.004
-
KCNQ channels mediate IKs, a slow K+ current regulating excitability in the rat node of RanvierThe Journal of Physiology 573:17–34.https://doi.org/10.1113/jphysiol.2006.106815
-
Inhibition of Na+/K+ ATPase potentiates synaptic transmission in tactile sensory neurons of the leechEuropean Journal of Neuroscience 25:159–167.https://doi.org/10.1111/j.1460-9568.2006.05257.x
-
Action potential energy efficiency varies among neuron types in vertebrates and invertebratesPLoS Computational Biology 6:e1000840.https://doi.org/10.1371/journal.pcbi.1000840
-
A method for decoding the neurophysiological spike-response transformJournal of Neuroscience Methods 184:337–356.https://doi.org/10.1016/j.jneumeth.2009.07.034
-
Synaptic modulation of the interspike interval signatures of bursting pyloric neuronsJournal of Neurophysiology 89:1363–1377.https://doi.org/10.1152/jn.00732.2002
-
On the cable theory of nerve conductionBulletin of Mathematical Biology 64:1069–1082.https://doi.org/10.1006/bulm.2002.0310
-
On the conduction velocity of nonmyelinated nerve fibersJournal of Integrative Neuroscience 3:115–124.https://doi.org/10.1142/S0219635204000415
-
Physiological evidence for a slow K+ conductance in human cutaneous afferentsThe Journal of Physiology 453:575–589.https://doi.org/10.1113/jphysiol.1992.sp019245
-
Modeling activity-dependent changes of axonal spike conduction in primary afferent C-nociceptorsJournal of Neurophysiology 111:1721–1735.https://doi.org/10.1152/jn.00777.2012
-
Activity-dependent hyperpolarization of human motor axons produced by natural activityThe Journal of Physiology 507 (Pt 3):919–925.https://doi.org/10.1111/j.1469-7793.1998.919bs.x
-
Integrative properties and design principles of axonsInternational Review of Neurobiology 18:1–40.https://doi.org/10.1016/S0074-7742(08)60032-X
-
Neural signal processing: the underestimated contribution of peripheral human C-fibersJournal of Neuroscience 22:6704–6712.
-
A continuum neuronal model for the instigation and propagation of cortical spreading depressionBulletin of Mathematical Biology 73:2773–2790.https://doi.org/10.1007/s11538-011-9647-3
Article and author information
Author details
Funding
National Institute of Neurological Disorders and Stroke (NS083319)
- Dirk Bucher
- Farzan Nadim
National Institute of Mental Health (MH060505)
- Farzan Nadim
National Institute of Neurological Disorders and Stroke (NS058825)
- Dirk Bucher
The funders had no role in study design, data collection and interpretation, or the decision to submit the work for publication.
Acknowledgements
Supported by NIH grants NS083319, MH60605 and NS058825.
Copyright
© 2017, Zhang et al.
This article is distributed under the terms of the Creative Commons Attribution License, which permits unrestricted use and redistribution provided that the original author and source are credited.
Metrics
-
- 1,304
- views
-
- 222
- downloads
-
- 17
- citations
Views, downloads and citations are aggregated across all versions of this paper published by eLife.
Citations by DOI
-
- 17
- citations for umbrella DOI https://doi.org/10.7554/eLife.25382






