1. Cell Biology
  2. Physics of Living Systems

Distinct roles of nonmuscle myosin II isoforms for establishing tension and elasticity during cell morphodynamics

  1. Kai Weißenbruch  Is a corresponding author
  2. Justin Grewe
  3. Marc Hippler
  4. Magdalena Fladung
  5. Moritz Tremmel
  6. Kathrin Stricker
  7. Ulrich Sebastian Schwarz  Is a corresponding author
  8. Martin Bastmeyer  Is a corresponding author
  1. Zoological Institute, Karlsruhe Institute of Technology (KIT), Germany
  2. Institute of Functional Interfaces (IFG), Karlsruhe Institute of Technology (KIT), Germany
  3. Institute for Theoretical Physics, University of Heidelberg, Germany
  4. BioQuant-Center for Quantitative Biology, University of Heidelberg, Germany
  5. Institute of Applied Physics, Karlsruhe Institute of Technology (KIT), Germany
  6. Institute for Biological and Chemical Systems - Biological Information Processing (IBCS-BIP), Karlsruhe Institute of Technology (KIT), Germany
https://doi.org/10.7554/eLife.71888
Share this article
Cite this article
  1. Kai Weißenbruch
  2. Justin Grewe
  3. Marc Hippler
  4. Magdalena Fladung
  5. Moritz Tremmel
  6. Kathrin Stricker
  7. Ulrich Sebastian Schwarz
  8. Martin Bastmeyer
(2021)
Distinct roles of nonmuscle myosin II isoforms for establishing tension and elasticity during cell morphodynamics
eLife 10:e71888.
https://doi.org/10.7554/eLife.71888
  2. Download BibTeX
  3. Download .RIS
10 figures, 4 tables and 3 additional files

Figures

Figure 1 with 4 supplements
Download asset Open asset
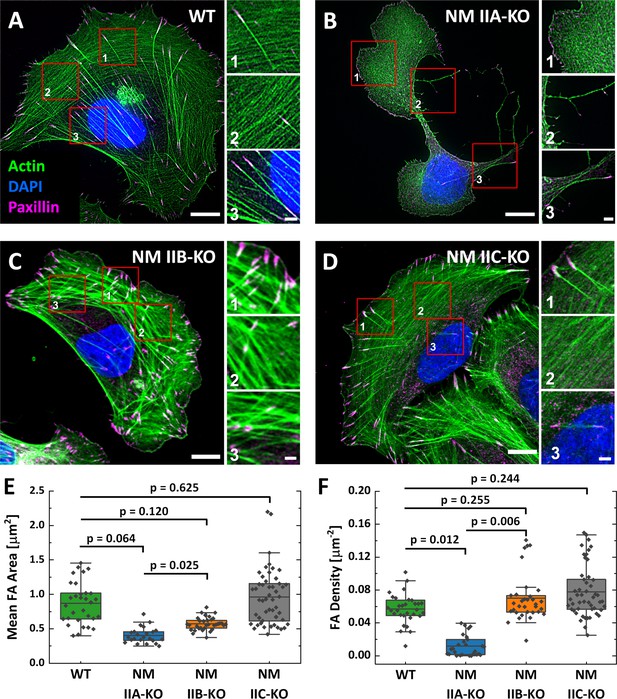
Phenotypes of NM IIA, NM IIB, and NM IIC-KO cell lines on homogenously coated substrates are very distinct.

(A) U2OS WT cells show a polarized phenotype with prominent dorsal stress fibers (dSF) (1), transverse arcs (tA) (2), and ventral stress fibers (vSF) (3). Mature focal adhesions (FA) are visualized by elongated paxillin clusters that localize at the distal ends of dSF or both ends of vSF. (B) The NM IIA-KO leads to drastic morphological changes and the loss of most SFs and mature FAs. The overall actin structure resembles a dense meshwork of fine actin filaments (1). At the trailing edge, long cell extensions remain (2) and the only bundled actin fibers resemble vSF (3). (C) NM IIB-KO cells reveal slight changes in SF organization and FA structure. dSF (1), tA (2) and vSF (3) are present but their distinct localization pattern is disturbed. (D) The phenotype of NM IIC-KO cells is comparable to the WT. dSF (1), tA (2) and vSF (3) localize in a distinct pattern along the cell axis of polarized cells. (E) The mean FA area per cell is reduced for NM IIA-KO and NM IIB-KO cells, whereas FA density is only reduced in NM IIA-KO cells (F). Scale bars represent 10 µm for overviews and 2 µm for insets of (A) - (D).

Figure 1—figure supplement 1
Download asset Open asset
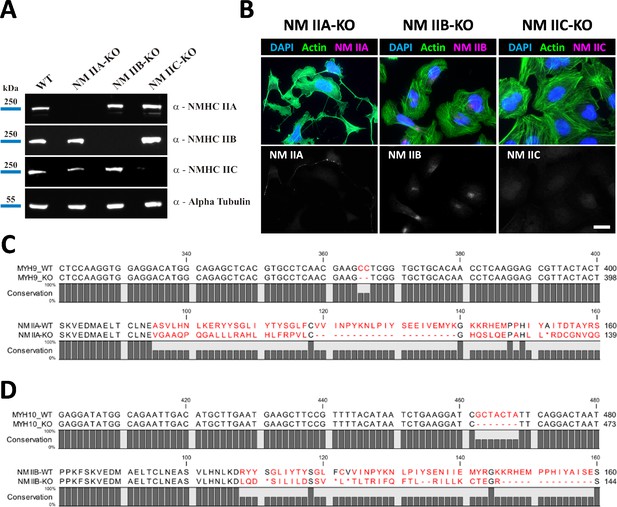
Knockout of NMHC IIA, NMHC IIB and NMHC IIC via CRISPR/Cas9.

NM II-KO cell lines were generated via CRISPR/Cas9 and the loss of protein expression was confirmed via western blot (A) and immunofluorescent labeling (B). Deletions in the coding sequences of the first coding exons (exon 2) of NMHC IIA (C) and NMHC IIB (D) lead to frame shifts and premature stop codons in corresponding protein sequences (marked by asteriscs). Scale bar represents 20 µm.

Figure 1—figure supplement 2
Download asset Open asset
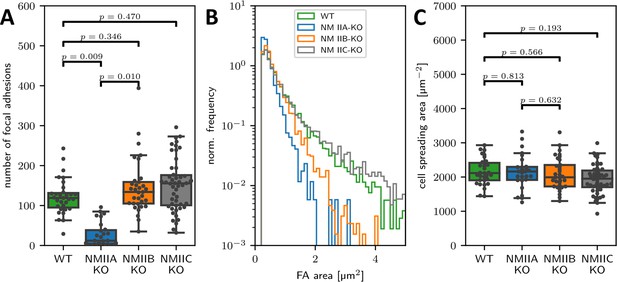
Quantification of FA number and cell spreading area.

Quantifications showing the number of focal adhesions (A), their size frequency (B), and the cell area (C) of WT and the respective NM II-KO cell lines. (A) NM IIA-KO cells show a reduced number of mature focal adhesions (larger than 0.25 µm²), while no difference was observed for NM IIB-KO and NM IIC-KO cells. (B) The frequency distribution of FAs is shifted to smaller sizes in NM IIA-KO and NM IIB-KO cells as compared to WT cells. No difference was observed for NM IIC-KO cells. (C) The cell spreading area is not affected by the loss of NM IIA, NM IIB, or NM IIC.

Figure 1—figure supplement 3
Download asset Open asset
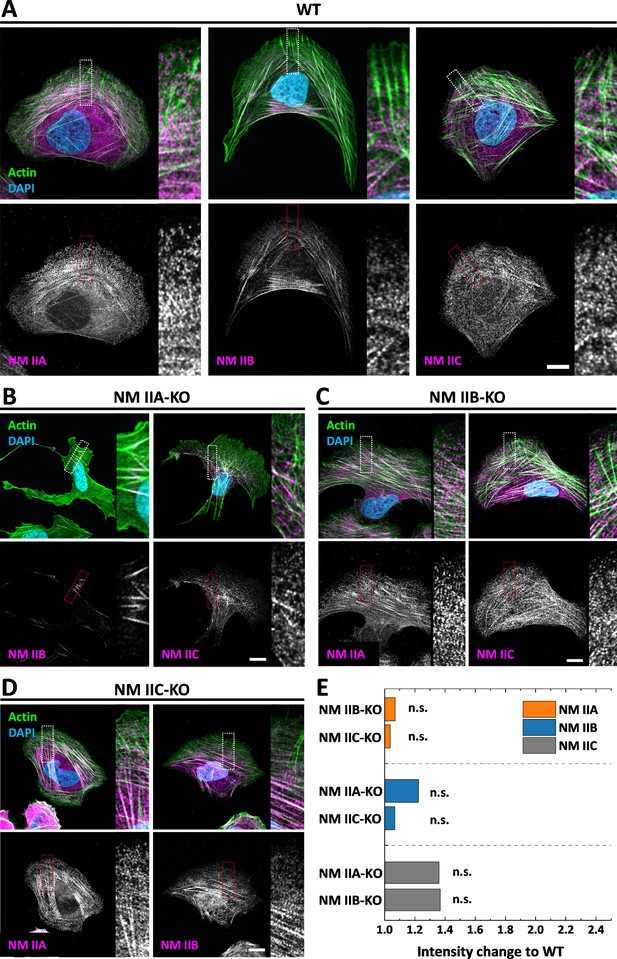
Paralog localization in NM II-KO cells.

Immunfluorescent labeling was used to analyze the localization and intensity of the NM II paralogs in U2OS WT or the respective NM II-KO cells. (A) In polarized WT cells, all isoforms co-localize with actin fibers throughout the cell body (NM IIA and NM IIC) or in the cell center (NM IIB). (B) In NM IIA-KO cells, the remaining paralogs show an altered localization pattern: NM IIB and NM IIC minifilaments are clustered along the remaining SFs in the cell center. (C) In NM IIB-KO cells, NM IIA and NM IIC minifilaments still localize throughout the cell body, comparable to WT cells. (D) The loss of NM IIC did not change the localization of the remaining paralogs. NM IIA is homogenously distrubuted and NM IIB accumulates in the cell center. (E) The mean fluorescence intensities of NM IIA-C was measured along segmented actin fibers and the fold change to the WT was calculated. All scenarios resulted in only mild intensity increases and no significant differences were observed. Scale bars represent 10 µm in (A)-(D).

Figure 1—figure supplement 4
Download asset Open asset
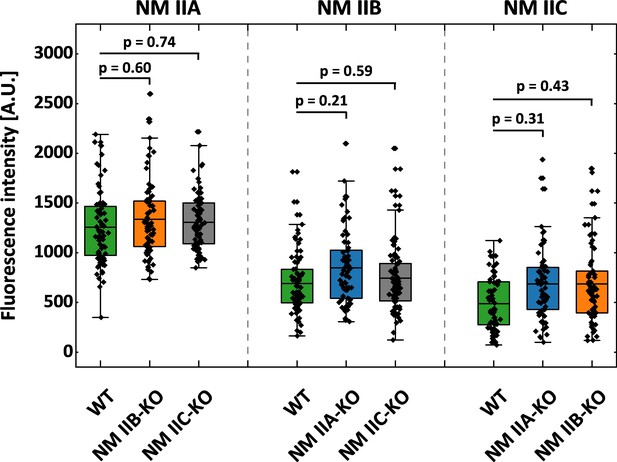
Intensity quantification of NM II-paralogs.

Segmented actin fibers were used to determine the fluorescence intensities of NM IIA-C in WT and the respective NM II-KO cells. Shown are the absolute intensity values, used to calculate the fold changes in Figure 1—figure supplement 3E.

Figure 2 with 1 supplement
Download asset Open asset
Overexpression of NM II B cannot compensate for the loss of NM II A.

(A) GFP intensities were measured along segmented actin fibers to compare the ratios of NM IIA and NM IIB in WT and NM IIB overexpressing NM IIA-KO cells. When expressed under the endogenous promoter, the mean GFP intensity of NM IIB is 4.5 times lower compared to NM IIA. To increase the total amount of NM IIB without the interference of NM IIA, NM IIA-KO cells were transiently transfected with exogenous GFP-NM IIB under the CMV promoter. Even in NM IIA-KO cells with high NM IIB expression, NM IIB filament distribution is less homogenous compared to NM IIA in WT cells. (B) Quantitative comparison of endogenous NM IIA and NM IIB in WT cells, and exogenous NM IIB in NM IIA-KO cells. (C) PRLC signal intensity was used as a marker for active NM II filaments. The quantitative comparison of WT, NM IIA-KO, and NM IIB overexpressing NM IIA-KO cells shows a substantial reduction of pRLC intensity in NM IIA-KO cells, which is not restored in NM IIB overexpressing NM IIA-KO cells. (D) NM IIB overexpression does not phenocopy the WT situation. Immunfluorescent labeling of the actin cytoskeleton (yellow) and the FA marker paxillin (magenta) revealed that SFs are still sparse and FAs are less mature. (E) Quantitative comparison of the mean FA size in WT, NM IIA-KO, and NM IIB overexpressing NM IIA-KO cells. The values for WT and NM IIA-KO are the same as for Figure 1E and are only shown for comparison. (F) AFM nanoindentation experiments were performed to measure the surface tension of the NM II-KO cell lines. Compared to the WT, NM IIA-KO cells possess a significantly lower surface tension, while it is significantly higher for NM IIB-KO cells. No difference was observed for NM IIC-KO cells. Scale bar represents 10 µm in (A and D).

Figure 2—figure supplement 1
Download asset Open asset
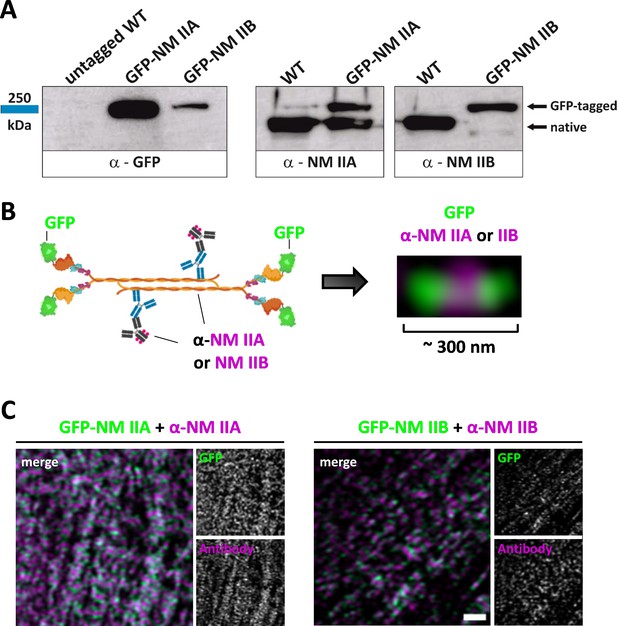
Generation of GFP-NM IIA and GFP-NM IIB fusion proteins.

GFP-NM IIA or GFP-NM IIB reporter cell lines were generated according to the guidelines in Koch et al., 2018. (A) Western blots depicting the insertion of the GFP tag in the knock-in cell lines and heterozygous expression of GFP with NM IIA or NM IIB. (B) Illustration depicting localization of the GFP tag and antibody binding sites in bipolar minifilaments. The GFP sequence was introduced at the 5’-End of the coding sequences, resulting in N-terminally tagged NM IIA or NM IIB fusion proteins. The antibodies for NM IIA or NM IIB bind at the C-terminal region, resulting in the depicted bipolar staining pattern. (C) Exemplary images of bipolar minifilaments in GFP-NM IIA or GFP-NM IIB cells. Scale bar in (C) represents 1 µm.

Figure 3 with 4 supplements
Download asset Open asset
Quantitative shape analysis on cross-shaped micropatterns reveals distinct phenotypes for NM IIA-KO and NM IIB-KO cells.

(A) U2OS WT cells show prominent invaginated actin arcs along the cell contour, with an invagination radius R and a spanning distance d (see inset). Quantitative image analysis reveals a positive R(d)-correlation (correlation coefficient r given at bottom right). Solid lines denote the bootstrapped mean fit of the dynamic tension-elasticity model (dTEM), black dashed lines denote the geometrically possible minimal radius. (B) U2OS NM IIA-KO cells form invaginated shapes on the cross-patterns despite their strongly perturbed shapes on homogenous substrates. The spanning distance of the arcs is shorter, but the positive correlation between R and d remains. (C) Actin arcs of U2OS NM IIB-KO cells are less invaginated compared to WT cells and their measured R(d)-correlation is very weak. (D) The phenotype of NM IIC-KO cells is comparable to WT and the R(d)-correlation is not affected. Scale bar represents 10 µm for (A) – (D).

Figure 3—figure supplement 1
Download asset Open asset
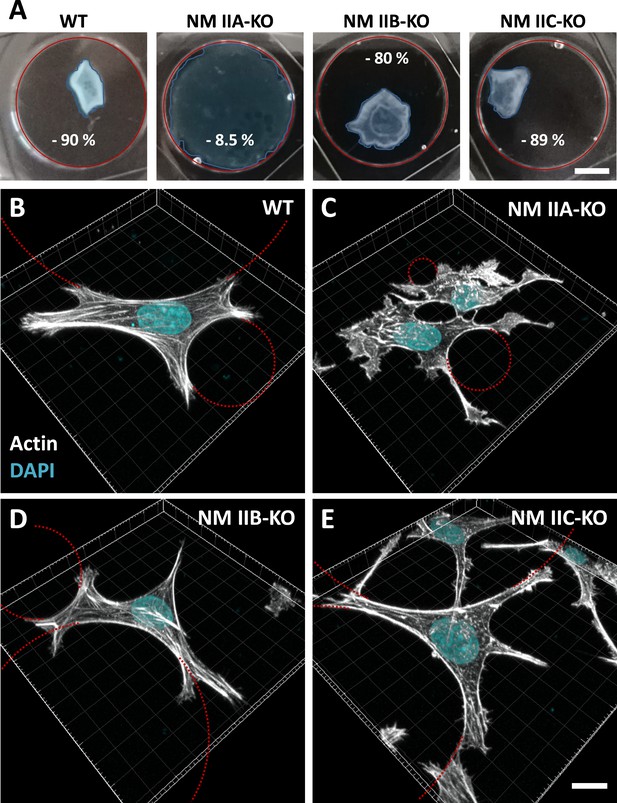
NM II-KO phenotypes in collagen gels show distinct contractile behaviors with prominent actin arcs along the cell contour.

(A) Cell seeded collagen gels (CSCG’s) were cultivated in suspension and the area was measured at the beginning and the end of the experiment. Red circles indicate the initial area, the blue area shows the CSCG after a total incubation time of 20 hr. WT cells contract the CSCG to 10% of the initial area. NM IIA-KO cells barely contract the gel and 91.5% of the initial size is still present after the incubation time. For NM IIB-KO cells, the contraction was also lower than in the WT case with 20% of the initial area left. No difference was observed for WT and NM IIC-KO cells. (B–E) 3D images of WT and NM II-KO cells, encased in collagen gels that were attached to the coverslip. All cell lines flattened in the collagen meshwork and showed prominent actin arcs along parts of the cell contour. For the sake of clarity, only some of the actin arcs were marked by the fitted circles in red. While WT, NM IIB-KO, and NM IIC-KO show comparable phenotypic features, the phenotype of NM IIA-KO cells is characterized by many lamellipodial protrusions and extensions of various shape and size. However, these protrusions are also often intersected by small actin arcs. Scale bars represent 5 mm for (A) and 15 µm for (B – E).

Figure 3—figure supplement 2
Download asset Open asset
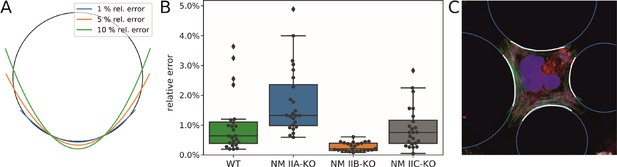
Circularity of invaginated actin arcs.

(A) The root mean squared deviation of the distance from points on the arc to the fitted center relative to the fitted radius is a measure for the circularity of the arc, as demonstrated here for parabolic curves. (B) Cell contours were fitted with the ImageJ/Fiji-plugin JFilament (Smith et al., 2010) and then fit to circular arcs with a custom-written software using the hyper least squares algorithm (Kanatani and Rangarajan, 2011). (C) For WT and all three KOs, circularity errors are relatively small, with typical values around 2% and few outliers above. The largest deviations are observed for NM IIA-KO.

Figure 3—figure supplement 3
Download asset Open asset
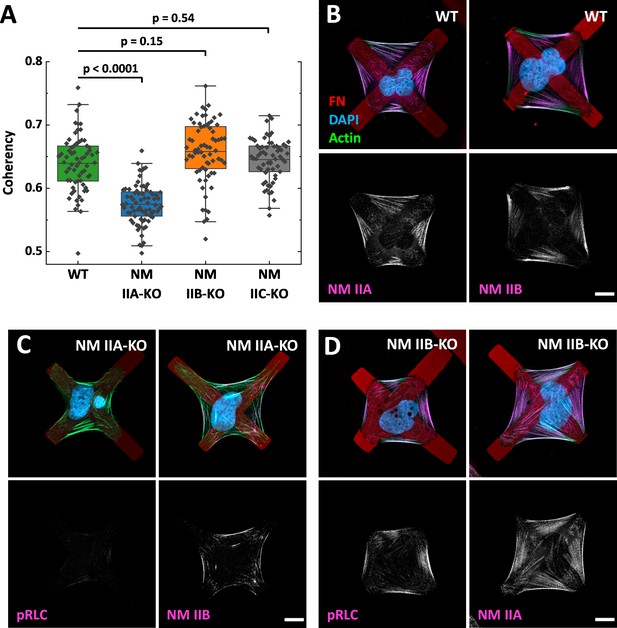
Localization of NM IIA and NM IIB minifilaments along peripheral actin arcs.

(A) The amount of actin fibers is quantified by the coherency of the structure tensor measured for the cell interior with a custom-made python script. Only NM IIA-KO cells show a significantly weaker coherency, demonstrating that these cells don’t develop internal SFs. (B) In WT cells, NM IIA, and NM IIB minifilaments localize along peripheral actin arcs and internal SFs. (C) In NM IIA-KO cells, the pRLC signal is almost absent and NM IIB minifilaments appear mainly along the actin arcs. (D) In NM IIB-KO cells, NM IIA minifilaments are homogenously distributed along the actin arcs and the internal SFs. The pRLC staining is comparable to the WT cells. Scale bars represent 10 µm for (A)-(C).

Figure 3—figure supplement 4
Download asset Open asset
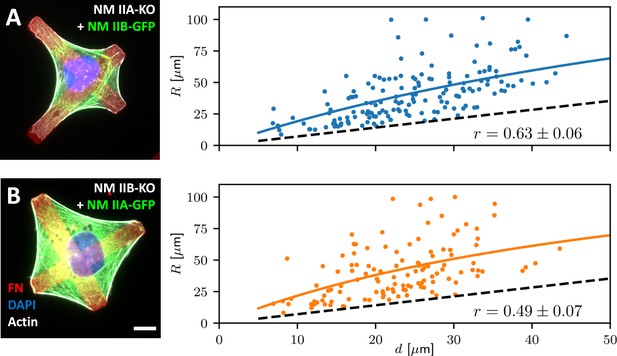
NM IIA or B overexpression on micropatterned substrates show the importance of the different motor qualities.

(A) GFP-tagged NM IIB was overexpressed in NM IIA-KO cells and the cells were seeded on micropatterned substrates. Higher quantities of NM IIB did not restore the phenotype in the absence of NM IIA. The cells still spread along the cross bars rather than spanning large passivated areas and the R(d)-correlation is positive, comparable to WT and untransfected NM IIA-KO cells. (B) Overexpressing GFP-tagged NM IIA in NM IIB-KO cells did not restore the R(d)-correlation, the values are comparably low to untransfected NM IIB-KO cells. Scale bars represent 10 µm for (A and B).

Figure 4 with 3 supplements
Download asset Open asset
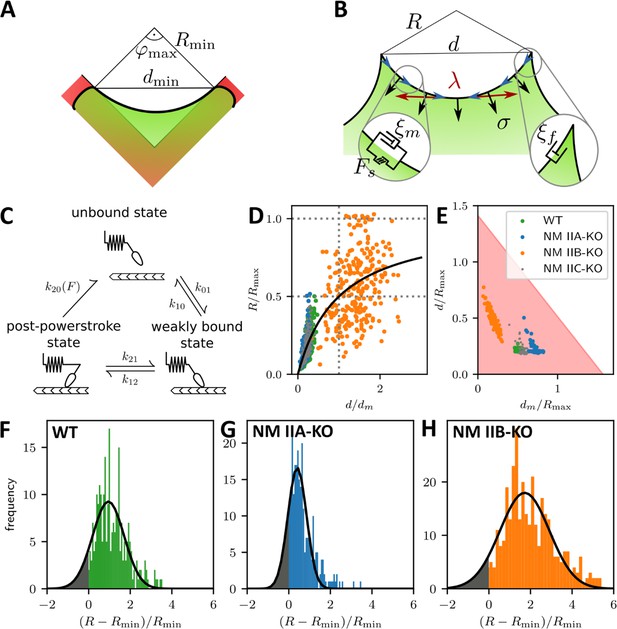
A dynamic tension-elasticity model (dTEM) connects the cellular phenotype to differences in the crossbridge cycling rates.

(A) Illustration depicting the geometrically possible minimal radius on the cross-shaped micropattern. The circular arcs on our cross pattern can have central angles of only up to 90°. (B) Schematics of the mathematical model. At each point along the cell contour, line tension λ and surface tension σ balance each other and thereby determine the circular arc shape. The insets show the frictional elements required to allow flow of the peripheral fiber (friction coefficients ξm and ξf for stress fibers and focal adhesions, respectively). The motor stall force is denoted as Fs. (C) Illustration depicting the three main mechanochemical states during the crossbridge cycle and the corresponding model rates. (D) Normalizing experimental results using the fit parameters from (A, B, C, D, E-H) yields a master curve. WT, NM IIA-KO, and NM IIC-KO cells fall into the linear regime, NM IIB-KO cells into the plateau regime, which corresponds to a loss of correlation. (E) dm/Rmax vs ratio of the maximum of the observed d-values to Rmax. The region marked in red shows where the central angle of the arc is smaller than 90°. Points denote bootstrapped fit results. (F–H) Distributions of differences between observed radius and minimum allowed radius normalized to the minimally allowed radius resemble Gaussian distributions with cut-offs. From this, we can estimate the fraction of non-formed rods (gray areas).

Figure 4—figure supplement 1
Download asset Open asset
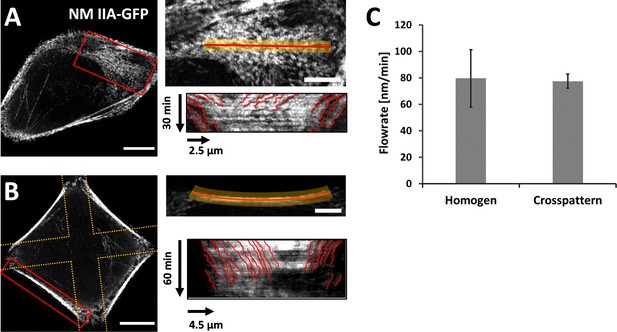
Cytoskeletal flow in actin SFs of cells on homogenously coated substrates and in actin arcs of cells on cross-shaped micropatterns.

U2OS WT cells were transfected with GFP-tagged NM IIA and cultivated on homogenously coated FN-substrates (A) or FN-coated cross-shaped micropatterns (B). Images were taken every minute for up to 1 hr using the AiryScan Mode. Kymographs were derived using the reslice mode of FIJI. The cytoskeletal flow in the kymograph was tracked manually and the flowrate was calculated from individual traces (depicted in red). (C) Mean values and standard deviations from all traces. Scale bars represent 10 µm in overviews and 5 µm in insets of (A and B).

Figure 4—animation 1
Download asset
GFP-NM IIA expressing U2OS WT cell showing cytoskeletal flow along SFs on homogenously-coated FN-substrates.

Cells were plated on cell culture dishes, coated with 10 µg ml−1 FN and imaged for 1 hr with a frame rate of 1 picture per 2 min using the AiryScan mode of an LSM 800 at ×63 magnification. The blue arrow depicts a central region with stable SF structures, where cytoskeletal flow to the center of the structure can be observed.

Figure 4—animation 2
Download asset
GFP-NM IIA expressing U2OS WT cell showing cytoskeletal flow along actin arcs on cross-shaped FN-patterned substrates.

Cells were plated on cross-shaped micropattern, functionalized with 10 µg ml−1 FN and blocked with 10 mg ml−1 BSA. Images were acquired for 1 hr with a frame rate of 1 picture per 1 min using the AiryScan mode of an LSM 800 at 63× magnification. Cytoskeletal flow can be observed along all four actin arcs.

Figure 5 with 10 supplements
Download asset Open asset
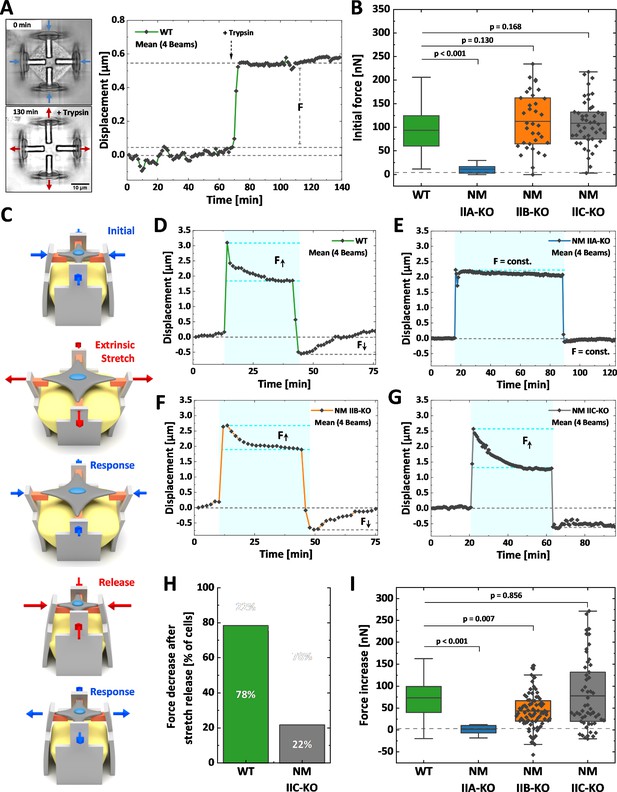
Differential contributions of NM II isoforms to cellular mechanoresponse.

Cells were cultured 3D micro-scaffolds composed of four non-adhesive walls, each with an inward directed protein-adhesive bar to guide cell attachment. Cells attach to the bars, form a cross-shaped morphology and pull the walls inwards. (A) Initial cellular tractions forces were determined by detaching the cell from the scaffold using trypsin/EDTA and measuring the corresponding average beam displacement as indicated in the plot. (B) Comparison of the initial forces of the different cell lines. Data for WT and NM IIA-KO have been reproduced from (B) of Hippler et al., 2020, therefore only the mean values are shown (originally published under the Creative Commons Attribution-Non Commercial 4.0 International Public License (CC BY-NC 4.0; https://creativecommons.org/licenses/by-nc/4.0/. Further reproduction of this panel would need to comply with the terms of this license)). No significant differences were observed between WT (mean value = 94 nN), NM IIB-KO (mean value = 112 nN), and NM IIC-KO cells (mean value = 110 nN). A significant decrease was observed for NM IIA-KO cells (mean value = 11 nN). (C) Illustration depicting the stretch-release cycle applied to the cells. (D-G) Examples of average beam displacements (corresponding to Figure 5—animations 5–8) are plotted as a function of time. The blue area depicts the time frame, in which the corresponding cell was stretched. (D) WT cells actively counteract the stretch and increase their contractile forces until reaching a plateau after ~ 30–40 min. After releasing the stretch, cellular contraction forces remained high, but decreased to the initial level after 20–30 min. (E) No cellular force response is observed, when applying the stretch-release cycle to NM IIA-KO cells, even after longer stretch periods. (F) NM IIB-KO cells increase their force after stretching and reach a plateau after 30–40 min. The force increase is lower compared to WT cells. After releasing the stretch, NM IIB-KO cells also reduce their forces until the initial set point is reached. (G) NM IIC-KO cells increase their force upon the stretch but do not relax to the initial setpoint within the observed timeframe. (H) The quantification shows that a force decrease after the stretch release was observed for 78% of the WT cells but only for 22% of the NM IIC-KO cells. (I) Comparison of the force increase of WT and NM II-KO cells, after being mechanically stretched. Data for WT and NM IIA-KO reproduced from (D) of Hippler et al., 2020, therefore only the mean values are shown (originally published under the Creative Commons Attribution-Non Commercial 4.0 International Public License (CC BY-NC 4.0; https://creativecommons.org/licenses/by-nc/4.0/. Further reproduction of this panel would need to comply with the terms of this license)). A mean increase of 73 nN was observed for WT cells and no force increase for NM IIA-KO cells (mean value = 0.29 nN). Compared to WT cells, NM IIB-KO cells display a significantly lower force increase (mean value = 41 nN), while NM IIC-KO cells show a comparable mean value. However, higher variations in the force response are observed for NM IIC-KO cells. Scale bar represents 10 µm in (A).

Figure 5—source code 1

Matlab code for displacement tracking and force calculation.

https://cdn.elifesciences.org/articles/71888/elife-71888-fig5-code1-v2.zip
Figure 5—figure supplement 1
Download asset Open asset
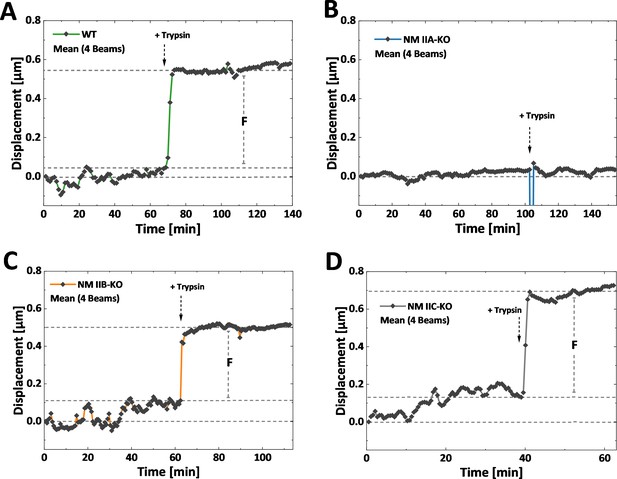
Initial force measurements of WT and NM II-KO cells.

To measure initial forces, cells were seeded on 3D microscaffolds without stimuli-responsive hydrogels and the displacement of the adhesive bars was tracked before and after the addition of trypsin. In all graphs, dashed lines denote reference points at the start of the measurement, at the time point of trypsin addition and at the end of the experiment. Exemplary mean displacement traces of a single WT (A), NM IIA-KO (B), NM IIB-KO (C), and NM IIC-KO (D) cell correspond to Figure 5—animations 1–4. No displacement was observed upon the addition of trypsin to NM IIA-KO cells, while WT, NM IIB-KO, and NM IIC-KO cells each showed a striking displacement. Note that the displacement in (B) at ~ 100 min resulted from focus issues in Figure 5—animation 2.

Figure 5—figure supplement 2
Download asset Open asset
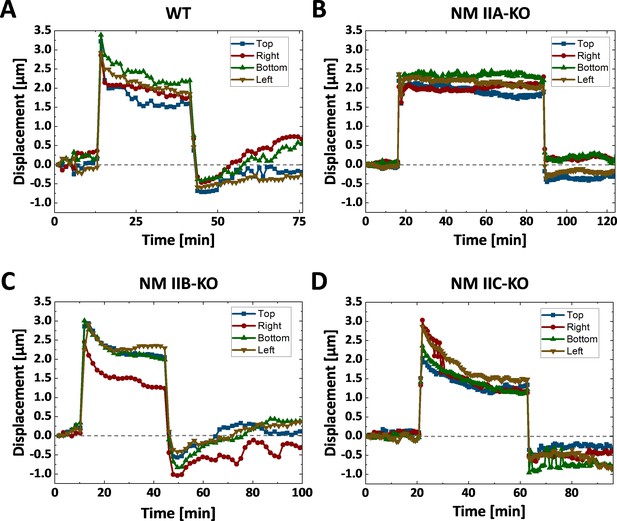
Displacements of individual beams for stretched WT and NM II-KO cells.

Cells were seeded on stimuli-responsive 3D microscaffolds and the displacements of individual adhesive beams during the stretch-release cycle was tracked. (A-D) The displacement traces from this figure correspond to the mean displacement traces in Figure 5A–D and Figure 5—animations 5–8. Dashed lines denote the reference point at the start of the measurement. (A) For the WT cell, all individual traces show a force increase after the stretch was applied and a force decrease after the stretch was released. (B) No force response was observed when stretching NM IIA-KO cells. (C) Individual beam displacements of a NM IIB-KO cell that show oscillatory motions in the cellular response, after the stretch was released (compare the red trace, corresponding to the right bar in Figure 5—animation 7). (D) Individual beam displacements of a NM IIC-KO, showing a force increase after the stretch but no force decrease during the time frame after the release.

Figure 5—animation 1
Download asset
Initial forces of a U2OS WT cell in a 3D-printed microscaffold.

Cells were cultivated in 3D-printed microscaffolds, coated with 10 µg ml−1 FN and imaged with a frame rate of 1 picture per 1.2 min using DIC at 40× magnification. The cell exerts force on the scaffold, thus displacing the adhesive beams. After 70 min, the imaging medium was replaced by 2 ml Trypsin/EDTA to detach the cell from the substrate and imaging was continued for another 70 min to ensure complete relaxation of the scaffold.

Figure 5—animation 2
Download asset
Initial forces of a U2OS NM IIA-KO cell in a 3D-printed microscaffold.

Cells were cultivated in 3D-printed microscaffolds, coated with 10 µg ml−1 FN and imaged with a frame rate of 1 picture per 1.2 min using DIC at ×40 magnification. Although the cell is moving in the scaffold, no displacements of the adhesive beams were observed. After 104 min, the imaging medium was replaced by 2 ml Trypsin/EDTA to detach the cell from the substrate and imaging was continued for another 50 min to ensure complete relaxation of the scaffold.

Figure 5—animation 3
Download asset
Initial forces of a U2OS NM IIB-KO cell in a 3D-printed microscaffold.

Cells were cultivated in 3D-printed microscaffolds, coated with 10 µg ml−1 FN and imaged with a frame rate of 1 picture per 1.2 min using DIC at ×40 magnification. The NM IIB-KO cell displaces the adhesive beams of the scaffold. After 62 min, the imaging medium was replaced by 2 ml Trypsin/EDTA to detach the cell from the substrate and imaging was continued for another 50 min to ensure complete relaxation of the scaffold.

Figure 5—animation 4
Download asset
Initial forces of a U2OS NM IIC-KO cell in a 3D-printed microscaffold.

Cells were cultivated in 3D-printed microscaffolds, coated with 10 µg ml−1 FN and imaged with a frame rate of 1 picture per 0.3 min using DIC at 40× magnification. The NM IIC-KO cell displaces the adhesive beams of the scaffold. After 23 min, the imaging medium was replaced by 2 ml Trypsin/EDTA to detach the cell from the substrate and imaging was continued for another 13 min to ensure complete relaxation of the scaffold.

Figure 5—animation 5
Download asset
Reactive forces of a U2OS WT cell in a 3D-printed microscaffold upon a stretch-release cycle.

Cells were cultivated in stimuli-responsive 3D-printed microscaffolds, coated with 10 µg ml−1 FN and imaged with a frame rate of 1 picture per 1.2 min using DIC at 40× magnification. After 13 min, the imaging medium was replaced by 2 ml imaging medium containing 20 mM 1-Adamantanecarboxylic acid to apply the stretch. The cell counteracts the stretch by increasing its force, thus pulling the beams inwards. After stretching the cell for 30 min, the medium was replaced by normal imaging medium to release the stretch and the cell was monitored for another 30 min, where the force again decreases, as indicated by the outward-directed beam displacement.

Figure 5—animation 6
Download asset
Reactive forces of a U2OS NM IIA-KO cell in a 3D-printed microscaffold upon a stretch-release cycle.

Cells were cultivated in stimuli-responsive 3D-printed microscaffolds, coated with 10 µg ml−1 FN and imaged with a frame rate of 1 picture per 1.2 min using DIC at 40× magnification. After 15 min, the imaging medium was replaced by 2 ml imaging medium containing 20 mM 1-Adamantanecarboxylic acid to apply the stretch. During the stretching time frame of 75 min, no beam displacement was observed. Similar, the cells did not displace the beams for 50 min, after the stretch was released.

Figure 5—animation 7
Download asset
Reactive forces of a U2OS NM IIB-KO cell in a 3D-printed microscaffold upon a stretch-release cycle.

Cells were cultivated in stimuli-responsive 3D-printed microscaffolds, coated with 10 µg ml−1 FN and imaged with a frame rate of 1 picture per 1.7 min using DIC at 40× magnification. After 10 min, the imaging medium was replaced by 2 ml imaging medium containing 20 mM 1-Adamantanecarboxylic acid to apply the stretch. The cell counteracts the stretch by increasing its force, thus pulling the beams inwards. After stretching the cell for 30 min, the medium was replaced by normal imaging medium to release the stretch and the cell was monitored for another 50 min, where the force again decreases, as indicated by the outward-directed beam displacement. Note that right beam repeatedly moves outwards and back inwards, indicating oscillatory force patterns.

Figure 5—animation 8
Download asset
Reactive forces of a U2OS NM IIC-KO cell in a 3D-printed microscaffold upon a stretch-release cycle.

Cells were cultivated in stimuli-responsive 3D-printed microscaffolds, coated with 10 µg ml−1 FN and imaged with a frame rate of 1 picture per 0.7 min using DIC at 40× magnification. After 20 min, the imaging medium was replaced by 2 ml imaging medium containing 20 mM 1-Adamantanecarboxylic acid to apply the stretch. The cell counteracts the stretch by increasing its force, thus pulling the beams inwards. After stretching the cell for 40 min, the medium was replaced by normal imaging medium to release the stretch and the cell was monitored for another 30 min. Besides small oscillations, no clear force decrease was observed during this time frame.

Appendix 1—figure 1
Download asset Open asset
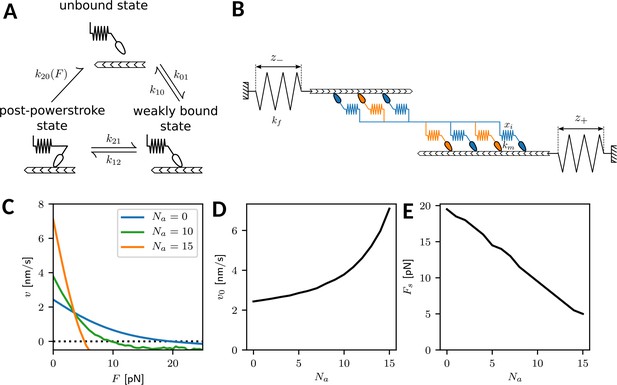
Crossbridge cycle model overview.

(A) Mechanochemical crossbridge cycle for myosin II. (B) Tug-of-war of two mixed motor ensembles working against external springs. (C) Force-velocity relation for an ensemble (one half of a minifilament) with N=15 motors with varying numbers Na of NM IIA motors. The number of NM IIB motors is Nb=N-Na. (D) Force-free velocity v0 and (E) stall force Fs as a function of Na.

Appendix 1—figure 2
Download asset Open asset
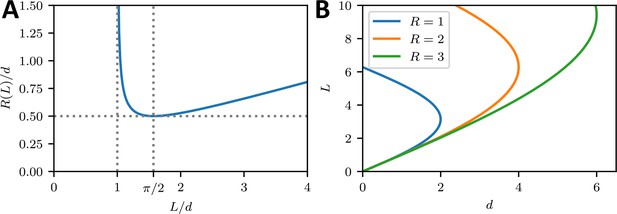
Geometrical relations between R, L and d.

(A) The arc radius R⁢(L) is a function of arc length L on the interval L∈(d,∞). For L/d<π/2 it is monotonically decreasing and for L/d>π/2 it is monotonically increasing. Normalizing R and L to the spanning distance d yields a universal result. (B) Due to the geometry, L⁢(d) is not a well defined function, as for each spanning distance there exist two solutions for a central angle 0<φ<2⁢π.

Appendix 1—figure 3
Download asset Open asset
Stability analysis.

(A) Change in dimensionless arc length l˙ for a range of spanning distances δ as a function of l for lm=2. For small spanning distances there are two steady states. The one at lower l is stable, while the other one is unstable. For increasing δ the system approaches a saddle-node distribution, beyond which both steady states vanish and the length of the peripheral arc increases indefinitely. (B) l˙ For a range of lm and spanning distance δ=1. Again the two fixed points vanish in a saddle node bifurcation.

Appendix 1—figure 4
Download asset Open asset
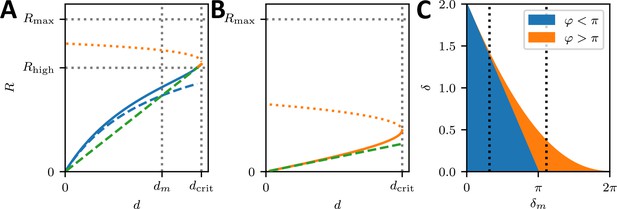
Predicted R(d)-correlations.

(A,B) R⁢(d) relationship predicted by the model. Blue solid lines are related to stable steady state lengths, where the arc is smaller than a half cicle, while dashed blue lines represent the result with approximation arcsin⁡x=x. Solid orange lines represent stable steady states where the central angle φ>π and dotted orange lines represent unstable steady states. The dashed green line denotes d=2⁢R, which corresponds to the circle of smallest radius by geometry. The colors in the phase diagrams represent parameter regions, where stable solutions can be found and represent arcs that are smaller (blue) and larger (orange) than half circles, respectively. In the white area, the arc curls inward and increases its radius indefinitely. (A) δm=1 (B) δm=3.5 (C) Phase diagram indicating whether the central angle is larger or smaller than π. The dashed vertical lines visualize the parameter range described by subfigure A and B, respectively.

Appendix 1—figure 5
Download asset Open asset
Phase diagram.

(a) Central angle φ and (b) Dimensionless radius r as a function of δ and δm. The white region represents unstable parameter configurations, while the colored areas represent the stable steady state central angle and radius respectively. The colored lines are contour lines of the central angle and indicate upper bounds for stability given that the maximum permissible central angle by the micropattern geometry.

Tables

Table 1
Mean bootstrapped fit values for the invaginated arcs.
Cell lineRmax [µm]dm [µm]dm /Rmax
WT199 ± 5100 ± 50.50 ± 0.02
NM IIA-KO190 ± 24137 ± 220.72 ± 0.04
NM IIB-KO98 ± 1820 ± 90.19 ± 0.05
NM IIC-KO194 ± 19110 ± 140.56 ± 0.03

  1. The error is given as the standard deviation of the bootstrapped fit results. Note that the fit was bounded such that Rmax < 200 µm. Therefore, in cases where Rmax is close to 200 µm, the fit only gives reasonable error estimates for the quotient dm /Rmax.

Key resources table
Reagent type
(species) or
resource		DesignationSource or
reference		IdentifiersAdditional
information
Gene (Homo sapiens)MYH9NCBINC_000022.11
Gene (Homo sapiens)MYH10NCBINC_000017.11
Gene (Homo sapiens)MYH14NCBINC_000019.10
Cell line (Homo sapiens)U2OSATCC# HTB-96
RRID:CVCL_0042
Cell line (Homo sapiens)U2OS
NM IIA-KO		This paperCRISPR/Cas9-generated knockout cell line; compare Materials section 2
Cell line (Homo sapiens)U2OS
NM IIB-KO		This paperCRISPR/Cas9-generated knockout cell line
Cell line (Homo sapiens)U2OS
NM IIC-KO		This paperCRISPR/Cas9-generated knockout cell line
Cell line (Homo sapiens)U2OS
GFP-NM IIA		This paperCRISPR/Cas9D10A-generated knock-in cell line; compare Materials section 2
Cell line (Homo sapiens)U2OS
GFP-NM IIB		This paperCRISPR/Cas9-generated Knock-in cell line
Transfected construct (Homo sapiens)CMV-GFP-NMHC IIARRID:addgene_11347PMID:11029059Gift from Robert Adelstein
Transfected construct (Homo sapiens)CMV-GFP-NMHC IIBRRID:addgene_11348PMID:11029059Gift from Robert Adelstein
Transfected construct (Homo sapiens)mApple-MyosinIIB-N-18RRID:addgene_54931RRID:Addgene_54931Gift from Michael Davidson
Recombinant DNA reagentpSPCas9(BB)−2A-Puro (PX459) V2.0RRID:addgene_62988PMID:24157548Gift from Feng Zhang
Recombinant DNA reagentpX335-U6-Chimeric_BB-CBh-hSpCas9n(D10A)RRID:addgene_42335PMID:23287718Gift from Feng Zhang
Recombinant DNA reagentpMK-RQ-MYH9This paperDonor sequence for HDR, compare Materials section 2 and 5
Recombinant DNA reagentpMK-RQ-MYH10This paperDonor sequence for HDR, compare Materials section 2 and 5
AntibodyAnti-Alpha-Tubulin (mouse monoclonal)Sigma-Aldrich#T5168
RRID:AB_477579		WB: (1:2000)
AntibodyAnti-fibronectin (mouse monoclonal)BD Biosciences#610077
RRID:AB_2105706		IF: (1:500
250 µg/ml)
AntibodyAnti-NMHC IIA (rabbit polyclonal)BioLegend#909801
RRID:AB_2565100		IF: (1:500)
WB: (1:1000
1 mg/ml)
AntibodyAnti-NMHC IIB (rabbit polyclonal)BioLegend#909901
RRID:AB_2565101		IF: (1:500)
WB: (1:1000
1 mg/ml)
AntibodyAnti-NMHC IIC (D4A7) (rabbit monoclonal)Cell signaling#8189S
RRID:AB_10886923		IF: (1:100)
WB: (1:1000)
AntibodyAnti-pMLC2 (Ser19) (mouse monoclonal)Cell signaling#3671S
RRID:AB_330248		IF: (1:200)
AntibodyAnti-Paxillin (mouse monoclonal)BD Biosciences#610619
RRID:AB_397951		IF: (1:500
250 µg/ml)
AntibodyAnti-GFP (rabbit polyclonal)abcam#ab6556
RRID:AB_305564		WB: (1:2000
0.5 mg/ml)
Peptide, recombinant proteinFibronectin from human plasmaSigma-Aldrich#F1056
RRID:AB_2830099		10 µg/ml
Peptide, recombinant proteinCollagen I and
Thin plate coating collagen I from rat tails		Enzo Life Sciences#ALX-522–435 and #ALX-522-440-00501 mg/ml final concentration
Chemical compound, drug1-Adamantanecarboxylic acidSigma-Aldrich#10639920 mM in DMEM pH 7
Software, algorithmDigital Image correlation and tracking
Version 1.2.0.0		MathWorks
MATLAB
(Eberl et al., 2006)		FileID 12413The custom-adapted script (see PMID:32967835) is provided as source code.
Software, algorithmCHOPCHOPhttps://chopchop.cbu.uib.no/
OtherPDMS Sylgard 184Dow Corning#000105989377For further instructions, see
PMID:23681634
Other1-OctadecylmercaptanSigma-Aldrich#O18581.5 mM in EtOH
OtherHS-C11-EG6-OHProChimia#TH-001-m11.n61 mM in EtOH
OtherTPE-TA TH-001-m11.n6Sigma-Aldrich#409073PMID:32967835
OtherPETASigma-Aldrich# 246794PMID:32967835
OtherHost-Guest system-based hydrogelHippler et al., 2020For detailed descriptions and composition, see PMID:32967835
OtherAlexa Fluor 488 or Alexa Fluor 647 coupled phalloidinThermoFisher Scientific#A12379 and #A22287
Table 2
Used gRNA and primer sequences.
Sequence (5 ‘→ 3‘)Target GeneDescription
GCACGTGCCTCAACGAAGCCTMYH9gRNA for DSB in
GCTGAAGGATCGCTACTATTCMYH10gRNA for DSB in Exon 2
GCGGAGTAGTACCGCTCCCGGMYH14gRNA for DSB in Exon 2
GCTTATAGCCAGGACCTAAGCMYH9gRNA for SSN in Exon 2
GTGCCGATAAGTATCTCTATGMYH9gRNA for SSN in Exon 2
GCAATTGCCTCTAAGAGAAGMYH10gRNA for SSN in Exon 2
GGCGCAGAGAACTGGACTCGMYH10gRNA for SSN in Exon 2
GCAAAGAGAAGAGGTGTGAGCMYH9Primer (fwd)_NM IIA-KO
AGTTCAAGGATGTCACCCCAMYH9Primer (rev)_NM IIA-KO
GTTAGTATGGCTGTGAAGAGGTMYH10Primer (fwd)_NM IIB-KO
TCAAAGAAAAGCAAGACATGGGTMYH10Primer (rev)_NM IIB-KO
AAGAAAGTTGTGCAGCCTGGMYH9Primer binding in LHA
GAGCCCTGAGTAGTAACGCTMYH9Primer binding in RHA
CATGTTTCTTGGAACCTGGCAMYH9Primer_5‘region of LHA_MYH9
GCAAACCCATCAGACAACCAMYH10Primer binding in LHA
ATTCTCTGCCAACTCCACCAMYH10Primer binding in RHA
CCTCTGCTAGCCCTTTGTGAMYH10Primer_5‘region of LHA_MYH10
GATGTTGCCGTCCTCCTTGAGFPPrimer (rev)_GFP

  1. Abbreviations: DSB = Double strand break; SSN = Single strand nick; fwd = forward; rev = reverse; LHA = Left homology arm; RHA = Right homology arm.

Appendix 1—table 1
Model parameters.
ParameterSymbolValueReferences
Transition rates [s-1]k20a⁢01.71Erdmann et al., 2016; Stam et al., 2015
k20b⁢00.35Erdmann et al., 2016; Stam et al., 2015
k010.2Erdmann et al., 2016; Stam et al., 2015
k100.4Rahman et al., 2018
k124.106Vilfan and Duke, 2003; Erdmann et al., 2016
k210.7Vilfan and Duke, 2003; Erdmann et al., 2016
Catch-path fractionΔc0.92Erdmann et al., 2016; Stam et al., 2015
Catch-path force scale [pN]fc1.66Erdmann et al., 2016; Stam et al., 2015
Slip-path force scale [pN]fs10.35Erdmann et al., 2016; Stam et al., 2015
Neck-linker stiffness [pN/nm]km0.7Erdmann et al., 2016; Stam et al., 2015
Powerstroke distance [nm]d8Vilfan and Duke, 2003; Erdmann et al., 2013; Erdmann and Schwarz, 2012
External springs [pN/nm]kf4Albert et al., 2014

Additional files

Source data 1

Raw data sets for quantitative evaluation.

https://cdn.elifesciences.org/articles/71888/elife-71888-data1-v2.zip
Source data 2

Western Blot raw images.

https://cdn.elifesciences.org/articles/71888/elife-71888-data2-v2.zip
Transparent reporting form
https://cdn.elifesciences.org/articles/71888/elife-71888-transrepform-v2.pdf

Download links

A two-part list of links to download the article, or parts of the article, in various formats.

Downloads (link to download the article as PDF)

Open citations (links to open the citations from this article in various online reference manager services)

Cite this article (links to download the citations from this article in formats compatible with various reference manager tools)

  1. Kai Weißenbruch
  2. Justin Grewe
  3. Marc Hippler
  4. Magdalena Fladung
  5. Moritz Tremmel
  6. Kathrin Stricker
  7. Ulrich Sebastian Schwarz
  8. Martin Bastmeyer
(2021)
Distinct roles of nonmuscle myosin II isoforms for establishing tension and elasticity during cell morphodynamics
eLife 10:e71888.
https://doi.org/10.7554/eLife.71888