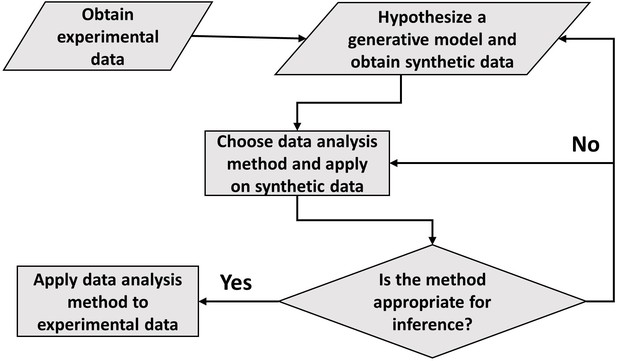
Figures and data in Distinguishing different modes of growth using single-cell data

Figures
Tables
Additional files

8 figures, 1 table and 2 additional files

Figures

Figure 1

Download asset Open asset

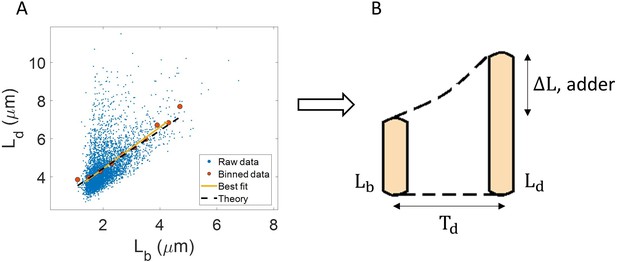
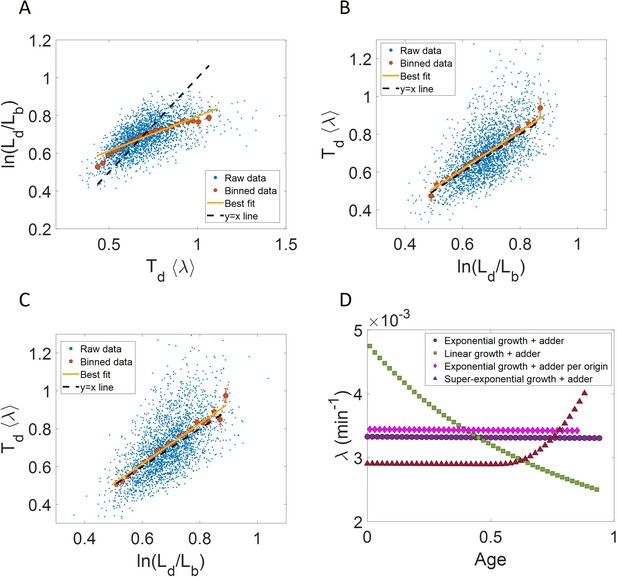
Utility of binning and linear regression.

(A) Length at division ( $L_{d}$ ) vs length at birth ( $L_{b}$ ) is plotted using data obtained by Tanouchi et al., 2017. Raw data is shown as blue dots. We find the trend in binned data (red) to be linear with the underlying best linear fit (yellow) following the equation, $L_{d} = 1.09 L_{b} + 2.24 μ m$ . This is close to the adder behavior with an underlying equation given by $L_{d} = L_{b} + Δ L$ , where $Δ L$ is the mean size added between birth and division (shown as black dashed line). B. A schematic of the adder mechanism is shown where the cell grows over its generation time ( $T_{d}$ ) and divides after addition of length $Δ L$ from birth. This ensures cell size homeostasis in single cells.

Figure 2 with 2 supplements

Download asset Open asset

Plots that could potentially lead to misinterpreting exponential growth.

(**A, B**) Data is obtained from experiments in M9 alanine medium ( $⟨ T_{d} ⟩$ = 214 min, N = 816 cells). (A) $\ln (\frac{L_{d}}{L_{b}})$ vs $⟨ λ ⟩ T_{d}$ plot is shown. The blue dots are the raw data, the red correspond to the binned data trend, the yellow line is the best linear fit obtained by performing linear regression on the raw data and the black dashed line is the y = x line. A priori, non-linear trend in binned data might point to growth being non-exponential. (B) $⟨ λ ⟩ T_{d}$ vs $\ln (\frac{L_{d}}{L_{b}})$ plot is shown for the same experiments. (**C, D**) Simulations of exponentially growing cells following the adder model are carried out for N = 2500 cells. The parameters used are provided in the Simulations section. (C) $\ln (\frac{L_{d}}{L_{b}})$ vs $⟨ λ ⟩ T_{d}$ plot is shown. The trend in binned data shown in red is non-linear and the best linear fit of raw data (yellow) deviates from the y = x line (black dashed line). The black dotted line is the expected trend obtained from theory (Equation 2). For parameters used in the simulations here, the black dotted line follows $\ln (\frac{L_{d}}{L_{b}}) = 1.26 ⟨ λ ⟩ T_{d} - 0.38 {(⟨ λ ⟩ T_{d})}^{2}$ . (D) $⟨ λ ⟩ T_{d}$ vs $\ln (\frac{L_{d}}{L_{b}})$ plot is shown with binned data in red and the best linear fit on raw data in yellow closely following the expected trend of y = x line (black dashed line). The theoretical binned data trend (black dotted line) is expected to follow the y = x trend. In all of these plots, the binned data is shown only for those bins with more than 15 data points in them.

Figure 2—figure supplement 1

Download asset Open asset

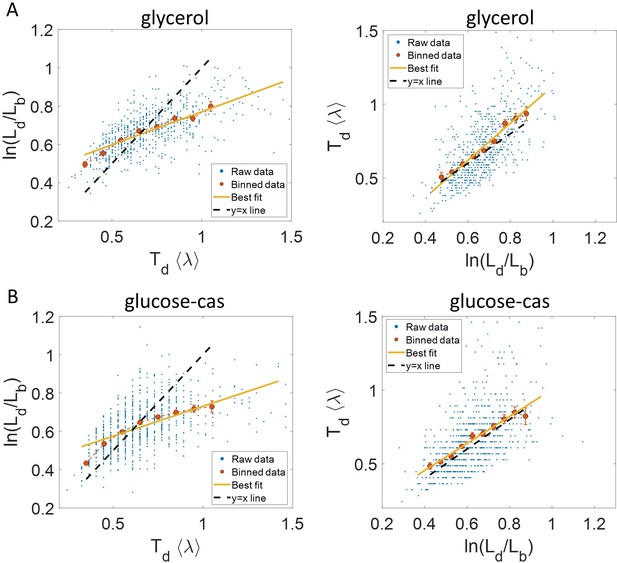
Experimental data: $\ln (\frac{L_{d}}{L_{b}})$ vs $⟨ λ ⟩ T_{d}$ (left) and $⟨ λ ⟩ T_{d}$ vs $\ln (\frac{L_{d}}{L_{b}})$ plot (right) is shown for, (A).

Cells growing in glycerol medium ( $⟨ T_{d} ⟩$ = 164 min, N = 648 cells). (B) Cells growing in glucose-cas medium ( $⟨ T_{d} ⟩$ = 65 min, N = 737 cells). Binned data (red), and the best linear fit (yellow) obtained by performing linear regression on the raw data deviate from the y = x line (black dashed line) in the case of $\ln (\frac{L_{d}}{L_{b}})$ vs $⟨ λ ⟩ T_{d}$ plots in both media. However, both binned data and the best linear fit are in close agreement with the y = x line (black dashed line) on interchanging the axes. In all of these plots, the binned data is shown only for those bins with more than 15 data points in them.

Figure 2—figure supplement 2

Download asset Open asset

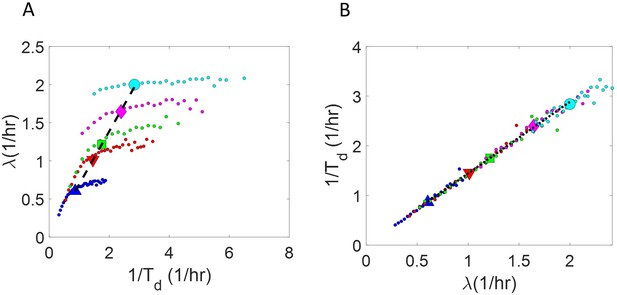
Binned data trend in growth rate ( $λ$ ) and inverse generation time ( $\frac{1}{T_{d}}$ ) plots.

(**A-B**) Simulations of the adder model for exponentially growing cells were carried out at multiple growth rates for N = 2500 cells. The size added between birth and division and the mean growth rates were extracted from Kennard et al., 2016. The CV of growth rates was greater for cells growing in slower-growth media. See the Simulations section for the parameter values. For these simulations, we show (A) $λ$ vs $\frac{1}{T_{d}}$ plot. (B) $\frac{1}{T_{d}}$ vs $λ$ plot. The smaller circles show the trend in binned data within a growth medium. Different colors correspond to different growth media. Population means are shown as larger markers. The population means agree with the expected y = $\ln (2)$ x line (black dashed line) in (A) but the trend within a single growth medium is non-linear and deviates from the y = $\ln (2)$ x line. However, in (B), population means across growth conditions and the trend in binned data within a single growth medium follow the expected y = $\frac{1}{\ln (2)}$ x line (black dotted line).

Figure 3 with 3 supplements

Download asset Open asset

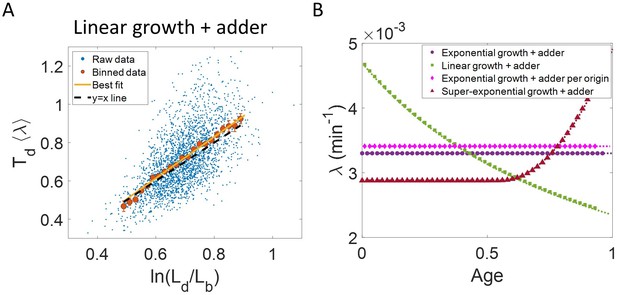
Differentiating linear growth from exponential growth.

(A) $⟨ λ ⟩ T_{d}$ vs $\ln (\frac{L_{d}}{L_{b}})$ plot is shown for simulations of linearly growing cells following the adder model for N = 2500 cell cycles. The binned data (red) and the best linear fit on raw data (yellow) closely follows the y = x trend (black dashed line) which could be incorrectly interpreted as cells undergoing exponential growth. (B) The binned data trend for growth rate vs age plot is shown as purple circles for simulations of N = 2500 cell cycles of exponentially growing cells following the adder model. We observe the trend to be nearly constant as expected for exponential growth (purple dotted line). Since the growth rate is fixed at the beginning of each cell cycle in the above simulations, we do not show error bars for each bin within the cell cycle. Also shown as green squares is the growth rate vs age plot for simulations of N = 2500 cell cycles of linearly growing cells following the adder model. As expected for linear growth, the binned growth rate decreases with age as $λ \propto \frac{1}{1 + a g e}$ (green dotted line). The binned growth rate trend (shown as magenta diamonds) is also found to be nearly constant as expected (shown as magenta dotted line) for the simulations of exponentially growing cells following the adder per origin model. We also show that the binned growth rate trend (red triangles) increases for simulations of the adder model with the cells undergoing faster than exponential growth. The trend is in agreement with the underlying growth rate function (shown as red dotted line) used in the simulations of super-exponential growth. Thus, the plot growth rate vs age provides a consistent method to identify the mode of growth. Parameters used in the above simulations of exponential, linear and super-exponential growth are derived from the experimental data in alanine medium. Details are provided in the Simulations section.

Figure 3—figure supplement 1

Download asset Open asset

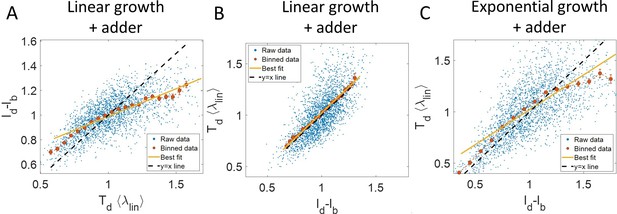
Predicting statistics based on a model of linear growth.

(**A-B**) Simulations of linearly growing cells following the adder model are carried out for N = 2500 cell cycles. (A) $l_{d} - l_{b}$ vs $⟨ λ_{l i n} ⟩ T_{d}$ plot is shown. The raw data is shown as blue dots. The binned data (in red) and the best linear fit on raw data (in yellow) deviate from the y = x line (black dashed line). Such a deviation can be predicted based on a model as discussed in detail in the Linear growth section. (B) $⟨ λ_{l i n} ⟩ T_{d}$ vs $l_{d} - l_{b}$ plot is shown. The binned data (in red) and the best linear fit on raw data (in yellow) agree with the y = x line (in black). (C) Simulations of exponentially growing cells following the adder model are carried out for N = 2500 cell cycles. $⟨ λ_{l i n} ⟩ T_{d}$ vs $l_{d} - l_{b}$ plot is shown. The binned data (in red) and the best linear fit on raw data (in yellow) deviate from the y = x line (in black) as expected for exponential growth. Parameters used in the simulations above are provided in the Simulations section.

Figure 3—figure supplement 2

Download asset Open asset

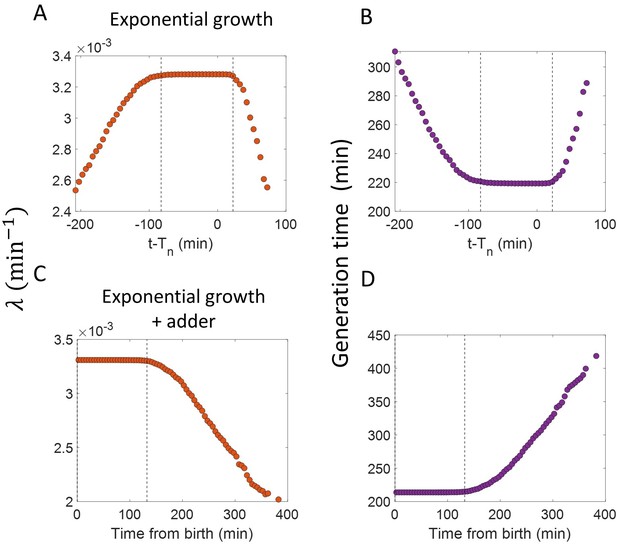
Inspection bias in the growth rate vs time plots obtained from simulations.

(A) The binned growth rate trend as a function of time from the onset of constriction (t- $T_{n}$ ) is shown in red. Time t- $T_{n} =$ 0 corresponds to onset of constriction. The plot is shown for simulations of exponentially growing cells carried out over N = 2500 cell cycles. Constriction length is determined by a constant length addition from birth and division occurs after a constant length addition from constriction. (B) The average generation time for the cells present in each bin of (A) is shown. (C) For simulations of exponentially growing cells following the adder model (N = 2500), the binned growth rate (in red) vs time from birth plot is shown. (D) The average generation time for the cells present in each bin of (C) is shown. The vertical dashed lines show the time range in which the generation times are approximately constant and hence, the effects of inspection bias are negligible. Within that time range, the growth rate trend is found to be constant, consistent with the assumption of exponential growth.

Figure 3—figure supplement 3

Download asset Open asset

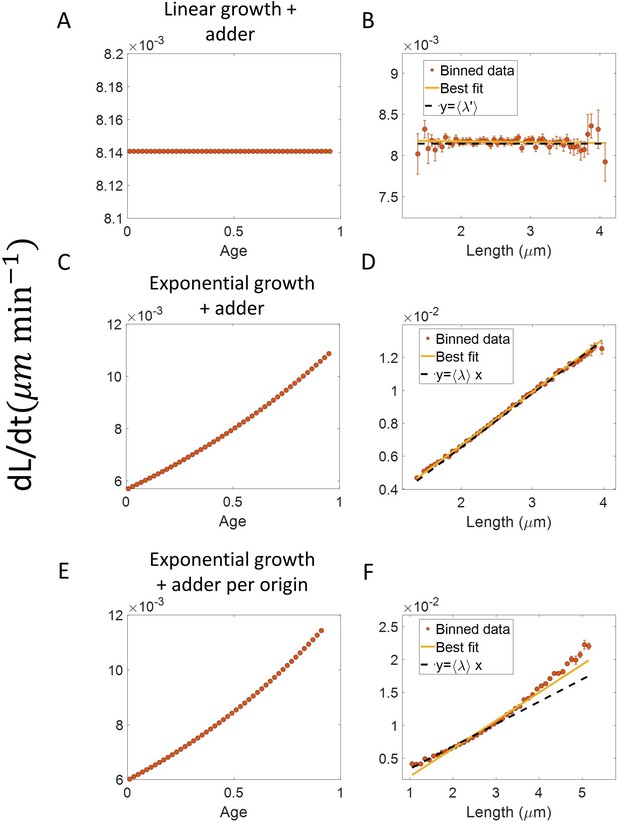
Differential methods of quantifying growth.

(**A-B**) Simulations of linearly growing cells following the adder model are carried out for N = 2500 cell cycles. Cell size ( $L$ ) data is recorded as a function of time within the cell cycle. (A) The red dots show the binned data for elongation speed as a function of age. The trend is almost constant in agreement with the linear growth assumption. (B) Elongation speed is also constant with cell size as expected for linear growth. The intercept value of the best linear fit on raw data (in yellow) provides the average elongation speed. (**C-D**) Simulations of exponentially growing cells following the adder model are carried out for N = 2500 cell cycles. (C) Elongation speed trend (in red) increases with age in agreement with the exponential growth assumption. (D) Elongation speed trend (in red) increases linearly with size. The slope of the best linear fit on raw data (in yellow) is equal to the average growth rate. (**E-F**) Simulations of exponentially growing cells following the adder per origin model are carried out for N = 2500 cell cycles. (E) Again, the elongation speed trend (in red) increases with age in agreement with the exponential growth assumption. (F) Elongation speed trend (in red) and the best linear fit on raw data (in yellow) deviates from the expected linear trend (black dashed line). This could be misinterpreted as non-exponential growth. Thus, we find that the binned data trend for the plot elongation speed vs size is model-dependent.

Figure 4 with 2 supplements

Download asset Open asset

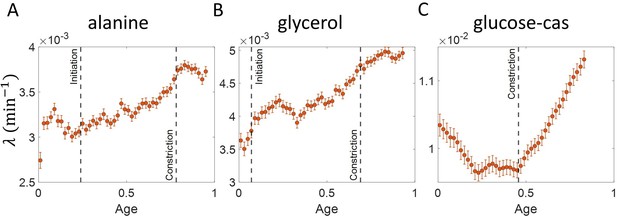
Growth rate vs age obtained from experiments: Growth rate vs age plots are shown for *E. coli* experimental data.

The red dots correspond to the binned data trends showing the variation in growth rate. The medium in which the experiments were conducted are (A) Alanine ( $⟨ T_{d} ⟩$ = 214 min) (B) Glycerol ( $⟨ T_{d} ⟩$ = 164 min) (C) Glucose-cas ( $⟨ T_{d} ⟩$ = 65 min). The error bars show the standard deviation of the growth rate in each bin scaled by $\frac{1}{\sqrt{N}}$ , where N is the number of cells in that bin. The dashed vertical lines mark the age at initiation of DNA replication (left line) and the start of septum formation (right line). In case of glucose-cas, the initiation age is not marked as it occurs in the mother cell.

Figure 4—figure supplement 1

Download asset Open asset

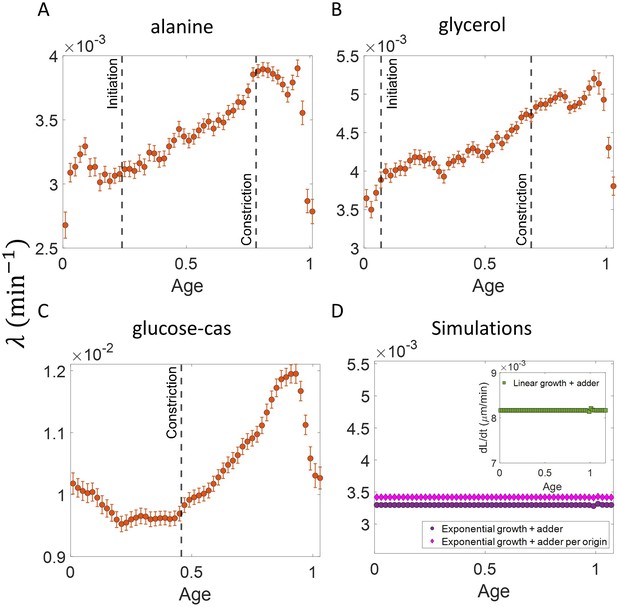
Growth rate vs age curves extended beyond the division event.

(**A,B,C**) The binned growth rate trend is shown in red as a function of age for *E. coli* experimental data. The trends are obtained using the cell size trajectories extending beyond the division event (age >1). The plots are shown for (A) Alanine medium (N = 720 cells) (B) Glycerol medium (N = 594 cells). (C) Glucose-cas medium (N = 664 cells). The error bars in all three plots represent the standard deviation of the growth rate in each bin scaled by $\frac{1}{\sqrt{N}}$ , where N is the number of cells in that bin. The growth rate trend appears to be periodic in each of the growth media that is, $λ$ at age ≈one is close to $λ$ at age ≈ 0. These trends agree with that of Figure 4 in the appropriate age ranges. (D) Simulations are carried out for N = 2500 cell cycles. The cell size trajectories are collected beyond the division event (age >1). The binned data trend for growth rate vs age plot is shown as purple circles for exponentially growing cells following the adder model. We observe the trend to be nearly constant as expected for exponential growth. The binned growth rate trend is also found to be nearly constant for the simulations of exponential growing cells following the adder per origin model (shown as magenta diamonds). (Inset) Shown as green squares is the elongation speed vs age plot for simulations of N = 2500 cell cycles of linearly growing cells following the adder model. As expected for linear growth, the binned elongation speed trend remains approximately constant with age. The growth rate trends for the models with exponential growth agree with that of Figure 3B. The elongation speed trend (inset) also agrees with the trend in Figure 3—figure supplement 3.

Figure 4—figure supplement 2

Download asset Open asset

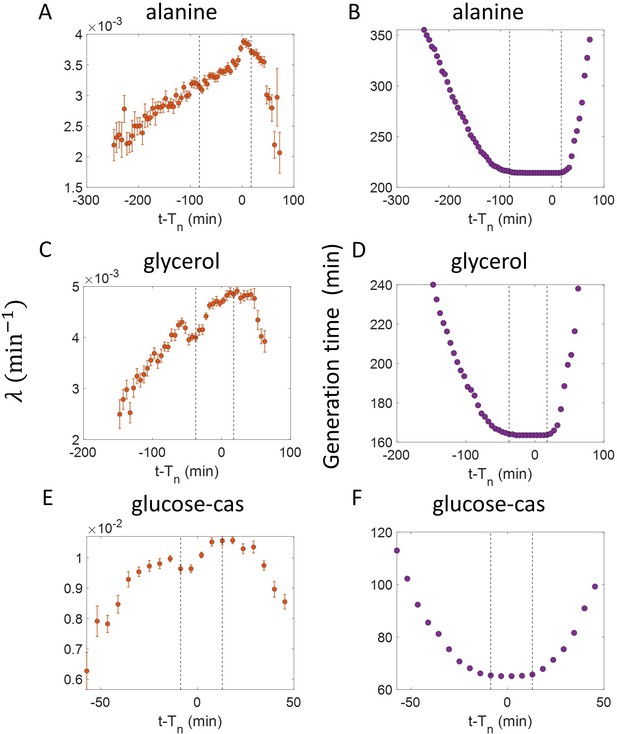
Inspection bias in the growth rate vs time from constriction plots obtained from experiments.

(**A,C,E**) The binned growth rate trend is shown in red as a function of time from the onset of constriction (t- $T_{n}$ ). Time t- $T_{n} =$ 0 corresponds to the onset of constriction for all cells considered. The plots are shown for (A). Alanine medium. (C) Glycerol medium. (E) Glucose-cas medium. The error bars in all three plots represent the standard deviation of the growth rate in each bin scaled by $\frac{1}{\sqrt{N}}$ , where N is the number of cells in that bin. (**B,D,F**) The average generation time for the cells present in each bin of (B) Alanine medium (A) (D) Glycerol medium (C) (F) Glucose-cas medium (E) are shown. The vertical dashed lines represent the time range within which the average generation time remains approximately constant. The growth rate trends within this time range are consistent with that in Figure 4 for the respective growth condition as there is negligible inspection bias.

Figure 5

Download asset Open asset

A flowchart of the general framework proposed in the paper to carry out data analysis.

Appendix 1—figure 1

Download asset Open asset

Length growth rate vs volume and surface area growth rate.

(A) Cell morphology of *E. coli* used in the model is shown. The *E. coli* cells are assumed to be cylindrical with hemispherical end caps. Before constriction, the cell elongates with constant width (2 $R$ ). However, after onset of constriction, the septum starts forming at the mid-cell. (B) Length growth rate as a function of age assuming that the total cell surface area growth is exponential, and the radius is constant ( $R$ = 0.35 $μ m$ ). (C) Length growth rate as a function of age assuming that the volume growth is exponential, radius is constant ( $R$ = 0.35 $μ m$ ) and septum surface grows at a constant rate.

Appendix 2—figure 1

Download asset Open asset

$\ln (\frac{L_{d}}{L_{b}})$ vs $⟨ T_{d} ⟩ λ$ and its flipped axes plots.

(**A-E**) $\ln (\frac{L_{d}}{L_{b}})$ vs $⟨ T_{d} ⟩ λ$ are shown for A. Experimental data in alanine medium. B. Experimental data in glycerol medium. C. Experimental data in glucose-cas medium. D. Simulations of the adder model where cells grow exponentially, carried out for N = 2500 cells. (E) Simulations of the adder model where cells grow linearly, carried out for N = 2500 cells. F-J. For the same order of the above experimental conditions and simulations, $⟨ T_{d} ⟩ λ$ vs $\ln (\frac{L_{d}}{L_{b}})$ plots are shown. In all of the plots, blue represents the raw data, red represents the binned data, and the yellow line represents the best linear fit obtained by applying linear regression on the raw data. In all of the plots, the slope of the best linear fit is close to zero. Thus, we find that these plots are not a suitable method to differentiate between linear and exponential growth as they provide a similar best linear fit.

Author response image 1

Download asset Open asset

Measurements at ∆L intervals: Results are shown for simulations carried out for N=2500 cells, where the cell length measurements are done at ∆L = 0. 05_µm intervals of length instead of equal intervals in time.

For exponentially growing cells following the adder model, (A) $\ln (\frac{L_{d}}{L_{b}}) v s < λ > T_{d}$ plot is shown. The binned data trend (red) and the best linear fit (yellow) deviate from the y=x line (black dashed line). (B) $< λ > T_{d} v s \ln (\frac{L_{d}}{L_{b}})$ plot is shown. The binned data trend (red) and the best linear fit (yellow) are close to the y=x dependence (black dashed line). C. For linearly growing cells following the adder model, the binned data trend (red) and the best linear fit (yellow) of the $< λ > T_{d} v s \ln (\frac{L_{d}}{L_{b}})$ plot closely follow the y=x dependence (black dashed line). D. The growth rate vs age plots for exponentially growing cells following the adder (purple circles) and adder per origin model (magenta diamonds) are constant while for linear growth (green squares) and super-exponential growth (red triangles) following adder model, the growth rate decreases and increases respectively in agreement with the underlying mode of growth. In summary, the results obtained for ∆L measurements are similar to that obtained for ∆t measurements.

Tables

Appendix 2—table 1

The slope and the intercept of the best linear fit along with their 95 % confidence intervals (CI) obtained on performing linear regression on experimental data.

The data is collected for cells growing in M9 alanine, glycerol and glucose-cas media (Tiruvadi-Krishnan et al., 2021).

Media	No. of	$T_{d}$	$\ln (\frac{L_{d}}{L_{b}}) v s ⟨ T_{d} ⟩ λ p l o t$		$⟨ T_{d} ⟩ λ v s \ln (\frac{L_{d}}{L_{b}}) p l o t$
Media	No. of	$T_{d}$	Slope (with 95% CI)	Intercept (with 95% CI)	Slope (with 95% CI)	Intercept (with 95% CI)
Alanine	816	214	0.04 (-0.01, 0.09)	0.65 (0.62, 0.69)	0.05 (-0.01, 0.12)	0.67 (0.63, 0.72)
Glycerol	648	164	–0.12 (-0.16,–0.07)	0.75 (0.71, 0.79)	–0.19 (-0.27,–0.11)	0.83 (0.78, 0.89)
Glucose-cas	737	65	0.11 (0.06, 0.16)	0.55 (0.52, 0.58)	0.16 (0.09, 0.23)	0.56 (0.51, 0.61)

Additional files

Transparent reporting form: https://cdn.elifesciences.org/articles/72565/elife-72565-transrepform1-v4.docx
Download elife-72565-transrepform1-v4.docx
Supplementary file 1 Supplementary Information.: https://cdn.elifesciences.org/articles/72565/elife-72565-supp1-v4.pdf
Download elife-72565-supp1-v4.pdf

Download links

A two-part list of links to download the article, or parts of the article, in various formats.

Downloads (link to download the article as PDF)

Open citations (links to open the citations from this article in various online reference manager services)

Mendeley

Cite this article (links to download the citations from this article in formats compatible with various reference manager tools)

Prathitha Kar
Sriram Tiruvadi-Krishnan
Jaana Männik
Jaan Männik
Ariel Amir

(2021)

Distinguishing different modes of growth using single-cell data

eLife 10:e72565.

https://doi.org/10.7554/eLife.72565

Figures

Utility of binning and linear regression.

Plots that could potentially lead to misinterpreting exponential growth.

Experimental data: $\ln (\frac{L_{d}}{L_{b}})$ vs $⟨ λ ⟩ T_{d}$ (left) and $⟨ λ ⟩ T_{d}$ vs $\ln (\frac{L_{d}}{L_{b}})$ plot (right) is shown for, (A).

Binned data trend in growth rate ( $λ$ ) and inverse generation time ( $\frac{1}{T_{d}}$ ) plots.

Differentiating linear growth from exponential growth.

Predicting statistics based on a model of linear growth.

Inspection bias in the growth rate vs time plots obtained from simulations.

Differential methods of quantifying growth.

Growth rate vs age obtained from experiments: Growth rate vs age plots are shown for E. coli experimental data.

Growth rate vs age curves extended beyond the division event.

Inspection bias in the growth rate vs time from constriction plots obtained from experiments.

A flowchart of the general framework proposed in the paper to carry out data analysis.

Length growth rate vs volume and surface area growth rate.

$\ln (\frac{L_{d}}{L_{b}})$ vs $⟨ T_{d} ⟩ λ$ and its flipped axes plots.

Measurements at ∆L intervals: Results are shown for simulations carried out for N=2500 cells, where the cell length measurements are done at ∆L = 0. 05_µm intervals of length instead of equal intervals in time.

Tables

The slope and the intercept of the best linear fit along with their 95 % confidence intervals (CI) obtained on performing linear regression on experimental data.

Additional files

Transparent reporting form

Supplementary file 1

Download links

Downloads (link to download the article as PDF)

Open citations (links to open the citations from this article in various online reference manager services)

Cite this article (links to download the citations from this article in formats compatible with various reference manager tools)

Be the first to read new articles from eLife

Share this article

Cite this article

Utility of binning and linear regression.

Plots that could potentially lead to misinterpreting exponential growth.

Experimental data: ln⁡(LdLb) vs ⟨λ⟩⁢Td (left) and ⟨λ⟩⁢Td vs ln⁡(LdLb) plot (right) is shown for, (A).

Binned data trend in growth rate (λ) and inverse generation time (1Td) plots.

Differentiating linear growth from exponential growth.

Predicting statistics based on a model of linear growth.

Inspection bias in the growth rate vs time plots obtained from simulations.

Differential methods of quantifying growth.

Growth rate vs age obtained from experiments: Growth rate vs age plots are shown for E. coli experimental data.

Growth rate vs age curves extended beyond the division event.

Inspection bias in the growth rate vs time from constriction plots obtained from experiments.

A flowchart of the general framework proposed in the paper to carry out data analysis.

Length growth rate vs volume and surface area growth rate.

ln⁡(LdLb)vs ⟨Td⟩⁢λ and its flipped axes plots.

Measurements at ∆L intervals: Results are shown for simulations carried out for N=2500 cells, where the cell length measurements are done at ∆L = 0. 05µm intervals of length instead of equal intervals in time.

The slope and the intercept of the best linear fit along with their 95 % confidence intervals (CI) obtained on performing linear regression on experimental data.

Transparent reporting form

Supplementary file 1

Downloads (link to download the article as PDF)

Open citations (links to open the citations from this article in various online reference manager services)

Cite this article (links to download the citations from this article in formats compatible with various reference manager tools)

Experimental data: $\ln (\frac{L_{d}}{L_{b}})$ vs $⟨ λ ⟩ T_{d}$ (left) and $⟨ λ ⟩ T_{d}$ vs $\ln (\frac{L_{d}}{L_{b}})$ plot (right) is shown for, (A).

Binned data trend in growth rate ( $λ$ ) and inverse generation time ( $\frac{1}{T_{d}}$ ) plots.

$\ln (\frac{L_{d}}{L_{b}})$ vs $⟨ T_{d} ⟩ λ$ and its flipped axes plots.

Measurements at ∆L intervals: Results are shown for simulations carried out for N=2500 cells, where the cell length measurements are done at ∆L = 0. 05_µm intervals of length instead of equal intervals in time.