Synthetic Biology: How to make an oscillator
- Eindhoven University of Technology, The Netherlands
Ever since it was discovered that the level of calcium ions inside a cell can oscillate (Woods et al., 1986), biologists have been intrigued by the periodic nature of many cellular signals. While we are slowly starting to grasp the many and varied roles that these periodic oscillations play in cellular communication, an open question remains: how are networks of genes able to generate sustained oscillations? Now, in eLife, Sebastian Maerkl and co-workers – including Henrike Niederholtmeyer and Zachary Sun as joint first authors – report that they have used a synthetic biology approach to reveal how simple gene circuits can produce robust oscillations in cells (Niederholtmeyer et al., 2015).
Initially, it was argued that periodic oscillations in the level of calcium ions and other cellular components had no role in signaling, but decades of research has revealed that periodic signals are better at relaying information than non-periodic signals (Rapp, 1987; Behar and Hoffman, 2010; Purvis and Lahav, 2013; Levine et al., 2013). Both types of signal can encode information in the size (amplitude) of the signal, but the frequency and phase of periodic signals can also encode information. As a result, periodic signals may be able to regulate complex cell processes more precisely than non-periodic signals. Importantly, recent advances in single-cell analysis and optogenetics have resulted in numerous in-depth studies that reveal how critical events, such as the determination of cell fate and multicellular communication, are controlled by periodic signals (the review by Sonnen and Auleha, 2014 describes other examples).
Mathematical analysis shows that an essential element of an oscillating circuit is an inhibitory feedback loop: if the activity of one gene in such a feedback loop increases, it activates other genes in the circuit that ultimately inhibit it (Rapp, 1987; Novak and Tyson, 2008; Purcell et al., 2010). This feedback loop needs to have an in-built time delay to enable the activities of the genes in the circuit to fluctuate in regular cycles.
The rise of synthetic biology has made it possible to design and construct synthetic networks in living cells that perform a specific role. In an early example of this, researchers at Princeton reported that they had constructed an oscillatory gene network in E. coli based on a cyclic network of three genes called the repressilator (Elowitz and Leibler, 2000; Figure 1). Theory predicts that the repressilator and other ring oscillators that have an odd number of genes (nodes) should be capable of producing sustained oscillations. However, since designing, building and testing new gene networks in living cells is a lengthy process, ring oscillators with more than three nodes have not been reported.
Figure 1
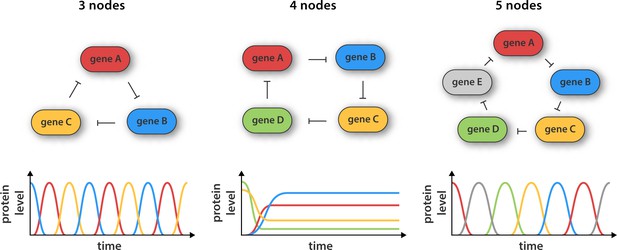
Synthetic gene networks containing three, four and five genes.
The genes in each circuit (top) are translated into protein products, with each protein product repressing the activity of another gene in the network (as indicated by the arrows). Theory predicts that cyclic networks of genes display oscillatory behavior when the number of nodes in the network is odd. Niederholtmeyer et al. found that a circuit consisting of three genes gave rise to well-defined oscillations with a period of up to 8 hr, and that a circuit containing five genes oscillated with a period of 19 hours. In contrast, and in line with theoretical predictions, a network consisting of four nodes did not oscillate: instead it reached a steady state where the activity of all the genes was constant over time.
Now Maerkl and co-workers – who are based at the École Polytechnique Fédérale de Lausanne and the California Institute of Technology – have made ring architectures containing three, four and five genes (Figure 1). They built their prototype genetic circuits in a cell-free system by combining microfluidic flow reactors with extracts from E. coli bacteria (Niederholtmeyer et al, 2013; Noireaux et al., 2003). The major advantage of this approach is that it significantly decreases the time taken for each design-build-test cycle because it removes the need for various laborious tasks, such as molecular cloning and collecting measurements from individual cells.
Using this strategy Niederholtmeyer, Sun et al. were able to confirm the prediction that oscillators with three or five nodes are able to generate oscillations, whereas oscillators with four nodes are not. The period of the five-node oscillator is about twice as long as the three-node oscillator, indicating that cells can tune the periodicity of signals by increasing the complexity of their genetic circuits. Next, the researchers transferred their prototyped designs to living E. coli cells and showed that the oscillation period in cells matched the oscillation period in the cell-free systems. This is an important result as it shows that cell-free systems can be used to accurately capture the behavior of cells, which paves the way for researchers to use synthetic biology approaches in cell-free systems to explore the complex regulatory mechanisms that operate inside cells (van Roekel et al., 2015). The latest work should also greatly speed up the construction of complex new gene networks in bacteria, which could have applications in biofuel production, medical diagnosis and experiments to explore the ways that cells process information.
References
-
Understanding the temporal codes of intra-cellular signalsCurrent Opinion in Genetics & Development 20:684–693.https://doi.org/10.1016/j.gde.2010.09.007
-
Functional roles of pulsing in genetic circuitsScience 342:1193–1200.https://doi.org/10.1126/science.1239999
-
Implementation of cell-free biological networks at steady stateProceedings of the National Academy of Sciences of the United States of America 110:15985–15990.https://doi.org/10.1073/pnas.1311166110
-
Principles of cell-free genetic circuit assemblyProceedings of the National Academy of Sciences of the United States of America 100:12672–12677.https://doi.org/10.1073/pnas.2135496100
-
Design principles of biochemical oscillatorsNature Reviews Molecular Cell Biology 9:981–991.https://doi.org/10.1038/nrm2530
-
A comparative analysis of synthetic genetic oscillatorsJournal of the Royal Society Interface 7:1503–1524.https://doi.org/10.1063/1.2978183
-
Why are so many biological systems periodic?Progress in Neurobiology 29:261–273.https://doi.org/10.1016/0301-0082(87)90023-2
-
Dynamic signal encoding—from cells to organismsSeminars in Cell & Developmental Biology 34:91–98.https://doi.org/10.1016/j.semcdb.2014.06.019
-
Programmable chemical reaction networks: emulating regulatory functions in living cells using a bottom-up approachChemical Society Reviews 44:7465–7483.https://doi.org/10.1039/C5CS00361J
Article and author information
Author details
Publication history
Copyright
© 2015, Rosier et al.
This article is distributed under the terms of the Creative Commons Attribution License, which permits unrestricted use and redistribution provided that the original author and source are credited.
Metrics
-
- 7,335
- views
-
- 652
- downloads
-
- 12
- citations
Views, downloads and citations are aggregated across all versions of this paper published by eLife.
Download links
A two-part list of links to download the article, or parts of the article, in various formats.
Downloads (link to download the article as PDF)
Open citations (links to open the citations from this article in various online reference manager services)
Cite this article (links to download the citations from this article in formats compatible with various reference manager tools)
Synthetic Biology: How to make an oscillator
eLife 4:e12260.
https://doi.org/10.7554/eLife.12260
Further reading
-
- Biochemistry and Chemical Biology
Mutations in the kinase and juxtamembrane domains of the MET Receptor Tyrosine Kinase are responsible for oncogenesis in various cancers and can drive resistance to MET-directed treatments. Determining the most effective inhibitor for each mutational profile is a major challenge for MET-driven cancer treatment in precision medicine. Here, we used a deep mutational scan (DMS) of ~5764 MET kinase domain variants to profile the growth of each mutation against a panel of 11 inhibitors that are reported to target the MET kinase domain. We validate previously identified resistance mutations, pinpoint common resistance sites across type I, type II, and type I ½ inhibitors, unveil unique resistance and sensitizing mutations for each inhibitor, and verify non-cross-resistant sensitivities for type I and type II inhibitor pairs. We augment a protein language model with biophysical and chemical features to improve the predictive performance for inhibitor-treated datasets. Together, our study demonstrates a pooled experimental pipeline for identifying resistance mutations, provides a reference dictionary for mutations that are sensitized to specific therapies, and offers insights for future drug development.
-
- Biochemistry and Chemical Biology
- Genetics and Genomics
RNA binding proteins (RBPs) containing intrinsically disordered regions (IDRs) are present in diverse molecular complexes where they function as dynamic regulators. Their characteristics promote liquid-liquid phase separation (LLPS) and the formation of membraneless organelles such as stress granules and nucleoli. IDR-RBPs are particularly relevant in the nervous system and their dysfunction is associated with neurodegenerative diseases and brain tumor development. Serpine1 mRNA-binding protein 1 (SERBP1) is a unique member of this group, being mostly disordered and lacking canonical RNA-binding domains. We defined SERBP1’s interactome, uncovered novel roles in splicing, cell division and ribosomal biogenesis, and showed its participation in pathological stress granules and Tau aggregates in Alzheimer’s brains. SERBP1 preferentially interacts with other G-quadruplex (G4) binders, implicated in different stages of gene expression, suggesting that G4 binding is a critical component of SERBP1 function in different settings. Similarly, we identified important associations between SERBP1 and PARP1/polyADP-ribosylation (PARylation). SERBP1 interacts with PARP1 and its associated factors and influences PARylation. Moreover, protein complexes in which SERBP1 participates contain mostly PARylated proteins and PAR binders. Based on these results, we propose a feedback regulatory model in which SERBP1 influences PARP1 function and PARylation, while PARylation modulates SERBP1 functions and participation in regulatory complexes.
