1. Evolutionary Biology
  2. Genetics and Genomics

Polygenic adaptation on height is overestimated due to uncorrected stratification in genome-wide association studies

  1. Mashaal Sohail  Is a corresponding author
  2. Robert M Maier  Is a corresponding author
  3. Andrea Ganna  Is a corresponding author
  4. Alex Bloemendal  Is a corresponding author
  5. Alicia R Martin  Is a corresponding author
  6. Michael C Turchin  Is a corresponding author
  7. Charleston WK Chiang
  8. Joel Hirschhorn  Is a corresponding author
  9. Mark J Daly  Is a corresponding author
  10. Nick Patterson  Is a corresponding author
  11. Benjamin Neale  Is a corresponding author
  12. Iain Mathieson  Is a corresponding author
  13. David Reich  Is a corresponding author
  14. Shamil R Sunyaev  Is a corresponding author
  1. Brigham and Women’s Hospital and Harvard Medical School, United States
  2. Harvard Medical School, United States
  3. Broad Institute of MIT and Harvard, United States
  4. Massachusetts General Hospital, United States
  5. Karolinska Institutet, Sweden
  6. University of Helsinki, Finland
  7. Brown University, United States
  8. University of Southern California, United States
  9. Boston Children’s Hospital, United States
  10. University of Pennsylvania, United States
  11. Howard Hughes Medical Institute, Harvard Medical School, United States
https://doi.org/10.7554/eLife.39702
Share this article
Cite this article
  1. Mashaal Sohail
  2. Robert M Maier
  3. Andrea Ganna
  4. Alex Bloemendal
  5. Alicia R Martin
  6. Michael C Turchin
  7. Charleston WK Chiang
  8. Joel Hirschhorn
  9. Mark J Daly
  10. Nick Patterson
  11. Benjamin Neale
  12. Iain Mathieson
  13. David Reich
  14. Shamil R Sunyaev
(2019)
Polygenic adaptation on height is overestimated due to uncorrected stratification in genome-wide association studies
eLife 8:e39702.
https://doi.org/10.7554/eLife.39702
  2. Download BibTeX
  3. Download .RIS

Abstract

Genetic predictions of height differ among human populations and these differences have been interpreted as evidence of polygenic adaptation. These differences were first detected using SNPs genome-wide significantly associated with height, and shown to grow stronger when large numbers of sub-significant SNPs were included, leading to excitement about the prospect of analyzing large fractions of the genome to detect polygenic adaptation for multiple traits. Previous studies of height have been based on SNP effect size measurements in the GIANT Consortium meta-analysis. Here we repeat the analyses in the UK Biobank, a much more homogeneously designed study. We show that polygenic adaptation signals based on large numbers of SNPs below genome-wide significance are extremely sensitive to biases due to uncorrected population stratification. More generally, our results imply that typical constructions of polygenic scores are sensitive to population stratification and that population-level differences should be interpreted with caution.

Editorial note: This article has been through an editorial process in which the authors decide how to respond to the issues raised during peer review. The Reviewing Editor's assessment is that all the issues have been addressed (see decision letter).

https://doi.org/10.7554/eLife.39702.001

Introduction

Most human complex traits are highly polygenic (Yang et al., 2010; Boyle et al., 2017). For example, height has been estimated to be modulated by as much as 4% of human allelic variation (Boyle et al., 2017; Zeng et al., 2018). Polygenic traits are expected to evolve differently from monogenic ones, through slight but coordinated shifts in the frequencies of a large numbers of alleles, each with mostly small effect. In recent years, multiple methods have sought to detect selection on polygenic traits by evaluating whether shifts in the frequency of trait-associated alleles are correlated with the signed effects of the alleles estimated by genome-wide association studies (GWAS) (Turchin et al., 2012; Berg and Coop, 2014; Mathieson et al., 2015; Robinson et al., 2015; Berg et al., 2017; Racimo et al., 2018; Guo et al., 2018).

Here we focus on a series of recent studies—some involving co-authors of the present manuscript—that have reported evidence of polygenic adaptation at alleles associated with height in Europeans. One set of studies observed that height-increasing alleles are systematically elevated in frequency in northern compared to southern European populations, a result that has subsequently been extended to ancient DNA (Turchin et al., 2012; Berg and Coop, 2014; Mathieson et al., 2015; Robinson et al., 2015; Berg et al., 2017; Racimo et al., 2018; Guo et al., 2018; Simonti et al., 2017). Another study using a very different methodology (singleton density scores, SDS) found that height-increasing alleles have systematically more recent coalescence times in the United Kingdom (UK) consistent with selection for increased height in the last few thousand years (Field et al., 2016a). In the present work, we assess polygenic adaptation on human height as a particular case of the effects that uncorrected population structure in GWAS can have on studies of complex traits.

Most of these previous studies have been based on SNP associations and effect sizes (summary statistics) reported by the GIANT Consortium, which most recently combined 79 individual GWAS through meta-analysis, including a total of 253,288 individuals (Lango Allen et al., 2010; Wood et al., 2014). Here, we show that the selection effects described in these studies are severely attenuated and in some cases no longer significant when using summary statistics derived from the UK Biobank, an independent and larger study that includes 336,474 genetically unrelated individuals who derive their recent ancestry almost entirely from the British Isles (identified as ‘white British ancestry’ by the UK Biobank) (Supplementary file 1). The UK Biobank analysis is based on a single cohort drawn from a relatively homogeneous population enabling better control of population stratification. Both datasets have high concordance even for low P value SNPs which do not reach genome-wide significance (Figure 1—figure supplement 1; genetic correlation between the two height studies is 0.94 [se = 0.0078]). Despite this concordance, we observe that small but systematic biases lead to the two datasets yielding qualitatively different conclusions with respect to signals of polygenic adaptation.

Results

Discrepancies in GWAS: population-level differences in height

To study population level differences among ancient and present-day European samples, we began by estimating ‘polygenic height scores’ as sums of allele frequencies at independent SNPs weighted by their effect sizes from GIANT. We used a set of different significance thresholds and strategies to correct for linkage disequilibrium as employed by previous studies, and replicated their signals for significant differences in genetic height across populations (Turchin et al., 2012; Berg and Coop, 2014; Mathieson et al., 2015; Robinson et al., 2015; Berg et al., 2017; Racimo et al., 2018; Guo et al., 2018; Simonti et al., 2017) (Figure 1a, Figure 1—figure supplement 2). We then repeated the analysis using summary statistics from a GWAS for height in the UK Biobank restricting to individuals of British Isles ancestry (hereafter referred to as the ‘white British' (WB) subset) and correcting for population stratification based on the first ten principal components (UK Biobank [UKB]; also referred to as ‘UKB Neale’ in the supplementary figures) (Churchhouse et al., 2017). This analysis resulted in a dramatic attenuation of differences in polygenic height scores (Figure 1a, Figure 1—figure supplements 2–4). The differences between ancient European populations also greatly attenuated (Figure 1a, Figure 1—figure supplement 5). Strikingly, the ordering of the scores for populations also changed depending on which GWAS was used to estimate genetic height both within Europe (Figure 1a, Figure 1—figure supplements 2–5) and globally (Figure 1—figure supplement 6), consistent with reports from a recent simulation study (Martin et al., 2017). The height scores were qualitatively similar only when we restricted to independent genome-wide significant SNPs in GIANT and the UK Biobank (p<5×10−8) (Figure 1—figure supplement 2b). This replicates the originally reported significant north-south difference in the allele frequency of the height-increasing allele (Turchin et al., 2012) or in genetic height (Berg and Coop, 2014) across Europe, as well as the finding of greater genetic height in ancient European steppe pastoralists than in ancient European farmers (Mathieson et al., 2015), although the signals are attenuated even here. Our observations suggest that tests of polygenic adaptation based on genome-wide significant SNPs are relatively consistent across different GWAS (Figure 1—figure supplement 2b) and that our concern is primarily directed towards the use of sub-significant SNPs in polygenic scores (Figure 1a, Figure 1—figure supplement 2a).

Figure 1 with 6 supplements see all
Download asset Open asset
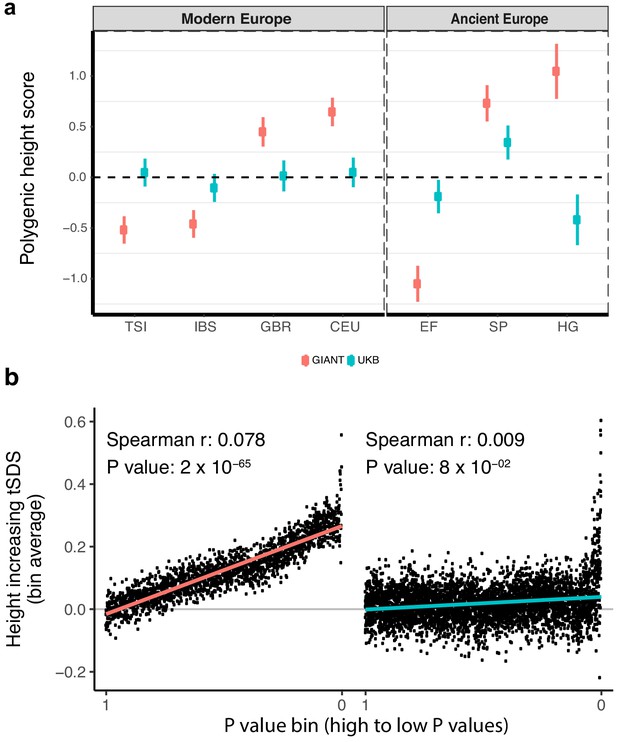
Polygenic height scores and tSDS scores based on GIANT and UK Biobank GWAS.

(a) Polygenic scores in present-day and ancient European populations are shown, centered by the average score across populations and standardized by the square root of the additive variance. Independent SNPs for the polygenic score from both GIANT (red) and the UK Biobank [UKB] (blue) were selected by picking the SNP with the lowest P value in each of 1700 independent LD blocks similarly to refs. (Berg et al., 2017; Racimo et al., 2018) (see Materials and methods). Present-day populations are shown from Northern Europe (CEU, GBR) and Southern Europe (IBS, TSI) from the 1000 genomes project; Ancient populations are shown in three meta-populations (HG = Hunter Gatherer (n = 162 individuals), EF = Early Farmer (n = 485 individuals), and SP = Steppe Ancestry (n = 465 individuals)) (see Supplementary file 2). Error bars are drawn at 95% credible intervals. See Figure 1—figure supplement 1 for analyses of concordance of effect size estimates between GIANT and UKB. See Figure 1—figure supplements 2–6 for polygenic height scores computed using other linkage disequilibrium pruning procedures, significance thresholds, summary statistics and populations. (b) tSDS for height-increasing allele in GIANT (left) and UK Biobank (right). The tSDS method was applied using pre-computed Singleton Density Scores for 4,451,435 autosomal SNPs obtained from 3195 individuals from the UK10K project (Field et al., 2016a; Field et al., 2016b) for SNPs associated with height in GIANT and the UK Biobank. SNPs were ordered by GWAS P value and grouped into bins of 1000 SNPs each. The mean tSDS score within each P value bin is shown on the y-axis. The Spearman correlation coefficient between the tSDS scores and GWAS P values, as well as the correlation standard errors and P values, were computed on the un-binned data. The gray line indicates the null-expectation, and the colored lines are the linear regression fit. The correlation is significant for GIANT (Spearman r = 0.078, p=1.55×10−65) but not for UK Biobank (Spearman r = −0.009, p=0.077). See Figure 1—source data 1 for figure data.

https://doi.org/10.7554/eLife.39702.002
Figure 1—source data 1

Polygenic height scores and tSDS scores based on GIANT and UK Biobank GWAS.

https://doi.org/10.7554/eLife.39702.009

Discrepancies in GWAS: height evolution within a single population

Next, we assessed if an independent measure, the ‘singleton density score (SDS)', which uses a coalescent approach to infer adaptation within a population, is equally as susceptible to biases in GWAS (Field et al., 2016a; Field et al., 2016b). SDS can be combined with GWAS effect size estimates to infer polygenic adaptation on complex traits (generating a ‘tSDS score’ by aligning the SDS sign to the trait-increasing allele). A tSDS score larger than zero for height-increasing alleles implies that these alleles have been increasing in frequency in a population over time due to natural selection. We replicate the original finding that SDS scores of the height-increasing allele computed in the UK population (using the UK10K dataset) increase with stronger association of the alleles to height as inferred by GIANT (Field et al., 2016a) across the entire P value spectrum (Spearman’s ρ = 0.078, p=1.55×10−65, Figure 1b). However, we observed that this signal of polygenic adaptation in the UK, measured using a Spearman correlation across all GWAS SNPs, disappeared when we used the UK Biobank height effect size estimates (ρ = 0.009, p=0.077, Figure 1b). These observations suggest that concerns about sub-significant SNPs should not only be directed towards population-level differences using polygenic scores but also to analyses of adaptation within a single population.

Population structure underlying discrepancies in GWAS

Discrepancies between GIANT and UK biobank

We propose that the qualitative difference between the polygenic adaptation signals in GIANT and the UK Biobank is due to the cumulative effect of subtle biases in each of the SNPs estimated in GIANT. This bias can arise due to incomplete control of the population structure in GWAS (Novembre and Barton, 2018). For example, if height were differentiated along a north-south axis because of differences in environment, any variant that is differentiated in frequency along the same axis would have an artificially large effect size estimated in the GWAS. Population structure is substantially less well controlled for in the GIANT study than in the UK Biobank study. This is both because the GIANT study population is more heterogeneous than that in the UK Biobank, and because population structure in the GIANT meta-analysis may not have been well controlled in some component cohorts due to their relatively small sizes (i.e., the ability to detect and correct population structure is dependent on sample size (Patterson et al., 2006; Price et al., 2006). The GIANT meta-analysis also found that such stratification effects worsen as SNPs below genome-wide significance are used to estimate height scores (Wood et al., 2014), consistent with our finding that the differences in genetic height among populations increase when including these SNPs.

We obtained direct confirmation that population structure is more correlated with effect size estimates in GIANT than to those in the UK Biobank. Figure 2a shows that the effect sizes estimated in GIANT, in contrast to those in the UKB, are highly correlated with the SNP loadings of several principal components of population structure (PC loadings). We also find that the UK Biobank estimates including individuals of diverse ancestry and not correcting for population structure (UKB all no PCs) show the same stratification effects as GIANT (Figure 2—figure supplements 1–3). Further, in line with our intuition regarding the effects of residual stratification on GWAS effect size estimates, we find that alleles that are more common in the Great Britain population (1000 genomes GBR) than in the Tuscan population from Italy (1000 genomes TSI) tend to be preferentially estimated as height-increasing according to the GIANT study but not according to the UKB study (Figure 2c, Figure 2—figure supplements 2–3).

Figure 2 with 4 supplements see all
Download asset Open asset
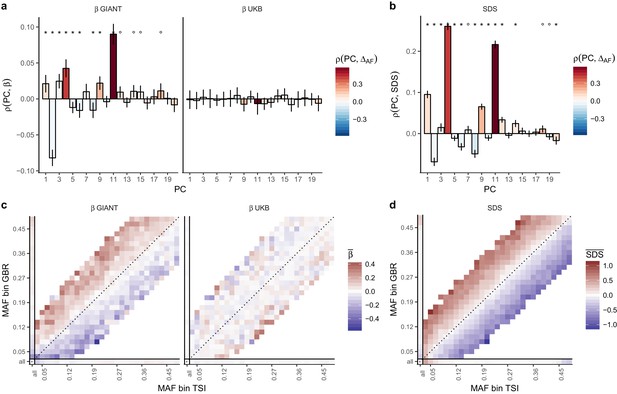
Evidence of stratification in height summary statistics.

Top row: Pearson Correlation coefficients of (a) PC loadings and height beta coefficients from GIANT and UKB, and (b) PC loadings and SDS (pre-computed in the UK10K) across all SNPs. PCs were computed in all 1000 genomes phase one samples (Abecasis et al., 2012). Colors indicate the correlation of each PC loading with the allele frequency difference between GBR and TSI, a proxy for the European North-South genetic differentiation. PC 4 and 11 are most highly correlated with the GBR - TSI allele frequency difference. Confidence intervals and P values are based on Jackknife standard errors (1000 blocks). Open circles indicate correlations significant at alpha = 0.05, stars indicate correlations significant after Bonferroni correction in 20 PCs (p<0.0025). Bottom row: Heat map after binning all SNPs by GBR and TSI minor allele frequency of (c) mean beta coefficients from GIANT and UKB, and (d) SDS scores for all SNPs. Only bins with at least 300 SNPs are shown. While the stratification effect in SDS is not unexpected, it can lead to false conclusions when applied to summary statistics that exhibit similar stratification effects. See Figure 2—figure supplements 1–3 for analyses of stratification effects in different summary statistics, and Supplementary file 3 for further description of stratification effects. UKB height betas exhibit stratification effects that are weaker, and in the opposite direction of the stratification effects in GIANT (see Figure 2—figure supplement 4 for a possible explanation). See Figure 2—source data 1 for figure data.

https://doi.org/10.7554/eLife.39702.010
Figure 2—source data 1

Evidence of stratification in height summary statistics.

https://doi.org/10.7554/eLife.39702.015

Effect size estimates from previously published family-based height GWAS

We analyzed previously released family-based effect size estimates based on an approach of Robinson et al. (2015) (NG2015 sibs). Surprisingly, we found that while these summary statistics produced significant polygenic adaptation signals, they were also correlated with PC loadings as well as with GBR-TSI allele frequency differences (Figure 2—figure supplements 1–3). This suggests that these estimates are also affected by population structure despite being computed within families and, therefore, in principle, robust to structure. Our own family-based estimates in the UK Biobank (UKB sibs all, UKB sibs WB) appear unconfounded and do not produce significant adaptation signals across the spectrum of associated SNPs (Figure 2—figure supplements 1–3). The residual structure in the original NG2015 sibs dataset is likely to reflect a technical artifact (personal communication from Peter Visscher, and note on their website [Program in Complex Trait Genomics, 2018]). Berg and colleagues (Berg et al., 2019) show that the updated NG2015 sibs summary statistics (posted in the public domain [Program in Complex Trait Genomics, 2018] in November 2018 during the revision of this manuscript) do not show significant signals of polygenic adaptation using either polygenic score differences in Europe or the tSDS metric in the UK.

Population structure within the UK biobank

We also note that the white British subset of the UKB data is not completely free of population stratification (as shown previously [Haworth et al., 2019]), although the magnitude of the potential confounding is much smaller than in the Continental European population (Figure 2—figure supplements 1–2). Interestingly, the north-south genetic cline in the UK tracks the height gradient in the opposite direction than in Continental Europe (Figure 2—figure supplements 2 and 4), and after correcting with principal components, we do not observe any evidence of residual stratification in comparison with the 1000 genomes data (Figure 2a,c). However, we cannot exclude the possibility of uncorrected population stratification, even in the UK Biobank, along axes not captured by the principal components of the 1000 genomes project data. For example, even for genome-wide significant SNPs (Figure 1—figure supplement 2b), polygenic scores for both modern and ancient individuals change when UKB summary statistics (WB ancestry controlling for 10 PCs) are used instead of GIANT. This shift, for example, for the ancient European hunter-gatherer polygenic score is troubling as different European populations are shown to have variable amounts of genetic ancestry from ancient ‘hunter-gatherer’ vs. ‘early farmer’ vs. ‘steppe ancestry’ populations (Haak et al., 2015; Galinsky et al., 2016), and could reflect residual stratification in the UKB GWAS not captured by the 1000 genomes PCs.

Effects of population structure on within-population adaptation inference

We proceeded to investigate the effects of uncontrolled population stratification in GWAS discussed above on a coalescent approach such as tSDS that relies on singleton density (Field et al., 2016a). In principle, this approach is robust to the type of population stratification that affects the allele-frequency based tests. However, there is a north-south cline in singleton density in Europe due to lower genetic diversity in northern than in southern Europeans, leading to singleton density being lower in northern than in southern regions (Sohail et al., 2017). As a consequence, SDS tends to be higher (corresponding to fewer singletons) in alleles more common in GBR than in TSI (Figure 2d). This cline in singleton density coincidentally parallels the phenotypic cline in height and the major axis of genome-wide genetic variation. Therefore, when we perform the tSDS test using GIANT, we find a higher SDS around the inferred height-increasing alleles, which tend, due to the uncontrolled population stratification in GIANT, to be at high frequency in northern Europe (Figure 2c). This effect does not appear when we use UK Biobank summary statistics because of the much lower level of population stratification and more modest variation in height. We find that SDS is not only correlated with GBR-TSI allele frequency differences, but with several principal component loadings across all SNPs (Figure 2b), and that these SDS-PC correlations often coincide with correlations between GIANT-estimated effect sizes and PC loadings (Figure 2a). We further find that the tSDS signal which is observed across the whole range of P values in some GWAS summary statistics can be mimicked by replacing SDS with GBR-TSI allele frequency differences (Figure 3a and c, Figure 3—figure supplements 1–4), suggesting that the tSDS signal at non-significant SNPs may be driven in part by residual population stratification.

Figure 3 with 5 supplements see all
Download asset Open asset
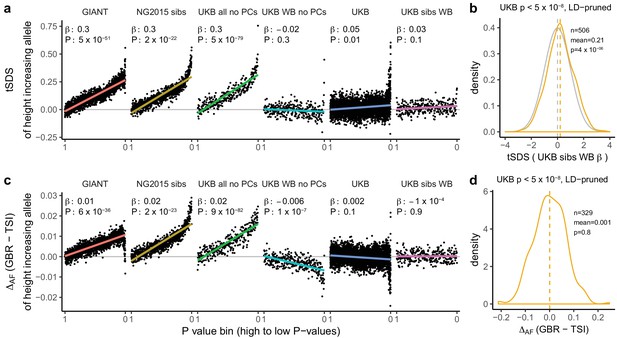
Height tSDS results for different summary statistics.

(a) Mean tSDS of the height increasing allele in each P value bin for six different summary statistics. The first two panels are computed analogously to Figure 4A and Figure S22 of Field et al. (2016a). In contrast to those Figures and to Figure 1b, the displayed betas and P values correspond to the slope and P value of the linear regression across all un-binned SNPs (rather than the Spearman correlation coefficient and Jackknife P values). The y-axis has been truncated at 0.75, and does not show the top bin for UKB all no PCs, which has a mean tSDS of 1.5. (b) tSDS distribution of the height increasing allele in 506 LD-independent SNPs which are genome-wide significant in a UKB height GWAS, where the beta coefficient is taken from a within sibling analysis in the UKB. The gray curve represents the standard normal null distribution, and we observe a significant shift. (c) Allele frequency difference between GBR and TSI of the height increasing allele in each P value bin for six different summary statistics. Betas and P values correspond to the slope and P value of the linear regression across all un-binned SNPs. The lowest P value bin in UKB all no PCs with a y-axis value of 0.06 has been omitted. (d) Allele frequency difference between GBR and TSI of the height increasing allele in 329 LD-independent SNPs which are genome-wide significant in a UKB height GWAS and were intersected with our set of 1000 genomes SNPs. There is no significant difference in frequency in these two populations, suggesting that tSDS shift at the genome-wide significant SNPs is not driven by population stratification at least due to this particular axis. The patterns shown here suggest that the positive tSDS values across the whole range of P values is a consequence of residual stratification. At the same time, the increase in tSDS at genome-wide significant, LD-independent SNPs in (b) cannot be explained by GBR - TSI allele frequency differences as shown in (d). See Figure 3—figure supplements 1–4 for other GWAS summary statistics for unpruned and LD-pruned SNPs. Binning SNPs by P value without LD-pruning can lead to unpredictable patterns at the low P value end, as the SNPs at the low P value end are less independent of each other than higher P value SNPs (Figure 3—figure supplement 5). See Figure 3—source data 1 for figure data.

https://doi.org/10.7554/eLife.39702.016
Figure 3—source data 1

Height tSDS results for different summary statistics.

https://doi.org/10.7554/eLife.39702.022

A residual signal of polygenic adaptation on height?

For polygenic adaptation within a population, a small but significant tSDS signal is observed in the UK when we restrict to genome-wide significant SNPs (p<5×10−8). This effect persists when using UK Biobank family-based estimates (UKB sibs WB) for genome-wide significant SNPs (Figure 3b), and is not driven by allele frequency differences between GBR and TSI (Figure 3d), suggesting an attenuated signal of polygenic adaptation in the UK that is driven by a much smaller number of SNPs than previously thought. Indeed, under most genetic architectures, a tSDS signal which is driven by natural selection is not expected to lead to an almost linear increase over the whole P value range in a well-powered GWAS. Instead, we would expect to see a greater difference between highly significant SNPs and non-significant SNPs, similar to the pattern observed in the UK Biobank (Figure 3a).

For population-level differences in height, we assessed whether any remaining variation in height polygenic scores among populations is driven by polygenic adaptation by testing against a null model of genetic drift (Berg and Coop, 2014). We re-computed polygenic height scores in the POPRES dataset to increase power for this analysis as it has larger sample sizes of northern and southern Europeans than the 1000 Genomes project (Nelson et al., 2008). We computed height scores using independent SNPs that are 1) genome-wide significant in the UK Biobank (‘gw-sig’, p<5×10−8) and 2) sub-significantly associated with height (‘sub-sig’, p<0.01) in different GWAS datasets. For each of these, we tested if population differences were significant due to an overall overdispersion (PQx), and if they were significant along a north-south cline (Plat) (Figure 4, Figure 4—figure supplements 1–2). Both gw-sig and sub-sig SNP-based scores computed using GIANT effect sizes showed significant overdispersion of height scores overall and along a latitude cline, consistent with previous results (Figure 4, Figure 4—figure supplements 1–2). However, the signal attenuated dramatically between sub-sig (Qx = 1100, PQx = 1×10−220) and gw-sig (Qx = 48, PQx = 2×10−4) height scores. In comparison, scores that were computed using the UK Biobank (UKB) effect sizes showed substantially attenuated differences using both sub-sig (Qx = 64, PQx = 5×10−7) and gw-sig (Qx = 33, PQx = 0.02) SNPs, and a smaller difference between the two scores. This suggests that the attenuation of the signal in GIANT is not only driven by a loss of power when using fewer gw-sig SNPs, but also reflects a decrease in stratification effects. The overdispersion signal disappeared entirely when the UK Biobank family based effect sizes were used (Figure 4, Figure 4—figure supplements 1–2). Moreover, Qx P values based on randomly ascertained SNPs and UK Biobank summary statistics are not uniformly distributed as would be expected if the theoretical null model is valid and if population structure is absent (Figure 4—figure supplement 3). The possibility of residual stratification effects even in the UK Biobank is also supported by a recent study (Haworth et al., 2019). Therefore, we remain cautious about interpreting any residual signals as ‘real’ signals of polygenic adaptation.

Figure 4 with 4 supplements see all
Download asset Open asset
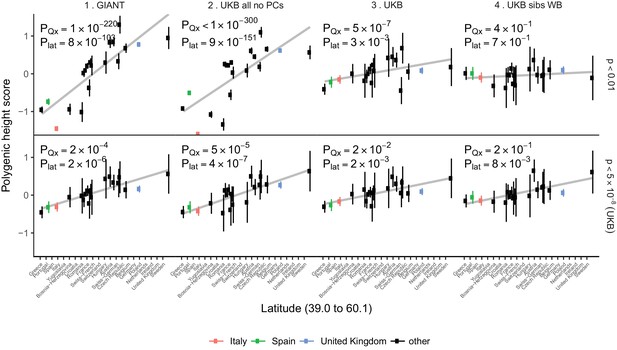
Polygenic height scores in POPRES populations show a residual albeit attenuated signal of polygenic adaptation for height.

Standardized polygenic height scores from four summary statistics for 19 POPRES populations with at least 10 samples per population, ordered by latitude (see Supplementary file 4). The grey line is the linear regression fit to the mean polygenic scores per population. Error bars represent 95% confidence intervals and are calculated in the same way as in Figure 1. SNPs which were overlapping between each set of the summary statistics and the POPRES SNPs were clumped using PLINK 1.9 with parameters r^2 < 0.1, 1 Mb distance, p<1. (Top) A number of independent SNPs was chosen for each summary statistic to match the number of SNPs which remained when clumping UKB at p<0.01. (Bottom) A set of independent SNPs with p<5×10−8 in the UK Biobank was selected and used to compute polygenic scores along with effect size estimates from each of the different summary statistics. The numbers on each plot show the Qx P value and the latitude covariance P value respectively for each summary statistic. See Figure 4—figure supplements 1–4 for other clumping strategies and GWAS summary statistics. See Figure 4—source data 1 for figure data.

https://doi.org/10.7554/eLife.39702.023
Figure 4—source data 1

Polygenic height scores in POPRES populations show a residual albeit attenuated signal of polygenic adaptation for height.

This reference was updated from its bioRxiv version to its now published version.

https://doi.org/10.7554/eLife.39702.028

Discussion

We have shown, by conducting a detailed analysis of human height, that estimates of population differences in polygenic scores are reduced when using the UK Biobank GWAS data relative to claims of previous studies that used GWAS meta-analyses such as GIANT. We find some evidence for population-level differences in genetic height, but it can only be robustly seen at highly significant SNPs, because any signal at less significant P values is dominated by the effect of residual population stratification. Even genome-wide significant SNPs in these analyses may be subtly affected by population structure, leading to continued overestimation of the effect. Thus, it is difficult to arrive at any quantitative conclusion regarding the proportion of the population differences that are due to statistical biases vs. population stratification of genetic height. Further, estimates of the number of independent genetic loci contributing to complex trait variation are sensitive to and likely confounded by residual population stratification.

We conclude that while effect estimates are highly concordant between GIANT and the UK Biobank when measured individually (Supplementary file 5–7, Figure 1—figure supplement 1), they are also influenced by residual population stratification that can mislead comparisons of complex traits across populations and inferences about polygenic adaptation. Although these biases are subtle, in the context of tests for polygenic adaptation, which are driven by small systematic shifts in allele frequency, they can create highly significant artificial signals especially when SNPs that are not genome-wide significant are used to estimate genetic height. Our results do not question the reliability of the genome-wide significant associations discovered in the GIANT cohort. However, we urge caution in the interpretation of signals of polygenic adaptation or between-population differences that are based on large number of sub-significant SNPs–particularly when using effect sizes derived from meta-analysis of heterogeneous cohorts which may be unable to fully control for population structure.

Our results have implications in other areas of human genetics research. For example, there is growing interest in polygenic scores that predict complex phenotypes from the aggregate effects of all allelic variants (Wray et al., 2007; Purcell et al., 2009; Vilhjálmsson et al., 2015; Chun et al., 2018). The observation that individuals with extreme values of polygenic scores exhibit many-fold elevated risk of common diseases raises hopes for their potential clinical utility (Ganna et al., 2013; Khera et al., 2018), and use for sociogenomics applications (Lee et al., 2018; Savage et al., 2018; Nagel et al., 2018). It is already clear that polygenic scores derived from European populations do not translate across populations on a global scale (Martin et al., 2017). Our analysis further suggests that subtle population structure, especially in GWAS that are meta-analyses of independent cohorts, could be an additional source of error in polygenic scores and affect their applicability even within populations. We also note that other factors such as gene by environment interactions can be an alternative confounding factor for GWAS effect sizes and polygenic scores.

Materials and methods

Genome-wide association studies (GWAS)

Request a detailed protocol

We analyzed height using publicly available summary statistics that were obtained either by meta-analysis of multiple GWAS or by a GWAS performed on a single large population. We used results from the GIANT Consortium (N = 253,288) (Wood et al., 2014) and a GWAS performed on individuals of the UK Biobank (‘UKB Neale’ or simply ‘UK Biobank (UKB)', N = 336,474) (Churchhouse et al., 2017) who derive their ancestry almost entirely from the British Isles (identified as ‘white British ancestry (WB)’ by the UK Biobank). The Neale lab’s GWAS uses a linear model with sex and 10 principal components as covariates. We also used an independent GWAS that included all UK Biobank European samples, allowing related individuals as well as population structure (‘UKB Loh’, N = 459,327) (Loh et al., 2018). Loh et al.’s GWAS uses a BOLT-LMM Bayesian mixed model (Loh et al., 2018). Association signals from the three studies are generally correlated for SNPs that are genome-wide significant in GIANT (see Yengo et al., 2018).

We also used previously published family-based effect size estimates (Robinson et al., 2015) (‘NG2015 sibs’) as well as a number of test summary statistics on the UK Biobank that we generated to study the effects of population stratification. These are: ‘UKB Neale new’ (Similar to UKB Neale, with less stringent ancestry definition and 20 PCs calculated within sample), ‘UKB all no PCs’ (All UK Biobank samples included in the GWAS without correction by principal components), ‘UKB all 10 PCs’ (All UK Biobank samples included in the GWAS with correction by 10 principal components), ‘UK WB no PCs’ (Only ‘white British ancestry’ samples included in the GWAS without correction by principal components), ‘UKB WB 10 PCs’ (Only ‘white British ancestry’ samples included in the GWAS with correction by 10 principal components), ‘UKB sibs all’ (All UK Biobank siblings included in the GWAS), ‘UKB sibs WB’ (Only UK Biobank ‘white British ancestry’ siblings included in the GWAS) (Please see Supplementary file 1 for sample sizes and other details).

Population genetic data for ancient and modern samples

Request a detailed protocol

We analyzed ancient and modern populations for which genotype data are publicly available. For ancient samples (Haak et al., 2015; Mathieson et al., 2018), we computed scores after dividing populations into three previously described broad ancestry labels (HG = Hunter Gatherer (n = 162 individuals), EF = Early Farmer (n = 485 individuals), and SP = Steppe Ancestry (n = 465 individuals)). For modern samples available through the 1000 genomes phase three release (Auton et al., 2015), we computed scores in two populations each from Northern Europe (GBR, CEU), Southern Europe (IBS, TSI), Africa (YRI, LWK), South Asia (PJL, BEB) and East Asia (CHB, JPT) (Figure 1a). In total, we analyzed 1112 ancient individuals, and 1005 modern individuals from 10 different populations in the 1000 genomes project (Supplementary file 2). We used the allele frequency differences between the GBR and TSI populations for a number of analyses to study population stratification (Figures 2–3). We also analyzed 19 European populations from the POPRES (Nelson et al., 2008) dataset with at least 10 samples per population (Figure 4—figure supplement 4).

All ancient samples had ‘pseudo-haploid’ genotype calls at 1240k sites generated by selecting a single sequence randomly for each individual at each SNP (Mathieson et al., 2018). Thus, there is only a single allele from each individual at each site, but adjacent alleles might come from either of the two haplotypes of the individual. We also re-computed scores in present-day 1000 genomes individuals using only pseudo-haploid calls at 1240 k sites to allow for a fair comparison between ancient and modern samples (Figure 1—figure supplement 6).

Polygenic scores

Request a detailed protocol

The polygenic scores, confidence intervals and test statistics (against the null model of genetic drift) were computed based on the methodology developed in references Berg and Coop, 2014 and Berg et al., 2017. We computed the polygenic score (Z) for a trait in a population by taking the sum of allele frequencies in that population across all L sites associated with the trait, weighting each allele’s frequency (pl) by its effect on the trait (βl).

Z= ∑lLβlpl

Al polygenic scores are plotted in centered standardized form (Z-μVA),

where μ=∑lβlpl-, VA=∑lβl2pl-(1-pl-), and pl- is the mean allele frequency across all populations analyzed. Source code repositories for the polygenic score analysis and computing scripts and source data for all the main figures have been made available at https://github.com/msohail88/polygenic_selection (Sohail, 2018; copy archived at https://github.com/elifesciences-publications/polygenic_selection) and https://github.com/uqrmaie1/sohail_maier_2019 (Sohail, 2019; copy archived at https://github.com/elifesciences-publications/sohail_maier_2019).

Polygenic scores were computed using independent GWAS SNPs associated with height in three main ways: (1) The genome was divided into ~1700 non-overlapping linkage disequilibrium (LD) blocks (using the approximately independent linkage disequilibrium blocks in the EUR population computed in Berisa and Pickrell, 2015), and the SNP with the lowest P value within each block was picked to give a set of ~1700 independent SNPs for each height GWAS used (all SNPs for which effect sizes are available were considered) similar to the analysis in Berg et al., 2017. In (2) and (3), Plink’s (Chang et al., 2015; Purcell and Chang, 2015) clumping procedure was used to make independent ‘clumps’ of SNPs for each GWAS at different P value thresholds. This procedure selects SNPs below a given P value threshold as index SNPs to start clumps around, and then reduces all SNPs below a given P value threshold that are in LD with these index SNPs (above an r2 threshold, 0.1) and within a physical distance of them (1 Mb) into clumps with them. Clumps are preferentially formed around index SNPs with the lowest P value in a greedy manner. The index SNP from each clump is then picked for further polygenic score analyses. The algorithm is also greedy such that each SNP will only appear in one clump if at all. We clumped each GWAS to obtain (2) a set of independent sub-significant SNPs associated with height (p<0.01) similarly to Robinson et al. (2015), and (3) a set of independent genome-wide significant SNPs associated with height (p<5×10−8). The 1000 genomes phase three dataset was used as the reference panel for computing LD for the clumping procedure.

The estimated effect sizes for these three sets of SNPs from each GWAS was used to compute scores. Only autosomal SNPs were used for all analyses to avoid creating artificial mean differences between populations with different numbers of males and females.

The 95% credible intervals were constructed by assuming that the posterior of the underlying population allele frequency is independent across loci and populations and follows a beta distribution. We updated a Uniform prior distribution with allele counts from ancient and modern populations to obtain the posterior distribution at each locus in each population. We estimated the variance of the polygenic score VZ using the variance of the posterior distribution at each locus, and computed the width of 95% credible intervals as 1.96VZ for each population.

The Qx test statistic measures the degree of overdispersion of the mean population polygenic score compared to a null model of genetic drift. It assumes that the vector of mean centered mean population polygenic score follows a multivariate normal distribution: Z ~ MVN(0, 2 VA F), where VA is the additive genetic variance of the ancestral population and F is a square matrix describing the population structure. This is equivalent to the univariate case of the test statistic used in Robinson et al. (2015). The latitude test statistic assumes that Y’Z ~ N(0, 2 VA Y’FY), where Y is a mean centered vector of latitudes for each population (Berg et al., 2019).

tSDS analysis

Request a detailed protocol

The Singleton Density Score (SDS) method identifies signatures of recent positive selection based on a maximum likelihood estimate of the log-ratio of the mean tip-branch length of the derived vs. the ancestral allele at a given SNP. The tip-branch lengths are inferred from the average distance of each allele to the nearest singleton SNP across all individuals in a sequencing panel. When the sign of the SDS scores is aligned with the trait-increasing or trait-decreasing allele in the effect estimates of a GWAS, the Spearman correlation between the resulting tSDS scores and the GWAS P values has been proposed as an estimate of recent positive selection on polygenic traits.

Here, we applied the tSDS method using pre-computed Singleton Density Scores for 4,451,435 autosomal SNPs obtained from 3195 individuals from the UK10K project (Field et al., 2016a; Field et al., 2016b) for SNPs associated with height in GIANT and the UK biobank (Figure 1b) and in different summary statistics (Figure 3). After normalizing SDS scores in each 1% allele frequency bin to mean zero and unit variance, excluding SNPs from the MHC region on chromosome six and aligning the sign of the SDS scores to the height increasing alleles (resulting in tSDS scores), we computed the Spearman correlation coefficient between the tSDS score and the GWAS P value. The tSDS Spearman correlation standard errors and P values were computed using a block-jackknife approach, where each block of 1% of all SNPs ordered by genomic location was left out and the Spearman correlation coefficient was computed on the remaining SNPs. We also compared the tSDS score distributions for only genome-wide significant SNPs (Figure 3b).

Population structure analysis

Request a detailed protocol

To compute SNP loadings of the principal components of population structure (PC loadings) in the 1000 genomes data (Figure 2), we first computed PC scores for each individual. We used SNPs that had matching alleles in 1000 genomes, GIANT and UK Biobank, that had minor allele frequency >5% in 1000 genomes, and that were not located in the MHC locus, the chromosome eight inversion region, or regions of long LD. After LD pruning to SNPs with r2 <0.2 relative to each other, PCA was performed in PLINK on the 187,160 remaining SNPs. In order to get SNP PC loadings for more SNPs than those that were used to compute PC scores, we performed linear regressions of the PC scores on the genotype allele count of each SNP (after controlling for sex) and used the resulting regression coefficients as the SNP PC loading estimates. The 1000 genomes phase one dataset (Abecasis et al., 2012) was used to compute the PC loadings.

Data availability

All newly generated UK Biobank height GWAS summary statistics have been made available at http://dx.doi.org/10.5061/dryad.8g5g6j4. Results from the GIANT Consortium (GWAS Anthropometric 2014 Height) were downloaded from https://portals.broadinstitute.org/collaboration/giant/index.php/GIANT_consortium_data_files#GWAS_Anthropometric_2014_Height. GWAS results from the UK Biobank ("UKB" or "UKB Neale") were downloaded from http://www.nealelab.is/uk-biobank. The previously published family-based effect size estimates ("NG2015 sibs") can be accessed here http://cnsgenomics.com/data/robinson_et_al_2015_ng/withinfam_summary_ht_bmi_release_March2016.tar.gz. The independent mixed model association analysis that included all UK Biobank individuals of European ancestry ("UKB Loh") was downloaded from https://data.broadinstitute.org/alkesgroup/UKBB/body_HEIGHTz.sumstats.gz. Approximately independent linkage disequilibrium blocks in human populations were downloaded for the EUR population from https://bitbucket.org/nygcresearch/ldetect-data/overview. Source code repositories for the polygenic score analysis in this manuscript and computing scripts and source data for all the main figures have been made available at https://github.com/msohail88/polygenic_selection and https://github.com/uqrmaie1/sohail_maier_2019 (copies archived at https://github.com/elifesciences-publications/polygenic_selection and https://github.com/elifesciences-publications/sohail_maier_2019, respectively).

The following data sets were generated
    1. Sohail M
    2. Maier RM
    3. Ganna A
    (2018) Dryad Digital Repository
    Data from: Polygenic adaptation on height is overestimated due to uncorrected stratification in genome-wide association studies.
    https://doi.org/10.5061/dryad.8g5g6j4

References

    1. Lango Allen H
    2. Estrada K
    3. Lettre G
    4. Berndt SI
    5. Weedon MN
    6. Rivadeneira F
    7. Willer CJ
    8. Jackson AU
    9. Vedantam S
    10. Raychaudhuri S
    11. Ferreira T
    12. Wood AR
    13. Weyant RJ
    14. Segrè AV
    15. Speliotes EK
    16. Wheeler E
    17. Soranzo N
    18. Park JH
    19. Yang J
    20. Gudbjartsson D
    21. Heard-Costa NL
    22. Randall JC
    23. Qi L
    24. Vernon Smith A
    25. Mägi R
    26. Pastinen T
    27. Liang L
    28. Heid IM
    29. Luan J
    30. Thorleifsson G
    31. Winkler TW
    32. Goddard ME
    33. Sin Lo K
    34. Palmer C
    35. Workalemahu T
    36. Aulchenko YS
    37. Johansson A
    38. Zillikens MC
    39. Feitosa MF
    40. Esko T
    41. Johnson T
    42. Ketkar S
    43. Kraft P
    44. Mangino M
    45. Prokopenko I
    46. Absher D
    47. Albrecht E
    48. Ernst F
    49. Glazer NL
    50. Hayward C
    51. Hottenga JJ
    52. Jacobs KB
    53. Knowles JW
    54. Kutalik Z
    55. Monda KL
    56. Polasek O
    57. Preuss M
    58. Rayner NW
    59. Robertson NR
    60. Steinthorsdottir V
    61. Tyrer JP
    62. Voight BF
    63. Wiklund F
    64. Xu J
    65. Zhao JH
    66. Nyholt DR
    67. Pellikka N
    68. Perola M
    69. Perry JR
    70. Surakka I
    71. Tammesoo ML
    72. Altmaier EL
    73. Amin N
    74. Aspelund T
    75. Bhangale T
    76. Boucher G
    77. Chasman DI
    78. Chen C
    79. Coin L
    80. Cooper MN
    81. Dixon AL
    82. Gibson Q
    83. Grundberg E
    84. Hao K
    85. Juhani Junttila M
    86. Kaplan LM
    87. Kettunen J
    88. König IR
    89. Kwan T
    90. Lawrence RW
    91. Levinson DF
    92. Lorentzon M
    93. McKnight B
    94. Morris AP
    95. Müller M
    96. Suh Ngwa J
    97. Purcell S
    98. Rafelt S
    99. Salem RM
    100. Salvi E
    101. Sanna S
    102. Shi J
    103. Sovio U
    104. Thompson JR
    105. Turchin MC
    106. Vandenput L
    107. Verlaan DJ
    108. Vitart V
    109. White CC
    110. Ziegler A
    111. Almgren P
    112. Balmforth AJ
    113. Campbell H
    114. Citterio L
    115. De Grandi A
    116. Dominiczak A
    117. Duan J
    118. Elliott P
    119. Elosua R
    120. Eriksson JG
    121. Freimer NB
    122. Geus EJ
    123. Glorioso N
    124. Haiqing S
    125. Hartikainen AL
    126. Havulinna AS
    127. Hicks AA
    128. Hui J
    129. Igl W
    130. Illig T
    131. Jula A
    132. Kajantie E
    133. Kilpeläinen TO
    134. Koiranen M
    135. Kolcic I
    136. Koskinen S
    137. Kovacs P
    138. Laitinen J
    139. Liu J
    140. Lokki ML
    141. Marusic A
    142. Maschio A
    143. Meitinger T
    144. Mulas A
    145. Paré G
    146. Parker AN
    147. Peden JF
    148. Petersmann A
    149. Pichler I
    150. Pietiläinen KH
    151. Pouta A
    152. Ridderstråle M
    153. Rotter JI
    154. Sambrook JG
    155. Sanders AR
    156. Schmidt CO
    157. Sinisalo J
    158. Smit JH
    159. Stringham HM
    160. Bragi Walters G
    161. Widen E
    162. Wild SH
    163. Willemsen G
    164. Zagato L
    165. Zgaga L
    166. Zitting P
    167. Alavere H
    168. Farrall M
    169. McArdle WL
    170. Nelis M
    171. Peters MJ
    172. Ripatti S
    173. van Meurs JB
    174. Aben KK
    175. Ardlie KG
    176. Beckmann JS
    177. Beilby JP
    178. Bergman RN
    179. Bergmann S
    180. Collins FS
    181. Cusi D
    182. den Heijer M
    183. Eiriksdottir G
    184. Gejman PV
    185. Hall AS
    186. Hamsten A
    187. Huikuri HV
    188. Iribarren C
    189. Kähönen M
    190. Kaprio J
    191. Kathiresan S
    192. Kiemeney L
    193. Kocher T
    194. Launer LJ
    195. Lehtimäki T
    196. Melander O
    197. Mosley TH
    198. Musk AW
    199. Nieminen MS
    200. O'Donnell CJ
    201. Ohlsson C
    202. Oostra B
    203. Palmer LJ
    204. Raitakari O
    205. Ridker PM
    206. Rioux JD
    207. Rissanen A
    208. Rivolta C
    209. Schunkert H
    210. Shuldiner AR
    211. Siscovick DS
    212. Stumvoll M
    213. Tönjes A
    214. Tuomilehto J
    215. van Ommen GJ
    216. Viikari J
    217. Heath AC
    218. Martin NG
    219. Montgomery GW
    220. Province MA
    221. Kayser M
    222. Arnold AM
    223. Atwood LD
    224. Boerwinkle E
    225. Chanock SJ
    226. Deloukas P
    227. Gieger C
    228. Grönberg H
    229. Hall P
    230. Hattersley AT
    231. Hengstenberg C
    232. Hoffman W
    233. Lathrop GM
    234. Salomaa V
    235. Schreiber S
    236. Uda M
    237. Waterworth D
    238. Wright AF
    239. Assimes TL
    240. Barroso I
    241. Hofman A
    242. Mohlke KL
    243. Boomsma DI
    244. Caulfield MJ
    245. Cupples LA
    246. Erdmann J
    247. Fox CS
    248. Gudnason V
    249. Gyllensten U
    250. Harris TB
    251. Hayes RB
    252. Jarvelin MR
    253. Mooser V
    254. Munroe PB
    255. Ouwehand WH
    256. Penninx BW
    257. Pramstaller PP
    258. Quertermous T
    259. Rudan I
    260. Samani NJ
    261. Spector TD
    262. Völzke H
    263. Watkins H
    264. Wilson JF
    265. Groop LC
    266. Haritunians T
    267. Hu FB
    268. Kaplan RC
    269. Metspalu A
    270. North KE
    271. Schlessinger D
    272. Wareham NJ
    273. Hunter DJ
    274. O'Connell JR
    275. Strachan DP
    276. Wichmann HE
    277. Borecki IB
    278. van Duijn CM
    279. Schadt EE
    280. Thorsteinsdottir U
    281. Peltonen L
    282. Uitterlinden AG
    283. Visscher PM
    284. Chatterjee N
    285. Loos RJ
    286. Boehnke M
    287. McCarthy MI
    288. Ingelsson E
    289. Lindgren CM
    290. Abecasis GR
    291. Stefansson K
    292. Frayling TM
    293. Hirschhorn JN
    (2010) Hundreds of variants clustered in genomic loci and biological pathways affect human height
    Nature 467:832–838.
    https://doi.org/10.1038/nature09410
  32. Software
    1. Purcell S
    2. Chang C
    (2015)
    PLINK 1
    GigaScience.
    1. Wood AR
    2. Esko T
    3. Yang J
    4. Vedantam S
    5. Pers TH
    6. Gustafsson S
    7. Chu AY
    8. Estrada K
    9. Luan J
    10. Kutalik Z
    11. Amin N
    12. Buchkovich ML
    13. Croteau-Chonka DC
    14. Day FR
    15. Duan Y
    16. Fall T
    17. Fehrmann R
    18. Ferreira T
    19. Jackson AU
    20. Karjalainen J
    21. Lo KS
    22. Locke AE
    23. Mägi R
    24. Mihailov E
    25. Porcu E
    26. Randall JC
    27. Scherag A
    28. Vinkhuyzen AA
    29. Westra HJ
    30. Winkler TW
    31. Workalemahu T
    32. Zhao JH
    33. Absher D
    34. Albrecht E
    35. Anderson D
    36. Baron J
    37. Beekman M
    38. Demirkan A
    39. Ehret GB
    40. Feenstra B
    41. Feitosa MF
    42. Fischer K
    43. Fraser RM
    44. Goel A
    45. Gong J
    46. Justice AE
    47. Kanoni S
    48. Kleber ME
    49. Kristiansson K
    50. Lim U
    51. Lotay V
    52. Lui JC
    53. Mangino M
    54. Mateo Leach I
    55. Medina-Gomez C
    56. Nalls MA
    57. Nyholt DR
    58. Palmer CD
    59. Pasko D
    60. Pechlivanis S
    61. Prokopenko I
    62. Ried JS
    63. Ripke S
    64. Shungin D
    65. Stancáková A
    66. Strawbridge RJ
    67. Sung YJ
    68. Tanaka T
    69. Teumer A
    70. Trompet S
    71. van der Laan SW
    72. van Setten J
    73. Van Vliet-Ostaptchouk JV
    74. Wang Z
    75. Yengo L
    76. Zhang W
    77. Afzal U
    78. Arnlöv J
    79. Arscott GM
    80. Bandinelli S
    81. Barrett A
    82. Bellis C
    83. Bennett AJ
    84. Berne C
    85. Blüher M
    86. Bolton JL
    87. Böttcher Y
    88. Boyd HA
    89. Bruinenberg M
    90. Buckley BM
    91. Buyske S
    92. Caspersen IH
    93. Chines PS
    94. Clarke R
    95. Claudi-Boehm S
    96. Cooper M
    97. Daw EW
    98. De Jong PA
    99. Deelen J
    100. Delgado G
    101. Denny JC
    102. Dhonukshe-Rutten R
    103. Dimitriou M
    104. Doney AS
    105. Dörr M
    106. Eklund N
    107. Eury E
    108. Folkersen L
    109. Garcia ME
    110. Geller F
    111. Giedraitis V
    112. Go AS
    113. Grallert H
    114. Grammer TB
    115. Gräßler J
    116. Grönberg H
    117. de Groot LC
    118. Groves CJ
    119. Haessler J
    120. Hall P
    121. Haller T
    122. Hallmans G
    123. Hannemann A
    124. Hartman CA
    125. Hassinen M
    126. Hayward C
    127. Heard-Costa NL
    128. Helmer Q
    129. Hemani G
    130. Henders AK
    131. Hillege HL
    132. Hlatky MA
    133. Hoffmann W
    134. Hoffmann P
    135. Holmen O
    136. Houwing-Duistermaat JJ
    137. Illig T
    138. Isaacs A
    139. James AL
    140. Jeff J
    141. Johansen B
    142. Johansson Å
    143. Jolley J
    144. Juliusdottir T
    145. Junttila J
    146. Kho AN
    147. Kinnunen L
    148. Klopp N
    149. Kocher T
    150. Kratzer W
    151. Lichtner P
    152. Lind L
    153. Lindström J
    154. Lobbens S
    155. Lorentzon M
    156. Lu Y
    157. Lyssenko V
    158. Magnusson PK
    159. Mahajan A
    160. Maillard M
    161. McArdle WL
    162. McKenzie CA
    163. McLachlan S
    164. McLaren PJ
    165. Menni C
    166. Merger S
    167. Milani L
    168. Moayyeri A
    169. Monda KL
    170. Morken MA
    171. Müller G
    172. Müller-Nurasyid M
    173. Musk AW
    174. Narisu N
    175. Nauck M
    176. Nolte IM
    177. Nöthen MM
    178. Oozageer L
    179. Pilz S
    180. Rayner NW
    181. Renstrom F
    182. Robertson NR
    183. Rose LM
    184. Roussel R
    185. Sanna S
    186. Scharnagl H
    187. Scholtens S
    188. Schumacher FR
    189. Schunkert H
    190. Scott RA
    191. Sehmi J
    192. Seufferlein T
    193. Shi J
    194. Silventoinen K
    195. Smit JH
    196. Smith AV
    197. Smolonska J
    198. Stanton AV
    199. Stirrups K
    200. Stott DJ
    201. Stringham HM
    202. Sundström J
    203. Swertz MA
    204. Syvänen AC
    205. Tayo BO
    206. Thorleifsson G
    207. Tyrer JP
    208. van Dijk S
    209. van Schoor NM
    210. van der Velde N
    211. van Heemst D
    212. van Oort FV
    213. Vermeulen SH
    214. Verweij N
    215. Vonk JM
    216. Waite LL
    217. Waldenberger M
    218. Wennauer R
    219. Wilkens LR
    220. Willenborg C
    221. Wilsgaard T
    222. Wojczynski MK
    223. Wong A
    224. Wright AF
    225. Zhang Q
    226. Arveiler D
    227. Bakker SJ
    228. Beilby J
    229. Bergman RN
    230. Bergmann S
    231. Biffar R
    232. Blangero J
    233. Boomsma DI
    234. Bornstein SR
    235. Bovet P
    236. Brambilla P
    237. Brown MJ
    238. Campbell H
    239. Caulfield MJ
    240. Chakravarti A
    241. Collins R
    242. Collins FS
    243. Crawford DC
    244. Cupples LA
    245. Danesh J
    246. de Faire U
    247. den Ruijter HM
    248. Erbel R
    249. Erdmann J
    250. Eriksson JG
    251. Farrall M
    252. Ferrannini E
    253. Ferrières J
    254. Ford I
    255. Forouhi NG
    256. Forrester T
    257. Gansevoort RT
    258. Gejman PV
    259. Gieger C
    260. Golay A
    261. Gottesman O
    262. Gudnason V
    263. Gyllensten U
    264. Haas DW
    265. Hall AS
    266. Harris TB
    267. Hattersley AT
    268. Heath AC
    269. Hengstenberg C
    270. Hicks AA
    271. Hindorff LA
    272. Hingorani AD
    273. Hofman A
    274. Hovingh GK
    275. Humphries SE
    276. Hunt SC
    277. Hypponen E
    278. Jacobs KB
    279. Jarvelin MR
    280. Jousilahti P
    281. Jula AM
    282. Kaprio J
    283. Kastelein JJ
    284. Kayser M
    285. Kee F
    286. Keinanen-Kiukaanniemi SM
    287. Kiemeney LA
    288. Kooner JS
    289. Kooperberg C
    290. Koskinen S
    291. Kovacs P
    292. Kraja AT
    293. Kumari M
    294. Kuusisto J
    295. Lakka TA
    296. Langenberg C
    297. Le Marchand L
    298. Lehtimäki T
    299. Lupoli S
    300. Madden PA
    301. Männistö S
    302. Manunta P
    303. Marette A
    304. Matise TC
    305. McKnight B
    306. Meitinger T
    307. Moll FL
    308. Montgomery GW
    309. Morris AD
    310. Morris AP
    311. Murray JC
    312. Nelis M
    313. Ohlsson C
    314. Oldehinkel AJ
    315. Ong KK
    316. Ouwehand WH
    317. Pasterkamp G
    318. Peters A
    319. Pramstaller PP
    320. Price JF
    321. Qi L
    322. Raitakari OT
    323. Rankinen T
    324. Rao DC
    325. Rice TK
    326. Ritchie M
    327. Rudan I
    328. Salomaa V
    329. Samani NJ
    330. Saramies J
    331. Sarzynski MA
    332. Schwarz PE
    333. Sebert S
    334. Sever P
    335. Shuldiner AR
    336. Sinisalo J
    337. Steinthorsdottir V
    338. Stolk RP
    339. Tardif JC
    340. Tönjes A
    341. Tremblay A
    342. Tremoli E
    343. Virtamo J
    344. Vohl MC
    345. Amouyel P
    346. Asselbergs FW
    347. Assimes TL
    348. Bochud M
    349. Boehm BO
    350. Boerwinkle E
    351. Bottinger EP
    352. Bouchard C
    353. Cauchi S
    354. Chambers JC
    355. Chanock SJ
    356. Cooper RS
    357. de Bakker PI
    358. Dedoussis G
    359. Ferrucci L
    360. Franks PW
    361. Froguel P
    362. Groop LC
    363. Haiman CA
    364. Hamsten A
    365. Hayes MG
    366. Hui J
    367. Hunter DJ
    368. Hveem K
    369. Jukema JW
    370. Kaplan RC
    371. Kivimaki M
    372. Kuh D
    373. Laakso M
    374. Liu Y
    375. Martin NG
    376. März W
    377. Melbye M
    378. Moebus S
    379. Munroe PB
    380. Njølstad I
    381. Oostra BA
    382. Palmer CN
    383. Pedersen NL
    384. Perola M
    385. Pérusse L
    386. Peters U
    387. Powell JE
    388. Power C
    389. Quertermous T
    390. Rauramaa R
    391. Reinmaa E
    392. Ridker PM
    393. Rivadeneira F
    394. Rotter JI
    395. Saaristo TE
    396. Saleheen D
    397. Schlessinger D
    398. Slagboom PE
    399. Snieder H
    400. Spector TD
    401. Strauch K
    402. Stumvoll M
    403. Tuomilehto J
    404. Uusitupa M
    405. van der Harst P
    406. Völzke H
    407. Walker M
    408. Wareham NJ
    409. Watkins H
    410. Wichmann HE
    411. Wilson JF
    412. Zanen P
    413. Deloukas P
    414. Heid IM
    415. Lindgren CM
    416. Mohlke KL
    417. Speliotes EK
    418. Thorsteinsdottir U
    419. Barroso I
    420. Fox CS
    421. North KE
    422. Strachan DP
    423. Beckmann JS
    424. Berndt SI
    425. Boehnke M
    426. Borecki IB
    427. McCarthy MI
    428. Metspalu A
    429. Stefansson K
    430. Uitterlinden AG
    431. van Duijn CM
    432. Franke L
    433. Willer CJ
    434. Price AL
    435. Lettre G
    436. Loos RJ
    437. Weedon MN
    438. Ingelsson E
    439. O'Connell JR
    440. Abecasis GR
    441. Chasman DI
    442. Goddard ME
    443. Visscher PM
    444. Hirschhorn JN
    445. Frayling TM
    446. Electronic Medical Records and Genomics (eMEMERGEGE) ConsortiumMIGen Consortium PAGEGE ConsortiumLifeLines Cohort Study
    (2014) Defining the role of common variation in the genomic and biological architecture of adult human height
    Nature Genetics 46:1173–1186.
    https://doi.org/10.1038/ng.3097

Article and author information

Author details

  1. Mashaal Sohail

    1. Division of Genetics, Department of Medicine, Brigham and Women’s Hospital and Harvard Medical School, Boston, United States
    2. Department of Biomedical Informatics, Harvard Medical School, Boston, United States
    3. Program in Medical and Population Genetics, Broad Institute of MIT and Harvard, Cambridge, United States
    Present address
    National Laboratory of Genomics for Biodiversity (UGA-LANGEBIO), Cinvestav, Irapuato, Mexico
    Contribution
    Conceptualization, Formal analysis, Investigation, Visualization, Methodology, Writing—original draft, Writing—review and editing
    Contributed equally with
    Robert M Maier, Benjamin Neale, Iain Mathieson, David Reich and Shamil R Sunyaev
    For correspondence
    mashaal33@gmail.com
    Competing interests
    No competing interests declared
    ORCID icon "This ORCID iD identifies the author of this article:" 0000-0002-6586-4403

  2. Robert M Maier

    1. Program in Medical and Population Genetics, Broad Institute of MIT and Harvard, Cambridge, United States
    2. Stanley Center for Psychiatric Research, Broad Institute of MIT and Harvard, Cambridge, United States
    3. Analytical and Translational Genetics Unit, Massachusetts General Hospital, Boston, United States
    Contribution
    Conceptualization, Formal analysis, Investigation, Visualization, Methodology, Writing—original draft, Writing—review and editing
    Contributed equally with
    Mashaal Sohail, Benjamin Neale, Iain Mathieson, David Reich and Shamil R Sunyaev
    For correspondence
    rmaier@broadinstitute.org
    Competing interests
    No competing interests declared
    ORCID icon "This ORCID iD identifies the author of this article:" 0000-0002-3044-090X

  3. Andrea Ganna

    1. Program in Medical and Population Genetics, Broad Institute of MIT and Harvard, Cambridge, United States
    2. Stanley Center for Psychiatric Research, Broad Institute of MIT and Harvard, Cambridge, United States
    3. Analytical and Translational Genetics Unit, Massachusetts General Hospital, Boston, United States
    4. Department of Medical Epidemiology and Biostatistics, Karolinska Institutet, Stockholm, Sweden
    5. Institute for Molecular Medicine Finland, University of Helsinki, Helsinki, Finland
    Contribution
    Formal analysis, Writing—review and editing
    For correspondence
    aganna@broadinstitute.org
    Competing interests
    No competing interests declared

  4. Alex Bloemendal

    1. Program in Medical and Population Genetics, Broad Institute of MIT and Harvard, Cambridge, United States
    2. Stanley Center for Psychiatric Research, Broad Institute of MIT and Harvard, Cambridge, United States
    3. Analytical and Translational Genetics Unit, Massachusetts General Hospital, Boston, United States
    Contribution
    Methodology, Writing—review and editing
    For correspondence
    bloem@broadinstitute.org
    Competing interests
    No competing interests declared

  5. Alicia R Martin

    1. Program in Medical and Population Genetics, Broad Institute of MIT and Harvard, Cambridge, United States
    2. Stanley Center for Psychiatric Research, Broad Institute of MIT and Harvard, Cambridge, United States
    3. Analytical and Translational Genetics Unit, Massachusetts General Hospital, Boston, United States
    Contribution
    Data curation, Writing—review and editing
    For correspondence
    armartin@broadinstitute.org
    Competing interests
    No competing interests declared

  6. Michael C Turchin

    1. Center for Computational Molecular Biology, Brown University, Providence, United States
    2. Department of Ecology and Evolutionary Biology, Brown University, Providence, United States
    Contribution
    Validation, Writing—review and editing
    For correspondence
    michael_turchin@brown.edu
    Competing interests
    No competing interests declared
    ORCID icon "This ORCID iD identifies the author of this article:" 0000-0003-3569-1529

  7. Charleston WK Chiang

    Department of Preventive Medicine, Center for Genetic Epidemiology, Keck School of Medicine, University of Southern California, Los Angeles, United States
    Contribution
    Validation, Writing—review and editing
    Competing interests
    No competing interests declared
    ORCID icon "This ORCID iD identifies the author of this article:" 0000-0002-0668-7865

  8. Joel Hirschhorn

    1. Program in Medical and Population Genetics, Broad Institute of MIT and Harvard, Cambridge, United States
    2. Departments of Pediatrics and Genetics, Harvard Medical School, Boston, United States
    3. Division of Endocrinology and Center for Basic and Translational Obesity Research, Boston Children’s Hospital, Boston, United States
    Contribution
    Validation, Methodology, Writing—review and editing
    For correspondence
    joel.hirschhorn@childrens.harvard.edu
    Competing interests
    No competing interests declared

  9. Mark J Daly

    1. Program in Medical and Population Genetics, Broad Institute of MIT and Harvard, Cambridge, United States
    2. Stanley Center for Psychiatric Research, Broad Institute of MIT and Harvard, Cambridge, United States
    3. Analytical and Translational Genetics Unit, Massachusetts General Hospital, Boston, United States
    4. Institute for Molecular Medicine Finland, University of Helsinki, Helsinki, Finland
    Contribution
    Methodology, Writing—review and editing
    For correspondence
    mjdaly@atgu.mgh.harvard.edu
    Competing interests
    No competing interests declared

  10. Nick Patterson

    1. Program in Medical and Population Genetics, Broad Institute of MIT and Harvard, Cambridge, United States
    2. Department of Genetics, Harvard Medical School, Boston, United States
    Contribution
    Methodology, Writing—review and editing
    For correspondence
    nickp@broadinstitute.org
    Competing interests
    No competing interests declared

  11. Benjamin Neale

    1. Program in Medical and Population Genetics, Broad Institute of MIT and Harvard, Cambridge, United States
    2. Stanley Center for Psychiatric Research, Broad Institute of MIT and Harvard, Cambridge, United States
    3. Analytical and Translational Genetics Unit, Massachusetts General Hospital, Boston, United States
    Contribution
    Supervision, Visualization, Methodology, Writing—review and editing
    Contributed equally with
    Mashaal Sohail, Robert M Maier, Iain Mathieson, David Reich and Shamil R Sunyaev
    For correspondence
    bneale@broadinstitute.org
    Competing interests
    Ben Neale is a member and on the scientific advisory board of Deep Genomics, a consultant for Camp4 Therapeutics Corporation, a consultant for Merck & Co., a consultant for Takeda Phamaceutical, and a consultant for Avanir Pharmaceuticals. None of these entities played a role in determining the content of this paper.

  12. Iain Mathieson

    Department of Genetics, Perelman School of Medicine, University of Pennsylvania, Philadelphia, United States
    Contribution
    Data curation, Supervision, Investigation, Methodology, Writing—review and editing
    Contributed equally with
    Mashaal Sohail, Robert M Maier, Benjamin Neale, David Reich and Shamil R Sunyaev
    For correspondence
    mathi@pennmedicine.upenn.edu
    Competing interests
    No competing interests declared

  13. David Reich

    1. Program in Medical and Population Genetics, Broad Institute of MIT and Harvard, Cambridge, United States
    2. Department of Genetics, Harvard Medical School, Boston, United States
    3. Howard Hughes Medical Institute, Harvard Medical School, Boston, United States
    Contribution
    Conceptualization, Supervision, Visualization, Methodology, Writing—original draft, Project administration, Writing—review and editing
    Contributed equally with
    Mashaal Sohail, Robert M Maier, Benjamin Neale, Iain Mathieson and Shamil R Sunyaev
    For correspondence
    reich@genetics.med.harvard.edu
    Competing interests
    No competing interests declared

  14. Shamil R Sunyaev

    1. Department of Biomedical Informatics, Harvard Medical School, Boston, United States
    2. Program in Medical and Population Genetics, Broad Institute of MIT and Harvard, Cambridge, United States
    3. Division of Genetics, Department of Medicine, Brigham and Women’s Hospital and Harvard Medical School, Boston, United States
    Contribution
    Conceptualization, Supervision, Methodology, Writing—original draft, Project administration, Writing—review and editing
    Contributed equally with
    Mashaal Sohail, Robert M Maier, Benjamin Neale, Iain Mathieson and David Reich
    For correspondence
    ssunyaev@rics.bwh.harvard.edu
    Competing interests
    No competing interests declared
    ORCID icon "This ORCID iD identifies the author of this article:" 0000-0001-5715-5677

Funding

National Institutes of Health (HG009088)

  • Mashaal Sohail
  • Robert M Maier
  • Benjamin Neale
  • Shamil R Sunyaev

National Institutes of Health (MH101244)

  • Mashaal Sohail
  • Robert M Maier
  • Benjamin Neale
  • Shamil R Sunyaev

Alfred P. Sloan Foundation (Sloan Research Fellowship)

  • Iain Mathieson

Charles E Kaufman Foundation (New Investigator Research Grant)

  • Iain Mathieson

Paul Allen Foundation (Allen Discovery Center)

  • David Reich

National Institutes of Health (GM100233)

  • David Reich

National Institutes of Health (HG006399)

  • David Reich

Howard Hughes Medical Institute (Investigator)

  • David Reich

National Institutes of Health (GM127131)

  • Shamil R Sunyaev

The funders had no role in study design, data collection and interpretation, or the decision to submit the work for publication.

Acknowledgements

We thank Alkes Price, Jeremy Berg, Graham Coop, Jonathan Pritchard, Matthew Robinson, Jian Yang, Peter Visscher, Hilary Finucane, John Novembre and Raymond Walters for useful discussions and comments that significantly improved the manuscript. The study was supported by National Institutes of Health grants HG009088, MH101244 (MS, RM, BN and SS) and GM127131 (SS). DR was supported by National Institutes of Health grants GM100233 and HG006399, an Allen Discovery Center grant from the Paul Allen Foundation, and the Howard Hughes Medical Institute. IM was supported by a Sloan Research Fellowship and a New Investigator Research Grant from the Charles E Kaufman foundation.

This research was conducted using the UK Biobank Resource applications 18597, 11898 and 31063.

Copyright

© 2019, Sohail et al.

This article is distributed under the terms of the Creative Commons Attribution License, which permits unrestricted use and redistribution provided that the original author and source are credited.

Metrics

  • 13,430
    views
  • 1,288
    downloads
  • 275
    citations

Views, downloads and citations are aggregated across all versions of this paper published by eLife.

Download links

A two-part list of links to download the article, or parts of the article, in various formats.

Downloads (link to download the article as PDF)

Open citations (links to open the citations from this article in various online reference manager services)

Cite this article (links to download the citations from this article in formats compatible with various reference manager tools)

  1. Mashaal Sohail
  2. Robert M Maier
  3. Andrea Ganna
  4. Alex Bloemendal
  5. Alicia R Martin
  6. Michael C Turchin
  7. Charleston WK Chiang
  8. Joel Hirschhorn
  9. Mark J Daly
  10. Nick Patterson
  11. Benjamin Neale
  12. Iain Mathieson
  13. David Reich
  14. Shamil R Sunyaev
(2019)
Polygenic adaptation on height is overestimated due to uncorrected stratification in genome-wide association studies
eLife 8:e39702.
https://doi.org/10.7554/eLife.39702

Share this article

https://doi.org/10.7554/eLife.39702

Categories and tags

Research organism

Further reading

    1. Evolutionary Biology
    2. Genetics and Genomics

    Reduced signal for polygenic adaptation of height in UK Biobank

    Jeremy J Berg, Arbel Harpak ... Graham Coop
    Research Communication

    Several recent papers have reported strong signals of selection on European polygenic height scores. These analyses used height effect estimates from the GIANT consortium and replication studies. Here, we describe a new analysis based on the the UK Biobank (UKB), a large, independent dataset. We find that the signals of selection using UKB effect estimates are strongly attenuated or absent. We also provide evidence that previous analyses were confounded by population stratification. Therefore, the conclusion of strong polygenic adaptation now lacks support. Moreover, these discrepancies highlight (1) that methods for correcting for population stratification in GWAS may not always be sufficient for polygenic trait analyses, and (2) that claims of differences in polygenic scores between populations should be treated with caution until these issues are better understood.

    Editorial note: This article has been through an editorial process in which the authors decide how to respond to the issues raised during peer review. The Reviewing Editor's assessment is that all the issues have been addressed (see decision letter).

    1. Evolutionary Biology
    2. Genetics and Genomics

    Population Genetics: Why structure matters

    Nick Barton, Joachim Hermisson, Magnus Nordborg
    Insight

    Great care is needed when interpreting claims about the genetic basis of human variation based on data from genome-wide association studies.

    1. Genetics and Genomics

    Quantitative Genetics: A Collection of Articles

    Edited by Patricia Wittkopp et al.
    Collection

    eLife publishes advances in quantitative genetics, including the genetic basis of complex traits, the maintenance of genetic variation, and their roles in evolution.